Interest Rate Volatility
|
|
|
- Luke Pierce
- 8 years ago
- Views:
Transcription
1 Interest Rate Volatility I. Volatility in fixed income markets Andrew Lesniewski Baruch College and Posnania Inc First Baruch Volatility Workshop New York June 16-18, 2015
2 Outline Linear interest rate derivatives 1 Linear interest rate derivatives 2 3
3 Fixed income markets Debt and debt related markets, also known as fixed income markets, account for the lion share of the world s financial markets. Instruments trading in these markets fall into the categories of cash instruments, or bonds, and derivative instruments, such as interest rate swaps, bond futures and credit default swaps. Bonds are debt instruments issued by various entities (such as sovereigns, corporations, municipalities, US Government Sponsored Enterprizes, etc), with the purposes of raising funds in the capital markets. Derivatives are synthetic instruments which extract specific features of commonly traded cash instruments. Depending on their purpose, fixed income instruments may exhibit very complex risk profiles. The value of a fixed income instrument may depend on the level and term structure of interest rates, credit characteristics of the underlying entity, foreign exchange levels, or prepayment speeds of the collateral pool of loans. In these lectures we focus on modeling volatility of interest rate derivatives and its implications for quantifying risk inherent to these instruments.
4 LIBOR and OIS Like all other financial markets, fixed income markets fluctuate, and the main driver of this variability is the current perception of future interest rates. Market participants have adopted the convention that a rally refers to falling interest rates, while a sell off refers to rising rates. Each fixed income instrument is a stream of known or contingent cash flows. Such cash flows are typically periodic, and are computed as a fixed or floating coupon, applied to a principal (or a notional principal). Much of the activity in the world capital markets is tied to the LIBOR rates. They are widely used as benchmarks for short term (overnight to 1 year) interest rates. A LIBOR (= London Interbank Offered Rate) rate is the interest rate at which banks offer (at least in principle) unsecured deposits to each other. Daily fixings of LIBOR are published by Thompson Reuters on behalf of the British Banking Association (BBA) on each London business day. These fixings are calculated from the quotes provided by a panel of participating banks. The details on the composition of the panels and how the fixings are calculated can found on the web site of the BBA.
5 LIBOR based instruments There is a variety of vanilla LIBOR based derivative instruments that are actively trading both on exchanges and over the counter (OTC): (i) LIBOR futures, (ii) forward rate agreements, (iii) interest rate swaps. Each of these instruments serves as an underlying for an option. Below we summarize the mechanics of these instruments without discussing their economic significance.
6 Forward rate agreements Forward rate agreements (FRAs) are OTC transactions, i.e. they are arranged between the counterparties without an involvement of an exchange. In a FRA transaction, counterparty A agrees to pay counterparty B LIBOR settling t years from now applied to a specified notional amount (say, $100 mm). In exchange, counterparty B pays counterparty A a pre-agreed interest rate (say, 3.05%) applied to the same notional. The contract matures on an anniversary T (say, 3 months) of the settlement date, and interest is computed on an act/360 day count basis. Anniversary dates generally follow the modified following business day convention. FRAs are quoted in terms of the annualized forward interest rate applied to the accrual period of the transaction.
7 Eurodollar futures Eurodollar futures, known also as the LIBOR futures, are exchange traded futures contracts on the 3 month LIBOR rate. They trade on the Chicago Mercantile Exchange, which also clears and settles the trades. In many ways, Eurodollar futures are similar to FRAs, except that their terms, such as contract sizes and settlement dates are standardized. Each of the contracts assumes a notional principal of $1,000,000. Interest on these contracts is computed on an act/360 day count basis assuming 90 day accrual period. In order to make a Eurodollar future resemble a bond, the market has adopted the convention according to which the forward rate R underlying the contract is quoted in terms of the price defined as 100 (1 R). For example, if R = 2.32%, the quoted price of the contract is Unlike a FRA, the Eurodollar future quoted price is linear in the underlying rate.
8 Eurodollar futures Ticker Price Open Int Volume EDZ EDF EDG EDH EDJ EDK EDM EDU EDZ EDH EDM EDU EDZ EDH EDM EDU EDZ EDH EDM EDU EDZ EDH EDM EDU Table: 1. Snapshot of the Eurodollar futures market
9 Swaps Linear interest rate derivatives A fixed for floating interest rate swap (or simply: a swap) is an OTC transaction in which two counterparties agree to exchange periodic interest payments on a pre-specified notional amount. One counterparty (the fixed payer) agrees to pay periodically the other counterparty (the fixed receiver) a fixed coupon (say, 3.35% per annum) in exchange for receiving periodic LIBOR applied to the same notional. Spot starting swaps based on LIBOR begin on a start date 2 business days from the current date and mature and pay interest on anniversary dates that use the same modified following business day conventions as the LIBOR index. Interest is usually computed on an act/360 day basis on the floating side of the swap and on 30/360 day basis in the fixed side of the pay. Typically, fixed payment dates ( coupon dates ) are semiannual (every 6 months), and floating payment dates are quarterly (every 3 months) to correspond to a 3 month LIBOR. In addition to spot starting swaps, forward starting swaps are routinely traded. In a forward starting swap, the first accrual period can be any business day beyond spot. Swaps (spot and forward starting) are quoted in terms of the fixed coupon.
10 Swaps Linear interest rate derivatives Table 2 below contains a snapshot of the swap rate market. Each rate is the break even rate on the swap of indicated tenor paying coupon semiannually on the 30/360 basis, versus receiving 3 month LIBOR. Tenor Rate (%) 2Y 0.690% 3Y 0.798% 4Y 1.008% 5Y 1.248% 7Y 1.690% 10Y 2.106% 12Y 2.298% 15Y 2.478% 20Y 2.599% 25Y 2.660% 30Y 2.694% Table: 2. Snapshot of the swap market taken on 12/13/2011
11 OIS Linear interest rate derivatives In the wake of the 2008 credit crunch, LIBOR s credibility as a funding rate was put to question. Part of the issue is the de facto absence of the interbank unsecured lending market which raises doubts over the validity of the quotes submitted by the participating banks to the BBA. As a result, rates referred to as the OIS rates, linked to the overnight rate controlled by the local (to the currency) central bank became increasingly important as benchmark funding rates. OIS stands for overnight indexed swap. An overnight indexed swap is a fixed for floating interest rate swap where the floating rate is based on a short term (overnight), daily compounded (rather quarterly, as in a LIBOR swap) interest rate. By default, both the fixed and floating legs accrue based on the act/360 basis. OIS swaps tend to be of short maturity, ranging from a few days to five years. In the USD market, OIS rates are calculated by reference to daily fed funds effective rate.
12 LIBOR / OIS basis The LIBOR / OIS spread, defined as the difference between the 3 month LIBOR and 3 month OIS rates, is an important indicator of stress in the capital markets. A wider spread is an indication of a decreased willingness to lend by major banks, while a tighter spread indicates easier availability of credit. The LIBOR / OIS spread is a gauge of market participants view of the credit worthiness of other financial institutions and the general availability of funds for lending purposes. The LIBOR / OIS spread has been historically hovering around 10 basis points. However, at times of elevated credit stress, the basis between LIBOR and OIS can be quite volatile. In the midst of the financial crisis that started in 2007, the spread between LIBOR and OIS was wildly volatile and peeked at an all-time high of 364 basis points in October 2008, indicating a severe credit crunch.
13 Multi-curve paradigm and valuation of swaps We now turn to the problem of the valuation of non-contingent (but not necessarily known) future cash flows. The building blocks required are: (i) Discount factors, which allow one to calculate present value of cash received in the future. (ii) Forward rates, which allow one to make assumptions as to the future levels of interest rates. Until 2008, it was common practice to use LIBOR as both the discount rate, i.e. the interest rate used for calculating the discount factors, as well as the index rate, i.e. the rate used as the forward rate. Since then, in the wake of the financial crisis, the industry has been steadily moving away from this practice, and adopted the multicurve paradigm to swap valuation. Since OIS is a better indicator of the costs of funding, it is used for discounting, while LIBOR is the index rate. It remains to be seen whether the fed funds effective rate will retain this role in the USD market.
14 Zero coupon bonds A zero coupon bond (or discount bond) is a cash instrument which pays a predefined principal amount, say $1, at a specified future date. More precisely, a zero coupon bond is characterized by two dates, the settlement date S which marks the start of the accrual period, and the maturity date T > S on which the payment is made. Its value at settlement is thus the present value (abbreviated PV) of $1 guaranteed to be paid at time T. In practice we are interested in the value P(t, S, T ) of the forward zero coupon bond for any valuation date t S. It is thus the time t value of zero coupon bond (whose face value is $1) which settles on the date S years from now and matures in T years. The forward zero coupon bond P (t, S, T ) is also called the (forward) discount factor. There is a useful no arbitrage relationship involving P(t, S, T ), namely: P (t, S, T ) = P (t, t, T ) P (t, t, S). (1) Throughout these presentations we adopt the following convention. If the valuation date is today, t = 0, then we denote the price of the zero coupon bond by P 0 (S, T ), i.e. P 0 (S, T ) P(0, S, T ). (2)
15 Forward rates The OIS forward rate F (t, S, T ) for start S and maturity T, as observed at time t, is defined as F (t, S, T ) = 1 ( 1 ) δ P(t, S, T ) 1, (3) where δ denotes the day count factor for the period [S, T ]. The discount factor P(t, S, T ) can be expressed in terms of F (t, S, T ) by means of the formula: 1 P(t, S, T ) = 1 + δf (t, S, T ). The LIBOR forward rate for start S and maturity T, as observed at time t, is denoted by L(t, S, T ). The LIBOR / OIS spread B(t, S, T ) is given by B(t, S, T ) = L(t, S, T ) F (t, S, T ). (4)
16 Forward rates Discount factors can be expressed in terms of the continuously compounded instantaneous forward rate f (t, s). For all practical purposes, we can think about f (t, s) as the forward overnight OIS rate. In terms of f (t, s), ( T ) P(t, S, T ) = exp f (t, s)ds. (5) S This equation is merely the definition of f (t, s), and expresses the discount factor as the result of continuous discounting of the value of a dollar between the value and maturity dates. Conversely, the instantaneous forward rate can be computed from the discount factor: 1 f (t, s) = P(t, S, T ) P(t, S, T ) T = T log P(t, S, T ) T =s. T =s (6)
17 Valuation of swaps We consider a swap which settles at T 0 0 and matures at T. We assume that the notional principal is $1. Let T c 1 <... < T c n c = T denote the coupon dates of the swap, and let 0 t T 0 denote the valuation date. The PV of the interest payments on the fixed leg of a swap is calculated by adding up the PVs of all future cash flows: n c P0 fix (t) = α j CP 0 (t, Tj c ), (7) j=1 where C is the coupon rate, P 0 (t, T c j ) are the discount factors to the valuation date, and α j are the day count fractions on the fixed leg. For example, on a standard USD swap paying semi-annual coupon, the α s correspond to the modified following 30/360 business day convention. It is useful to write this formula as where A 0 (t) = is the annuity function of the swap. P fix 0 (t) = CA 0 (t), (8) 1 j n c α j P 0 (t, T c j ), (9)
18 Valuation of swaps Likewise, let T1 f <... < T n f f = T denote the LIBOR payment dates of the swap. The valuation formula for the swap s floating leg reads then: P0 float (t) = δ j L j P 0 (t, Tj f ). (10) 1 j n f Here L j = L 0 (T f j 1, T f j ) (11) is the LIBOR forward rate for settlement at T f j 1, and δ j is the day count fraction applying to the floating leg. In the USD, the payments are quarterly, and the δ s correspond to the modified following act/360 business day convention. The PV of a swap is the difference between the PVs of the fixed and floating legs: P 0 (t) = P0 fix (t) Pfloat 0 (t). A break-even (or mid-market) swap has zero PV: P0 fix (t) = Pfloat 0 (t). That uniquely determines the break-even swap rate: S 0 (T 0, T ) = Pfloat 0 (t). (12) A 0 (t)
19 Vanilla interest rate options There are several types of liquidly traded options on interest rates: (i) Caps / floors: these are options on LIBOR forwards. (ii) Eurodollar options, which are options on Eurodollar futures. (iii) Swaptions, which are options on swaps Below we summarize some basic facts about these instruments and market conventions for their pricing.
20 Caps and floors Caps and floors are baskets of European calls (called caplets) and puts (called floorlets) on LIBOR forward rates. They trade over the counter. Let us consider, for example, a 10 year spot starting cap struck at 2.50%. It consists of 39 caplets each of which expires on the 3 month anniversary of today s date. A caplet pays max(current LIBOR fixing 2.50%, 0) act/360 day count fraction. The payment is made at the end of the 3 month period covered by the LIBOR contract, and follows the modified business day convention. Notice that the very first period is excluded from the cap: this is because the current LIBOR fixing is already known and no optionality is left in that period.
21 Caps and floors In addition to spot starting caps and floors, forward starting instruments are traded. For example, a 1 year 5 years (in the market lingo: 1 by 5 ) cap struck at 2.50% consists of 16 caplets struck at 2.50%, the first of which matures one year from today. The final maturity of the contract is 5 years, meaning that the last caplets matures 4 years and 9 months from today (with appropriate business dates adjustments). Unlike the case of spot starting caps, the first period is included into the structure, as the first LIBOR fixing is unknown. Note that the total maturity of the m n cap is n years. The definitions of floors are similar with the understanding that a floorlet pays max(strike current LIBOR fixing, 0) act/360 day count fraction at the end of the corresponding period.
22 Eurodollar options Eurodollar options are standardized contracts traded at the Merc. These are short dated American style calls and puts on Eurodollar futures. At each time, options on the first eight quarterly Eurodollar futures contracts and on two front serial futures are listed. Their expirations coincide with the maturity dates of the underlying Eurodollar contracts. The exchange sets the strikes for the options spaced every 25 basis points (or 12.5 bp for the front contracts). The options are cash settled. Strike Calls Puts Table: 3. ED options: March 2012 expirations. Price of the underlying
23 Eurodollar options In addition to the quarterly and serial contracts, a number of midcurve options are listed on the Merc. These are American style calls and puts with expirations between three months and one year on longer dated Eurodollar futures. Their expirations do not coincide with the maturity on the underlying futures contracts, which mature one, two, or four years later. The prices of all Eurodollar options are quoted in ticks. Strike Calls Puts Table: 4. ED options: June 2012 expirations. Price of the underlying 99.31
24 Swaptions European swaptions are European calls and puts (in the market lingo they are called receivers and payers, respectively) on interest rate swaps. A holder of a payer swaption has the right, upon exercise, to pay fixed coupon on a swap of contractually defined terms. Likewise, a holder of a receiver swaption has the right to receive fixed on a swap. Swaptions are traded over the counter. For example, a 2.50% 1Y 5Y ( 1 into 5 ) receiver swaption gives the holder the right to receive 2.50% on a 5 year swap starting in 1 year. More precisely, the option holder has the right to exercise the option on the 1 year anniversary of today (with the usual business day convention adjustments) in which case they enter into a receiver swap starting two business days thereafter. Similarly, a 3.50% 5Y 10Y ( 5 into 10 ) payer swaption gives the holder the right to pay 3.50% on a 10 year swap starting in 5 years. Note that the total maturity of the m n swaption is m + n years. Since a swap can be viewed as a particular basket of underlying LIBOR forwards, a swaption is an option on a basket of forwards.
25 Black s model The market quotes the prices on interest rate options is in terms of Black s model. We assume that a forward rate F (t), such as a LIBOR forward or a forward swap rate (do not confuse with the OIS rate!), follows a driftless lognormal process, df (t) = σf (t) dw (t). (13) Here W (t) is a Wiener process, and σ is the lognormal volatility. It is understood here, that we have chosen a numeraire N with the property that, in the units of that numeraire, F (t) is a tradable asset. The process F (t) is thus a martingale, and we let Q denote the corresponding measure. The prices of calls and puts are given by the Black-Scholes formulas: P call (T, K, F 0, σ) = N (0) [ F 0 N(d +) KN(d ) ], N (0) B call (T, K, F 0, σ), P put (T, K, F 0, σ) = N (0) [ F 0 N( d +) + KN( d ) ] N (0) B put (T, K, F 0, σ). Here, N (x) is the cumulative normal distribution, and ( d ± = log F 0 K ± 1 ) 2 σ2 T /σ T. (15) (14)
26 Valuation of caps and floors A cap is a basket of options on LIBOR forward rates. Consider the OIS forward rate F (S, T ) spanning the accrual period [S, T ]. Its time t S value F (t, S, T ) can be expressed in terms of discount factors: F (t, S, T ) = 1 δ = 1 δ ( ) P(t, t, S) P(t, t, T ) 1 P(t, t, S) P(t, t, T ) P(t, t, T ) The interpretation of this identity is that F (t, S, T ) is a tradable asset if we use the zero coupon bond maturing in T years as numeraire. Indeed, the trade is as follows: (i) Buy 1/δ face value of the zero coupon bond for maturity S. (ii) Sell 1/δ face value of the zero coupon bond for maturity T. The value of this position in the units of P(t, t, T ) is F (t, S, T ). An OIS forward rate can thus be modeled as a martingale! We call the corresponding martingale measure the T -forward measure and denote it by Q T.. (16)
27 Deterministic basis assumption Consider now a LIBOR forward L(S, T ) spanning the same accrual period. Throughout these presentations we assume that the LIBOR / OIS spread is deterministic (rather than stochastic). This assumption is, clearly, a gross oversimplification of reality but it has some merits. There are no liquidly trading options on this spread, and thus calibrating a model with a stochastic spread is problematic. From the conceptual point of view, the picture is more transparent with a deterministic spread. Namely, we know from the discussion above that L(t, S, T ) = F (t, S, T ) + B(t, S, T ) = 1 δ P(t, t, S) P(t, t, T ) + δb(t, S, T )P(t, t, T ) P(t, t, T ). (17) This shows that the LIBOR forward is a martingale under the T -forward measure Q T.
28 Deterministic basis assumption This assumption generalizes the deterministic shift extension idea [1] to multi-curve context (as well as multi-factor modeling). In this framework, the discount rate and index rate are allowed to be different. We recognize the fact that fluctuations of the funding rate and index rate do not generally share the same set of risk factors [5], and the basis spread between LIBOR rate and OIS rate is, in fact, stochastic [6], [4]. There are, however, valid reasons (in addition to simplicity) that the common set of risk factors assumption is defensible as a working assumption: (i) The basis LIBOR / OIS remains positive. (ii) Liquid volatility products on the basis LIBOR / OIS are not available, and making the basis spread stochastic results in non-calibrateable and non-hedgeable parameters.
29 Valuation of caps and floors Choosing, for now, the underlying process to be lognormal (given by (13)), we conclude that the price of a call on L(S, T ) (or caplet) is given by P caplet (T, K, L 0, σ) = δp 0 (0, T )B call (S, K, L 0, σ), (18) where L 0 denotes here today s value of the forward, namely L(0, S, T ) = L 0 (S, T ). Since a cap is a basket of caplets, its value is the sum of the values of the constituent caplets: P cap = n δ j B call (T j 1, K, L j, σ j )P 0 (0, T j ), (19) j=1 where δ j is the day count fraction applying to the accrual period starting at T j 1 and ending at T j, and L j is the LIBOR forward rate for that period. Notice that, in the formula above, the date T j 1 has to be adjusted to accurately reflect the expiration date of the option (2 business days before the start of the accrual period). Similarly, the value of a floor is P floor = n δ j B put (T j 1, K, L j, σ j )P 0 (0, T j ). (20) j=1
30 Valuation of swaptions Consider a swap that settles at T 0 and matures at T. Let S(t, T 0, T ) denote the corresponding (break-even) forward swap rate observed at time t < T 0. We know from the discussion above that the forward swap rate is given by S(t, T 0, T ) = 1 j n δ f j L j P(t, T val, Tj f ), (21) A(t, T val, T 0, T ) where T val T 0 is the valuation date of the swap. Here, B j is the LIBOR / OIS spread, and A(t, T val, T 0, T ) is the forward annuity function: A(t, T val, T 0, T ) = α j P(t, T val, Tj c ). (22) 1 j n c We can write S(t, T 0, T ) as S(t, T 0, T ) = P(t, t, T 0) P(t, t, T ) + 1 j n δ f j B j P(t, t, Tj f). (23) A(t, t, T 0, T ) The forward annuity function A(t, t, T 0, T ) is the time t present value of an annuity paying $1 on the dates T c 1,..., T c n c, as observed at t.
31 Valuation of swaptions The interpretation of (23) is that S(t, T 0, T ) is a tradable asset if we use the annuity as numeraire (recall that we are assuming that all the LIBOR / OIS spreads are deterministic). Indeed, consider the following trade: (i) Buy $1 face value of the zero coupon bond for maturity T 0. (ii) Sell $1 face value of the zero coupon bond for maturity T. (iii) Buy a stream of δ j B j face value zero coupon bonds for each maturity T f j. A forward swap rate can thus be modeled as a martingale! The corresponding martingale measure is called the swap measure. Choosing, again, the lognormal process (13), we conclude that today s value of a receiver and payer swaptions are given by P rec = A 0 (T 0, T )B put (T 0, K, S 0, σ), P pay = A 0 (T 0, T )B call (T 0, K, S 0, σ), respectively. Here A 0 (T 0, T ) = A(0, 0, T 0, T ), i.e. A 0 (T 0, T ) = α j P 0 (0, Tj c ) (25) 1 j n c (all discounting is done to today), and S 0 is today s value of the forward swap rate S 0 (T 0, T ). (24)
32 Beyond Black s model The basic premise of Black s model, that σ is independent of T, K, and F 0, is not supported by the interest rates markets. In fact, option implied volatilities exhibit: (i) Term structure: At the money volatility depends on the option expiration. (ii) Smile (or skew): For a given expiration, there is a pronounced dependence of implied volatilities on the option strike. These phenomena became pronounced in the mid nineties or so and, in order to accurately value and risk manage options portfolios, refinements to Black s model are necessary. Modeling term structure of volatility is hard, and not much progress has been made. We will discuss some empirical facts later in this presentation. Our main focus will be on modeling volatility smile.
33 Local volatility models A class of models extending Black s model, called local volatility models, are specified as follows: df (t) = C(t, F (t))dw (t), (26) where C(t, F ) is a certain effective instantaneous volatility. The idea is that even though the exact nature of volatility (it could be stochastic) is unknown, one can, in principle, use the market prices of options in order to recover the risk neutral probability distribution of the underlying asset. To see this, note that d dk (F K )+ = d ( ) (F K )θ(f K ) dk = θ(f K ) (F K )δ(f K ) = θ(f K ), and thus d 2 dk 2 (F K )+ = δ(f K ).
34 Local volatility models This implies that d 2 dk 2 EQ [(F T K ) + ] = E Q [δ(f T K )]. Let g T (F, F 0 ) denote the terminal probability distribution function of the forward swap rate S. From the equality above we infer that d 2 dk 2 EQ [(F T K ) + ] = δ(f K )g T (F, F 0 )df. = g T (K, F 0 ). Consequently, the terminal probability distribution can (in principle) be computed from the option prices [3]. This, in turn, will allow us to find an effective ( local ) specification C(t, F ) of the underlying process so that the implied volatilities match the market implied volatilities. Empirical studies (see e.g. [2]) show that depending on the level of rates, their dynamics may be more akin to that of a lognormal model (very low or very high rates) or a normal model (intermediate range).
35 Normal model The dynamics for the forward rate F (t) in the normal model reads df (t) = σdw (t), (27) under a suitable choice of numeraire. The parameter σ is appropriately called the normal volatility. Prices of European calls and puts are now given by: P call (T, K, F 0, σ) = N (0) σ T ( d +N(d +) + N (d +) ), N (0) B call n P put (T, K, F 0, σ) = N (0) σ T (T, K, F 0, σ), ( d N (d ) + N (d ) N (0) Bn put (T, K, F 0, σ), ) (28) where d ± = ± F 0 K σ T. (29) The normal model is (in addition to the lognormal model) an important benchmark in terms of which implied volatilities are quoted.
36 CEV model The CEV model (for constant elasticity of variance ), interpolates smoothly between the normal and lognormal models: df (t) = σf (t) β dw (t), (30) where β < 1. In order for the dynamics to be well defined, we have to prevent F (t) from becoming negative (otherwise F (t) β would turn imaginary!). To this end, we specify a boundary condition at F = 0. It can be (i) Dirichlet (absorbing): F 0 = 0. Solution exists for all values of β, or (ii) Neumann (reflecting): F 0 = 0. Solution exists for 1 2 β < 1. The CEV model requires solving a terminal value problem for a partial differential equation, namely the backward Kolmogorov equation: t B(t, x) σ2 x B(T, x) = 2β 2 B(t, x) = 0, x 2 { (x K ) +, for a call, (K x) +, for a put, This equation has to be supplemented by a boundary condition at zero x. Pricing formulas for the CEV model can be obtained in a closed (albeit somewhat complicated) form. (31)
37 Swaption volatilities Table 5 contains the December 13, 2011 snapshot of the at the money swaption market. The rows in the matrix represent the swaption expiration and the columns represent the tenor of the underlying swap. Each entry in the table represents the premium of a swaption straddle expressed as a percentage of the notional on the underlying swap. 1Y 2Y 3Y 4Y 5Y 7Y 10Y 15Y 20Y 30Y 1M 0.06% 0.11% 0.18% 0.27% 0.37% 0.67% 1.10% 1.70% 2.17% 2.94% 3M 0.10% 0.20% 0.31% 0.48% 0.68% 1.18% 1.91% 2.90% 3.69% 5.02% 6M 0.14% 0.30% 0.47% 0.74% 1.04% 1.73% 2.71% 4.06% 5.17% 6.97% 1Y 0.21% 0.35% 0.75% 1.16% 1.60% 2.51% 3.82% 5.56% 7.05% 9.45% 2Y 0.40% 0.85% 1.37% 1.94% 2.55% 3.66% 5.26% 7.38% 9.23% 12.20% 3Y 0.62% 1.26% 1.91% 2.58% 3.25% 4.50% 6.26% 8.61% 10.64% 13.77% 4Y 0.78% 1.54% 2.28% 3.02% 3.75% 5.11% 7.00% 9.52% 11.66% 15.11% 5Y 0.88% 1.74% 2.56% 3.35% 4.13% 5.58% 7.57% 10.21% 12.49% 16.15% 7Y 0.97% 1.90% 2.78% 3.63% 4.44% 5.97% 8.09% 10.81% 13.16% 16.86% 10Y 1.01% 1.96% 2.86% 3.71% 4.53% 6.08% 8.22% 10.86% 13.12% 16.71% Table: 5. ATM swaption prices
38 Swaption volatilities Table 6 contains the implied normal volatilities corresponding to the swaption prices in Table 5. 1Y 2Y 3Y 4Y 5Y 7Y 10Y 15Y 20Y 30Y 1M M M Y Y Y Y Y Y Y Table: 6. Swaption ATM normal volatilities (in basis points)
39 Empirical dynamics of the ATM swaption volatilities Swaption volatilities exhibit a pronounced term structure both in their dependence on the expiration as well as in their dependence on the underlying tenor. This term structure has a persistent dynamics whose characteristics withstand market regime switches. We analyse the dynamics of the ATM swaption volatility matrix using recent market data, from January 01, 2003 through September 16, This time window includes the 2007 / 2008 financial crisis, as well as the pre-crisis and post-crisis periods. We include swaptions of maturities of 1M, 3M, 6M, 1Y, 2Y, 3Y, 4Y, 5Y, 7Y and 10Y, and underlying tenors ranging from 1Y to 30Y. Our presentation is based on the work of Shi [7].
40 Term structure of ATM swaption volatilities The term structure of ATM swaption volatilities can be well captured by the hump function : σ (t) = (at + b)e λt + µ. (32) The parameters of this function have clear intuitive meaning: (i) µ is the volatility at long expiry, as σ( ) = µ; (ii) b + µ is the instantaneous volatility level, since σ(0) = b + µ; (iii) a is the slope at short expiries; (iv) λ is the rate of exponential decay for long expiries. The hump function captures well the volatility regimes over the period January 2003 through September 2014, as illustrated by the graphs below. On these graphs, the black circles indicate market data, and the blue line is the fitted hump function.
41 Term structure of ATM swaption volatilities Figure 1 shows the shapes of the ATM swaption volatilities during the pre-crisis period. Note the hump in the short end (around 6M - 2Y). Figure: 1. Term structure ATM swaption volatilities on September 16, 2004.
42 Term structure of ATM swaption volatilities Figure 2 shows the shapes of the ATM swaption volatilities during the crisis period. Note the absence of the hump in the short end. Figure: 2. Term structure ATM swaption volatilities on September 16, 2008.
43 Term structure of ATM swaption volatilities Figure 3 shows the shapes of the ATM swaption volatilities during the post-crisis period. Note that the hump has reappeared. Figure: 3. Term structure ATM swaption volatilities on September 16, 2014.
44 Term structure of ATM swaption volatilities In the three graphs below we show the term structure of the parameters a, b, λ, µ as functions of the underlying tenor T. Each of the term structures can be fitted to a power function. We illustrate the dependence of the parameters on T separately for each of the market regimes: pre-crisis, crisis, and post-crisis. The values of the parameters are indicated by a green circle while the blue line is the fitted power function.
45 Term structure ATM swaption volatilities Figure: 4. Tenor dependence of the hump function parameters during the pre-crisis period.
46 Term structure ATM swaption volatilities Figure: 5. Tenor dependence of the hump function parameters during the crisis period.
47 Term structure ATM swaption volatilities Figure: 6. Tenor dependence of the hump function parameters during the pre-crisis period.
48 PCA of ATM swaption volatilities In order to identify the factors that drive the dynamics of volatility matrix, we perform principal component analysis (PCA) on the time series of the ATM swaption volatility matrices. For this purpose, each swaption volatility matrix is regarded a vector in a 100-dimensional space. The analysis was performed over three time windows: pre-crisis: from January 1, 2003 through December 31, 2003, crisis: from January 01, 2008 to December 31, 2008, post-crisis: from June 01, 2013 to May 31, Interestingly, the first three principal components (PC1, PC2, and PC3) together explain over 94% of the variance of the volatility matrix. The dominant principal component can be interpreted as parallel shift of the volatility matrix. The second and third components can be identified as tilts along the tenor and option expiration dimensions, respectively. This structure is present during each of the three market regimes.
49 PCA of ATM swaption volatilities During the pre-crisis period, 94.5% of the variance are explained by the three principal components, with PC1 explaining 77.1% of the variance. Figure: 7. PC1 of ATM swaption volatility in 2003.
50 PCA of ATM swaption volatilities PC2 explains 12.6% of the variance. It corresponds to a tilt along the tenor axis Figure: 8. PC2 of ATM swaption volatility in 2003.
51 PCA of ATM swaption volatilities PC3 explains 4.8% of the total variance. It corresponds to a tilt of the short dated volatility. Figure: 9. PC3 of ATM swaption volatility in 2003.
52 PCA of ATM swaption volatilities The three following graphs (Figures 10, 11, and 12) show a similar picture for the crisis period. The first three components explain 80.0%, 15.0% and 2.5% of the total variance, respectively, with a total of 97.5%. Figure: 10. PC1 of ATM swaption volatility in 2008.
53 PCA of ATM swaption volatilities Figure: 11. PC2 of ATM swaption volatility in 2008.
54 PCA of ATM swaption volatilities Figure: 12. PC3 of ATM swaption volatility in 2008.
55 PCA of ATM swaption volatilities Finally, Figures 13, 14, and 15 pertain to the post-crisis period. The first three components explain 77.8%, 14.5% and 4.4% of the variance, respectively, with a total of 96.8%. Figure: 13. PC1 of ATM swaption volatility from June 2013 to June 2014.
56 PCA of ATM swaption volatilities Figure: 14. PC2 of ATM swaption volatility from June 2013 to June 2014.
57 PCA of ATM swaption volatilities Figure: 15. PC3 of ATM swaption volatility from June 2013 to June 2014.
58 References Brigo, D., and Mercurio, F.: Interest Rate Models - Theory and Practice, Springer Verlag (2006). Deguillaume, N., Rebonato, R., and Pogudin, A.: The nature of the dependence of the magnitude of rate moves on the rates levels: a universal relationship, Quantitative Finance, 13, (2013). Dupire, B.: Pricing with a smile, Risk Magazine, July, (1994). Fujii, Y., Shimada, M., and Takahashi, A.: A market model of interest rates with dynamic basis spreads in the presence of collateral and multiple currencies, Wilmott Magazine, July, (2011). Kijima, K., Tanaka, K., and Wong, T.: A multi-quality model of interest rates, Quantitative Finance, 9, (2009). Mercurio, F., and Xie, Z.: The basis goes stochastic, Risk Magazine, December, (2012). Shi, F.: Empirical dynamics of the swaption volatility matrix (2014).
INTEREST RATES AND FX MODELS
 INTEREST RATES AND FX MODELS 4. Convexity and CMS Andrew Lesniewski Courant Institute of Mathematical Sciences New York University New York February 20, 2013 2 Interest Rates & FX Models Contents 1 Introduction
INTEREST RATES AND FX MODELS 4. Convexity and CMS Andrew Lesniewski Courant Institute of Mathematical Sciences New York University New York February 20, 2013 2 Interest Rates & FX Models Contents 1 Introduction
The TED spread trade: illustration of the analytics using Bloomberg
 The TED spread trade: illustration of the analytics using Bloomberg Aaron Nematnejad January 2003 1 The views, thoughts and opinions expressed in this article represent those of the author in his individual
The TED spread trade: illustration of the analytics using Bloomberg Aaron Nematnejad January 2003 1 The views, thoughts and opinions expressed in this article represent those of the author in his individual
Eurodollar Futures, and Forwards
 5 Eurodollar Futures, and Forwards In this chapter we will learn about Eurodollar Deposits Eurodollar Futures Contracts, Hedging strategies using ED Futures, Forward Rate Agreements, Pricing FRAs. Hedging
5 Eurodollar Futures, and Forwards In this chapter we will learn about Eurodollar Deposits Eurodollar Futures Contracts, Hedging strategies using ED Futures, Forward Rate Agreements, Pricing FRAs. Hedging
1. The forward curve
 1. The forward curve Andrew Lesniewsi January 28, 2008 Contents 1 LIBOR and LIBOR based instruments 1 1.1 Forward rate agreements...................... 2 1.2 LIBOR futures........................... 3
1. The forward curve Andrew Lesniewsi January 28, 2008 Contents 1 LIBOR and LIBOR based instruments 1 1.1 Forward rate agreements...................... 2 1.2 LIBOR futures........................... 3
Cash-settled swaptions How wrong are we?
 Cash-settled swaptions How wrong are we? Marc Henrard Quantitative Research - OpenGamma 7th Fixed Income Conference, Berlin, 7 October 2011 ... Based on: Cash-settled swaptions: How wrong are we? Available
Cash-settled swaptions How wrong are we? Marc Henrard Quantitative Research - OpenGamma 7th Fixed Income Conference, Berlin, 7 October 2011 ... Based on: Cash-settled swaptions: How wrong are we? Available
Manual for SOA Exam FM/CAS Exam 2.
 Manual for SOA Exam FM/CAS Exam 2. Chapter 7. Derivatives markets. c 2009. Miguel A. Arcones. All rights reserved. Extract from: Arcones Manual for the SOA Exam FM/CAS Exam 2, Financial Mathematics. Fall
Manual for SOA Exam FM/CAS Exam 2. Chapter 7. Derivatives markets. c 2009. Miguel A. Arcones. All rights reserved. Extract from: Arcones Manual for the SOA Exam FM/CAS Exam 2, Financial Mathematics. Fall
The Irony In The Derivatives Discounting
 The Irony In The Derivatives Discounting Marc Henrard Head of Quantitative Research, Banking Department, Bank for International Settlements, CH-4002 Basel (Switzerland), e-mail: Marc.Henrard@bis.org Abstract
The Irony In The Derivatives Discounting Marc Henrard Head of Quantitative Research, Banking Department, Bank for International Settlements, CH-4002 Basel (Switzerland), e-mail: Marc.Henrard@bis.org Abstract
The new ACI Diploma. Unit 2 Fixed Income & Money Markets. Effective October 2014
 The new ACI Diploma Unit 2 Fixed Income & Money Markets Effective October 2014 8 Rue du Mail, 75002 Paris - France T: +33 1 42975115 - F: +33 1 42975116 - www.aciforex.org The new ACI Diploma Objective
The new ACI Diploma Unit 2 Fixed Income & Money Markets Effective October 2014 8 Rue du Mail, 75002 Paris - France T: +33 1 42975115 - F: +33 1 42975116 - www.aciforex.org The new ACI Diploma Objective
Interest Rate and Currency Swaps
 Interest Rate and Currency Swaps Eiteman et al., Chapter 14 Winter 2004 Bond Basics Consider the following: Zero-Coupon Zero-Coupon One-Year Implied Maturity Bond Yield Bond Price Forward Rate t r 0 (0,t)
Interest Rate and Currency Swaps Eiteman et al., Chapter 14 Winter 2004 Bond Basics Consider the following: Zero-Coupon Zero-Coupon One-Year Implied Maturity Bond Yield Bond Price Forward Rate t r 0 (0,t)
INTEREST RATES AND FX MODELS
 INTEREST RATES AND FX MODELS 1. LIBOR and OIS Andrew Lesniewsi Courant Institute of Mathematical Sciences New Yor University New Yor January 26, 2012 2 Interest Rates & FX Models Contents 1 Introduction
INTEREST RATES AND FX MODELS 1. LIBOR and OIS Andrew Lesniewsi Courant Institute of Mathematical Sciences New Yor University New Yor January 26, 2012 2 Interest Rates & FX Models Contents 1 Introduction
INTEREST RATES AND FX MODELS
 INTEREST RATES AND FX MODELS 8. Portfolio greeks Andrew Lesniewski Courant Institute of Mathematical Sciences New York University New York March 27, 2013 2 Interest Rates & FX Models Contents 1 Introduction
INTEREST RATES AND FX MODELS 8. Portfolio greeks Andrew Lesniewski Courant Institute of Mathematical Sciences New York University New York March 27, 2013 2 Interest Rates & FX Models Contents 1 Introduction
Introduction to swaps
 Introduction to swaps Steven C. Mann M.J. Neeley School of Business Texas Christian University incorporating ideas from Teaching interest rate and currency swaps" by Keith C. Brown (Texas-Austin) and Donald
Introduction to swaps Steven C. Mann M.J. Neeley School of Business Texas Christian University incorporating ideas from Teaching interest rate and currency swaps" by Keith C. Brown (Texas-Austin) and Donald
The interest volatility surface
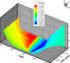 The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
Eris Interest Rate Swap Futures: Flex Contract Specifications
 Eris Interest Rate Swap Futures: Flex Contract Specifications Trading Hours Contract Structure Contract Size Trading Conventions Swap Futures Leg Conventions Effective Date Cash Flow Alignment Date ( CFAD
Eris Interest Rate Swap Futures: Flex Contract Specifications Trading Hours Contract Structure Contract Size Trading Conventions Swap Futures Leg Conventions Effective Date Cash Flow Alignment Date ( CFAD
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies
 Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
How To Understand A Rates Transaction
 International Swaps and Derivatives Association, Inc. Disclosure Annex for Interest Rate Transactions This Annex supplements and should be read in conjunction with the General Disclosure Statement. NOTHING
International Swaps and Derivatives Association, Inc. Disclosure Annex for Interest Rate Transactions This Annex supplements and should be read in conjunction with the General Disclosure Statement. NOTHING
 DERIVATIVES Presented by Sade Odunaiya Partner, Risk Management Alliance Consulting DERIVATIVES Introduction Forward Rate Agreements FRA Swaps Futures Options Summary INTRODUCTION Financial Market Participants
DERIVATIVES Presented by Sade Odunaiya Partner, Risk Management Alliance Consulting DERIVATIVES Introduction Forward Rate Agreements FRA Swaps Futures Options Summary INTRODUCTION Financial Market Participants
Bootstrapping the interest-rate term structure
 Bootstrapping the interest-rate term structure Marco Marchioro www.marchioro.org October 20 th, 2012 Bootstrapping the interest-rate term structure 1 Summary (1/2) Market quotes of deposit rates, IR futures,
Bootstrapping the interest-rate term structure Marco Marchioro www.marchioro.org October 20 th, 2012 Bootstrapping the interest-rate term structure 1 Summary (1/2) Market quotes of deposit rates, IR futures,
SWAPTION PRICING OPENGAMMA QUANTITATIVE RESEARCH
 SWAPTION PRICING OPENGAMMA QUANTITATIVE RESEARCH Abstract. Implementation details for the pricing of European swaptions in different frameworks are presented.. Introduction This note describes the pricing
SWAPTION PRICING OPENGAMMA QUANTITATIVE RESEARCH Abstract. Implementation details for the pricing of European swaptions in different frameworks are presented.. Introduction This note describes the pricing
Introduction to Eris Exchange Interest Rate Swap Futures
 Introduction to Eris Exchange Interest Rate Swap Futures Overview Eris Exchange interest rate swap futures ( Eris contracts ) have been designed to replicate the net cash flows associated with plain-vanilla,
Introduction to Eris Exchange Interest Rate Swap Futures Overview Eris Exchange interest rate swap futures ( Eris contracts ) have been designed to replicate the net cash flows associated with plain-vanilla,
Introduction to Options. Derivatives
 Introduction to Options Econ 422: Investment, Capital & Finance University of Washington Summer 2010 August 18, 2010 Derivatives A derivative is a security whose payoff or value depends on (is derived
Introduction to Options Econ 422: Investment, Capital & Finance University of Washington Summer 2010 August 18, 2010 Derivatives A derivative is a security whose payoff or value depends on (is derived
Interest rate Derivatives
 Interest rate Derivatives There is a wide variety of interest rate options available. The most widely offered are interest rate caps and floors. Increasingly we also see swaptions offered. This note will
Interest rate Derivatives There is a wide variety of interest rate options available. The most widely offered are interest rate caps and floors. Increasingly we also see swaptions offered. This note will
MONEY MARKET SUBCOMMITEE(MMS) FLOATING RATE NOTE PRICING SPECIFICATION
 MONEY MARKET SUBCOMMITEE(MMS) FLOATING RATE NOTE PRICING SPECIFICATION This document outlines the use of the margin discounting methodology to price vanilla money market floating rate notes as endorsed
MONEY MARKET SUBCOMMITEE(MMS) FLOATING RATE NOTE PRICING SPECIFICATION This document outlines the use of the margin discounting methodology to price vanilla money market floating rate notes as endorsed
PRICING OF SWAPS AND SWAPTIONS
 MSC. FINANCE MASTER THESIS DEP. OF ECONOMICS AND BUSINESS AARHUS MARCH 2013 PRICING OF SWAPS AND SWAPTIONS MSC. FINANCE STUDENT: MIKKEL KRONBORG OLESEN STUDENT NR.: 283755 SUPERVISOR: PETER LØCHTE JØRGENSEN
MSC. FINANCE MASTER THESIS DEP. OF ECONOMICS AND BUSINESS AARHUS MARCH 2013 PRICING OF SWAPS AND SWAPTIONS MSC. FINANCE STUDENT: MIKKEL KRONBORG OLESEN STUDENT NR.: 283755 SUPERVISOR: PETER LØCHTE JØRGENSEN
Caps and Floors. John Crosby
 Caps and Floors John Crosby Glasgow University My website is: http://www.john-crosby.co.uk If you spot any typos or errors, please email me. My email address is on my website Lecture given 19th February
Caps and Floors John Crosby Glasgow University My website is: http://www.john-crosby.co.uk If you spot any typos or errors, please email me. My email address is on my website Lecture given 19th February
Analytical Research Series
 EUROPEAN FIXED INCOME RESEARCH Analytical Research Series INTRODUCTION TO ASSET SWAPS Dominic O Kane January 2000 Lehman Brothers International (Europe) Pub Code 403 Summary An asset swap is a synthetic
EUROPEAN FIXED INCOME RESEARCH Analytical Research Series INTRODUCTION TO ASSET SWAPS Dominic O Kane January 2000 Lehman Brothers International (Europe) Pub Code 403 Summary An asset swap is a synthetic
Margin Calculation Methodology and Derivatives and Repo Valuation Methodology
 Margin Calculation Methodology and Derivatives and Repo Valuation Methodology 1 Overview This document presents the valuation formulas for interest rate derivatives and repo transactions implemented in
Margin Calculation Methodology and Derivatives and Repo Valuation Methodology 1 Overview This document presents the valuation formulas for interest rate derivatives and repo transactions implemented in
No-arbitrage conditions for cash-settled swaptions
 No-arbitrage conditions for cash-settled swaptions Fabio Mercurio Financial Engineering Banca IMI, Milan Abstract In this note, we derive no-arbitrage conditions that must be satisfied by the pricing function
No-arbitrage conditions for cash-settled swaptions Fabio Mercurio Financial Engineering Banca IMI, Milan Abstract In this note, we derive no-arbitrage conditions that must be satisfied by the pricing function
4. ANNEXURE 3 : PART 3 - FOREIGN EXCHANGE POSITION RISK
 Annexure 3 (PRR) - Part 3, Clause 18 - Foreign Exchange Position Risk Amount 4 ANNEXURE 3 : PART 3 - FOREIGN EXCHANGE POSITION RISK (a) CLAUSE 18 - FOREIGN EXCHANGE POSITION RISK AMOUNT (i) Rule PART 3
Annexure 3 (PRR) - Part 3, Clause 18 - Foreign Exchange Position Risk Amount 4 ANNEXURE 3 : PART 3 - FOREIGN EXCHANGE POSITION RISK (a) CLAUSE 18 - FOREIGN EXCHANGE POSITION RISK AMOUNT (i) Rule PART 3
ASSET LIABILITY MANAGEMENT Significance and Basic Methods. Dr Philip Symes. Philip Symes, 2006
 1 ASSET LIABILITY MANAGEMENT Significance and Basic Methods Dr Philip Symes Introduction 2 Asset liability management (ALM) is the management of financial assets by a company to make returns. ALM is necessary
1 ASSET LIABILITY MANAGEMENT Significance and Basic Methods Dr Philip Symes Introduction 2 Asset liability management (ALM) is the management of financial assets by a company to make returns. ALM is necessary
24. Pricing Fixed Income Derivatives. through Black s Formula. MA6622, Ernesto Mordecki, CityU, HK, 2006. References for this Lecture:
 24. Pricing Fixed Income Derivatives through Black s Formula MA6622, Ernesto Mordecki, CityU, HK, 2006. References for this Lecture: John C. Hull, Options, Futures & other Derivatives (Fourth Edition),
24. Pricing Fixed Income Derivatives through Black s Formula MA6622, Ernesto Mordecki, CityU, HK, 2006. References for this Lecture: John C. Hull, Options, Futures & other Derivatives (Fourth Edition),
Learning Curve Forward Rate Agreements Anuk Teasdale
 Learning Curve Forward Rate Agreements Anuk Teasdale YieldCurve.com 2004 Page 1 In this article we review the forward rate agreement. Money market derivatives are priced on the basis of the forward rate,
Learning Curve Forward Rate Agreements Anuk Teasdale YieldCurve.com 2004 Page 1 In this article we review the forward rate agreement. Money market derivatives are priced on the basis of the forward rate,
Fixed Income Portfolio Management. Interest rate sensitivity, duration, and convexity
 Fixed Income ortfolio Management Interest rate sensitivity, duration, and convexity assive bond portfolio management Active bond portfolio management Interest rate swaps 1 Interest rate sensitivity, duration,
Fixed Income ortfolio Management Interest rate sensitivity, duration, and convexity assive bond portfolio management Active bond portfolio management Interest rate swaps 1 Interest rate sensitivity, duration,
Guaranteed Annuity Options
 Guaranteed Annuity Options Hansjörg Furrer Market-consistent Actuarial Valuation ETH Zürich, Frühjahrssemester 2008 Guaranteed Annuity Options Contents A. Guaranteed Annuity Options B. Valuation and Risk
Guaranteed Annuity Options Hansjörg Furrer Market-consistent Actuarial Valuation ETH Zürich, Frühjahrssemester 2008 Guaranteed Annuity Options Contents A. Guaranteed Annuity Options B. Valuation and Risk
On the Valuation of Power-Reverse Duals and Equity-Rates Hybrids
 On the Valuation of Power-Reverse Duals and Equity-Rates Hybrids Oliver Caps oliver.caps@dkib.com RMT Model Validation Rates Dresdner Bank Examples of Hybrid Products Pricing of Hybrid Products using a
On the Valuation of Power-Reverse Duals and Equity-Rates Hybrids Oliver Caps oliver.caps@dkib.com RMT Model Validation Rates Dresdner Bank Examples of Hybrid Products Pricing of Hybrid Products using a
Hedging Interest-Rate Risk: A Primer in Instruments. & Accounting. September 29, 2015. Presented by: Ruth Hardie
 Hedging Interest-Rate Risk: A Primer in Instruments September 29, 2015 & Accounting Presented by: Ruth Hardie Hedge Trackers, LLC Integrated Derivative Management Interest Rate, Foreign Currency and Commodities
Hedging Interest-Rate Risk: A Primer in Instruments September 29, 2015 & Accounting Presented by: Ruth Hardie Hedge Trackers, LLC Integrated Derivative Management Interest Rate, Foreign Currency and Commodities
Equity-index-linked swaps
 Equity-index-linked swaps Equivalent to portfolios of forward contracts calling for the exchange of cash flows based on two different investment rates: a variable debt rate (e.g. 3-month LIBOR) and the
Equity-index-linked swaps Equivalent to portfolios of forward contracts calling for the exchange of cash flows based on two different investment rates: a variable debt rate (e.g. 3-month LIBOR) and the
The Depository Trust & Clearing Corporation. DTCC GCF Repo Index
 The Depository Trust & Clearing Corporation DTCC GCF Repo Index The DTCC GCF REPO INDEX is a service offering of DTCC Solutions LLC. Introduction On November 1, 2010 The Depository Trust & Clearing Corporation
The Depository Trust & Clearing Corporation DTCC GCF Repo Index The DTCC GCF REPO INDEX is a service offering of DTCC Solutions LLC. Introduction On November 1, 2010 The Depository Trust & Clearing Corporation
FIN 472 Fixed-Income Securities Forward Rates
 FIN 472 Fixed-Income Securities Forward Rates Professor Robert B.H. Hauswald Kogod School of Business, AU Interest-Rate Forwards Review of yield curve analysis Forwards yet another use of yield curve forward
FIN 472 Fixed-Income Securities Forward Rates Professor Robert B.H. Hauswald Kogod School of Business, AU Interest-Rate Forwards Review of yield curve analysis Forwards yet another use of yield curve forward
The properties of interest rate swaps An investigation of the price setting of illiquid interest rates swaps and the perfect hedging portfolios.
 The properties of interest rate swaps An investigation of the price setting of illiquid interest rates swaps and the perfect hedging portfolios. Max Lindquist 12/23/211 Abstract The main purpose of this
The properties of interest rate swaps An investigation of the price setting of illiquid interest rates swaps and the perfect hedging portfolios. Max Lindquist 12/23/211 Abstract The main purpose of this
550.444 Introduction to Financial Derivatives
 550.444 Introduction to Financial Derivatives Week of October 7, 2013 Interest Rate Futures Where we are Last week: Forward & Futures Prices/Value (Chapter 5, OFOD) This week: Interest Rate Futures (Chapter
550.444 Introduction to Financial Derivatives Week of October 7, 2013 Interest Rate Futures Where we are Last week: Forward & Futures Prices/Value (Chapter 5, OFOD) This week: Interest Rate Futures (Chapter
How To Calculate Interest Rate Derivative Options
 The Pricing and Hedging of Interest-Rate Derivatives: Theory and Practice Ser-Huang Poon 1, Richard C. Stapleton 2 and Marti G. Subrahmanyam 3 April 28, 2005 1 Manchester Business School 2 Manchester Business
The Pricing and Hedging of Interest-Rate Derivatives: Theory and Practice Ser-Huang Poon 1, Richard C. Stapleton 2 and Marti G. Subrahmanyam 3 April 28, 2005 1 Manchester Business School 2 Manchester Business
Floating rate Payments 6m Libor. Fixed rate payments 1 300000 337500-37500 2 300000 337500-37500 3 300000 337500-37500 4 300000 325000-25000
 Introduction: Interest rate swaps are used to hedge interest rate risks as well as to take on interest rate risks. If a treasurer is of the view that interest rates will be falling in the future, he may
Introduction: Interest rate swaps are used to hedge interest rate risks as well as to take on interest rate risks. If a treasurer is of the view that interest rates will be falling in the future, he may
Bond Options, Caps and the Black Model
 Bond Options, Caps and the Black Model Black formula Recall the Black formula for pricing options on futures: C(F, K, σ, r, T, r) = Fe rt N(d 1 ) Ke rt N(d 2 ) where d 1 = 1 [ σ ln( F T K ) + 1 ] 2 σ2
Bond Options, Caps and the Black Model Black formula Recall the Black formula for pricing options on futures: C(F, K, σ, r, T, r) = Fe rt N(d 1 ) Ke rt N(d 2 ) where d 1 = 1 [ σ ln( F T K ) + 1 ] 2 σ2
MONEY MARKET FUTURES. FINANCE TRAINER International Money Market Futures / Page 1 of 22
 MONEY MARKET FUTURES 1. Conventions and Contract Specifications... 3 2. Main Markets of Money Market Futures... 7 3. Exchange and Clearing House... 8 4. The Margin System... 9 5. Comparison: Money Market
MONEY MARKET FUTURES 1. Conventions and Contract Specifications... 3 2. Main Markets of Money Market Futures... 7 3. Exchange and Clearing House... 8 4. The Margin System... 9 5. Comparison: Money Market
IFRS Practice Issues for Banks:
 IFRS Practice Issues for Banks: Fair value measurement of derivatives the basics September 2012 kpmg.com/ifrs Contents Highlighting the path to fair value for derivatives 1 1. Introduction 2 2. How are
IFRS Practice Issues for Banks: Fair value measurement of derivatives the basics September 2012 kpmg.com/ifrs Contents Highlighting the path to fair value for derivatives 1 1. Introduction 2 2. How are
19. Interest Rate Swaps
 19. Interest Rate Swaps Reading: Stigum 19 on Swaps. See also Hull who builds from the idea (mentioned in Stigum) that swaps are like a portfolio of forward contracts. Daily Financial Times includes bid-ask
19. Interest Rate Swaps Reading: Stigum 19 on Swaps. See also Hull who builds from the idea (mentioned in Stigum) that swaps are like a portfolio of forward contracts. Daily Financial Times includes bid-ask
Zero-Coupon Bonds (Pure Discount Bonds)
 Zero-Coupon Bonds (Pure Discount Bonds) The price of a zero-coupon bond that pays F dollars in n periods is F/(1 + r) n, where r is the interest rate per period. Can meet future obligations without reinvestment
Zero-Coupon Bonds (Pure Discount Bonds) The price of a zero-coupon bond that pays F dollars in n periods is F/(1 + r) n, where r is the interest rate per period. Can meet future obligations without reinvestment
CFA Level -2 Derivatives - I
 CFA Level -2 Derivatives - I EduPristine www.edupristine.com Agenda Forwards Markets and Contracts Future Markets and Contracts Option Markets and Contracts 1 Forwards Markets and Contracts 2 Pricing and
CFA Level -2 Derivatives - I EduPristine www.edupristine.com Agenda Forwards Markets and Contracts Future Markets and Contracts Option Markets and Contracts 1 Forwards Markets and Contracts 2 Pricing and
Hot Topics in Financial Markets Lecture 1: The Libor Scandal
 Hot Topics in Financial Markets Lecture 1: The Libor Scandal Spot and Forward Interest Rates Libor Libor-Dependent Financial Instruments The Scandal 2 Spot Interest Rates Bond Market The yield on a bond
Hot Topics in Financial Markets Lecture 1: The Libor Scandal Spot and Forward Interest Rates Libor Libor-Dependent Financial Instruments The Scandal 2 Spot Interest Rates Bond Market The yield on a bond
Assumptions: No transaction cost, same rate for borrowing/lending, no default/counterparty risk
 Derivatives Why? Allow easier methods to short sell a stock without a broker lending it. Facilitates hedging easily Allows the ability to take long/short position on less available commodities (Rice, Cotton,
Derivatives Why? Allow easier methods to short sell a stock without a broker lending it. Facilitates hedging easily Allows the ability to take long/short position on less available commodities (Rice, Cotton,
Learning Curve An introduction to the use of the Bloomberg system in swaps analysis Received: 1st July, 2002
 Learning Curve An introduction to the use of the Bloomberg system in swaps analysis Received: 1st July, 2002 Aaron Nematnejad works in the fixed income analytics team at Bloomberg L.P. in London. He graduated
Learning Curve An introduction to the use of the Bloomberg system in swaps analysis Received: 1st July, 2002 Aaron Nematnejad works in the fixed income analytics team at Bloomberg L.P. in London. He graduated
A Teaching Note on Pricing and Valuing Interest Rate Swaps Using LIBOR and OIS Discounting
 A Teaching Note on Pricing and Valuing Interest Rate Swaps Using LIBOR and OIS Discounting June 202 Donald J. Smith Associate Professor of Finance Boston University School of Management 595 Commonwealth
A Teaching Note on Pricing and Valuing Interest Rate Swaps Using LIBOR and OIS Discounting June 202 Donald J. Smith Associate Professor of Finance Boston University School of Management 595 Commonwealth
VALUATION OF PLAIN VANILLA INTEREST RATES SWAPS
 Graduate School of Business Administration University of Virginia VALUATION OF PLAIN VANILLA INTEREST RATES SWAPS Interest-rate swaps have grown tremendously over the last 10 years. With this development,
Graduate School of Business Administration University of Virginia VALUATION OF PLAIN VANILLA INTEREST RATES SWAPS Interest-rate swaps have grown tremendously over the last 10 years. With this development,
How To Understand Credit Default Swaps
 The CDS market: A primer Including computational remarks on Default Probabilities online Roland Beck, Risk Analysis Group Folie 2 The CDS market: A primer Credit Default Swaps Short Introduction CDS are
The CDS market: A primer Including computational remarks on Default Probabilities online Roland Beck, Risk Analysis Group Folie 2 The CDS market: A primer Credit Default Swaps Short Introduction CDS are
Options: Definitions, Payoffs, & Replications
 Options: Definitions, s, & Replications Liuren Wu Zicklin School of Business, Baruch College Options Markets Liuren Wu (Baruch) s Options Markets 1 / 34 Definitions and terminologies An option gives the
Options: Definitions, s, & Replications Liuren Wu Zicklin School of Business, Baruch College Options Markets Liuren Wu (Baruch) s Options Markets 1 / 34 Definitions and terminologies An option gives the
NASDAQ OMX CASH FLOW MARGIN
 NASDAQ OMX CASH FLOW MARGIN Margin methodology guide for Nordic fixed income products. 11/3/2015 NASDAQ OMX Stockholm (NOMX) EXECUTIVE SUMMARY This document describes the NASDAQ OMX Cash Flow Margin (CFM)
NASDAQ OMX CASH FLOW MARGIN Margin methodology guide for Nordic fixed income products. 11/3/2015 NASDAQ OMX Stockholm (NOMX) EXECUTIVE SUMMARY This document describes the NASDAQ OMX Cash Flow Margin (CFM)
ANALYSIS OF FIXED INCOME SECURITIES
 ANALYSIS OF FIXED INCOME SECURITIES Valuation of Fixed Income Securities Page 1 VALUATION Valuation is the process of determining the fair value of a financial asset. The fair value of an asset is its
ANALYSIS OF FIXED INCOME SECURITIES Valuation of Fixed Income Securities Page 1 VALUATION Valuation is the process of determining the fair value of a financial asset. The fair value of an asset is its
Application of Interest Rate Swaps in Indian Insurance Industry Amruth Krishnan Rohit Ajgaonkar Guide: G.LN.Sarma
 Institute of Actuaries of India Application of Interest Rate Swaps in Indian Insurance Industry Amruth Krishnan Rohit Ajgaonkar Guide: G.LN.Sarma 21 st IFS Seminar Indian Actuarial Profession Serving the
Institute of Actuaries of India Application of Interest Rate Swaps in Indian Insurance Industry Amruth Krishnan Rohit Ajgaonkar Guide: G.LN.Sarma 21 st IFS Seminar Indian Actuarial Profession Serving the
Published in Journal of Investment Management, Vol. 13, No. 1 (2015): 64-83
 Published in Journal of Investment Management, Vol. 13, No. 1 (2015): 64-83 OIS Discounting, Interest Rate Derivatives, and the Modeling of Stochastic Interest Rate Spreads John Hull and Alan White Joseph
Published in Journal of Investment Management, Vol. 13, No. 1 (2015): 64-83 OIS Discounting, Interest Rate Derivatives, and the Modeling of Stochastic Interest Rate Spreads John Hull and Alan White Joseph
SwapEx Contract Specifications. USD LIBOR Interest Rate Swaps: Fixedto-Floating
 SwapEx Contract Specifications USD LIBOR Interest Rate Swaps: Fixedto-Floating There are two types of USD LIBOR Interest Rate Swaps; Fixed-to-Floating contracts available for trading on SwapEx: Fixed Rate
SwapEx Contract Specifications USD LIBOR Interest Rate Swaps: Fixedto-Floating There are two types of USD LIBOR Interest Rate Swaps; Fixed-to-Floating contracts available for trading on SwapEx: Fixed Rate
LIBOR vs. OIS: The Derivatives Discounting Dilemma
 LIBOR vs. OIS: The Derivatives Discounting Dilemma John Hull PRMIA May 2012 1 Agenda OIS and LIBOR CVA and DVA The Main Result Potential Sources of Confusion FVA and DVA See John Hull and Alan White: LIBOR
LIBOR vs. OIS: The Derivatives Discounting Dilemma John Hull PRMIA May 2012 1 Agenda OIS and LIBOR CVA and DVA The Main Result Potential Sources of Confusion FVA and DVA See John Hull and Alan White: LIBOR
CURRENT STANDARDS ON FIXED INCOME
 CURRENT STANDARDS ON FIXED INCOME PRICING FIXED INCOME SECURITIES AGAINST AN INTEREST SWAP CURVE December 2012 CNO Association régie par la loi du 1 er juillet 1901 8 rue du Mail 75002 Paris http://www.cnofrance.org
CURRENT STANDARDS ON FIXED INCOME PRICING FIXED INCOME SECURITIES AGAINST AN INTEREST SWAP CURVE December 2012 CNO Association régie par la loi du 1 er juillet 1901 8 rue du Mail 75002 Paris http://www.cnofrance.org
Miscellaneous. Simone Freschi freschi.simone@gmail.com Tommaso Gabriellini tommaso.gabriellini@mpscs.it. Università di Siena
 Miscellaneous Simone Freschi freschi.simone@gmail.com Tommaso Gabriellini tommaso.gabriellini@mpscs.it Head of Global MPS Capital Services SpA - MPS Group Università di Siena ini A.A. 2014-2015 1 / 1 A
Miscellaneous Simone Freschi freschi.simone@gmail.com Tommaso Gabriellini tommaso.gabriellini@mpscs.it Head of Global MPS Capital Services SpA - MPS Group Università di Siena ini A.A. 2014-2015 1 / 1 A
Buy = Pay Fixed, Receive Float -or- Pay Float +/- Spread, Receive Float Sell = Receive Fixed, Pay Float -or- Receive Float +/- Spread, Pay Float
 VIA Electronic Submission Office of the Secretariat Commodity Futures Trading Commission Three Lafayette Centre 1155 21 st Street, N.W. Washington, DC 20581 Re: Javelin SEF, LLC To Whom It May Concern,
VIA Electronic Submission Office of the Secretariat Commodity Futures Trading Commission Three Lafayette Centre 1155 21 st Street, N.W. Washington, DC 20581 Re: Javelin SEF, LLC To Whom It May Concern,
Analysis of Deterministic Cash Flows and the Term Structure of Interest Rates
 Analysis of Deterministic Cash Flows and the Term Structure of Interest Rates Cash Flow Financial transactions and investment opportunities are described by cash flows they generate. Cash flow: payment
Analysis of Deterministic Cash Flows and the Term Structure of Interest Rates Cash Flow Financial transactions and investment opportunities are described by cash flows they generate. Cash flow: payment
Dataline A look at current financial reporting issues
 Dataline A look at current financial reporting issues No. 2013-25 December 10, 2013 What s inside: Overview...1 Background... 2 OIS discounting an illustration... 3 Implications for market participants...
Dataline A look at current financial reporting issues No. 2013-25 December 10, 2013 What s inside: Overview...1 Background... 2 OIS discounting an illustration... 3 Implications for market participants...
Interest Rate Futures. Chapter 6
 Interest Rate Futures Chapter 6 1 Day Count Convention The day count convention defines: The period of time to which the interest rate applies. The period of time used to calculate accrued interest (relevant
Interest Rate Futures Chapter 6 1 Day Count Convention The day count convention defines: The period of time to which the interest rate applies. The period of time used to calculate accrued interest (relevant
Study on the Volatility Smile of EUR/USD Currency Options and Trading Strategies
 Prof. Joseph Fung, FDS Study on the Volatility Smile of EUR/USD Currency Options and Trading Strategies BY CHEN Duyi 11050098 Finance Concentration LI Ronggang 11050527 Finance Concentration An Honors
Prof. Joseph Fung, FDS Study on the Volatility Smile of EUR/USD Currency Options and Trading Strategies BY CHEN Duyi 11050098 Finance Concentration LI Ronggang 11050527 Finance Concentration An Honors
In this chapter we will learn about. Treasury Notes and Bonds, Treasury Inflation Protected Securities,
 2 Treasury Securities In this chapter we will learn about Treasury Bills, Treasury Notes and Bonds, Strips, Treasury Inflation Protected Securities, and a few other products including Eurodollar deposits.
2 Treasury Securities In this chapter we will learn about Treasury Bills, Treasury Notes and Bonds, Strips, Treasury Inflation Protected Securities, and a few other products including Eurodollar deposits.
Lecture 12. Options Strategies
 Lecture 12. Options Strategies Introduction to Options Strategies Options, Futures, Derivatives 10/15/07 back to start 1 Solutions Problem 6:23: Assume that a bank can borrow or lend money at the same
Lecture 12. Options Strategies Introduction to Options Strategies Options, Futures, Derivatives 10/15/07 back to start 1 Solutions Problem 6:23: Assume that a bank can borrow or lend money at the same
Bond Price Arithmetic
 1 Bond Price Arithmetic The purpose of this chapter is: To review the basics of the time value of money. This involves reviewing discounting guaranteed future cash flows at annual, semiannual and continuously
1 Bond Price Arithmetic The purpose of this chapter is: To review the basics of the time value of money. This involves reviewing discounting guaranteed future cash flows at annual, semiannual and continuously
Best Practices in Asset Liability Management
 Best Practices in Asset Liability Management Frank Wilary Principal Wilary Winn LLC September 22, 2014 1 Topics Covered Best practices related to ALM modeling Best practices related to managing the ALM
Best Practices in Asset Liability Management Frank Wilary Principal Wilary Winn LLC September 22, 2014 1 Topics Covered Best practices related to ALM modeling Best practices related to managing the ALM
Lecture 09: Multi-period Model Fixed Income, Futures, Swaps
 Lecture 09: Multi-period Model Fixed Income, Futures, Swaps Prof. Markus K. Brunnermeier Slide 09-1 Overview 1. Bond basics 2. Duration 3. Term structure of the real interest rate 4. Forwards and futures
Lecture 09: Multi-period Model Fixed Income, Futures, Swaps Prof. Markus K. Brunnermeier Slide 09-1 Overview 1. Bond basics 2. Duration 3. Term structure of the real interest rate 4. Forwards and futures
With the derivative markets having changed dramatically since the 2008 financial crisis,
 Avoiding Collateral Surprises: Managing Multi-Currency CSAs Anna Barbashova, Numerix - 24 Jan 2013 This article explores multi-currency credit support annexes (CSAs) in the derivatives area and their potential
Avoiding Collateral Surprises: Managing Multi-Currency CSAs Anna Barbashova, Numerix - 24 Jan 2013 This article explores multi-currency credit support annexes (CSAs) in the derivatives area and their potential
Bank of America AAA 10.00% T-Bill +.30% Hypothetical Resources BBB 11.90% T-Bill +.80% Basis point difference 190 50
 Swap Agreements INTEREST RATE SWAP AGREEMENTS An interest rate swap is an agreement to exchange interest rate payments on a notional principal amount over a specific period of time. Generally a swap exchanges
Swap Agreements INTEREST RATE SWAP AGREEMENTS An interest rate swap is an agreement to exchange interest rate payments on a notional principal amount over a specific period of time. Generally a swap exchanges
Tenor Adjustments for a Basis Swap
 Tenor Adjustments for a Basis Swap by Chandrakant Maheshwari Praveen Maheshwari Table of Contents 1. Introduction 3 2. Tenor Adjustment Methodology for a Basis Swap 3 3. Why this Tenor Spread so important
Tenor Adjustments for a Basis Swap by Chandrakant Maheshwari Praveen Maheshwari Table of Contents 1. Introduction 3 2. Tenor Adjustment Methodology for a Basis Swap 3 3. Why this Tenor Spread so important
Butterflies, Condors, and Jelly Rolls: Derivatives Explained
 Butterflies, Condors, and Jelly Rolls: Derivatives Explained American Translators Association 47 th Annual Conference, New Orleans November 1, 2006 Ralf Lemster 1 Derivatives Explained What are derivatives?
Butterflies, Condors, and Jelly Rolls: Derivatives Explained American Translators Association 47 th Annual Conference, New Orleans November 1, 2006 Ralf Lemster 1 Derivatives Explained What are derivatives?
Hedging with Futures and Options: Supplementary Material. Global Financial Management
 Hedging with Futures and Options: Supplementary Material Global Financial Management Fuqua School of Business Duke University 1 Hedging Stock Market Risk: S&P500 Futures Contract A futures contract on
Hedging with Futures and Options: Supplementary Material Global Financial Management Fuqua School of Business Duke University 1 Hedging Stock Market Risk: S&P500 Futures Contract A futures contract on
1.2 Structured notes
 1.2 Structured notes Structured notes are financial products that appear to be fixed income instruments, but contain embedded options and do not necessarily reflect the risk of the issuing credit. Used
1.2 Structured notes Structured notes are financial products that appear to be fixed income instruments, but contain embedded options and do not necessarily reflect the risk of the issuing credit. Used
Learning Curve Interest Rate Futures Contracts Moorad Choudhry
 Learning Curve Interest Rate Futures Contracts Moorad Choudhry YieldCurve.com 2004 Page 1 The market in short-term interest rate derivatives is a large and liquid one, and the instruments involved are
Learning Curve Interest Rate Futures Contracts Moorad Choudhry YieldCurve.com 2004 Page 1 The market in short-term interest rate derivatives is a large and liquid one, and the instruments involved are
Introduction to Derivative Instruments Part 1 Link n Learn
 Introduction to Derivative Instruments Part 1 Link n Learn June 2014 Webinar Participants Elaine Canty Manager Financial Advisory Deloitte & Touche Ireland ecanty@deloitte.ie +353 1 417 2991 Christopher
Introduction to Derivative Instruments Part 1 Link n Learn June 2014 Webinar Participants Elaine Canty Manager Financial Advisory Deloitte & Touche Ireland ecanty@deloitte.ie +353 1 417 2991 Christopher
Options On Credit Default Index Swaps
 Options On Credit Default Index Swaps Yunkang Liu and Peter Jäckel 20th May 2005 Abstract The value of an option on a credit default index swap consists of two parts. The first one is the protection value
Options On Credit Default Index Swaps Yunkang Liu and Peter Jäckel 20th May 2005 Abstract The value of an option on a credit default index swap consists of two parts. The first one is the protection value
Coupon Bonds and Zeroes
 Coupon Bonds and Zeroes Concepts and Buzzwords Coupon bonds Zero-coupon bonds Bond replication No-arbitrage price relationships Zero rates Zeroes STRIPS Dedication Implied zeroes Semi-annual compounding
Coupon Bonds and Zeroes Concepts and Buzzwords Coupon bonds Zero-coupon bonds Bond replication No-arbitrage price relationships Zero rates Zeroes STRIPS Dedication Implied zeroes Semi-annual compounding
ACI THE FINANCIAL MARKETS ASSOCIATION
 ACI THE FINANCIAL MARKETS ASSOCIATION EXAMINATION FORMULAE 2009 VERSION page number INTEREST RATE..2 MONEY MARKET..... 3 FORWARD-FORWARDS & FORWARD RATE AGREEMENTS..4 FIXED INCOME.....5 FOREIGN EXCHANGE
ACI THE FINANCIAL MARKETS ASSOCIATION EXAMINATION FORMULAE 2009 VERSION page number INTEREST RATE..2 MONEY MARKET..... 3 FORWARD-FORWARDS & FORWARD RATE AGREEMENTS..4 FIXED INCOME.....5 FOREIGN EXCHANGE
Fixed-Income Securities. Assignment
 FIN 472 Professor Robert B.H. Hauswald Fixed-Income Securities Kogod School of Business, AU Assignment Please be reminded that you are expected to use contemporary computer software to solve the following
FIN 472 Professor Robert B.H. Hauswald Fixed-Income Securities Kogod School of Business, AU Assignment Please be reminded that you are expected to use contemporary computer software to solve the following
Interest Rate Swaps. Key Concepts and Buzzwords. Readings Tuckman, Chapter 18. Swaps Swap Spreads Credit Risk of Swaps Uses of Swaps
 Interest Rate Swaps Key Concepts and Buzzwords Swaps Swap Spreads Credit Risk of Swaps Uses of Swaps Readings Tuckman, Chapter 18. Counterparty, Notional amount, Plain vanilla swap, Swap rate Interest
Interest Rate Swaps Key Concepts and Buzzwords Swaps Swap Spreads Credit Risk of Swaps Uses of Swaps Readings Tuckman, Chapter 18. Counterparty, Notional amount, Plain vanilla swap, Swap rate Interest
Derivatives, Measurement and Hedge Accounting
 Derivatives, Measurement and Hedge Accounting IAS 39 11 June 2008 Contents Derivatives and embedded derivatives Definition Sample of products Accounting treatment Measurement Active market VS Inactive
Derivatives, Measurement and Hedge Accounting IAS 39 11 June 2008 Contents Derivatives and embedded derivatives Definition Sample of products Accounting treatment Measurement Active market VS Inactive
Product Descriptions Credit Derivatives. Credit Derivatives Product Descriptions
 Credit Derivatives Product Descriptions 1 Products Credit Derivatives Indices Credit Derivatives Tranches Credit Derivatives Options Product Specifications Credit Derivatives Indices A credit default swap
Credit Derivatives Product Descriptions 1 Products Credit Derivatives Indices Credit Derivatives Tranches Credit Derivatives Options Product Specifications Credit Derivatives Indices A credit default swap
Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008
 : A Stern School of Business New York University Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008 Outline 1 2 3 4 5 6 se notes review the principles underlying option pricing and some of
: A Stern School of Business New York University Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008 Outline 1 2 3 4 5 6 se notes review the principles underlying option pricing and some of
Finance 350: Problem Set 6 Alternative Solutions
 Finance 350: Problem Set 6 Alternative Solutions Note: Where appropriate, the final answer for each problem is given in bold italics for those not interested in the discussion of the solution. I. Formulas
Finance 350: Problem Set 6 Alternative Solutions Note: Where appropriate, the final answer for each problem is given in bold italics for those not interested in the discussion of the solution. I. Formulas
Risk and Return in the Canadian Bond Market
 Risk and Return in the Canadian Bond Market Beyond yield and duration. Ronald N. Kahn and Deepak Gulrajani (Reprinted with permission from The Journal of Portfolio Management ) RONALD N. KAHN is Director
Risk and Return in the Canadian Bond Market Beyond yield and duration. Ronald N. Kahn and Deepak Gulrajani (Reprinted with permission from The Journal of Portfolio Management ) RONALD N. KAHN is Director
Advanced forms of currency swaps
 Advanced forms of currency swaps Basis swaps Basis swaps involve swapping one floating index rate for another. Banks may need to use basis swaps to arrange a currency swap for the customers. Example A
Advanced forms of currency swaps Basis swaps Basis swaps involve swapping one floating index rate for another. Banks may need to use basis swaps to arrange a currency swap for the customers. Example A
Understanding Futures on the DTCC GCF Repo Index
 Understanding Futures on the DTCC GCF Repo Index White Paper June 2014 This material may not be reproduced or redistributed in whole or in part without the express, prior written consent of Intercontinental
Understanding Futures on the DTCC GCF Repo Index White Paper June 2014 This material may not be reproduced or redistributed in whole or in part without the express, prior written consent of Intercontinental
w w w.c a t l e y l a k e m a n.c o m 0 2 0 7 0 4 3 0 1 0 0
 A ADR-Style: for a derivative on an underlying denominated in one currency, where the derivative is denominated in a different currency, payments are exchanged using a floating foreign-exchange rate. The
A ADR-Style: for a derivative on an underlying denominated in one currency, where the derivative is denominated in a different currency, payments are exchanged using a floating foreign-exchange rate. The
Accounting for Derivatives. Rajan Chari Senior Manager rchari@deloitte.com
 Accounting for Derivatives Rajan Chari Senior Manager rchari@deloitte.com October 3, 2005 Derivative Instruments to be Discussed Futures Forwards Options Swaps Copyright 2004 Deloitte Development LLC.
Accounting for Derivatives Rajan Chari Senior Manager rchari@deloitte.com October 3, 2005 Derivative Instruments to be Discussed Futures Forwards Options Swaps Copyright 2004 Deloitte Development LLC.
Binary options. Giampaolo Gabbi
 Binary options Giampaolo Gabbi Definition In finance, a binary option is a type of option where the payoff is either some fixed amount of some asset or nothing at all. The two main types of binary options
Binary options Giampaolo Gabbi Definition In finance, a binary option is a type of option where the payoff is either some fixed amount of some asset or nothing at all. The two main types of binary options
Master s Thesis. Pricing Constant Maturity Swap Derivatives
 Master s Thesis Pricing Constant Maturity Swap Derivatives Thesis submitted in partial fulfilment of the requirements for the Master of Science degree in Stochastics and Financial Mathematics by Noemi
Master s Thesis Pricing Constant Maturity Swap Derivatives Thesis submitted in partial fulfilment of the requirements for the Master of Science degree in Stochastics and Financial Mathematics by Noemi
FORWARDS AND EUROPEAN OPTIONS ON CDO TRANCHES. John Hull and Alan White. First Draft: December, 2006 This Draft: March 2007
 FORWARDS AND EUROPEAN OPTIONS ON CDO TRANCHES John Hull and Alan White First Draft: December, 006 This Draft: March 007 Joseph L. Rotman School of Management University of Toronto 105 St George Street
FORWARDS AND EUROPEAN OPTIONS ON CDO TRANCHES John Hull and Alan White First Draft: December, 006 This Draft: March 007 Joseph L. Rotman School of Management University of Toronto 105 St George Street
CS 522 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options
 CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
