Caps and Floors. John Crosby
|
|
|
- Margaret Harper
- 8 years ago
- Views:
Transcription
1 Caps and Floors John Crosby Glasgow University My website is: If you spot any typos or errors, please me. My address is on my website Lecture given 19th February 2014 for the M.Sc. and Ph.D. courses in Finance / Financial Economics at Glasgow University Adam Smith Business School File date 4th February 2014 at 10.23
2 Introduction and motivation Caps and floors are amongst the simplest and most common types of interest-rate derivatives. They are very widely used by corporations for managing interest-rate risk. Individuals also buy caps (perhaps without knowing it) when they buy capped mortgages. In this lecture: 1./ We will recap different definitions and meanings of interest-rates (spot interest-rates, LIBOR rates, forward interest-rates) and their relationships to zero-coupon bonds. 2./ We will analyse caps and floors and derive pricing relationships. 3./ We will also examine the relationship between forward contracts and futures contracts focussing on the difference between a 3 month FRA (forward-rate agreement) and a 3 month LIBOR (Eurodollar) futures contract.
3 Assumptions We will make some key assumptions (which are essentially the same as those used to derive the Black-Scholes option pricing formula for stock options) throughout this lecture: The key assumption we make is that we assume the absence of arbitrage. We also assume that markets are frictionless eg continuous trading (in zero-coupon bonds) is possible, there are no transactions costs (i.e. no bid-offer spreads, it is possible to borrow and lend at the same rate), etc.
4 Recap on interest-rate definitions Calendar time is denoted by t. We let the price, at time t, of a zero-coupon bond which matures at time T be P (t, T ). Note three important (but I hope obvious) things: Firstly, P (t, T ) is known when the valuation date is on or after t. Secondly, by contrast, P (t, T ) for any t greater than the valuation date is a stochastic quantity. Thirdly, P (T, T ) = 1, for any T. We denote the (continuously-compounded) instantaneous short interest-rate, at time t, by r(t). Note that r(t) will, throughout this lecture, be stochastic.
5 Introduction to LIBOR In the interest-rate markets, one of the main interest-rates quoted is the LIBOR rate. LIBOR is quoted as a simple (i.e. without compounding) interest-rate and is the rate that a bank will lend money to another credit-worthy institution (although we draw no distinction between the bank lending and the bank accepting deposits) from time T 1 to time T 2. (We will not, in this lecture, consider credit-related issues). We let τ be the time in years between T 1 and T 2 but adjusted for the day-count fraction. So roughly, τ T 2 T 1. In practice, τ is often approximately 0.25 or 0.50 (corresponding to 3 months or 6 months) but LIBOR is quoted for periods from overnight to one year.
6 Introduction to LIBOR 2 Suppose today is time T 1. We are given the price P (T 1, T 2 ) (ie at time T 1 - this is the meaning of the first argument of P ) of a zero-coupon bond which matures at time T 2. We are given a LIBOR rate L(T 1, T 1, T 2 ), at time T 1, from time T 1 to time T 2 (the meaning of the two arguments equal to T 1 will be clear shortly). What is the relationship between L(T 1, T 1, T 2 ) and P (T 1, T 2 )? It is: P (T 1, T 2 ) = τl(t 1, T 1, T 2 ) or L(T 1, T 1, T 2 ) = 1 ( 1 ) τ P (T 1, T 2 ) 1, (1) where, again, τ is the time in years between T 1 and T 2 (but adjusted for the day-count fraction: τ T 2 T 1 ).
7 Introduction to spot, short interest-rates Today is still time T 1. We are still given the price P (T 1, T 2 ) of a zero-coupon bond which matures at time T 2. We are given a continuously-compounded spot interest-rate R(T 1, T 2 ) from time T 1 to time T 2. What is the relationship between R(T 1, T 2 ) and P (T 1, T 2 )? It is: P (T 1, T 2 ) = exp( R(T 1, T 2 )(T 2 T 1 )) or R(T 1, T 2 ) = 1 (T 2 T 1 ) log P (T 1, T 2 ). (2) What is the relationship between the (continuously-compounded) instantaneous short interest-rate r(t), at time t, for t [T 1, T 2 ] and P (T 1, T 2 ). It is: P (T 1, T 2 ) = E Q T 1 [exp( T2 T 1 r(u)du)]. (3) Here, E Q T 1 [ ] denotes expectations, at time T 1, under some risk-neutral measure Q.
8 Introduction to spot, short interest-rates 2 Again: P (T 1, T 2 ) = E Q T 1 [exp( T2 T 1 r(u)du)]. (4) Note that: P (T 1, T 2 ) exp( T 2 T 1 r(u)du). (P (T 1, T 2 ) would be equal to exp( T 2 T 1 r(u)du) in the special case that r(t) is deterministic - but we won t be assuming that). Note that equation (4) has a simple interpretation: Standard theory tells us that prices are expected (under Q) discounted payoffs. The zero-coupon bond pays one at time T 2, so: in agreement with equation (4). P (T 1, T 2 ) = E Q T 1 [exp( T2 T 1 r(u)du) 1 ], (5)
9 Introduction to spot, short interest-rates 3 The spot interest-rate R(T 1, T 2 ) from time T 1 to time T 2 can be interpreted as an average interest-rate over the time period [T 1, T 2 ] and also as the yield on the zero-coupon bond which matures at time T 2. The instantaneous short interest-rate r(t) should be interpreted as a stochastic process. We know what r(t) is at time t. For u > t, r(u) is only known as calendar time advances to time u. Then the instantaneous short interest-rate r(u) is known and can be interpreted as the interest-rate on a bank account over the infinitesimally short time period from u to u + du.
10 Forward LIBOR rate We denote the LIBOR rate observed, at time t, which sets at time T 1 and is paid at time T 2 by L(t, T 1, T 2 ), with t < T 1 < T 2. But what does it mean observed at time t since the LIBOR rate itself will only be known at time T 1 (with T 1 > t) (and the actual interest payment will be paid at time T 2 (with T 2 > T 1 > t))?
11 Forward LIBOR rate replication 1 How can I replicate, at time t, receiving a guaranteed payment of L(T 1, T 1, T 2 ) at time T 2 where I will only know the value of L(T 1, T 1, T 2 ) at time T 1, with T 1 > t? Consider the following strategy: At time t: I buy 1/τ zero-coupon bonds maturing at time T 1 for a price of P (t, T 1 )/τ and I simultaneously sell 1/τ zero-coupon bonds maturing at time T 2 for a price of P (t, T 2 )/τ. At time T 1, my T 1 maturity bonds mature and I place the principal amount of 1/τ on deposit at the (now known) rate of L(T 1, T 1, T 2 ). I will get this back with interest at time T 2 and receive (principal + interest) (1 + τl(t 1, T 1, T 2 ))/τ. I will have to pay 1/τ at time T 2 to repay the T 2 maturity bonds which mature. Therefore at time T 2, I receive a net amount of: ((1 + τl(t 1, T 1, T 2 ))/τ) (1/τ) = L(T 1, T 1, T 2 ).
12 Forward LIBOR rate replication 2 In short, I receive a guaranteed (in the assumed absence of credit-risk) payment of L(T 1, T 1, T 2 ) at time T 2 from my trades. How much did it cost me to enter into the trades? At time t, I paid P (t, T 1 )/τ to buy 1/τ zero-coupon bonds maturing at time T 1 and I received P (t, T 2 )/τ for selling 1/τ zero-coupon bonds maturing at time T 2. So the net cost, at time t, was: (P (t, T 1 )/τ) (P (t, T 2 )/τ) = (P (t, T 1 ) P (t, T 2 ))./τ Therefore, in the assumed absence of arbitrage, the value, at time t, of receiving a payment of L(T 1, T 1, T 2 ) at time T 2, is: (P (t, T 1 ) P (t, T 2 ))/τ. More mathematically: E Q t [exp( T2 t r(s)ds)l(t 1, T 1, T 2 )] = (P (t, T 1 ) P (t, T 2 ))/τ. (6) Here, E Q t [ ] denotes expectation, at time t, under the risk-neutral equivalent martingale measure Q. We recall that r(s) is the (continuously-compounded) instantaneous short interest-rate, at time s.
13 Forward LIBOR rate replication 3 The previous slide gives the answer to our question on slide Forward LIBOR rate. Given the prices, at time t, of zero-coupon bonds maturing at time T 1 and at time T 2, and a simple trading strategy, we can perfectly replicate, a payment of L(T 1, T 1, T 2 ) at time T 2. We do not have to wait until time T 1 when the LIBOR rate becomes known. Therefore, we define L(t, T 1, T 2 ), for t < T 1, via: L(t, T 1, T 2 ) = (P (t, T 1) P (t, T 2 ))/τ P (t, T 2 ) = 1 τ (P (t, T 1) P (t, T 2 ) 1). This is the same as: τl(t, T 1, T 2 ) = P (t, T 2) P (t, T 1 ).
14 FRA - forward-rate agreement The last two slides define an instrument called a FRA. A FRA is a forward contract on forward LIBOR i.e. on L(t, T 1, T 2 ). It has a payoff at time T 2 equal to: L(T 1, T 1, T 2 ) K, where K is chosen so that the contract costs nothing to enter into at time t. Therefore, K must be equal to: K = L(t, T 1, T 2 ). FRA s are very actively traded in the markets. One will hear them referred to as, for examples, the 3 X 6 FRA. It means T 1 is (approximately) 3 months from t and T 2 is (approximately) 6 months from t.
15 Caps A caplet is an option which has a payoff at time T 2 equal to: where K is the strike. max(l(t 1, T 1, T 2 ) K, 0), Note the sequence of times implicit in a caplet: The LIBOR rate sets (i.e. becomes known) at time T 1. This means the payoff of the caplet is known at time T 1 also. The payoff is actually made at some later time T 2 (i.e. T 2 > T 1 ) which is also the payment time of the LIBOR rate. We are interested in the price of the caplet at some time t. To be interesting, we assume that t < T 1. Before discussing the valuation of caplets. We briefly discuss some practicalities.
16 Practicalities of caps Notional amounts of fifty million pounds (or equivalent in dollars or euros) are fairly standard in the caps market. Caps are a linear combination of caplets with dates defined via T 0 < T 1 < T 2 <... < T N. Therefore, we focus on caplets since given a pricing formula for caplets, we can price caps trivially by adding together the price of each individual caplet. When the sequence of dates is such that the payoff of the first caplet is known (or will be known) on the date the cap is entered into, the convention is to disregard the first caplet. So a semi-annual two year cap, entered into on 6th Feb 2009, with final maturity 7th Feb 2011, only has three underlying caplets (with payoff dates 8th February 2010, 9th August 2010 and 7th February 2011). Floors are the put version of caps and can similarly be decomposed into linear combinations of floorlets. A floorlet has a payoff at time T 2 equal to: max(k L(T 1, T 1, T 2 ), 0).
17 Practicalities of caps 2 We will discuss the pricing of caps shortly. As with options on other asset classes (such as equities), prices are quoted in the market in the form of Black implied volatilities (the meaning of which, we discuss shortly). As with other asset classes, these implied volatilities display considerable variation with maturity and strike. In other words, we see skews and smiles in implied volatilities as well as significant time-dependence. Caps for maturities 1y, 18m, 2y, 3y, 4y, 5y, 10y, 15y, 20y, 25y and 30y trade at a variety of strikes from close to zero per cent to, perhaps, around 5 per cent above the prevailing forward LIBOR rate. The most liquid are close to at-the-money but there is reasonable liquidity even in caps which are significantly out-of-the-money.
18 Caplet 1 A caplet is an option which has a payoff at time T 2 equal to: max(l(t 1, T 1, T 2 ) K, 0). Note the sequence of times implicit in a caplet: The LIBOR rate sets (i.e. becomes known) at time T 1. This means the payoff of the caplet is known at time T 1 also. The payoff is actually made at some later time T 2 (i.e. T 2 > T 1 ) which is also the payment time of the LIBOR rate. Since 1 (1 + τl(t 1, T 1, T 2 )) = P (T 1, T 2 ), we can write: L(T 1, T 1, T 2 ) = 1 τ ( 1 P (T 1, T 2 ) 1). Again, τ is the day-count adjusted time in years between T 1 and T 2.
19 Caplet 2 Therefore, the caplet payoff at time T 2 is: max(l(t 1, T 1, T 2 ) K, 0) = max( 1 τ ( 1 P (T 1, T 2 ) 1) K, 0). Now lets pause. The caplet payoff is known at time T 1 but made at time T 2. The price of a zero-coupon bond, at time T 1, maturing at time T 2 is P (T 1, T 2 ). Therefore, the caplet payoff max(l(t 1, T 1, T 2 ) K, 0) at time T 2 is completely equivalent to a payoff of P (T 1, T 2 ) max(l(t 1, T 1, T 2 ) K, 0) at time T 1. This must be true because, at time T 1, one could simply place the known amount of P (T 1, T 2 ) max(l(t 1, T 1, T 2 ) K, 0) on deposit or in zero-coupon bonds maturing at time T 2. At time T 2, one would have max(l(t 1, T 1, T 2 ) K, 0). But P (T 1, T 2 ) max(l(t 1, T 1, T 2 ) K, 0) = P (T 1, T 2 ) max( 1 τ ( 1 P (T 1, T 2 ) 1) K, 0) = 1 τ max(1 (1 + Kτ)P (T 1, T 2 ), 0).
20 Caplet 3 So a caplet with a payoff at time T 2 equal to max(l(t 1, T 1, T 2 ) K, 0) is equivalent to an option with a payoff at time T 1 equal to: 1 τ max(1 (1 + Kτ)P (T 1, T 2 ), 0). But this is just the same as (1 + Kτ)/τ options with strike K 1/(1 + Kτ) paying max(k P (T 1, T 2 ), 0) at time T 1. But max(k P (T 1, T 2 ), 0) paid at time T 1 is the same as the payoff, at time T 1, of a put option on the price, at time T 1, of a zero-coupon bond maturing at time T 2, where the strike of the put option is K. So a caplet is a put option on a zero-coupon bond. So to price a caplet, we need to be able to price an option on a zero-coupon bond. We will do this shortly.
21 Caplet 4 How do we price an option on a zero-coupon bond? We will now answer this question. It is somewhat similar to the derivation of the Black-Scholes formula for an option on a stock but with a key difference: Bond prices and interest-rates are stochastic. We assume that the dynamics of the price P (t, T ) of a zero coupon bond, at time t, maturing at time T i (here, i is 1 or 2) are: dp (t, T i ) P (t, T i ) = µ i(t)dt + σ P (t, T i )dz P i (t). (7) It is intuitive that, in a risk-neutral world, the drift term µ i (t) is equal to r(t) (after all, a zero-coupon bond is a non-dividend-paying asset) but we will not yet make that identification. We will assume that the SDE above specifies the dynamics in the real-world objective probability measure P (hence the superscript P in dzi P (t) in the SDE) and go through a hedging argument to derive the equivalent of the Black-Scholes PDE.
22 Caplet 5 We consider an option which pays, at time T 1, an amount C(T 1 ) (for example, C(T 1 ) max(p (T 1, T 2 ) K, 0) or C(T 1 ) max(k P (T 1, T 2 ), 0)). We denote the price, at time t, of the option by C(t). It is intuitive that the price C(t) actually depends on both P (t, T 1 ) and P (t, T 2 ). That is to say: C(t) C(t, P (t, T 1 ), P (t, T 2 )). Using the multi-dimensional form of Ito s lemma and writing P (t, T 1 ) P 1 and P (t, T 2 ) P 2 : dc(t) = C(t) dt + C(t) (µ 1 dt + σ P (t, T 1 )dz1 P (t))p 1 + C(t) (µ 2 dt + σ P (t, T 2 )dz2 P (t))p 2 t P 1 P 2 ( 1 2 C(t) + 2 P1 2 σp(t, 2 T 1 )P C(t) 2 P2 2 σp(t, 2 T 2 )P2 2 2 C(t) ) + ρ 12 σ P (t, T 1 )σ P (t, T 2 )P 1 P 2 dt. P 1 P 2 Here ρ 12 is the (assumed deterministic) instantaneous correlation between dz P 1 (t) and dz P 2 (t).
23 Caplet 6 We set up a self-financing portfolio consisting, at time t, of long one option, short C(t) P 1 bonds maturing at time T 1 and short C(t) P 2 bonds maturing at time T 2. We do not hold any position in cash at any time. The portfolio always has zero net aggregate investment. The value of the portfolio at time t is 0 = C(t) C(t) P 1 P 1 C(t) P 2 P 2. The left-hand-side of the last equation is simply the statement of zero net aggregate investment, for all t. We stress that the short positions in bonds maturing at time T 1 and at time T 2 are dynamic positions which are changing through time (analogously to the dynamic delta-hedge used in deriving the Black-Scholes pde for stock options).
24 Caplet 7 The change in the value of the portfolio, during the period t to t + dt is: dc(t) C(t) P 1 dp 1 C(t) P 2 dp 2 = C(t) dt t ( C(t) P1 2 σp(t, 2 T 1 )P C(t) P2 2 σp(t, 2 T 2 )P2 2 + ρ 12 2 C(t) P 1 P 2 σ P (t, T 1 )σ P (t, T 2 )P 1 P 2 ) dt, where we used slide Caplet 5. Note that the terms with dz P 1 (t) and dz P 2 (t) have cancelled so this is an instantaneously risk-free portfolio. The portfolio is always worth zero - because it always has zero net aggregate investment. Therefore, in the absence of arbitrage, it must have a realised return of exactly zero, for all t. Therefore: dc(t) C(t) P 1 dp 1 C(t) P 2 dp 2 = 0.
25 Caplet 8 Or: 0 = C(t) t C(t) P1 2 σp(t, 2 T 1 )P C(t) P2 2 σp(t, 2 T 2 )P2 2 + ρ 12 2 C(t) P 1 P 2 σ P (t, T 1 )σ P (t, T 2 )P 1 P 2. This is a pde - essentially, the equivalent of the Black-Scholes pde. We would like to reduce it to a pde in one state variable. How can we do this?
26 Caplet 9 We guess (and show later that it is a good guess) that the price of the option C(t) C(t, P (t, T 1 ), P (t, T 2 )) is actually a function of the forward bond price defined by: Specifically, we write: F (t) F (t, T 2 ) P (t, T 1) P (t, T 2 ) P 1 P 2. C(t) C(t, P (t, T 1 ), P (t, T 2 )) P (t, T 2 )G(t, F (t)) P 2 G(t). Then: C(t) P 1 C(t) P 2 2 C(t) P C(t) P 1 P 2 G(t) G(t) G(t) 1 = P 2 = P 2 = G(t) F P 1 F P 2 G(t) F G(t) = G(t) + P 2 = G(t) + P 2 F P 2 F = 2 G(t) F 2 1 P 2, = F 2 G(t) F 2 1 P 2. 2 C(t) P 2 2 = F 2 2 G(t) F 2 1 P 2, F, ( P1 ) P 2 2 = G(t) G(t) G(t) F,
27 Caplet 10 Let us check our equation for zero net aggregate investment: The right-hand-side becomes: C(t) C(t) P 1 P 1 C(t) P 2 P 2 0 = C(t) C(t) P 1 P 1 C(t) P 2 P 2. = P 2 G(t) G(t) ( F P G(t) ( P1 )) 1 G(t) + P 2 F P2 2 P 2 = 0. (8) So our zero net aggregate investment condition is automatically satisfied, for all t.
28 Caplet 11 The pde becomes: G(t) 0 = P 2 t P 2 which is the same as: where: Σ 2 (t) P 2 G(t) 2 F 2 σ2 P(t, T 1 )F 2 2 G(t) F 2 σ2 P(t, T 2 )F 2 2 G(t) P 2 ρ 12 F σ P(t, T 2 1 )σ P (t, T 2 )F 2, 0 = G(t) t Σ2 (t) 2 G(t) F 2 F 2, (9) ( ) σp(t, 2 T 1 ) + σp(t, 2 T 2 ) ρ 12 σ P (t, T 1 )σ P (t, T 2 ). This is just the Black-Scholes pde with zero interest-rates, zero dividend yield and volatility Σ(t).
29 Forward measure We know that we can write the price of the option, at time t, in the form: C(t) = E Q t [exp( T1 t r(s)ds)c(t 1 )]. However, from the pde in equation (9), we can also evaluate G(t) by writing it in the form: G(t) = E Q t [G(T 1 )] = E Q t [G(T 1, P (T 1, T 1 ) )], (10) P (T 1, T 2 ) where, Q defines a new probability measure (called the forward measure) in which we form expectations as if interest-rates and dividend yields in the Black-Scholes pde are zero (the former explains why there is no term of the form exp( T 1 t r(s)ds) in equation (10)). However, C(t) = P (t, T 2 )G(t) and C(T 1 ) = P (T 1, T 2 )G(T 1 ). Therefore: C(t) = P (t, T 2 )E Q t [ C(T [G(T 1 )] = P (t, T 2 )E Q 1 ) ] t. (11) P (T 1, T 2 )
30 Forward measure 2 Suppose, we consider a payoff, at time T 1, equal to P (T 1, T 2 ). Since P (T 1, T 2 ) paid at time T 1 is the same as 1 paid at time T 2, we know that, trivially, C(t) = P (t, T 2 ). From our pricing formulae, we get: So: C(t) = E Q t [exp( T1 t r(s)ds)p (T 1, T 2 )] and C(t) = P (t, T 2 )E Q t [ P (T 1, T 2 )) P (T 1, T 2 ) ] = P (t, T 2). P (t, T 2 ) = E Q t [exp( T1 t r(s)ds)p (T 1, T 2 )] = P (t, T 2 ), which is simply confirmation of what we already know. It also confirms that, under the risk-neutral equivalent martingale measure Q, the drift rate on a zero-coupon bond, at time t, is r(t).
31 Forward measure 3 More pertinently, we consider a payoff, at time T 1, equal to 1. We know that, trivially, C(t) = P (t, T 1 ). From our pricing formulae, we get: So: C(t) = E Q t [exp( T1 P (t, T 1 ) = E Q t [exp( t r(s)ds) 1] and C(t) = P (t, T 2 )E Q T1 Since P (T 1, T 1 ) = 1, let us write the last equation: t r(s)ds) 1] = P (t, T 2 )E Q P (t, T 1 ) P (t, T 2 ) = EQ t [ P (T 1, T 1 ) P (T 1, T 2 ) ]. t [ t [ 1 P (T 1, T 2 ) ]. 1 P (T 1, T 2 ) ]. This means that in the measure Q, the quantity P (t, T 1 )/P (t, T 2 ) is a martingale - the value of the quantity at time t is the expectation of its value at time T 1. This is the first time you will have encountered the idea of a change of measure in this way. Our approach has been long-winded but it can be made simpler when you have studied Girsanov s theorem.
32 Forward measure 4 Again: P (t, T 1 ) P (t, T 2 ) = EQ t [ P (T 1, T 1 ) P (T 1, T 2 ) ]. Since, 1 + τl(t, T 1, T 2 ) = P (t, T 1 )/P (t, T 2 ) and 1 + τl(t 1, T 1, T 2 ) = P (T 1, T 1 )/P (T 1, T 2 ). We can also write: L(t, T 1, T 2 ) = E Q t [L(T 1, T 1, T 2 )]. (12) This means that in the measure Q, the forward LIBOR rate is also a martingale. Recall, that in equation (6): E Q t [exp( T2 t r(s)ds)l(t 1, T 1, T 2 )] = (P (t, T 1 ) P (t, T 2 ))/τ, hence rearranging L(t, T 1, T 2 ) = EQ t [exp( T 2 t r(s)ds)l(t 1, T 1, T 2 )]. (13) P (t, T 2 )
33 Forward measure 5 Again: L(t, T 1, T 2 ) = E Q t [L(T 1, T 1, T 2 )]. L(t, T 1, T 2 ) = EQ t [exp( T 2 t r(s)ds)l(t 1, T 1, T 2 )]. P (t, T 2 ) Note how the forward LIBOR rate L(t, T 1, T 2 ) is a martingale under the measure Q but NOT under the risk-neutral equivalent martingale measure Q. Note further that the second expression for L(t, T 1, T 2 ) is much more complicated since it involves an expectation under Q of the two product of two stochastic quantities exp( T 2 t r(s)ds) and L(T 1, T 1, T 2 ) and, furthermore, these quantities are certainly NOT mathematically independent.
34 Summary so far Let us summarize and review our analysis so far: It is possible to perfectly hedge an option on a zero-coupon bond by dynamic trading in zero coupon bonds of two different maturities. Under the risk-neutral equivalent martingale measure Q, zero-coupon bonds have a drift, at time t, equal to r(t). Under the forward measure Q, the forward bond price and the forward LIBOR rate are martingales. They are NOT martingales under the risk-neutral equivalent martingale measure Q.
35 Caplet pricing formulae We will now derive two specific caplet pricing formulae under two different assumptions for the dynamics of bond prices or forward LIBOR rates. Note that these formulae are not consistent with one another. They are consistent with the assumptions made to derive them but these assumptions are different for the two cases. The first specific caplet pricing formula comes from making the assumption that zero-coupon bond prices have a deterministic (lognormal) volatility. In other words, in equation (7), the terms σ P (t, T i ), for i equals 1 or 2 are deterministic and zero-coupon bond prices are lognormally distributed.
36 First caplet pricing formula - lognormal bond prices - 1 We wanted to price an option on a zero-coupon bond paying max(k P (T 1, T 2 ), 0) at time T 1 but so as to be able to price both call and put options, we consider a payoff max(η(k P (T 1, T 2 )), 0) at time T 1, where η is 1 or 1. In other words, But C(t) P (t, T 2 )G(t). Therefore, from (9): C(T 1 ) = max(η(k P (T 1, T 2 )), 0). G(T 1 ) = C(T 1 )/P (T 1, T 2 ) = max(η(k P (T 1, T 2 )), 0)/P (T 1, T 2 ) = K max ( 1 η( P (T 1, T 2 ) 1 K ), 0) = K max ( η( P (T 1, T 1 ) P (T 1, T 2 ) 1 K ), 0), since P (T 1, T 1 ) 1 = K max(η(f (T 1, T 2 ) 1 K ), 0). Therefore, the payoff in terms of G(T 1 ) is just like K call (if η = 1) options on the forward price struck at 1/K. We can write down the price in terms of G(t) because it is the same as the Black-Scholes formula with zero interest-rates, zero dividend yield and volatility Σ(t).
37 First caplet pricing formula - lognormal bond prices - 2 This uses the fact that, because the bond volatilities σ P (t, T i ) are deterministic, so is Σ(t). The option price in terms of G(t) is: G(t) = ηk ( F (t, T 2 )N(ηd 1 ) (1/K )N(ηd 2 ) ) = ηk ( P (t, T 1 ) P (t, T 2 ) N(ηd 1) (1/K )N(ηd 2 ) ). Note that (conveniently) this equation does NOT involve r(t).
38 First caplet pricing formula - lognormal bond prices - 3 The option price in terms of C(t) P (t, T 2 )G(t) is: where C(t) = P (t, T 2 )ηk ( P (t, T 1 ) P (t, T 2 ) N(ηd 1) (1/K )N(ηd 2 ) ) C(t) = K η ( P (t, T 1 )N(ηd 1 ) (1/K )P (t, T 2 )N(ηd 2 ) ) C(t) = η(k P (t, T 1 )N(ηd 1 ) P (t, T 2 )N(ηd 2 )), d 1 = log((p (t, T 1)/P (t, T 2 ))/(1/K )) ˆΣ 2 (T 1 t) ˆΣ(T 1 t) 1/2, d 2 = d 1 ˆΣ(T 1 t) 1/2. and ˆΣ is defined by: ˆΣ 2 (T 1 t) = T1 t ( ) σp(s, 2 T 1 ) + σp(s, 2 T 2 ) ρ 12 σ P (s, T 1 )σ P (s, T 2 ) ds.
39 First caplet pricing formula - lognormal bond prices - 4 From slide Caplet 3, a caplet is equivalent to (1 + Kτ)/τ options with strike K 1/(1 + Kτ) paying max(k P (T 1, T 2 ), 0) at time T 1. So from above, the caplet price, at time t, is: ((1 + Kτ)/τ)η(K P (t, T 1 )N(ηd 1 ) P (t, T 2 )N(ηd 2 )) = ((1 + Kτ)/τ)η((1/(1 + Kτ))P (t, T 1 )N(ηd 1 ) P (t, T 2 )N(ηd 2 )) = (1/τ)η(P (t, T 1 )N(ηd 1 ) (1 + Kτ)P (t, T 2 )N(ηd 2 )) = (1/τ)ηP (t, T 2 )((1 + τl(t, T 1, T 2 ))N(ηd 1 ) (1 + τk)n(ηd 2 )). (14)
40 First caplet pricing formula - lognormal bond prices - 5 Now all we need is a specific form for the bond volatilities σ P (t, T i ): There is more than one possibility for this. We will limit ourselves to mentioning only one. In the extended Vasicek model (also known as a one factor Hull and White model or a one factor Gaussian Heath, Jarrow and Morton model), we have: σ P (t, T i ) = σ r α (1 exp( α(t i t))), where σ r and α are positive constants. It is a one-factor model, so ρ 12 = 1. Pricing a caplet is now a trivial exercise in high-school algebra in computing ˆΣ 2.
41 Discussion on first caplet pricing formula The extended Vasicek (Hull and White) model has a number of attractive features (which you will learn about in detail on another day). It is a no-arbitrage model which is automatically consistent with any initial term-structure of interest-rates and it can be used to, very efficiently, price a very wide range of exotic (i.e. non-standard) interest-rate derivatives. However, in terms of pricing caps, the formula above is, in practice, mostly used in reverse: One uses the formula above in calibrating the extended Vasicek model i.e. to determine the values of the model parameters (σ r and α) which give the best fit to the market prices of caps (in practice, vanilla (standard European) swaptions are also often used). The market-standard model is the Black (1976) model. The Black (1976) model assumes that the forward LIBOR rate underlying the cap is lognormally distributed with volatility σ L.
42 Second caplet pricing formula - Black (lognormal LIBOR rates) - 1 We have already seen that under the forward measure Q, the forward LIBOR rate is a martingale. We assume it follows a SDE under Q as follows: dl(t, T 1, T 2 ) L(t, T 1, T 2 ) = σ Ldz Q (t). (15) We know the caplet payoff max(l(t 1, T 1, T 2 ) K, 0) at time T 2 is completely equivalent to a payoff of P (T 1, T 2 ) max(l(t 1, T 1, T 2 ) K, 0) at time T 1. Now, we use equation (11): C(t) = P (t, T 2 )E Q t = P (t, T 2 )E Q t [P (T 1, T 2 ) max(l(t 1, T 1, T 2 ) K, 0) ] P (T 1, T 2 ) [ max(l(t1, T 1, T 2 ) K, 0) ]. (16)
43 Second caplet pricing formula - Black (lognormal LIBOR rates) - 2 Equations (15) and (16) and comparison with the standard Black-Scholes formula enable us to write down the pricing formula immediately. The caplet price, at time t, is: where, here, C(t) = P (t, T 2 )(L(t, T 1, T 2 )N(d 1 ) KN(d 2 )), d 1 = log(l(t, T 1, T 2 )/K) σ2 L (T 1 t) σ L (T 1 t) 1/2 and d 2 = d 1 σ L (T 1 t) 1/2. Note that P (t, T 2 ) appears as the discounting term. In the formulae for d 1 and d 2, it is T 1 which appears. This is intuitive - the optionality finishes at time T 1 (since the payoff will then be known with certainty) although the actual payoff is not paid until time T 2.
44 Discussion on second caplet pricing formula i.e. Black (1976) As already remarked, the market-standard model is the Black (1976) model. It is, in fact, more than a model - it is the market price quotation system. In other words, cap prices are quoted in the market as Black (1976) implied volatilities for a stated maturity and strike. Because these implied volatilities, in practice, vary with strike (which is contrary to what is implied by the assumptions of the model), there is a sense in which the Black (1976) model represents the wrong volatility number to put into wrong formula to give the right price. Nonetheless, it is the market-standard model and the LIBOR market model (also known as the BGM model (after Brace, Gatarek and Musiela)) is based upon it.
45 3 month LIBOR futures contracts We have already seen that a FRA is a forward contract on a LIBOR rate. We will now discuss futures contracts on LIBOR rates. We will refer to them as 3 month LIBOR futures contracts (sometimes they are called 3 month Eurodollar futures contracts but the term Eurodollar is a slight misnomer as such contracts occur in all the major currencies (USD, EUR, STG, AUD, CAD, JPY, etc)). In practice, all these contracts are on 3 month LIBOR (or an equivalent 3 month rate) but there is nothing special about the 3 month term and our analysis does not depend on it. We mention a few practicalities first: These 3 month LIBOR futures contracts are extremely actively traded. The amounts traded are huge and the bid-offer spreads are tiny (often equivalent to on a futures price of, say, eg. the difference between an implied LIBOR interest-rate of, say, % and %). In USD, 3 month LIBOR futures contracts trade with a futures maturity of upto ten years (and in other currencies, for upto five years or more).
46 3 month LIBOR futures contracts 2 How do 3 month LIBOR futures contracts work? The delivery (or settlement) price is 100 minus a 3 month LIBOR rate expressed as a percentage. We denote the futures contract maturity by T 1. At time T 1, the LIBOR rate L(T 1, T 1, T 2 ) underlying the futures contract is observed. Then 100(1 L(T 1, T 1, T 2 )) is the delivery (or settlement) price of the futures contract. For 3 month LIBOR futures contracts, τ T 2 T 1 is approximately 0.25 years although we will not assume that. Our aim is to derive the theoretical value of this futures contract, at time t, with t < T 1. Before we can do that, we need to fully understand how futures contracts work.
47 3 month LIBOR futures contracts 3 Like a forward contract, it costs nothing up-front to enter into a futures contract. However, whereas with a forward contract, a single exchange of cashflows takes place at maturity, with futures contracts, there is a continuous (in practice, daily) mark-to-market procedure. An example helps: Suppose we buy a futures contract on 8th February when its market futures price is Suppose its end-of-day settlement futures price (i.e. the closing futures price) on the exchange on 8th February is An amount of = 0.12 (i.e. our gain) is paid into our account on that day by the exchange. Suppose we keep the position and its end-of-day settlement futures price on the exchange on 9th February is We have to pay an amount of = 0.15 to the exchange on that day. Suppose we keep the position and its end-of-day settlement futures price on the exchange on 10th February is We have to pay an amount of = 0.11 to the exchange on that day. (To clarify even more, this means we will have paid a net amount of = 0.14 = to the exchange by that time). This mark-to-market procedure continues until the futures contract matures at time T 1.
48 3 month LIBOR futures contracts 4 Our aim is to derive the theoretical value of this futures contract, at time t, with t < T 1. In other words, what value should be placed at time t to enter into a futures contract whose delivery price, at time T 1, is 100(1 L(T 1, T 1, T 2 )). Note two further key points: 1./ We use the word value rather than price to emphasise that is costs nothing up-front to enter into a futures contract. 2./ Obviously, it is the market that sets the market futures price. We are aiming to derive the theoretical value as it will show key points about futures contracts and their relationship to forward contracts.
49 3 month LIBOR futures contracts 5 We let the theoretical value of the futures contract, at time t, be G(t, T 1, T 2 ). The specification of the delivery price implies: G(T 1, T 1, T 2 ) = 100(1 L(T 1, T 1, T 2 )) = 100(1 1 τ ( 1 P (T 1, T 2 ) 1) ). (17) As with pricing FRA s and caplets, we will go through a replication or hedging argument. In fact, the derivation is very similar to that for caplets.
50 3 month LIBOR futures contracts 6 It is intuitive that G(t, T 1, T 2 ) actually depends on both P (t, T 1 ) and P (t, T 2 ). That is to say: G(t) G(t, T 1, T 2 ) G(t, P (t, T 1 ), P (t, T 2 )). Using the multi-dimensional form of Ito s lemma and writing P (t, T 1 ) P 1 and P (t, T 2 ) P 2 : dg(t) = G(t) dt + G(t) (µ 1 dt + σ P (t, T 1 )dz1 P (t))p 1 + G(t) (µ 2 dt + σ P (t, T 2 )dz2 P (t))p 2 t P 1 P 2 ( 1 2 G(t) + 2 P1 2 σp(t, 2 T 1 )P G(t) 2 P2 2 σp(t, 2 T 2 )P2 2 2 G(t) ) + ρ 12 σ P (t, T 1 )σ P (t, T 2 )P 1 P 2 dt. P 1 P 2 As before, ρ 12 is the (assumed deterministic) instantaneous correlation between dz P 1 (t) and dz P 2 (t).
51 3 month LIBOR futures contracts 7 We set up a self-financing portfolio consisting, at time t, of long one futures contract, short G(t) P 1 bonds maturing at time T 1 and short G(t) P 2 bonds maturing at time T 2. We do not hold any position in cash at any time (which means that, for example, any gains from the futures contract are invested in bonds). The portfolio always has zero net aggregate investment. The value of the portfolio at time t is: 0 = 0 G(t) P 1 P 1 G(t) P 2 P 2. (18) The left-hand-side of the last equation is simply the statement of zero net aggregate investment, for all t. The 0 on the right-hand-side is the statement that there is no up-front cost to buying (or selling) a futures contract. It is clear that, in order to satisfy equation (18), G(t) P 1 and G(t) P 2 must be of different sign.
52 3 month LIBOR futures contracts 8 The change in the value of the portfolio during the period t to t + dt is: dg(t) G(t) P 1 dp 1 G(t) P 2 dp 2 = G(t) dt t ( G(t) P1 2 σp(t, 2 T 1 )P G(t) P2 2 σp(t, 2 T 2 )P2 2 + ρ 12 2 G(t) P 1 P 2 σ P (t, T 1 )σ P (t, T 2 )P 1 P 2 ) dt, where we used slide 3 month LIBOR futures contracts 6. Note that the terms with dz P 1 (t) and dz P 2 (t) have cancelled so this is an instantaneously risk-free portfolio. The portfolio is always worth zero - because it always has zero net aggregate investment. Therefore, in the absence of arbitrage, it must have a realised return of exactly zero, for all t. Therefore: dg(t) G(t) P 1 dp 1 G(t) P 2 dp 2 = 0.
53 3 month LIBOR futures contracts 9 Therefore: 0 = G(t) t G(t) P1 2 σp(t, 2 T 1 )P G(t) P2 2 σp(t, 2 T 2 )P2 2 + ρ 12 2 G(t) P 1 P 2 σ P (t, T 1 )σ P (t, T 2 )P 1 P 2. Note that this is the same pde as we derived for caplets (although the zero net aggregate investment condition is different). We must solve it together with the conditions defined by equations (17) and (18).
54 3 month LIBOR futures contracts 10 To do this, we make the assumption that zero-coupon bond prices have a deterministic (lognormal) volatility (eg. as in the extended Vasicek model). We guess a solution of the form: G(t, T 1, T 2 ) = 100(1 1 τ (P (t, T 1 ) P (t, T 2 ) exp(υ(t, T 1, T 2 )) 1 ) ), where Υ(t) Υ(t, T 1, T 2 ) is a purely deterministic quantity (to be found). Then we have: G(t) = 1 ( Υ(t) P (t, T 1 ) t τ t P (t, T 2 ) exp(υ(t, T 1, T 2 )) ) 2 G(t), P1 2 = 0, 2 G(t) P2 2 = 1 (2P (t, T 1 ) τ P (t, T 2 ) exp(υ(t, T 1, T 3 2 )) ), 2 G(t) = 1 ( 1 P 1 P 2 τ P (t, T 2 ) exp(υ(t, T 1, T 2 2 )) ).
55 3 month LIBOR futures contracts 11 Substituting into the pde (and simplifying) we get: Hence, Υ(t) = 0 = Υ(t) t T1 t + σ 2 P(t, T 2 ) ρ 12 σ P (t, T 1 )σ P (t, T 2 ). ( ) σp(s, 2 T 2 ) ρ 12 σ P (s, T 1 )σ P (s, T 2 ) ds. Once we substitute the specific forms for σ P (t, T 1 ) and σ P (t, T 2 ) (eg. from the extended Vasicek model on slide First caplet pricing formula - lognormal bond prices - 5 ), we can trivially compute a specific form for Υ(t).
56 3 month LIBOR futures contracts 12 It is straightforward to verify that equations (17) and (18) are satisfied. So, our solution is: G(t, T 1, T 2 ) = 100(1 1 τ (P (t, T 1 ) P (t, T 2 ) exp(υ(t, T 1, T 2 )) 1 ) ).
57 3 month LIBOR futures contracts 13 We make two important points. The first point shows that G(t, T 1, T 2 ) is a martingale under the risk-neutral equivalent martingale measure Q. To see this, define H(t) H(t, T 1, T 2 ) via: H(t, T 1, T 2 ) P (t, T 1) P (t, T 2 ) exp(υ(t, T 1, T 2 )). Under Q, the dynamics of zero coupon bond prices are: dp (t, T i ) P (t, T i ) = r(t)dt + σ P(t, T i )dz Q i (t). (19) Then, by Ito s lemma, (after a little algebra - I want you to prove this at the computer lab) the dynamics of H(t) are: dh(t) H(t) = σ P(t, T 1 )dz Q 1 (t) σ P(t, T 2 )dz Q 2 (t). (20)
58 3 month LIBOR futures contracts 14 Therefore, H(t) is a martingale under Q and hence G(t, T 1, T 2 ) is also martingale under the risk-neutral equivalent martingale measure Q. We saw in slide Summary so far, that the forward LIBOR rate L(t, T 1, T 2 ) is a martingale under the measure Q but NOT under the risk-neutral equivalent martingale measure Q. By contrast, G(t, T 1, T 2 ) (which is the value of a futures contract on forward LIBOR) is a martingale under the risk-neutral equivalent martingale measure Q (but NOT under the measure Q ). This is a highly intuitive result.
59 3 month LIBOR futures contracts 15 The second point is as follows: Note that if interest-rates were to be deterministic, then σ P (t, T 1 ) and σ P (t, T 2 ) would be zero and Υ(t) would be zero so that G(t, T 1, T 2 ) would be equal to 100(1 L(t, T 1, T 2 )) - in other words, if interest-rates were to be deterministic then the value of the futures contract would be essentially (ignoring constant factors) the same as a FRA rate (which is the fair rate on a forward contract on forward LIBOR). However, in general, we can write: Υ(t) = T1 t Covar ( dp (s, T 2 ) P (s, T 2 ), dh(s) ) ds. H(s) We certainly expect Υ(t) to be positive because we expect bond prices of different maturities to be positively correlated. Therefore, we expect G(t, T 1, T 2 ) to be less than 100(1 L(t, T 1, T 2 )).
60 3 month LIBOR futures contracts 16 Let us think about the intuition in being long a futures contract. If the market futures price goes up, then I will be paid the price change by the exchange which I can then place on deposit. If the market price goes down, then I will have to borrow in order to pay the price change to the exchange. But the interest-rate that I will get on my deposit or pay on my borrowing is negatively correlated with the market futures price (since the market futures price is essentially proportional to one minus an interest-rate). Therefore, on average, when market futures price goes up, I will receive a lower rate on deposit for my gains and, on average, when market futures price goes down, I will have to pay a higher interest-rate on my borrowings to fund my losses. Therefore, on average, I seem to lose whichever way interest-rates move.
61 3 month LIBOR futures contracts 17 In an arbitrage-free market, I expect this to be reflected in the prices in the market. Therefore, I expect G(t, T 1, T 2 ) to be less than 100(1 L(t, T 1, T 2 )), which is what we have already seen. Note that this effect is purely a function of interest-rate volatility (and its consequences for correlations between changes in interest-rates and changes in market futures prices) - it has got nothing to do with, for example, the shape of the yield curve. This effect is known as the convexity adjustment or convexity bias. The effect is very significant, especially for long-dated futures contracts i.e. those for which T 1 is quite large (we have already noted 3 month LIBOR futures contracts trade with a futures maturity of upto ten years i.e. T 1 t can be approximately 10). In the computer lab, we will quantify this effect.
62 Futures contracts to build yield curves We have derived the theoretical value of a 3 month LIBOR futures contract. Of course, the market sets the market futures price. The theoretical value is most useful in solving the reverse problem: Given Υ(t, T 1, T 2 ) and given the market futures price, what does it imply for forward LIBOR rates and hence for discount factors. In other words, the market futures prices of 3 month LIBOR futures contracts (with different T 1 ) are used to build yield-curves. If I fail to take into account the effects of the convexity adjustment term (i.e. of Υ(t, T 1, T 2 )), then my yield-curve will be wrong. Then I will also misprice FRA s, swaps and other interest-rate derivatives.
63 Futures contracts to build yield curves 2 In the early 1990 s, a number of financial institutions are reputed to have lost a lot of money (and others to have made a lot of money (reputed to be > 500 million US dollars)) by failing to recognise the necessity of incorporating the convexity adjustment term when valuing 3 month LIBOR futures contracts or when building yield-curves from market futures prices. The financial institutions that lost a lot of money were effectively (and erroneously) always assuming that Υ(t, T 1, T 2 ) = 0.
64 Summary and General Conclusions We have priced FRA s, caplets and 3 month LIBOR futures contracts. As with pricing stock options, the key to pricing these instruments is to construct replicating portfolios or hedges and then the assumption of no-arbitrage gives us pricing formulae. Foward LIBOR rates are martingales under the forward measure Q whereas futures contracts on forward LIBOR rates are martingales under the risk-neutral equivalent martingale measure Q. The tool of changing measure from Q to Q is a vital tool that you will come across in greater detail later in your course.
The Black-Scholes Formula
 FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price
 On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
Bond Options, Caps and the Black Model
 Bond Options, Caps and the Black Model Black formula Recall the Black formula for pricing options on futures: C(F, K, σ, r, T, r) = Fe rt N(d 1 ) Ke rt N(d 2 ) where d 1 = 1 [ σ ln( F T K ) + 1 ] 2 σ2
Bond Options, Caps and the Black Model Black formula Recall the Black formula for pricing options on futures: C(F, K, σ, r, T, r) = Fe rt N(d 1 ) Ke rt N(d 2 ) where d 1 = 1 [ σ ln( F T K ) + 1 ] 2 σ2
INTEREST RATES AND FX MODELS
 INTEREST RATES AND FX MODELS 4. Convexity and CMS Andrew Lesniewski Courant Institute of Mathematical Sciences New York University New York February 20, 2013 2 Interest Rates & FX Models Contents 1 Introduction
INTEREST RATES AND FX MODELS 4. Convexity and CMS Andrew Lesniewski Courant Institute of Mathematical Sciences New York University New York February 20, 2013 2 Interest Rates & FX Models Contents 1 Introduction
Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008
 : A Stern School of Business New York University Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008 Outline 1 2 3 4 5 6 se notes review the principles underlying option pricing and some of
: A Stern School of Business New York University Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008 Outline 1 2 3 4 5 6 se notes review the principles underlying option pricing and some of
CS 522 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options
 CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
Determination of Forward and Futures Prices. Chapter 5
 Determination of Forward and Futures Prices Chapter 5 Fundamentals of Futures and Options Markets, 8th Ed, Ch 5, Copyright John C. Hull 2013 1 Consumption vs Investment Assets Investment assets are assets
Determination of Forward and Futures Prices Chapter 5 Fundamentals of Futures and Options Markets, 8th Ed, Ch 5, Copyright John C. Hull 2013 1 Consumption vs Investment Assets Investment assets are assets
Bootstrapping the interest-rate term structure
 Bootstrapping the interest-rate term structure Marco Marchioro www.marchioro.org October 20 th, 2012 Bootstrapping the interest-rate term structure 1 Summary (1/2) Market quotes of deposit rates, IR futures,
Bootstrapping the interest-rate term structure Marco Marchioro www.marchioro.org October 20 th, 2012 Bootstrapping the interest-rate term structure 1 Summary (1/2) Market quotes of deposit rates, IR futures,
Session IX: Lecturer: Dr. Jose Olmo. Module: Economics of Financial Markets. MSc. Financial Economics
 Session IX: Stock Options: Properties, Mechanics and Valuation Lecturer: Dr. Jose Olmo Module: Economics of Financial Markets MSc. Financial Economics Department of Economics, City University, London Stock
Session IX: Stock Options: Properties, Mechanics and Valuation Lecturer: Dr. Jose Olmo Module: Economics of Financial Markets MSc. Financial Economics Department of Economics, City University, London Stock
Chapter 1: Financial Markets and Financial Derivatives
 Chapter 1: Financial Markets and Financial Derivatives 1.1 Financial Markets Financial markets are markets for financial instruments, in which buyers and sellers find each other and create or exchange
Chapter 1: Financial Markets and Financial Derivatives 1.1 Financial Markets Financial markets are markets for financial instruments, in which buyers and sellers find each other and create or exchange
On Market-Making and Delta-Hedging
 On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
Finance 350: Problem Set 6 Alternative Solutions
 Finance 350: Problem Set 6 Alternative Solutions Note: Where appropriate, the final answer for each problem is given in bold italics for those not interested in the discussion of the solution. I. Formulas
Finance 350: Problem Set 6 Alternative Solutions Note: Where appropriate, the final answer for each problem is given in bold italics for those not interested in the discussion of the solution. I. Formulas
Caput Derivatives: October 30, 2003
 Caput Derivatives: October 30, 2003 Exam + Answers Total time: 2 hours and 30 minutes. Note 1: You are allowed to use books, course notes, and a calculator. Question 1. [20 points] Consider an investor
Caput Derivatives: October 30, 2003 Exam + Answers Total time: 2 hours and 30 minutes. Note 1: You are allowed to use books, course notes, and a calculator. Question 1. [20 points] Consider an investor
Eurodollar Futures, and Forwards
 5 Eurodollar Futures, and Forwards In this chapter we will learn about Eurodollar Deposits Eurodollar Futures Contracts, Hedging strategies using ED Futures, Forward Rate Agreements, Pricing FRAs. Hedging
5 Eurodollar Futures, and Forwards In this chapter we will learn about Eurodollar Deposits Eurodollar Futures Contracts, Hedging strategies using ED Futures, Forward Rate Agreements, Pricing FRAs. Hedging
The Irony In The Derivatives Discounting
 The Irony In The Derivatives Discounting Marc Henrard Head of Quantitative Research, Banking Department, Bank for International Settlements, CH-4002 Basel (Switzerland), e-mail: Marc.Henrard@bis.org Abstract
The Irony In The Derivatives Discounting Marc Henrard Head of Quantitative Research, Banking Department, Bank for International Settlements, CH-4002 Basel (Switzerland), e-mail: Marc.Henrard@bis.org Abstract
Computational Finance Options
 1 Options 1 1 Options Computational Finance Options An option gives the holder of the option the right, but not the obligation to do something. Conversely, if you sell an option, you may be obliged to
1 Options 1 1 Options Computational Finance Options An option gives the holder of the option the right, but not the obligation to do something. Conversely, if you sell an option, you may be obliged to
Lecture 12. Options Strategies
 Lecture 12. Options Strategies Introduction to Options Strategies Options, Futures, Derivatives 10/15/07 back to start 1 Solutions Problem 6:23: Assume that a bank can borrow or lend money at the same
Lecture 12. Options Strategies Introduction to Options Strategies Options, Futures, Derivatives 10/15/07 back to start 1 Solutions Problem 6:23: Assume that a bank can borrow or lend money at the same
Pricing Forwards and Futures I: The Basic Theory
 Chapter 3.1 Introduction 3 Pricing Forwards and Futures I: The Basic Theory This chapter and the next mark the begining of our exploration into the pricing of derivative securities. This chapter is mainly
Chapter 3.1 Introduction 3 Pricing Forwards and Futures I: The Basic Theory This chapter and the next mark the begining of our exploration into the pricing of derivative securities. This chapter is mainly
1 The Black-Scholes model: extensions and hedging
 1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
Master s Thesis. Pricing Constant Maturity Swap Derivatives
 Master s Thesis Pricing Constant Maturity Swap Derivatives Thesis submitted in partial fulfilment of the requirements for the Master of Science degree in Stochastics and Financial Mathematics by Noemi
Master s Thesis Pricing Constant Maturity Swap Derivatives Thesis submitted in partial fulfilment of the requirements for the Master of Science degree in Stochastics and Financial Mathematics by Noemi
Introduction to Arbitrage-Free Pricing: Fundamental Theorems
 Introduction to Arbitrage-Free Pricing: Fundamental Theorems Dmitry Kramkov Carnegie Mellon University Workshop on Interdisciplinary Mathematics, Penn State, May 8-10, 2015 1 / 24 Outline Financial market
Introduction to Arbitrage-Free Pricing: Fundamental Theorems Dmitry Kramkov Carnegie Mellon University Workshop on Interdisciplinary Mathematics, Penn State, May 8-10, 2015 1 / 24 Outline Financial market
Valuing Stock Options: The Black-Scholes-Merton Model. Chapter 13
 Valuing Stock Options: The Black-Scholes-Merton Model Chapter 13 Fundamentals of Futures and Options Markets, 8th Ed, Ch 13, Copyright John C. Hull 2013 1 The Black-Scholes-Merton Random Walk Assumption
Valuing Stock Options: The Black-Scholes-Merton Model Chapter 13 Fundamentals of Futures and Options Markets, 8th Ed, Ch 13, Copyright John C. Hull 2013 1 The Black-Scholes-Merton Random Walk Assumption
Option Valuation. Chapter 21
 Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Lecture 4: The Black-Scholes model
 OPTIONS and FUTURES Lecture 4: The Black-Scholes model Philip H. Dybvig Washington University in Saint Louis Black-Scholes option pricing model Lognormal price process Call price Put price Using Black-Scholes
OPTIONS and FUTURES Lecture 4: The Black-Scholes model Philip H. Dybvig Washington University in Saint Louis Black-Scholes option pricing model Lognormal price process Call price Put price Using Black-Scholes
Martingale Pricing Applied to Options, Forwards and Futures
 IEOR E4706: Financial Engineering: Discrete-Time Asset Pricing Fall 2005 c 2005 by Martin Haugh Martingale Pricing Applied to Options, Forwards and Futures We now apply martingale pricing theory to the
IEOR E4706: Financial Engineering: Discrete-Time Asset Pricing Fall 2005 c 2005 by Martin Haugh Martingale Pricing Applied to Options, Forwards and Futures We now apply martingale pricing theory to the
How To Price A Call Option
 Now by Itô s formula But Mu f and u g in Ū. Hence τ θ u(x) =E( Mu(X) ds + u(x(τ θ))) 0 τ θ u(x) E( f(x) ds + g(x(τ θ))) = J x (θ). 0 But since u(x) =J x (θ ), we consequently have u(x) =J x (θ ) = min
Now by Itô s formula But Mu f and u g in Ū. Hence τ θ u(x) =E( Mu(X) ds + u(x(τ θ))) 0 τ θ u(x) E( f(x) ds + g(x(τ θ))) = J x (θ). 0 But since u(x) =J x (θ ), we consequently have u(x) =J x (θ ) = min
Forwards, Swaps and Futures
 IEOR E4706: Financial Engineering: Discrete-Time Models c 2010 by Martin Haugh Forwards, Swaps and Futures These notes 1 introduce forwards, swaps and futures, and the basic mechanics of their associated
IEOR E4706: Financial Engineering: Discrete-Time Models c 2010 by Martin Haugh Forwards, Swaps and Futures These notes 1 introduce forwards, swaps and futures, and the basic mechanics of their associated
Determination of Forward and Futures Prices
 Determination of Forward and Futures Prices Options, Futures, and Other Derivatives, 8th Edition, Copyright John C. Hull 2012 Short selling A popular trading (arbitrage) strategy is the shortselling or
Determination of Forward and Futures Prices Options, Futures, and Other Derivatives, 8th Edition, Copyright John C. Hull 2012 Short selling A popular trading (arbitrage) strategy is the shortselling or
Interest Rate and Currency Swaps
 Interest Rate and Currency Swaps Eiteman et al., Chapter 14 Winter 2004 Bond Basics Consider the following: Zero-Coupon Zero-Coupon One-Year Implied Maturity Bond Yield Bond Price Forward Rate t r 0 (0,t)
Interest Rate and Currency Swaps Eiteman et al., Chapter 14 Winter 2004 Bond Basics Consider the following: Zero-Coupon Zero-Coupon One-Year Implied Maturity Bond Yield Bond Price Forward Rate t r 0 (0,t)
Exam MFE Spring 2007 FINAL ANSWER KEY 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D
 Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008
 FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 Options These notes consider the way put and call options and the underlying can be combined to create hedges, spreads and combinations. We will consider the
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 Options These notes consider the way put and call options and the underlying can be combined to create hedges, spreads and combinations. We will consider the
Chapter 5 Financial Forwards and Futures
 Chapter 5 Financial Forwards and Futures Question 5.1. Four different ways to sell a share of stock that has a price S(0) at time 0. Question 5.2. Description Get Paid at Lose Ownership of Receive Payment
Chapter 5 Financial Forwards and Futures Question 5.1. Four different ways to sell a share of stock that has a price S(0) at time 0. Question 5.2. Description Get Paid at Lose Ownership of Receive Payment
Institutional Finance 08: Dynamic Arbitrage to Replicate Non-linear Payoffs. Binomial Option Pricing: Basics (Chapter 10 of McDonald)
 Copyright 2003 Pearson Education, Inc. Slide 08-1 Institutional Finance 08: Dynamic Arbitrage to Replicate Non-linear Payoffs Binomial Option Pricing: Basics (Chapter 10 of McDonald) Originally prepared
Copyright 2003 Pearson Education, Inc. Slide 08-1 Institutional Finance 08: Dynamic Arbitrage to Replicate Non-linear Payoffs Binomial Option Pricing: Basics (Chapter 10 of McDonald) Originally prepared
Option pricing. Vinod Kothari
 Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
Lecture 21 Options Pricing
 Lecture 21 Options Pricing Readings BM, chapter 20 Reader, Lecture 21 M. Spiegel and R. Stanton, 2000 1 Outline Last lecture: Examples of options Derivatives and risk (mis)management Replication and Put-call
Lecture 21 Options Pricing Readings BM, chapter 20 Reader, Lecture 21 M. Spiegel and R. Stanton, 2000 1 Outline Last lecture: Examples of options Derivatives and risk (mis)management Replication and Put-call
Mathematical Finance
 Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
Pricing Forwards and Swaps
 Chapter 7 Pricing Forwards and Swaps 7. Forwards Throughout this chapter, we will repeatedly use the following property of no-arbitrage: P 0 (αx T +βy T ) = αp 0 (x T )+βp 0 (y T ). Here, P 0 (w T ) is
Chapter 7 Pricing Forwards and Swaps 7. Forwards Throughout this chapter, we will repeatedly use the following property of no-arbitrage: P 0 (αx T +βy T ) = αp 0 (x T )+βp 0 (y T ). Here, P 0 (w T ) is
Assumptions: No transaction cost, same rate for borrowing/lending, no default/counterparty risk
 Derivatives Why? Allow easier methods to short sell a stock without a broker lending it. Facilitates hedging easily Allows the ability to take long/short position on less available commodities (Rice, Cotton,
Derivatives Why? Allow easier methods to short sell a stock without a broker lending it. Facilitates hedging easily Allows the ability to take long/short position on less available commodities (Rice, Cotton,
Pricing Interest-Rate- Derivative Securities
 Pricing Interest-Rate- Derivative Securities John Hull Alan White University of Toronto This article shows that the one-state-variable interest-rate models of Vasicek (1977) and Cox, Ingersoll, and Ross
Pricing Interest-Rate- Derivative Securities John Hull Alan White University of Toronto This article shows that the one-state-variable interest-rate models of Vasicek (1977) and Cox, Ingersoll, and Ross
The Behavior of Bonds and Interest Rates. An Impossible Bond Pricing Model. 780 w Interest Rate Models
 780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
Forward Price. The payoff of a forward contract at maturity is S T X. Forward contracts do not involve any initial cash flow.
 Forward Price The payoff of a forward contract at maturity is S T X. Forward contracts do not involve any initial cash flow. The forward price is the delivery price which makes the forward contract zero
Forward Price The payoff of a forward contract at maturity is S T X. Forward contracts do not involve any initial cash flow. The forward price is the delivery price which makes the forward contract zero
Derivatives: Principles and Practice
 Derivatives: Principles and Practice Rangarajan K. Sundaram Stern School of Business New York University New York, NY 10012 Sanjiv R. Das Leavey School of Business Santa Clara University Santa Clara, CA
Derivatives: Principles and Practice Rangarajan K. Sundaram Stern School of Business New York University New York, NY 10012 Sanjiv R. Das Leavey School of Business Santa Clara University Santa Clara, CA
The interest volatility surface
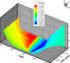 The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
CFA Level -2 Derivatives - I
 CFA Level -2 Derivatives - I EduPristine www.edupristine.com Agenda Forwards Markets and Contracts Future Markets and Contracts Option Markets and Contracts 1 Forwards Markets and Contracts 2 Pricing and
CFA Level -2 Derivatives - I EduPristine www.edupristine.com Agenda Forwards Markets and Contracts Future Markets and Contracts Option Markets and Contracts 1 Forwards Markets and Contracts 2 Pricing and
Guaranteed Annuity Options
 Guaranteed Annuity Options Hansjörg Furrer Market-consistent Actuarial Valuation ETH Zürich, Frühjahrssemester 2008 Guaranteed Annuity Options Contents A. Guaranteed Annuity Options B. Valuation and Risk
Guaranteed Annuity Options Hansjörg Furrer Market-consistent Actuarial Valuation ETH Zürich, Frühjahrssemester 2008 Guaranteed Annuity Options Contents A. Guaranteed Annuity Options B. Valuation and Risk
第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model
 1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
Advanced Fixed Income Analytics Lecture 1
 Advanced Fixed Income Analytics Lecture 1 Backus & Zin/April 1, 1999 Vasicek: The Fixed Income Benchmark 1. Prospectus 2. Models and their uses 3. Spot rates and their properties 4. Fundamental theorem
Advanced Fixed Income Analytics Lecture 1 Backus & Zin/April 1, 1999 Vasicek: The Fixed Income Benchmark 1. Prospectus 2. Models and their uses 3. Spot rates and their properties 4. Fundamental theorem
Lecture 6 Black-Scholes PDE
 Lecture 6 Black-Scholes PDE Lecture Notes by Andrzej Palczewski Computational Finance p. 1 Pricing function Let the dynamics of underlining S t be given in the risk-neutral measure Q by If the contingent
Lecture 6 Black-Scholes PDE Lecture Notes by Andrzej Palczewski Computational Finance p. 1 Pricing function Let the dynamics of underlining S t be given in the risk-neutral measure Q by If the contingent
EXP 481 -- Capital Markets Option Pricing. Options: Definitions. Arbitrage Restrictions on Call Prices. Arbitrage Restrictions on Call Prices 1) C > 0
 EXP 481 -- Capital Markets Option Pricing imple arbitrage relations Payoffs to call options Black-choles model Put-Call Parity Implied Volatility Options: Definitions A call option gives the buyer the
EXP 481 -- Capital Markets Option Pricing imple arbitrage relations Payoffs to call options Black-choles model Put-Call Parity Implied Volatility Options: Definitions A call option gives the buyer the
Pricing of an Exotic Forward Contract
 Pricing of an Exotic Forward Contract Jirô Akahori, Yuji Hishida and Maho Nishida Dept. of Mathematical Sciences, Ritsumeikan University 1-1-1 Nojihigashi, Kusatsu, Shiga 525-8577, Japan E-mail: {akahori,
Pricing of an Exotic Forward Contract Jirô Akahori, Yuji Hishida and Maho Nishida Dept. of Mathematical Sciences, Ritsumeikan University 1-1-1 Nojihigashi, Kusatsu, Shiga 525-8577, Japan E-mail: {akahori,
Introduction to Options. Derivatives
 Introduction to Options Econ 422: Investment, Capital & Finance University of Washington Summer 2010 August 18, 2010 Derivatives A derivative is a security whose payoff or value depends on (is derived
Introduction to Options Econ 422: Investment, Capital & Finance University of Washington Summer 2010 August 18, 2010 Derivatives A derivative is a security whose payoff or value depends on (is derived
FIN 472 Fixed-Income Securities Forward Rates
 FIN 472 Fixed-Income Securities Forward Rates Professor Robert B.H. Hauswald Kogod School of Business, AU Interest-Rate Forwards Review of yield curve analysis Forwards yet another use of yield curve forward
FIN 472 Fixed-Income Securities Forward Rates Professor Robert B.H. Hauswald Kogod School of Business, AU Interest-Rate Forwards Review of yield curve analysis Forwards yet another use of yield curve forward
Learning Curve Interest Rate Futures Contracts Moorad Choudhry
 Learning Curve Interest Rate Futures Contracts Moorad Choudhry YieldCurve.com 2004 Page 1 The market in short-term interest rate derivatives is a large and liquid one, and the instruments involved are
Learning Curve Interest Rate Futures Contracts Moorad Choudhry YieldCurve.com 2004 Page 1 The market in short-term interest rate derivatives is a large and liquid one, and the instruments involved are
The Black-Scholes Model
 Chapter 4 The Black-Scholes Model 4. Introduction Easily the best known model of option pricing, the Black-Scholes model is also one of the most widely used models in practice. It forms the benchmark model
Chapter 4 The Black-Scholes Model 4. Introduction Easily the best known model of option pricing, the Black-Scholes model is also one of the most widely used models in practice. It forms the benchmark model
How To Calculate Interest Rate Derivative Options
 The Pricing and Hedging of Interest-Rate Derivatives: Theory and Practice Ser-Huang Poon 1, Richard C. Stapleton 2 and Marti G. Subrahmanyam 3 April 28, 2005 1 Manchester Business School 2 Manchester Business
The Pricing and Hedging of Interest-Rate Derivatives: Theory and Practice Ser-Huang Poon 1, Richard C. Stapleton 2 and Marti G. Subrahmanyam 3 April 28, 2005 1 Manchester Business School 2 Manchester Business
No-arbitrage conditions for cash-settled swaptions
 No-arbitrage conditions for cash-settled swaptions Fabio Mercurio Financial Engineering Banca IMI, Milan Abstract In this note, we derive no-arbitrage conditions that must be satisfied by the pricing function
No-arbitrage conditions for cash-settled swaptions Fabio Mercurio Financial Engineering Banca IMI, Milan Abstract In this note, we derive no-arbitrage conditions that must be satisfied by the pricing function
Black-Scholes Equation for Option Pricing
 Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
INTEREST RATE SWAP (IRS)
 INTEREST RATE SWAP (IRS) 1. Interest Rate Swap (IRS)... 4 1.1 Terminology... 4 1.2 Application... 11 1.3 EONIA Swap... 19 1.4 Pricing and Mark to Market Revaluation of IRS... 22 2. Cross Currency Swap...
INTEREST RATE SWAP (IRS) 1. Interest Rate Swap (IRS)... 4 1.1 Terminology... 4 1.2 Application... 11 1.3 EONIA Swap... 19 1.4 Pricing and Mark to Market Revaluation of IRS... 22 2. Cross Currency Swap...
Chapter 3: Commodity Forwards and Futures
 Chapter 3: Commodity Forwards and Futures In the previous chapter we study financial forward and futures contracts and we concluded that are all alike. Each commodity forward, however, has some unique
Chapter 3: Commodity Forwards and Futures In the previous chapter we study financial forward and futures contracts and we concluded that are all alike. Each commodity forward, however, has some unique
A BOUND ON LIBOR FUTURES PRICES FOR HJM YIELD CURVE MODELS
 A BOUND ON LIBOR FUTURES PRICES FOR HJM YIELD CURVE MODELS VLADIMIR POZDNYAKOV AND J. MICHAEL STEELE Abstract. We prove that for a large class of widely used term structure models there is a simple theoretical
A BOUND ON LIBOR FUTURES PRICES FOR HJM YIELD CURVE MODELS VLADIMIR POZDNYAKOV AND J. MICHAEL STEELE Abstract. We prove that for a large class of widely used term structure models there is a simple theoretical
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies
 Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
One Period Binomial Model
 FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 One Period Binomial Model These notes consider the one period binomial model to exactly price an option. We will consider three different methods of pricing
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 One Period Binomial Model These notes consider the one period binomial model to exactly price an option. We will consider three different methods of pricing
Explaining the Lehman Brothers Option Adjusted Spread of a Corporate Bond
 Fixed Income Quantitative Credit Research February 27, 2006 Explaining the Lehman Brothers Option Adjusted Spread of a Corporate Bond Claus M. Pedersen Explaining the Lehman Brothers Option Adjusted Spread
Fixed Income Quantitative Credit Research February 27, 2006 Explaining the Lehman Brothers Option Adjusted Spread of a Corporate Bond Claus M. Pedersen Explaining the Lehman Brothers Option Adjusted Spread
The Black-Scholes pricing formulas
 The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
On the Valuation of Power-Reverse Duals and Equity-Rates Hybrids
 On the Valuation of Power-Reverse Duals and Equity-Rates Hybrids Oliver Caps oliver.caps@dkib.com RMT Model Validation Rates Dresdner Bank Examples of Hybrid Products Pricing of Hybrid Products using a
On the Valuation of Power-Reverse Duals and Equity-Rates Hybrids Oliver Caps oliver.caps@dkib.com RMT Model Validation Rates Dresdner Bank Examples of Hybrid Products Pricing of Hybrid Products using a
Option Pricing. Chapter 11 Options on Futures. Stefan Ankirchner. University of Bonn. last update: 13/01/2014 at 14:25
 Option Pricing Chapter 11 Options on Futures Stefan Ankirchner University of Bonn last update: 13/01/2014 at 14:25 Stefan Ankirchner Option Pricing 1 Agenda Forward contracts Definition Determining forward
Option Pricing Chapter 11 Options on Futures Stefan Ankirchner University of Bonn last update: 13/01/2014 at 14:25 Stefan Ankirchner Option Pricing 1 Agenda Forward contracts Definition Determining forward
Convenient Conventions
 C: call value. P : put value. X: strike price. S: stock price. D: dividend. Convenient Conventions c 2015 Prof. Yuh-Dauh Lyuu, National Taiwan University Page 168 Payoff, Mathematically Speaking The payoff
C: call value. P : put value. X: strike price. S: stock price. D: dividend. Convenient Conventions c 2015 Prof. Yuh-Dauh Lyuu, National Taiwan University Page 168 Payoff, Mathematically Speaking The payoff
Jung-Soon Hyun and Young-Hee Kim
 J. Korean Math. Soc. 43 (2006), No. 4, pp. 845 858 TWO APPROACHES FOR STOCHASTIC INTEREST RATE OPTION MODEL Jung-Soon Hyun and Young-Hee Kim Abstract. We present two approaches of the stochastic interest
J. Korean Math. Soc. 43 (2006), No. 4, pp. 845 858 TWO APPROACHES FOR STOCHASTIC INTEREST RATE OPTION MODEL Jung-Soon Hyun and Young-Hee Kim Abstract. We present two approaches of the stochastic interest
BINOMIAL OPTION PRICING
 Darden Graduate School of Business Administration University of Virginia BINOMIAL OPTION PRICING Binomial option pricing is a simple but powerful technique that can be used to solve many complex option-pricing
Darden Graduate School of Business Administration University of Virginia BINOMIAL OPTION PRICING Binomial option pricing is a simple but powerful technique that can be used to solve many complex option-pricing
The Intuition Behind Option Valuation: A Teaching Note
 The Intuition Behind Option Valuation: A Teaching Note Thomas Grossman Haskayne School of Business University of Calgary Steve Powell Tuck School of Business Dartmouth College Kent L Womack Tuck School
The Intuition Behind Option Valuation: A Teaching Note Thomas Grossman Haskayne School of Business University of Calgary Steve Powell Tuck School of Business Dartmouth College Kent L Womack Tuck School
Manual for SOA Exam FM/CAS Exam 2.
 Manual for SOA Exam FM/CAS Exam 2. Chapter 7. Derivatives markets. c 2009. Miguel A. Arcones. All rights reserved. Extract from: Arcones Manual for the SOA Exam FM/CAS Exam 2, Financial Mathematics. Fall
Manual for SOA Exam FM/CAS Exam 2. Chapter 7. Derivatives markets. c 2009. Miguel A. Arcones. All rights reserved. Extract from: Arcones Manual for the SOA Exam FM/CAS Exam 2, Financial Mathematics. Fall
Review of Basic Options Concepts and Terminology
 Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
Introduction to Mathematical Finance
 Introduction to Mathematical Finance Martin Baxter Barcelona 11 December 2007 1 Contents Financial markets and derivatives Basic derivative pricing and hedging Advanced derivatives 2 Banking Retail banking
Introduction to Mathematical Finance Martin Baxter Barcelona 11 December 2007 1 Contents Financial markets and derivatives Basic derivative pricing and hedging Advanced derivatives 2 Banking Retail banking
Two-State Options. John Norstad. j-norstad@northwestern.edu http://www.norstad.org. January 12, 1999 Updated: November 3, 2011.
 Two-State Options John Norstad j-norstad@northwestern.edu http://www.norstad.org January 12, 1999 Updated: November 3, 2011 Abstract How options are priced when the underlying asset has only two possible
Two-State Options John Norstad j-norstad@northwestern.edu http://www.norstad.org January 12, 1999 Updated: November 3, 2011 Abstract How options are priced when the underlying asset has only two possible
Interest rate Derivatives
 Interest rate Derivatives There is a wide variety of interest rate options available. The most widely offered are interest rate caps and floors. Increasingly we also see swaptions offered. This note will
Interest rate Derivatives There is a wide variety of interest rate options available. The most widely offered are interest rate caps and floors. Increasingly we also see swaptions offered. This note will
Moreover, under the risk neutral measure, it must be the case that (5) r t = µ t.
 LECTURE 7: BLACK SCHOLES THEORY 1. Introduction: The Black Scholes Model In 1973 Fisher Black and Myron Scholes ushered in the modern era of derivative securities with a seminal paper 1 on the pricing
LECTURE 7: BLACK SCHOLES THEORY 1. Introduction: The Black Scholes Model In 1973 Fisher Black and Myron Scholes ushered in the modern era of derivative securities with a seminal paper 1 on the pricing
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com
 Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Some Practical Issues in FX and Equity Derivatives
 Some Practical Issues in FX and Equity Derivatives Phenomenology of the Volatility Surface The volatility matrix is the map of the implied volatilities quoted by the market for options of different strikes
Some Practical Issues in FX and Equity Derivatives Phenomenology of the Volatility Surface The volatility matrix is the map of the implied volatilities quoted by the market for options of different strikes
Overview. Option Basics. Options and Derivatives. Professor Lasse H. Pedersen. Option basics and option strategies
 Options and Derivatives Professor Lasse H. Pedersen Prof. Lasse H. Pedersen 1 Overview Option basics and option strategies No-arbitrage bounds on option prices Binomial option pricing Black-Scholes-Merton
Options and Derivatives Professor Lasse H. Pedersen Prof. Lasse H. Pedersen 1 Overview Option basics and option strategies No-arbitrage bounds on option prices Binomial option pricing Black-Scholes-Merton
ASimpleMarketModel. 2.1 Model Assumptions. Assumption 2.1 (Two trading dates)
 2 ASimpleMarketModel In the simplest possible market model there are two assets (one stock and one bond), one time step and just two possible future scenarios. Many of the basic ideas of mathematical finance
2 ASimpleMarketModel In the simplest possible market model there are two assets (one stock and one bond), one time step and just two possible future scenarios. Many of the basic ideas of mathematical finance
Option Pricing Beyond Black-Scholes Dan O Rourke
 Option Pricing Beyond Black-Scholes Dan O Rourke January 2005 1 Black-Scholes Formula (Historical Context) Produced a usable model where all inputs were easily observed Coincided with the introduction
Option Pricing Beyond Black-Scholes Dan O Rourke January 2005 1 Black-Scholes Formula (Historical Context) Produced a usable model where all inputs were easily observed Coincided with the introduction
The new ACI Diploma. Unit 2 Fixed Income & Money Markets. Effective October 2014
 The new ACI Diploma Unit 2 Fixed Income & Money Markets Effective October 2014 8 Rue du Mail, 75002 Paris - France T: +33 1 42975115 - F: +33 1 42975116 - www.aciforex.org The new ACI Diploma Objective
The new ACI Diploma Unit 2 Fixed Income & Money Markets Effective October 2014 8 Rue du Mail, 75002 Paris - France T: +33 1 42975115 - F: +33 1 42975116 - www.aciforex.org The new ACI Diploma Objective
Forward Contracts and Forward Rates
 Forward Contracts and Forward Rates Outline and Readings Outline Forward Contracts Forward Prices Forward Rates Information in Forward Rates Reading Veronesi, Chapters 5 and 7 Tuckman, Chapters 2 and 16
Forward Contracts and Forward Rates Outline and Readings Outline Forward Contracts Forward Prices Forward Rates Information in Forward Rates Reading Veronesi, Chapters 5 and 7 Tuckman, Chapters 2 and 16
Miscellaneous. Simone Freschi freschi.simone@gmail.com Tommaso Gabriellini tommaso.gabriellini@mpscs.it. Università di Siena
 Miscellaneous Simone Freschi freschi.simone@gmail.com Tommaso Gabriellini tommaso.gabriellini@mpscs.it Head of Global MPS Capital Services SpA - MPS Group Università di Siena ini A.A. 2014-2015 1 / 1 A
Miscellaneous Simone Freschi freschi.simone@gmail.com Tommaso Gabriellini tommaso.gabriellini@mpscs.it Head of Global MPS Capital Services SpA - MPS Group Università di Siena ini A.A. 2014-2015 1 / 1 A
FIXED-INCOME SECURITIES. Chapter 11. Forwards and Futures
 FIXED-INCOME SECURITIES Chapter 11 Forwards and Futures Outline Futures and Forwards Types of Contracts Trading Mechanics Trading Strategies Futures Pricing Uses of Futures Futures and Forwards Forward
FIXED-INCOME SECURITIES Chapter 11 Forwards and Futures Outline Futures and Forwards Types of Contracts Trading Mechanics Trading Strategies Futures Pricing Uses of Futures Futures and Forwards Forward
Fixed Income Portfolio Management. Interest rate sensitivity, duration, and convexity
 Fixed Income ortfolio Management Interest rate sensitivity, duration, and convexity assive bond portfolio management Active bond portfolio management Interest rate swaps 1 Interest rate sensitivity, duration,
Fixed Income ortfolio Management Interest rate sensitivity, duration, and convexity assive bond portfolio management Active bond portfolio management Interest rate swaps 1 Interest rate sensitivity, duration,
Options Pricing. This is sometimes referred to as the intrinsic value of the option.
 Options Pricing We will use the example of a call option in discussing the pricing issue. Later, we will turn our attention to the Put-Call Parity Relationship. I. Preliminary Material Recall the payoff
Options Pricing We will use the example of a call option in discussing the pricing issue. Later, we will turn our attention to the Put-Call Parity Relationship. I. Preliminary Material Recall the payoff
24. Pricing Fixed Income Derivatives. through Black s Formula. MA6622, Ernesto Mordecki, CityU, HK, 2006. References for this Lecture:
 24. Pricing Fixed Income Derivatives through Black s Formula MA6622, Ernesto Mordecki, CityU, HK, 2006. References for this Lecture: John C. Hull, Options, Futures & other Derivatives (Fourth Edition),
24. Pricing Fixed Income Derivatives through Black s Formula MA6622, Ernesto Mordecki, CityU, HK, 2006. References for this Lecture: John C. Hull, Options, Futures & other Derivatives (Fourth Edition),
Pricing Frameworks for Securitization of Mortality Risk
 1 Pricing Frameworks for Securitization of Mortality Risk Andrew Cairns Heriot-Watt University, Edinburgh Joint work with David Blake & Kevin Dowd Updated version at: http://www.ma.hw.ac.uk/ andrewc 2
1 Pricing Frameworks for Securitization of Mortality Risk Andrew Cairns Heriot-Watt University, Edinburgh Joint work with David Blake & Kevin Dowd Updated version at: http://www.ma.hw.ac.uk/ andrewc 2
VALUATION IN DERIVATIVES MARKETS
 VALUATION IN DERIVATIVES MARKETS September 2005 Rawle Parris ABN AMRO Property Derivatives What is a Derivative? A contract that specifies the rights and obligations between two parties to receive or deliver
VALUATION IN DERIVATIVES MARKETS September 2005 Rawle Parris ABN AMRO Property Derivatives What is a Derivative? A contract that specifies the rights and obligations between two parties to receive or deliver
Barrier Options. Peter Carr
 Barrier Options Peter Carr Head of Quantitative Financial Research, Bloomberg LP, New York Director of the Masters Program in Math Finance, Courant Institute, NYU March 14th, 2008 What are Barrier Options?
Barrier Options Peter Carr Head of Quantitative Financial Research, Bloomberg LP, New York Director of the Masters Program in Math Finance, Courant Institute, NYU March 14th, 2008 What are Barrier Options?
Published in Journal of Investment Management, Vol. 13, No. 1 (2015): 64-83
 Published in Journal of Investment Management, Vol. 13, No. 1 (2015): 64-83 OIS Discounting, Interest Rate Derivatives, and the Modeling of Stochastic Interest Rate Spreads John Hull and Alan White Joseph
Published in Journal of Investment Management, Vol. 13, No. 1 (2015): 64-83 OIS Discounting, Interest Rate Derivatives, and the Modeling of Stochastic Interest Rate Spreads John Hull and Alan White Joseph
Hedging with Futures and Options: Supplementary Material. Global Financial Management
 Hedging with Futures and Options: Supplementary Material Global Financial Management Fuqua School of Business Duke University 1 Hedging Stock Market Risk: S&P500 Futures Contract A futures contract on
Hedging with Futures and Options: Supplementary Material Global Financial Management Fuqua School of Business Duke University 1 Hedging Stock Market Risk: S&P500 Futures Contract A futures contract on
Call and Put. Options. American and European Options. Option Terminology. Payoffs of European Options. Different Types of Options
 Call and Put Options A call option gives its holder the right to purchase an asset for a specified price, called the strike price, on or before some specified expiration date. A put option gives its holder
Call and Put Options A call option gives its holder the right to purchase an asset for a specified price, called the strike price, on or before some specified expiration date. A put option gives its holder
Jorge Cruz Lopez - Bus 316: Derivative Securities. Week 11. The Black-Scholes Model: Hull, Ch. 13.
 Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
OPTIONS, FUTURES, & OTHER DERIVATI
 Fifth Edition OPTIONS, FUTURES, & OTHER DERIVATI John C. Hull Maple Financial Group Professor of Derivatives and Risk Manage, Director, Bonham Center for Finance Joseph L. Rotinan School of Management
Fifth Edition OPTIONS, FUTURES, & OTHER DERIVATI John C. Hull Maple Financial Group Professor of Derivatives and Risk Manage, Director, Bonham Center for Finance Joseph L. Rotinan School of Management
Lecture. S t = S t δ[s t ].
![Lecture. S t = S t δ[s t ]. Lecture. S t = S t δ[s t ].](/thumbs/28/12512709.jpg) Lecture In real life the vast majority of all traded options are written on stocks having at least one dividend left before the date of expiration of the option. Thus the study of dividends is important
Lecture In real life the vast majority of all traded options are written on stocks having at least one dividend left before the date of expiration of the option. Thus the study of dividends is important
Chapter 11 Options. Main Issues. Introduction to Options. Use of Options. Properties of Option Prices. Valuation Models of Options.
 Chapter 11 Options Road Map Part A Introduction to finance. Part B Valuation of assets, given discount rates. Part C Determination of risk-adjusted discount rate. Part D Introduction to derivatives. Forwards
Chapter 11 Options Road Map Part A Introduction to finance. Part B Valuation of assets, given discount rates. Part C Determination of risk-adjusted discount rate. Part D Introduction to derivatives. Forwards
Introduction to Binomial Trees
 11 C H A P T E R Introduction to Binomial Trees A useful and very popular technique for pricing an option involves constructing a binomial tree. This is a diagram that represents di erent possible paths
11 C H A P T E R Introduction to Binomial Trees A useful and very popular technique for pricing an option involves constructing a binomial tree. This is a diagram that represents di erent possible paths
THE FUNDAMENTAL THEOREM OF ARBITRAGE PRICING
 THE FUNDAMENTAL THEOREM OF ARBITRAGE PRICING 1. Introduction The Black-Scholes theory, which is the main subject of this course and its sequel, is based on the Efficient Market Hypothesis, that arbitrages
THE FUNDAMENTAL THEOREM OF ARBITRAGE PRICING 1. Introduction The Black-Scholes theory, which is the main subject of this course and its sequel, is based on the Efficient Market Hypothesis, that arbitrages
