Lecture 18 Time-dependent perturbation theory
|
|
|
- Eugene Shaw
- 7 years ago
- Views:
Transcription
1 Lecture 18 Time-dependent perturbation theory
2 Time-dependent perturbation theory So far, we have focused on quantum mechanics of systems described by Hamiltonians that are time-independent. In such cases, time dependence of wavefunction developed through time-evolution operator, Û = e iĥt/, i.e. for Ĥ n = E n n, ψ(t) = e iĥt/ ψ(0) }{{} P n c n(0) n = n e ie nt/ c n (0) n Although suitable for closed quantum systems, formalism fails to describe interaction with an external environment, e.g. EM field. In such cases, more convenient to describe induced interactions of small isolated system, Ĥ0, through time-dependent interaction V (t). In this lecture, we will develop a formalism to treat such time-dependent perturbations.
3 Time-dependent perturbation theory So far, we have focused on quantum mechanics of systems described by Hamiltonians that are time-independent. In such cases, time dependence of wavefunction developed through time-evolution operator, Û = e iĥt/, i.e. for Ĥ n = E n n, ψ(t) = e iĥt/ ψ(0) }{{} P n c n(0) n = n e ie nt/ c n (0) n Although suitable for closed quantum systems, formalism fails to describe interaction with an external environment, e.g. EM field. In such cases, more convenient to describe induced interactions of small isolated system, Ĥ0, through time-dependent interaction V (t). In this lecture, we will develop a formalism to treat such time-dependent perturbations.
4 Time-dependent perturbation theory: outline Time-dependent potentials: general formalism Time-dependent perturbation theory Sudden perturbation Harmonic perturbations: Fermi s Golden Rule
5 Time-dependent potentials: general formalism Consider Hamiltonian Ĥ(t) =Ĥ0 + V (t), where all time dependence enters through the potential V (t). So far, we have focused on Schrödinger representation, where dynamics specified by time-dependent wavefunction, i t ψ(t) S = Ĥ ψ(t) S However, to develop time-dependent perturbation theory for Ĥ(t) =Ĥ 0 + V (t), it is convenient to turn to a new representation known as the Interaction representation: ψ(t) I = e iĥ 0 t/ ψ(t) S, ψ(0) I = ψ(0) S
6 Time-dependent potentials: general formalism Consider Hamiltonian Ĥ(t) =Ĥ0 + V (t), where all time dependence enters through the potential V (t). So far, we have focused on Schrödinger representation, where dynamics specified by time-dependent wavefunction, i t ψ(t) S = Ĥ ψ(t) S However, to develop time-dependent perturbation theory for Ĥ(t) =Ĥ 0 + V (t), it is convenient to turn to a new representation known as the Interaction representation: ψ(t) I = e iĥ 0 t/ ψ(t) S, ψ(0) I = ψ(0) S
7 Time-dependent potentials: general formalism ψ(t) I = e iĥ0t/ ψ(t) S, ψ(0) I = ψ(0) S In the interaction representation, wavefunction obeys the following equation of motion: We therefore have that i t ψ(t) I = e iĥ 0 t/ (i t Ĥ0) ψ(t) S = e iĥ0t/ (Ĥ Ĥ 0 ) ψ(t) S = e iĥ 0 t/ V (t)e iĥ 0 t/ ψ(t) I }{{} V I (t) i t ψ(t) I = V I (t) ψ(t) I, V I (t) =e iĥ 0 t/ V (t)e iĥ 0 t/
8 Time-dependent potentials: general formalism i t ψ(t) I = V I (t) ψ(t) I, V I (t) =e iĥ0t/ V (t)e iĥ0t/ Then, if we form eigenfunction expansion, ψ(t) I = n c n(t) n, where Ĥ0 n = E n n, i t c n (t) n = e iĥ0t/ V (t)e iĥ0t/ c n (t) n n n i n ċ n (t) n = n c n (t)e iĥ0t/ V (t) e iĥ0t/ n }{{} e ie nt/ n If we now contract with a general state m ċ n (t) m n = c n (t) m e iĥ 0 t/ V (t)e ient/ n }{{}}{{} n n δ mn m e ie mt/ i ċ m (t) = n m V (t) n e i(e m E n )t/ c n (t)
9 Time-dependent potentials: general formalism i t ψ(t) I = V I (t) ψ(t) I, V I (t) =e iĥ0t/ V (t)e iĥ0t/ Then, if we form eigenfunction expansion, ψ(t) I = n c n(t) n, where Ĥ0 n = E n n, i t c n (t) n = e iĥ0t/ V (t)e iĥ0t/ c n (t) n n n i n ċ n (t) n = n c n (t)e iĥ0t/ V (t) e iĥ0t/ n }{{} e ie nt/ n If we now contract with a general state m ċ n (t) m n = c n (t) m e iĥ 0 t/ V (t)e ient/ n }{{}}{{} n n δ mn m e ie mt/ i ċ m (t) = n m V (t) n e i(e m E n )t/ c n (t)
10 Time-dependent potentials: general formalism i ċ m (t) = n m V (t) n e i(e m E n )t/ c n (t) So, in summary, if we expand wavefunction ψ(t) I = n c n(t) n, where Ĥ 0 n = E n n, the Schrödinger equation, i t ψ(t) I = V I (t) ψ(t) I with V I (t) =e iĥ 0 t/ V (t)e iĥ 0 t/ translates to the relation, i ċ m (t) = n V mn (t)e iω mnt c n (t) where V mn (t) = m V (t) m and ω mn = 1 (E m E n )= ω nm.
11 Example: Dynamics of a driven two-level system i ċ m (t) = n V mn (t)e iω mnt c n (t) Consider an atom with just two available atomic levels, 1 and 2, with energies E 1 and E 2. In the eigenbasis, the time-independent Hamiltonian can be written as ( ) E1 0 Ĥ 0 = E E E 2 Note that the two-level atom mirrors a spin 1/2 system. If the system is driven by an electric field, E(r, t) =E 0 (r) cos(ωt), and the states have different parity, close to resonance, ω ω 21 ω 21, the effective interaction potential is given by ( ) V (t) δe iωt δe iω e iωt 2 1 δ e iω where the matrix element, δ = 1 E 2 is presumed real.
12 Example: Dynamics of a driven two-level system i ċ m (t) = n V mn (t)e iω mnt c n (t) Consider an atom with just two available atomic levels, 1 and 2, with energies E 1 and E 2. In the eigenbasis, the time-independent Hamiltonian can be written as ( ) E1 0 Ĥ 0 = E E E 2 Note that the two-level atom mirrors a spin 1/2 system. If the system is driven by an electric field, E(r, t) =E 0 (r) cos(ωt), and the states have different parity, close to resonance, ω ω 21 ω 21, the effective interaction potential is given by ( ) V (t) δe iωt δe iω e iωt 2 1 δ e iω where the matrix element, δ = 1 E 2 is presumed real.
13 Example: Dynamics of a driven two-level system Ĥ 0 + V (t) = ( ) E1 0 0 E 2 + δ ( 0 e iωt e iω ) The electric field therefore induces transitions between the states. If we expand the spinor-like wavefunction in eigenstates of Ĥ0, i.e. ψ(t) I = c 1 (t) 1 + c 2 (t) 2, the equation i ċ m (t) = n V mn (t)e iω mnt c n (t) translates to the quantum dynamics ( 0 e i t c = δ i(ω ω 21)t e i(ω ω 21)t 0 ) c(t), ω 21 = 1 (E 2 E 1 ) where c(t) =(c 1 (t) c 2 (t)).
14 Example: Dynamics of a driven two-level system i t c = δ ( 0 e i(ω ω 21)t e i(ω ω 21)t 0 ) c(t), ω 21 = 1 (E 2 E 1 ) Expanding this equation, we find i ċ 1 = δe i(ω ω 21)t c 2, i ċ 2 = δe i(ω ω 21)t c 1 from which we obtain an equation for c 2, c 2 (t)+ i(ω ω 21 )ċ 2 (t)+ ( δ ) 2 c 2 (t) =0 With the initial conditions, c 1 (0) = 1 and c 2 (0) = 0, i.e. particle starts in state 1, we obtain the solution, c 2 (t) =e i(ω ω 21)t/2 sin(ωt) where Ω = ((δ/ ) 2 +(ω ω 21 ) 2 /4) 1/2 is known as Rabi frequency.
15 Example: Dynamics of a driven two-level system i t c = δ ( 0 e i(ω ω 21)t e i(ω ω 21)t 0 ) c(t), ω 21 = 1 (E 2 E 1 ) Expanding this equation, we find i ċ 1 = δe i(ω ω 21)t c 2, i ċ 2 = δe i(ω ω 21)t c 1 from which we obtain an equation for c 2, c 2 (t)+ i(ω ω 21 )ċ 2 (t)+ ( δ ) 2 c 2 (t) =0 With the initial conditions, c 1 (0) = 1 and c 2 (0) = 0, i.e. particle starts in state 1, we obtain the solution, c 2 (t) =e i(ω ω 21)t/2 sin(ωt) where Ω = ((δ/ ) 2 +(ω ω 21 ) 2 /4) 1/2 is known as Rabi frequency.
16 Example: Dynamics of a driven two-level system c 2 (t) =Ae i(ω ω 21)t/2 sin(ωt), Ω= ( ( ) 2 δ + ( ) ) 2 1/2 ω ω21 2 Together with c 1 (t) = i δ ei(ω ω 21)tċ 2, we obtain the normalization, δ A = and /4 δ+ 2 (ω ω 2 21 c 2 (t) 2 = δ 2 δ (ω ω 21 ) 2 /4 sin2 Ωt, c 1 (t) 2 =1 c 2 (t) 2 Periodic solution describes transfer of probability between states 1 and 2. Maximum probability of occupying state 2 is Lorentzian, c 2 (t) 2 max = δ 2 δ (ω ω 21 ) 2 /4, taking the value of unity at resonance, ω = ω 21.
17 Example: Dynamics of a driven two-level system c 2 (t) =Ae i(ω ω 21)t/2 sin(ωt), Ω= ( ( ) 2 δ + ( ) ) 2 1/2 ω ω21 2 Together with c 1 (t) = i δ ei(ω ω 21)tċ 2, we obtain the normalization, δ A = and /4 δ+ 2 (ω ω 2 21 c 2 (t) 2 = δ 2 δ (ω ω 21 ) 2 /4 sin2 Ωt, c 1 (t) 2 =1 c 2 (t) 2 Periodic solution describes transfer of probability between states 1 and 2. Maximum probability of occupying state 2 is Lorentzian, c 2 (t) 2 max = δ 2 δ (ω ω 21 ) 2 /4, taking the value of unity at resonance, ω = ω 21.
18 Rabi oscillations: persistent current qubit It is different to prepare and analyse ideal atomic two-level system. However, circuits made of superconducting loops provide access to two-level systems. These have been of great interest since they (may yet) provide a platform to develop qubit operation and quantum logic circuits. By exciting transitions between levels using a microwave pulse, coherence of the system has been recorded through Rabi oscillations.
19 Rabi oscillations: persistent current qubit It is different to prepare and analyse ideal atomic two-level system. However, circuits made of superconducting loops provide access to two-level systems. These have been of great interest since they (may yet) provide a platform to develop qubit operation and quantum logic circuits. By exciting transitions between levels using a microwave pulse, coherence of the system has been recorded through Rabi oscillations.
20 Time-dependent perturbation theory For a general time-dependent Hamiltonian, Ĥ = Ĥ0 + V (t), an analytical solution is usually infeasible. However, as for the time-independent Schrödinger equation, we can develop to a perturbative expansion (in powers of interaction): ψ(t) I = n c n (t) n, c n (t) =c (0) n + c (1) n (t)+c (2) n (t)+ where Ĥ 0 n = E n n, c n (m) O(V m ), and c n (0) (time-independent) initial state of the system. represents some As with the Schrödinger representation, in the interaction representation, ψ(t) I related to inital state ψ( ) I, at time, through a time-evolution operator, ψ(t) I = Û I (t, ) ψ( ) I
21 Time-dependent perturbation theory For a general time-dependent Hamiltonian, Ĥ = Ĥ0 + V (t), an analytical solution is usually infeasible. However, as for the time-independent Schrödinger equation, we can develop to a perturbative expansion (in powers of interaction): ψ(t) I = n c n (t) n, c n (t) =c (0) n + c (1) n (t)+c (2) n (t)+ where Ĥ 0 n = E n n, c n (m) O(V m ), and c n (0) (time-independent) initial state of the system. represents some As with the Schrödinger representation, in the interaction representation, ψ(t) I related to inital state ψ( ) I, at time, through a time-evolution operator, ψ(t) I = Û I (t, ) ψ( ) I
22 Time-dependent perturbation theory ψ(t) I = Û I (t, ) ψ( ) I Substituted into Schrödinger equation i t ψ(t) I = V I (t) ψ(t) I, i t Û I (t, ) ψ( ) I = V I (t)ûi(t, ) ψ( ) I Since this is true for any initial state ψ( ) I, we must have i t Û I (t, )=V I (t)ûi(t, ) with the boundary condition U I (, )=I. Integrating to t, i dt t Û I (t, )=i (ÛI(t, ) I), i.e. Û I (t, )=I i dt V I (t )ÛI(t, ) provides self-consistent equation for U I (t, ),
23 Time-dependent perturbation theory ψ(t) I = Û I (t, ) ψ( ) I Substituted into Schrödinger equation i t ψ(t) I = V I (t) ψ(t) I, i t Û I (t, ) ψ( ) I = V I (t)ûi(t, ) ψ( ) I Since this is true for any initial state ψ( ) I, we must have i t Û I (t, )=V I (t)ûi(t, ) with the boundary condition U I (, )=I. Integrating to t, i dt t Û I (t, )=i (ÛI(t, ) I), i.e. Û I (t, )=I i dt V I (t )ÛI(t, ) provides self-consistent equation for U I (t, ),
24 Time-dependent perturbation theory ψ(t) I = Û I (t, ) ψ( ) I Substituted into Schrödinger equation i t ψ(t) I = V I (t) ψ(t) I, i t Û I (t, ) ψ( ) I = V I (t)ûi(t, ) ψ( ) I Since this is true for any initial state ψ( ) I, we must have i t Û I (t, )=V I (t)ûi(t, ) with the boundary condition U I (, )=I. Integrating to t, i dt t Û I (t, )=i (ÛI(t, ) I), i.e. Û I (t, )=I i dt V I (t )ÛI(t, ) provides self-consistent equation for U I (t, ),
25 Time-dependent perturbation theory Û I (t, )=I i dt V I (t )ÛI(t, ) If we substitute ÛI(t, ) on right hand side, Û I (t, )=I i dt V I (t ) ( + i ) 2 dt V I (t ) dt V I (t )Û I (t, ) Iterating this procedure, Û I (t, )= n=0 ( i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) where term n = 0 translates to I.
26 Time-dependent perturbation theory Û I (t, )=I i ( dt V I (t ) I i dt V I (t )Û I (t, ) ) If we substitute ÛI(t, ) on right hand side, Û I (t, )=I i dt V I (t ) ( + i ) 2 dt V I (t ) dt V I (t )Û I (t, ) Iterating this procedure, Û I (t, )= n=0 ( i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) where term n = 0 translates to I.
27 Time-dependent perturbation theory Û I (t, )=I i dt V I (t )ÛI(t, ) If we substitute ÛI(t, ) on right hand side, Û I (t, )=I i dt V I (t ) ( + i ) 2 dt V I (t ) dt V I (t )Û I (t, ) Iterating this procedure, Û I (t, )= n=0 ( i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) where term n = 0 translates to I.
28 Time-dependent perturbation theory Û I (t, )= n=0 ( i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) Remark: Since operators V I (t) appear as a time-ordered sequence, with t n t n 1 t 1 t this expression is sometimes written as [ Û I (t, )=T e i R t dt V I (t ) ] where T denotes the time-ordering operator and is understood as the identity above. Note that, for V independent of t, ÛI(t, )=e i Vt reminiscent of the usual time-evolution operator for time-independent Ĥ.
29 Time-dependent perturbation theory Û I (t, )= n=0 ( i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) If a system is prepared in an initial state, i at time t =, at a subsequent time, t, the system will be in a final state, ÛI(t, ) i. Using the resolution of identity, n n n = I, we therefore have Û I (t, ) i = n n c n (t) {}}{ n Û I (t, ) i From relation above, the coefficients in the expansion given by c n (t) =δ ni i 1 2 dt dt n V I (t ) i dt n V I (t )V I (t ) i +
30 Time-dependent perturbation theory Û I (t, )= n=0 ( i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) If a system is prepared in an initial state, i at time t =, at a subsequent time, t, the system will be in a final state, ÛI(t, ) i. Using the resolution of identity, n n n = I, we therefore have Û I (t, ) i = n n c n (t) {}}{ n Û I (t, ) i From relation above, the coefficients in the expansion given by c n (t) =δ ni i 1 2 dt dt n V I (t ) i dt n V I (t )V I (t ) i +
31 Time-dependent perturbation theory Û I (t, )= n=0 ( i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) If a system is prepared in an initial state, i at time t =, at a subsequent time, t, the system will be in a final state, ÛI(t, ) i. Using the resolution of identity, n n n = I, we therefore have Û I (t, ) i = n n c n (t) {}}{ n ÛI(t, ) i From relation above, the coefficients in the expansion given by c n (t) =δ ni i 1 2 dt dt n V I (t ) i dt m n V I (t ) m m V I (t ) i +
32 Time-dependent perturbation theory c n (t) =δ ni i 1 2 dt dt n V I (t ) i dt n V I (t ) m m V I (t ) i + m Recalling the definition, V I (t) =e iĥ 0 t/ V (t)e iĥ 0 t/, the matrix elements entering the coefficients are then given by n V I (t) m = n e iĥ0t/ V (t)e iĥ0t/ m [ ] i = n V (t) m exp }{{} (E n E m ) V }{{} nm e iω nmt where V nm (t) = n V (t) m denote matrix elements between the basis states of Ĥ0 on the perturbation, and ω nm =(E n E m )/.
33 Time-dependent perturbation theory c n (t) =δ ni i 1 2 dt dt n V I (t ) i dt n V I (t ) m m V I (t ) i + m Therefore, using the relation, n V I (t) m = n V (t) m e iω nmt, c n (1) (t) = i c n (2) (t) = 1 2 m dt e iω ni t dt V ni (t ) dt e iω nmt +iω mi t V nm (t )V mi (t ) As a result, we obtain transition probability i n i, P i n (t) = c n (t) 2 = c (1) n + c (2) n + 2
34 Example: Kicked oscillator Suppose quantum harmonic oscillator, Ĥ = ω(a a +1/2), prepared in ground state 0 at time t =. If it is perturbed by weak (transient) electric field, V (t) = eex e t2 /τ 2 what is probability of finding it in first excited state, 1, at t =+? Working to first order in V, P 0 1 c (1) 1 2 where c (1) 1 (t) = i dt e iω 10t V 10 (t ) with V 10 (t )= ee 1 x 0 e t 2 /τ 2 and ω 10 = ω
35 Example: Kicked oscillator Suppose quantum harmonic oscillator, Ĥ = ω(a a +1/2), prepared in ground state 0 at time t =. If it is perturbed by weak (transient) electric field, V (t) = eex e t2 /τ 2 what is probability of finding it in first excited state, 1, at t =+? Working to first order in V, P 0 1 c (1) 1 2 where c (1) 1 (t) = i dt e iω 10t V 10 (t ) with V 10 (t )= ee 1 x 0 e t 2 /τ 2 and ω 10 = ω
36 Example: Kicked oscillator c (1) 1 (t) = i dt e iωt V 10 (t ), V 10 (t )= ee 1 x 0 e t 2 /τ 2 Using the ladder operator formalism, with 1 = a 0 and x = 2mω (a + a ), 1 x 0 = 2mω 0 a(a + a ) 0 = With = dt e iωt e t 2 /τ 2 = πτ exp [ 14 ω2 τ 2 ], c (1) π 1 (t )=ieeτ 2 2m ω e ω τ 2 /4 2mω Transition probability, ( P 0 1 c (1) 1 (t) 2 =(eeτ) 2 π ) e ω2 τ 2 /2 2m ω Note that P 0 1 is maximal for τ 1/ω.
37 Example: Kicked oscillator c (1) 1 (t) = i dt e iωt V 10 (t ), V 10 (t )= ee 1 x 0 e t 2 /τ 2 Using the ladder operator formalism, with 1 = a 0 and x = 2mω (a + a ), 1 x 0 = 2mω 0 a(a + a ) 0 = With = dt e iωt e t 2 /τ 2 = πτ exp [ 14 ω2 τ 2 ], c (1) π 1 (t )=ieeτ 2 2m ω e ω τ 2 /4 2mω Transition probability, ( P 0 1 c (1) 1 (t) 2 =(eeτ) 2 π ) e ω2 τ 2 /2 2m ω Note that P 0 1 is maximal for τ 1/ω.
38 Example: Kicked oscillator c (1) 1 (t) = i dt e iωt V 10 (t ), V 10 (t )= ee 1 x 0 e t 2 /τ 2 Using the ladder operator formalism, with 1 = a 0 and x = 2mω (a + a ), 1 x 0 = 2mω 0 a(a + a ) 0 = With = dt e iωt e t 2 /τ 2 = πτ exp [ 14 ω2 τ 2 ], c (1) π 1 (t )=ieeτ 2 2m ω e ω τ 2 /4 2mω Transition probability, ( P 0 1 c (1) 1 (t) 2 =(eeτ) 2 π ) e ω2 τ 2 /2 2m ω Note that P 0 1 is maximal for τ 1/ω.
39 Sudden perturbation quantum quench Suppose there is a switch from Ĥ 0 to Ĥ 0 in a time shorter than any other characteristic scale perturbation theory is irrelevant: If system is initially in eigenstate n of Ĥ 0, time evolution after switch will just follow that of Ĥ 0, i.e. simply expand initial state as a sum over eigenstates of Ĥ 0, n = n n n n, n(t) = n e ie n t/ n n n Non-trivial part of the problem lies in establishing that the change is sudden enough. This is achieved by estimating the actual time taken for the Hamiltonian to change, and the periods of motion associated with the state n and with its transitions to neighbouring states.
40 Sudden perturbation quantum quench Suppose there is a switch from Ĥ 0 to Ĥ 0 in a time shorter than any other characteristic scale perturbation theory is irrelevant: If system is initially in eigenstate n of Ĥ 0, time evolution after switch will just follow that of Ĥ 0, i.e. simply expand initial state as a sum over eigenstates of Ĥ 0, n = n n n n, n(t) = n e ie n t/ n n n Non-trivial part of the problem lies in establishing that the change is sudden enough. This is achieved by estimating the actual time taken for the Hamiltonian to change, and the periods of motion associated with the state n and with its transitions to neighbouring states.
41 Harmonic perturbations: Fermi s Golden Rule Consider system prepared in initial state i and perturbed by a periodic harmonic potential V (t) =Ve iωt which is abruptly switched on at time t = 0. e.g. atom perturbed by an external oscillating electric field. What is the probability that, at some later time t, the system is in state f? To first order in perturbation theory, c (1) f (t) = i dt e iω fit V fi (t ) i.e. probability of effecting transition after a time t, P i f (t) c (1) f (t) 2 = i f V i ei(ω fi ω)t/2 sin((ω fi ω)t/2) (ω fi ω)/2 2
42 Harmonic perturbations: Fermi s Golden Rule Consider system prepared in initial state i and perturbed by a periodic harmonic potential V (t) =Ve iωt which is abruptly switched on at time t = 0. e.g. atom perturbed by an external oscillating electric field. What is the probability that, at some later time t, the system is in state f? To first order in perturbation theory, c (1) f (t) = i dt e iω fit V fi (t ) i.e. probability of effecting transition after a time t, P i f (t) c (1) f (t) 2 = i f V i ei(ω fi ω)t/2 sin((ω fi ω)t/2) (ω fi ω)/2 2
43 Harmonic perturbations: Fermi s Golden Rule Consider system prepared in initial state i and perturbed by a periodic harmonic potential V (t) =Ve iωt which is abruptly switched on at time t = 0. e.g. atom perturbed by an external oscillating electric field. What is the probability that, at some later time t, the system is in state f? To first order in perturbation theory, c (1) f (t) = i 0 dt f V i e i(ω fi ω)t = i f V i ei(ω fi ω)t 1 i(ω fi ω) i.e. probability of effecting transition after a time t, P i f (t) c (1) f (t) 2 = i f V i ei(ω fi ω)t/2 sin((ω fi ω)t/2) (ω fi ω)/2 2
44 Harmonic perturbations: Fermi s Golden Rule Consider system prepared in initial state i and perturbed by a periodic harmonic potential V (t) =Ve iωt which is abruptly switched on at time t = 0. e.g. atom perturbed by an external oscillating electric field. What is the probability that, at some later time t, the system is in state f? To first order in perturbation theory, c (1) f (t) = i 0 dt f V i e i(ω fi ω)t = i f V i ei(ω fi ω)t 1 i(ω fi ω) i.e. probability of effecting transition after a time t, P i f (t) c (1) f (t) 2 = i f V i ei(ω fi ω)t/2 sin((ω fi ω)t/2) (ω fi ω)/2 2
45 Harmonic perturbations: Fermi s Golden Rule Consider system prepared in initial state i and perturbed by a periodic harmonic potential V (t) =Ve iωt which is abruptly switched on at time t = 0. e.g. atom perturbed by an external oscillating electric field. What is the probability that, at some later time t, the system is in state f? To first order in perturbation theory, c (1) f (t) = i 0 dt f V i e i(ω fi ω)t = i f V i ei(ω fi ω)t 1 i(ω fi ω) i.e. probability of effecting transition after a time t, P i f (t) c (1) f (t) 2 = 1 ( ) 2 2 f V sin((ωfi ω)t/2) i 2 (ω fi ω)/2
46 Harmonic perturbations: Fermi s Golden Rule P i f (t) 1 2 f V i 2 ( sin((ωfi ω)t/2) (ω fi ω)/2 ) 2 Setting α =(ω fl ω)/2, probability sin 2 (αt)/α 2 with a peak at α = 0 maximum value t 2, width O(1/t) total weight O(t). ( ) 2 1 sin(αt) For large t, lim = πδ(α) =2πδ(2α) t t α Fermi s Golden rule: transition rate, R i f (t) = lim t P i f (t) t = 2π 2 f V i 2 δ(ω fi ω)
47 Harmonic perturbations: Fermi s Golden Rule P i f (t) 1 2 f V i 2 ( sin((ωfi ω)t/2) (ω fi ω)/2 ) 2 Setting α =(ω fl ω)/2, probability sin 2 (αt)/α 2 with a peak at α = 0 maximum value t 2, width O(1/t) total weight O(t). ( ) 2 1 sin(αt) For large t, lim = πδ(α) =2πδ(2α) t t α Fermi s Golden rule: transition rate, R i f (t) = lim t P i f (t) t = 2π 2 f V i 2 δ(ω fi ω)
48 Harmonic perturbations: Fermi s Golden Rule R i f (t) = 2π 2 f V i 2 δ(ω fi ω) This result shows that, for a transition to occur, to satisfy energy conservation we must have: (a) final states exist over a continuous energy range to match E = ω for fixed perturbation frequency ω, or (b) perturbation must cover sufficiently wide spectrum of frequency so that a discrete transition with E = ω is possible. For any two discrete pair of states i and f, since V fi 2 = V if 2, we have P i f = P f i statement of detailed balance.
49 Harmonic perturbations: second order transitions Although first order perturbation theory often sufficient, sometimes f V i = 0 by symmetry (e.g. parity, selection rules, etc.). In such cases, transition may be accomplished by indirect route through other non-zero matrix elements. At second order of perturbation theory, c (2) f (t) = 1 2 dt m dt e iω fmt +iω mi t V fm (t )V mi (t ) If harmonic potential perturbation is gradually switched on, V (t) =e εt Ve iωt, ε 0, with the initial time, c (2) f (t) = 1 2 f V m m V i m dt dt e i(ω fm ω iε)t e i(ω mi ω iε)t
50 Harmonic perturbations: second order transitions Although first order perturbation theory often sufficient, sometimes f V i = 0 by symmetry (e.g. parity, selection rules, etc.). In such cases, transition may be accomplished by indirect route through other non-zero matrix elements. At second order of perturbation theory, c (2) f (t) = 1 2 dt m dt e iω fmt +iω mi t V fm (t )V mi (t ) If harmonic potential perturbation is gradually switched on, V (t) =e εt Ve iωt, ε 0, with the initial time, c (2) f (t) = 1 2 f V m m V i m dt dt e i(ω fm ω iε)t e i(ω mi ω iε)t
51 Harmonic perturbations: second-order transitions From time integral, c (2) n = 1 2 ei(ω fi 2ω)t e 2εt ω fi 2ω 2iε m f V m m V i ω mi ω iε Leads to transition rate (ε 0): d dt c(2) n (t) 2 = 2π 4 m f V m m V i ω mi ω iε 2 δ(ω fi 2ω) This translates to a transition in which system gains energy 2 ω from harmonic perturbation, i.e. two photons are absorbed Physically, first photon takes effects virtual transition to short-lived intermediate state with energy ω m. If an atom in an arbitrary state is exposed to monochromatic light, other second order processes in which two photons are emitted, or one is absorbed and one emitted are also possible.
52 Harmonic perturbations: second-order transitions From time integral, c (2) n = 1 2 ei(ω fi 2ω)t e 2εt ω fi 2ω 2iε m f V m m V i ω mi ω iε Leads to transition rate (ε 0): d dt c(2) n (t) 2 = 2π 4 m f V m m V i ω mi ω iε 2 δ(ω fi 2ω) This translates to a transition in which system gains energy 2 ω from harmonic perturbation, i.e. two photons are absorbed Physically, first photon takes effects virtual transition to short-lived intermediate state with energy ω m. If an atom in an arbitrary state is exposed to monochromatic light, other second order processes in which two photons are emitted, or one is absorbed and one emitted are also possible.
53 Harmonic perturbations: second-order transitions From time integral, c (2) n = 1 2 ei(ω fi 2ω)t e 2εt ω fi 2ω 2iε m f V m m V i ω mi ω iε Leads to transition rate (ε 0): d dt c(2) n (t) 2 = 2π 4 m f V m m V i ω mi ω iε 2 δ(ω fi 2ω) This translates to a transition in which system gains energy 2 ω from harmonic perturbation, i.e. two photons are absorbed Physically, first photon takes effects virtual transition to short-lived intermediate state with energy ω m. If an atom in an arbitrary state is exposed to monochromatic light, other second order processes in which two photons are emitted, or one is absorbed and one emitted are also possible.
54 Time-dependent perturbation theory: summary For a general time-dependent Hamiltonian, Ĥ = Ĥ 0 + V (t), in which all time-dependence containing in potential V (t), the wavefunction can be expressed in the interaction representation, ψ(t) I = e iĥ 0 t/ ψ(t) S, ψ(0) I = ψ(0) S In this representation, the time-dependent Schrödinger equation takes the form, i t ψ(t) I = V I (t) ψ(t) I, V I (t) =e iĥ 0 t/ V (t)e iĥ 0 t/ If we expand ψ(t) I = n c n(t) n in basis of time-independent Hamiltonian, Ĥ0 n = E n n, the Schrödinger equation translates to i ċ m (t) = n V mn (t)e iω mnt c n (t) where V mn (t) = m V (t) m and ω mn = 1 (E m E n )= ω nm.
55 Time-dependent perturbation theory: summary For a general time-dependent Hamiltonian, Ĥ = Ĥ 0 + V (t), in which all time-dependence containing in potential V (t), the wavefunction can be expressed in the interaction representation, ψ(t) I = e iĥ 0 t/ ψ(t) S, ψ(0) I = ψ(0) S In this representation, the time-dependent Schrödinger equation takes the form, i t ψ(t) I = V I (t) ψ(t) I, V I (t) =e iĥ 0 t/ V (t)e iĥ 0 t/ If we expand ψ(t) I = n c n(t) n in basis of time-independent Hamiltonian, Ĥ0 n = E n n, the Schrödinger equation translates to i ċ m (t) = n V mn (t)e iω mnt c n (t) where V mn (t) = m V (t) m and ω mn = 1 (E m E n )= ω nm.
56 Time-dependent perturbation theory: summary For a general time-dependent Hamiltonian, Ĥ = Ĥ 0 + V (t), in which all time-dependence containing in potential V (t), the wavefunction can be expressed in the interaction representation, ψ(t) I = e iĥ 0 t/ ψ(t) S, ψ(0) I = ψ(0) S In this representation, the time-dependent Schrödinger equation takes the form, i t ψ(t) I = V I (t) ψ(t) I, V I (t) =e iĥ 0 t/ V (t)e iĥ 0 t/ If we expand ψ(t) I = n c n(t) n in basis of time-independent Hamiltonian, Ĥ0 n = E n n, the Schrödinger equation translates to i ċ m (t) = n V mn (t)e iω mnt c n (t) where V mn (t) = m V (t) m and ω mn = 1 (E m E n )= ω nm.
57 Time-dependent perturbation theory: summary For a general time-dependent potential, V (t), the wavefunction can be expanded as a power series in the interaction, ψ(t) I = n c n (t) n, c n (t) =c (0) n + c (1) n (t)+c (2) n (t)+ The coefficents can be expressed as matrix elements of the time-evolution operator, c n (t) = n Û I (t, ) i, where ( Û I (t, )= i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) n=0 From first two terms in the series, we have c n (1) (t) = i c n (2) (t) = 1 2 m dt e iω ni t dt V ni (t ) dt e iω nmt +iω mi t V nm (t )V mi (t )
58 Time-dependent perturbation theory: summary For a general time-dependent potential, V (t), the wavefunction can be expanded as a power series in the interaction, ψ(t) I = n c n (t) n, c n (t) =c (0) n + c (1) n (t)+c (2) n (t)+ The coefficents can be expressed as matrix elements of the time-evolution operator, c n (t) = n Û I (t, ) i, where ( Û I (t, )= i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) n=0 From first two terms in the series, we have c n (1) (t) = i c n (2) (t) = 1 2 m dt e iω ni t dt V ni (t ) dt e iω nmt +iω mi t V nm (t )V mi (t )
59 Time-dependent perturbation theory: summary For a general time-dependent potential, V (t), the wavefunction can be expanded as a power series in the interaction, ψ(t) I = n c n (t) n, c n (t) =c (0) n + c (1) n (t)+c (2) n (t)+ The coefficents can be expressed as matrix elements of the time-evolution operator, c n (t) = n Û I (t, ) i, where ( Û I (t, )= i ) n n 1 dt 1 dt n V I (t 1 )V I (t 2 ) V I (t n ) n=0 From first two terms in the series, we have c n (1) (t) = i c n (2) (t) = 1 2 m dt e iω ni t dt V ni (t ) dt e iω nmt +iω mi t V nm (t )V mi (t )
60 Time-dependent perturbation theory: summary c n (1) (t) = i dt e iω ni t V ni (t ) For a harmonic perturbation, V (t) =Ve iωt, turned on at t = 0, the leading term in series translates to transition rate, R i f (t) = lim t P i f (t) t = 2π 2 f V i 2 δ(ω fi ω) Fermi s Golden rule. If this term vanishes by symmetry, transitions can be effected by second and higher order processes through intermediate states. In the next lecture, we will apply these ideas to the consideration of radiative transitions in atoms.
61 Time-dependent perturbation theory: summary c n (1) (t) = i dt e iω ni t V ni (t ) For a harmonic perturbation, V (t) =Ve iωt, turned on at t = 0, the leading term in series translates to transition rate, R i f (t) = lim t P i f (t) t = 2π 2 f V i 2 δ(ω fi ω) Fermi s Golden rule. If this term vanishes by symmetry, transitions can be effected by second and higher order processes through intermediate states. In the next lecture, we will apply these ideas to the consideration of radiative transitions in atoms.
1 Variational calculation of a 1D bound state
 TEORETISK FYSIK, KTH TENTAMEN I KVANTMEKANIK FÖRDJUPNINGSKURS EXAMINATION IN ADVANCED QUANTUM MECHAN- ICS Kvantmekanik fördjupningskurs SI38 för F4 Thursday December, 7, 8. 13. Write on each page: Name,
TEORETISK FYSIK, KTH TENTAMEN I KVANTMEKANIK FÖRDJUPNINGSKURS EXAMINATION IN ADVANCED QUANTUM MECHAN- ICS Kvantmekanik fördjupningskurs SI38 för F4 Thursday December, 7, 8. 13. Write on each page: Name,
Time dependence in quantum mechanics Notes on Quantum Mechanics
 Time dependence in quantum mechanics Notes on Quantum Mechanics http://quantum.bu.edu/notes/quantummechanics/timedependence.pdf Last updated Thursday, November 20, 2003 13:22:37-05:00 Copyright 2003 Dan
Time dependence in quantum mechanics Notes on Quantum Mechanics http://quantum.bu.edu/notes/quantummechanics/timedependence.pdf Last updated Thursday, November 20, 2003 13:22:37-05:00 Copyright 2003 Dan
Time Ordered Perturbation Theory
 Michael Dine Department of Physics University of California, Santa Cruz October 2013 Quantization of the Free Electromagnetic Field We have so far quantized the free scalar field and the free Dirac field.
Michael Dine Department of Physics University of California, Santa Cruz October 2013 Quantization of the Free Electromagnetic Field We have so far quantized the free scalar field and the free Dirac field.
Multi-dimensional spectroscopy
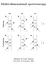 Multi-dimensional spectroscopy GB SE EA Rephasing 1 1 1 2 1 1 1 1 1 t2 1 1 Nonrephasing 1 1 1 2 1 1 1 1 1 1 1 t 2 Thomas la Cour Jansen University of Groningen, 29 Front cover: The double sided Feynman
Multi-dimensional spectroscopy GB SE EA Rephasing 1 1 1 2 1 1 1 1 1 t2 1 1 Nonrephasing 1 1 1 2 1 1 1 1 1 1 1 t 2 Thomas la Cour Jansen University of Groningen, 29 Front cover: The double sided Feynman
Concept 2. A. Description of light-matter interaction B. Quantitatities in spectroscopy
 Concept 2 A. Description of light-matter interaction B. Quantitatities in spectroscopy Dipole approximation Rabi oscillations Einstein kinetics in two-level system B. Absorption: quantitative description
Concept 2 A. Description of light-matter interaction B. Quantitatities in spectroscopy Dipole approximation Rabi oscillations Einstein kinetics in two-level system B. Absorption: quantitative description
Laser-Control of Molecular Dynamics. Dissertation
 Laser-Control of Molecular Dynamics Dissertation zur Erlangung des naturwissenschaftlichen Doktorgrades der Bayerischen Julius-Maximilians-Universität Würzburg vorgelegt von Stefanie Gräfe aus Düsseldorf
Laser-Control of Molecular Dynamics Dissertation zur Erlangung des naturwissenschaftlichen Doktorgrades der Bayerischen Julius-Maximilians-Universität Würzburg vorgelegt von Stefanie Gräfe aus Düsseldorf
Introduction to Schrödinger Equation: Harmonic Potential
 Introduction to Schrödinger Equation: Harmonic Potential Chia-Chun Chou May 2, 2006 Introduction to Schrödinger Equation: Harmonic Potential Time-Dependent Schrödinger Equation For a nonrelativistic particle
Introduction to Schrödinger Equation: Harmonic Potential Chia-Chun Chou May 2, 2006 Introduction to Schrödinger Equation: Harmonic Potential Time-Dependent Schrödinger Equation For a nonrelativistic particle
PHY4604 Introduction to Quantum Mechanics Fall 2004 Practice Test 3 November 22, 2004
 PHY464 Introduction to Quantum Mechanics Fall 4 Practice Test 3 November, 4 These problems are similar but not identical to the actual test. One or two parts will actually show up.. Short answer. (a) Recall
PHY464 Introduction to Quantum Mechanics Fall 4 Practice Test 3 November, 4 These problems are similar but not identical to the actual test. One or two parts will actually show up.. Short answer. (a) Recall
Chemistry 102 Summary June 24 th. Properties of Light
 Chemistry 102 Summary June 24 th Properties of Light - Energy travels through space in the form of electromagnetic radiation (EMR). - Examples of types of EMR: radio waves, x-rays, microwaves, visible
Chemistry 102 Summary June 24 th Properties of Light - Energy travels through space in the form of electromagnetic radiation (EMR). - Examples of types of EMR: radio waves, x-rays, microwaves, visible
Time-bin entanglement of quasi-particles in semiconductor devices. Luca Chirolli, Vittorio Giovannetti, Rosario Fazio, Valerio Scarani
 Time-bin entanglement of quasi-particles in semiconductor devices Luca Chirolli, Vittorio Giovannetti, Rosario Fazio, Valerio Scarani The concept of energy-time entanglement τ 1 τ 2 total energy well defined
Time-bin entanglement of quasi-particles in semiconductor devices Luca Chirolli, Vittorio Giovannetti, Rosario Fazio, Valerio Scarani The concept of energy-time entanglement τ 1 τ 2 total energy well defined
Assessment Plan for Learning Outcomes for BA/BS in Physics
 Department of Physics and Astronomy Goals and Learning Outcomes 1. Students know basic physics principles [BS, BA, MS] 1.1 Students can demonstrate an understanding of Newton s laws 1.2 Students can demonstrate
Department of Physics and Astronomy Goals and Learning Outcomes 1. Students know basic physics principles [BS, BA, MS] 1.1 Students can demonstrate an understanding of Newton s laws 1.2 Students can demonstrate
Quantum Mechanics: Postulates
 Quantum Mechanics: Postulates 5th April 2010 I. Physical meaning of the Wavefunction Postulate 1: The wavefunction attempts to describe a quantum mechanical entity (photon, electron, x-ray, etc.) through
Quantum Mechanics: Postulates 5th April 2010 I. Physical meaning of the Wavefunction Postulate 1: The wavefunction attempts to describe a quantum mechanical entity (photon, electron, x-ray, etc.) through
Rate of convergence towards Hartree dynamics
 Rate of convergence towards Hartree dynamics Benjamin Schlein, LMU München and University of Cambridge Universitá di Milano Bicocca, October 22, 2007 Joint work with I. Rodnianski 1. Introduction boson
Rate of convergence towards Hartree dynamics Benjamin Schlein, LMU München and University of Cambridge Universitá di Milano Bicocca, October 22, 2007 Joint work with I. Rodnianski 1. Introduction boson
NMR for Physical and Biological Scientists Thomas C. Pochapsky and Susan Sondej Pochapsky Table of Contents
 Preface Symbols and fundamental constants 1. What is spectroscopy? A semiclassical description of spectroscopy Damped harmonics Quantum oscillators The spectroscopic experiment Ensembles and coherence
Preface Symbols and fundamental constants 1. What is spectroscopy? A semiclassical description of spectroscopy Damped harmonics Quantum oscillators The spectroscopic experiment Ensembles and coherence
ENERGY TRANSFER IN THE WEAK AND STRONG COUPLING REGIME
 ERC Starting Grant 2011 Dipar)mento di Scienze Chimiche Università degli Studi di Padova via Marzolo 1, 35131 Padova Italy ENERGY TRANSFER IN THE WEAK AND STRONG COUPLING REGIME [1] Vekshin, N. L. Energy
ERC Starting Grant 2011 Dipar)mento di Scienze Chimiche Università degli Studi di Padova via Marzolo 1, 35131 Padova Italy ENERGY TRANSFER IN THE WEAK AND STRONG COUPLING REGIME [1] Vekshin, N. L. Energy
Operator methods in quantum mechanics
 Chapter 3 Operator methods in quantum mechanics While the wave mechanical formulation has proved successful in describing the quantum mechanics of bound and unbound particles, some properties can not be
Chapter 3 Operator methods in quantum mechanics While the wave mechanical formulation has proved successful in describing the quantum mechanics of bound and unbound particles, some properties can not be
- thus, the total number of atoms per second that absorb a photon is
 Stimulated Emission of Radiation - stimulated emission is referring to the emission of radiation (a photon) from one quantum system at its transition frequency induced by the presence of other photons
Stimulated Emission of Radiation - stimulated emission is referring to the emission of radiation (a photon) from one quantum system at its transition frequency induced by the presence of other photons
Eðlisfræði 2, vor 2007
 [ Assignment View ] [ Print ] Eðlisfræði 2, vor 2007 30. Inductance Assignment is due at 2:00am on Wednesday, March 14, 2007 Credit for problems submitted late will decrease to 0% after the deadline has
[ Assignment View ] [ Print ] Eðlisfræði 2, vor 2007 30. Inductance Assignment is due at 2:00am on Wednesday, March 14, 2007 Credit for problems submitted late will decrease to 0% after the deadline has
Basic Concepts in Nuclear Physics
 Basic Concepts in Nuclear Physics Paolo Finelli Corso di Teoria delle Forze Nucleari 2011 Literature/Bibliography Some useful texts are available at the Library: Wong, Nuclear Physics Krane, Introductory
Basic Concepts in Nuclear Physics Paolo Finelli Corso di Teoria delle Forze Nucleari 2011 Literature/Bibliography Some useful texts are available at the Library: Wong, Nuclear Physics Krane, Introductory
Perpetual motion and driven dynamics of a mobile impurity in a quantum fluid
 and driven dynamics of a mobile impurity in a quantum fluid Oleg Lychkovskiy Russian Quantum Center Seminaire du LPTMS, 01.12.2015 Seminaire du LPTMS, 01.12.2015 1 / Plan of the talk 1 Perpetual motion
and driven dynamics of a mobile impurity in a quantum fluid Oleg Lychkovskiy Russian Quantum Center Seminaire du LPTMS, 01.12.2015 Seminaire du LPTMS, 01.12.2015 1 / Plan of the talk 1 Perpetual motion
PQM Supplementary Notes: Spin, topology, SU(2) SO(3) etc
 PQM Supplementary Notes: Spin, topology, SU(2) SO(3) etc (away from the syllabus, but interesting material, based on notes by Dr J.M. Evans) 1 Rotations and Non-contractible Loops Rotations in R 3 can
PQM Supplementary Notes: Spin, topology, SU(2) SO(3) etc (away from the syllabus, but interesting material, based on notes by Dr J.M. Evans) 1 Rotations and Non-contractible Loops Rotations in R 3 can
The Role of Electric Polarization in Nonlinear optics
 The Role of Electric Polarization in Nonlinear optics Sumith Doluweera Department of Physics University of Cincinnati Cincinnati, Ohio 45221 Abstract Nonlinear optics became a very active field of research
The Role of Electric Polarization in Nonlinear optics Sumith Doluweera Department of Physics University of Cincinnati Cincinnati, Ohio 45221 Abstract Nonlinear optics became a very active field of research
Till now, almost all attention has been focussed on discussing the state of a quantum system.
 Chapter 13 Observables and Measurements in Quantum Mechanics Till now, almost all attention has been focussed on discussing the state of a quantum system. As we have seen, this is most succinctly done
Chapter 13 Observables and Measurements in Quantum Mechanics Till now, almost all attention has been focussed on discussing the state of a quantum system. As we have seen, this is most succinctly done
The quantum mechanics of particles in a periodic potential: Bloch s theorem
 Handout 2 The quantum mechanics of particles in a periodic potential: Bloch s theorem 2.1 Introduction and health warning We are going to set up the formalism for dealing with a periodic potential; this
Handout 2 The quantum mechanics of particles in a periodic potential: Bloch s theorem 2.1 Introduction and health warning We are going to set up the formalism for dealing with a periodic potential; this
Basic Principles of Magnetic Resonance
 Basic Principles of Magnetic Resonance Contents: Jorge Jovicich jovicich@mit.edu I) Historical Background II) An MR experiment - Overview - Can we scan the subject? - The subject goes into the magnet -
Basic Principles of Magnetic Resonance Contents: Jorge Jovicich jovicich@mit.edu I) Historical Background II) An MR experiment - Overview - Can we scan the subject? - The subject goes into the magnet -
NMR and IR spectra & vibrational analysis
 Lab 5: NMR and IR spectra & vibrational analysis A brief theoretical background 1 Some of the available chemical quantum methods for calculating NMR chemical shifts are based on the Hartree-Fock self-consistent
Lab 5: NMR and IR spectra & vibrational analysis A brief theoretical background 1 Some of the available chemical quantum methods for calculating NMR chemical shifts are based on the Hartree-Fock self-consistent
8.04: Quantum Mechanics Professor Allan Adams Massachusetts Institute of Technology. Problem Set 5
 8.04: Quantum Mechanics Professor Allan Adams Massachusetts Institute of Technology Tuesday March 5 Problem Set 5 Due Tuesday March 12 at 11.00AM Assigned Reading: E&R 6 9, App-I Li. 7 1 4 Ga. 4 7, 6 1,2
8.04: Quantum Mechanics Professor Allan Adams Massachusetts Institute of Technology Tuesday March 5 Problem Set 5 Due Tuesday March 12 at 11.00AM Assigned Reading: E&R 6 9, App-I Li. 7 1 4 Ga. 4 7, 6 1,2
Chapter 9 Unitary Groups and SU(N)
 Chapter 9 Unitary Groups and SU(N) The irreducible representations of SO(3) are appropriate for describing the degeneracies of states of quantum mechanical systems which have rotational symmetry in three
Chapter 9 Unitary Groups and SU(N) The irreducible representations of SO(3) are appropriate for describing the degeneracies of states of quantum mechanical systems which have rotational symmetry in three
Spectroscopy and Regions of the Spectrum
 Basics 9 Spectroscopy and Regions of the Spectrum Different regions of the spectrum probe different types of energy levels of an atomic or molecular system. It is not uncommon to refer to a spectroscopic
Basics 9 Spectroscopy and Regions of the Spectrum Different regions of the spectrum probe different types of energy levels of an atomic or molecular system. It is not uncommon to refer to a spectroscopic
Blackbody radiation derivation of Planck s radiation low
 Blackbody radiation derivation of Planck s radiation low 1 Classical theories of Lorentz and Debye: Lorentz (oscillator model): Electrons and ions of matter were treated as a simple harmonic oscillators
Blackbody radiation derivation of Planck s radiation low 1 Classical theories of Lorentz and Debye: Lorentz (oscillator model): Electrons and ions of matter were treated as a simple harmonic oscillators
Group Theory and Chemistry
 Group Theory and Chemistry Outline: Raman and infra-red spectroscopy Symmetry operations Point Groups and Schoenflies symbols Function space and matrix representation Reducible and irreducible representation
Group Theory and Chemistry Outline: Raman and infra-red spectroscopy Symmetry operations Point Groups and Schoenflies symbols Function space and matrix representation Reducible and irreducible representation
Contents. Goldstone Bosons in 3He-A Soft Modes Dynamics and Lie Algebra of Group G:
 ... Vlll Contents 3. Textures and Supercurrents in Superfluid Phases of 3He 3.1. Textures, Gradient Energy and Rigidity 3.2. Why Superfuids are Superfluid 3.3. Superfluidity and Response to a Transverse
... Vlll Contents 3. Textures and Supercurrents in Superfluid Phases of 3He 3.1. Textures, Gradient Energy and Rigidity 3.2. Why Superfuids are Superfluid 3.3. Superfluidity and Response to a Transverse
Lecture 5 Motion of a charged particle in a magnetic field
 Lecture 5 Motion of a charged particle in a magnetic field Charged particle in a magnetic field: Outline 1 Canonical quantization: lessons from classical dynamics 2 Quantum mechanics of a particle in a
Lecture 5 Motion of a charged particle in a magnetic field Charged particle in a magnetic field: Outline 1 Canonical quantization: lessons from classical dynamics 2 Quantum mechanics of a particle in a
Implementation of Digital Signal Processing: Some Background on GFSK Modulation
 Implementation of Digital Signal Processing: Some Background on GFSK Modulation Sabih H. Gerez University of Twente, Department of Electrical Engineering s.h.gerez@utwente.nl Version 4 (February 7, 2013)
Implementation of Digital Signal Processing: Some Background on GFSK Modulation Sabih H. Gerez University of Twente, Department of Electrical Engineering s.h.gerez@utwente.nl Version 4 (February 7, 2013)
MASTER OF SCIENCE IN PHYSICS MASTER OF SCIENCES IN PHYSICS (MS PHYS) (LIST OF COURSES BY SEMESTER, THESIS OPTION)
 MASTER OF SCIENCE IN PHYSICS Admission Requirements 1. Possession of a BS degree from a reputable institution or, for non-physics majors, a GPA of 2.5 or better in at least 15 units in the following advanced
MASTER OF SCIENCE IN PHYSICS Admission Requirements 1. Possession of a BS degree from a reputable institution or, for non-physics majors, a GPA of 2.5 or better in at least 15 units in the following advanced
EEE1001/PHY1002. Magnetic Circuits. The circuit is of length l=2πr. B andφ circulate
 1 Magnetic Circuits Just as we view electric circuits as related to the flow of charge, we can also view magnetic flux flowing around a magnetic circuit. The sum of fluxes entering a point must sum to
1 Magnetic Circuits Just as we view electric circuits as related to the flow of charge, we can also view magnetic flux flowing around a magnetic circuit. The sum of fluxes entering a point must sum to
Teoretisk Fysik KTH. Advanced QM (SI2380), test questions 1
 Teoretisk Fysik KTH Advanced QM (SI238), test questions NOTE THAT I TYPED THIS IN A HURRY AND TYPOS ARE POSSIBLE: PLEASE LET ME KNOW BY EMAIL IF YOU FIND ANY (I will try to correct typos asap - if you
Teoretisk Fysik KTH Advanced QM (SI238), test questions NOTE THAT I TYPED THIS IN A HURRY AND TYPOS ARE POSSIBLE: PLEASE LET ME KNOW BY EMAIL IF YOU FIND ANY (I will try to correct typos asap - if you
Three Pictures of Quantum Mechanics. Thomas R. Shafer April 17, 2009
 Three Pictures of Quantum Mechanics Thomas R. Shafer April 17, 2009 Outline of the Talk Brief review of (or introduction to) quantum mechanics. 3 different viewpoints on calculation. Schrödinger, Heisenberg,
Three Pictures of Quantum Mechanics Thomas R. Shafer April 17, 2009 Outline of the Talk Brief review of (or introduction to) quantum mechanics. 3 different viewpoints on calculation. Schrödinger, Heisenberg,
Lecture 3: Optical Properties of Bulk and Nano. 5 nm
 Lecture 3: Optical Properties of Bulk and Nano 5 nm The Previous Lecture Origin frequency dependence of χ in real materials Lorentz model (harmonic oscillator model) 0 e - n( ) n' n '' n ' = 1 + Nucleus
Lecture 3: Optical Properties of Bulk and Nano 5 nm The Previous Lecture Origin frequency dependence of χ in real materials Lorentz model (harmonic oscillator model) 0 e - n( ) n' n '' n ' = 1 + Nucleus
How To Find The Optimal Control Function On A Unitary Operation
 Quantum Computation as Geometry arxiv:quant-ph/0603161v2 21 Mar 2006 Michael A. Nielsen, Mark R. Dowling, Mile Gu, and Andrew C. Doherty School of Physical Sciences, The University of Queensland, Queensland
Quantum Computation as Geometry arxiv:quant-ph/0603161v2 21 Mar 2006 Michael A. Nielsen, Mark R. Dowling, Mile Gu, and Andrew C. Doherty School of Physical Sciences, The University of Queensland, Queensland
Quantum Computing for Beginners: Building Qubits
 Quantum Computing for Beginners: Building Qubits Suzanne Gildert Condensed Matter Physics Research (Quantum Devices Group) University of Birmingham 28/03/2007 Overview of this presentation What is a Qubit?
Quantum Computing for Beginners: Building Qubits Suzanne Gildert Condensed Matter Physics Research (Quantum Devices Group) University of Birmingham 28/03/2007 Overview of this presentation What is a Qubit?
PHY114 S11 Term Exam 3
 PHY4 S Term Exam S. G. Rajeev Mar 2 20 2:0 pm to :45 pm PLEASE write your workshop number and your workshop leader s name at the top of your book, so that you can collect your graded exams at the workshop.
PHY4 S Term Exam S. G. Rajeev Mar 2 20 2:0 pm to :45 pm PLEASE write your workshop number and your workshop leader s name at the top of your book, so that you can collect your graded exams at the workshop.
Light as a Wave. The Nature of Light. EM Radiation Spectrum. EM Radiation Spectrum. Electromagnetic Radiation
 The Nature of Light Light and other forms of radiation carry information to us from distance astronomical objects Visible light is a subset of a huge spectrum of electromagnetic radiation Maxwell pioneered
The Nature of Light Light and other forms of radiation carry information to us from distance astronomical objects Visible light is a subset of a huge spectrum of electromagnetic radiation Maxwell pioneered
Raman Scattering Theory David W. Hahn Department of Mechanical and Aerospace Engineering University of Florida (dwhahn@ufl.edu)
 Introduction Raman Scattering Theory David W. Hahn Department of Mechanical and Aerospace Engineering University of Florida (dwhahn@ufl.edu) The scattering of light may be thought of as the redirection
Introduction Raman Scattering Theory David W. Hahn Department of Mechanical and Aerospace Engineering University of Florida (dwhahn@ufl.edu) The scattering of light may be thought of as the redirection
Coupling Magnetic Signals to a SQUID Amplifier
 SQUID Application Note 105-0 Coupling Magnetic Signals to a SQUID Amplifier Matching the effective inductances of the Pickup Coil and the Input Coil to detect and couple magnetic flux maximizes the sensitivity
SQUID Application Note 105-0 Coupling Magnetic Signals to a SQUID Amplifier Matching the effective inductances of the Pickup Coil and the Input Coil to detect and couple magnetic flux maximizes the sensitivity
Name Date Class ELECTRONS IN ATOMS. Standard Curriculum Core content Extension topics
 13 ELECTRONS IN ATOMS Conceptual Curriculum Concrete concepts More abstract concepts or math/problem-solving Standard Curriculum Core content Extension topics Honors Curriculum Core honors content Options
13 ELECTRONS IN ATOMS Conceptual Curriculum Concrete concepts More abstract concepts or math/problem-solving Standard Curriculum Core content Extension topics Honors Curriculum Core honors content Options
Quantum Mechanics and Representation Theory
 Quantum Mechanics and Representation Theory Peter Woit Columbia University Texas Tech, November 21 2013 Peter Woit (Columbia University) Quantum Mechanics and Representation Theory November 2013 1 / 30
Quantum Mechanics and Representation Theory Peter Woit Columbia University Texas Tech, November 21 2013 Peter Woit (Columbia University) Quantum Mechanics and Representation Theory November 2013 1 / 30
Influence of strong noise on the Adiabatic Quantum Computer
 Fakultät für Physik Institut für Theoretische Festkörperphysik Influence of strong noise on the Adiabatic Quantum Computer Der Einfluss von starkem Rauschen auf den Adiabatischen Quanten Computer Diploma
Fakultät für Physik Institut für Theoretische Festkörperphysik Influence of strong noise on the Adiabatic Quantum Computer Der Einfluss von starkem Rauschen auf den Adiabatischen Quanten Computer Diploma
Quantum computation with phase drift errors.
 Quantum computation with phase drift errors. César Miquel 1,,3,JuanPabloPaz 1,,3, and Wojciech Hubert Zurek 1,3 1 Institute for Theoretical Physics, University of California, Santa Barbara, CA 9316 3,
Quantum computation with phase drift errors. César Miquel 1,,3,JuanPabloPaz 1,,3, and Wojciech Hubert Zurek 1,3 1 Institute for Theoretical Physics, University of California, Santa Barbara, CA 9316 3,
Pulsed Fourier Transform NMR The rotating frame of reference. The NMR Experiment. The Rotating Frame of Reference.
 Pulsed Fourier Transform NR The rotating frame of reference The NR Eperiment. The Rotating Frame of Reference. When we perform a NR eperiment we disturb the equilibrium state of the sstem and then monitor
Pulsed Fourier Transform NR The rotating frame of reference The NR Eperiment. The Rotating Frame of Reference. When we perform a NR eperiment we disturb the equilibrium state of the sstem and then monitor
arxiv:quant-ph/0002033v2 12 Jun 2000
 Multi-Valued Logic Gates for Quantum Computation Ashok Muthukrishnan and C. R. Stroud, Jr. The Institute of Optics, University of Rochester, Rochester, New York 1467 (February 1, 008) arxiv:quant-ph/000033v
Multi-Valued Logic Gates for Quantum Computation Ashok Muthukrishnan and C. R. Stroud, Jr. The Institute of Optics, University of Rochester, Rochester, New York 1467 (February 1, 008) arxiv:quant-ph/000033v
Excitation transfer and energy exchange processes for modeling the Fleischmann-Pons excess heat effect
 Hagelstein, P.L. and I. Chaudhary. Excitation transfer and energy exchange processes for modeling the Fleischmann-Pons excess heat effect. in ICCF-14 International Conference on Condensed Matter Nuclear
Hagelstein, P.L. and I. Chaudhary. Excitation transfer and energy exchange processes for modeling the Fleischmann-Pons excess heat effect. in ICCF-14 International Conference on Condensed Matter Nuclear
19.6. Finding a Particular Integral. Introduction. Prerequisites. Learning Outcomes. Learning Style
 Finding a Particular Integral 19.6 Introduction We stated in Block 19.5 that the general solution of an inhomogeneous equation is the sum of the complementary function and a particular integral. We have
Finding a Particular Integral 19.6 Introduction We stated in Block 19.5 that the general solution of an inhomogeneous equation is the sum of the complementary function and a particular integral. We have
NMR SPECTROSCOPY. Basic Principles, Concepts, and Applications in Chemistry. Harald Günther University of Siegen, Siegen, Germany.
 NMR SPECTROSCOPY Basic Principles, Concepts, and Applications in Chemistry Harald Günther University of Siegen, Siegen, Germany Second Edition Translated by Harald Günther JOHN WILEY & SONS Chichester
NMR SPECTROSCOPY Basic Principles, Concepts, and Applications in Chemistry Harald Günther University of Siegen, Siegen, Germany Second Edition Translated by Harald Günther JOHN WILEY & SONS Chichester
Advanced Quantum Physics
 Advanced Quantum Physics Aim of the course Building upon the foundations of wave mechanics, this course will introduce and develop the broad field of quantum physics including: Quantum mechanics of point
Advanced Quantum Physics Aim of the course Building upon the foundations of wave mechanics, this course will introduce and develop the broad field of quantum physics including: Quantum mechanics of point
= N 2 = 3π2 n = k 3 F. The kinetic energy of the uniform system is given by: 4πk 2 dk h2 k 2 2m. (2π) 3 0
 Chapter 1 Thomas-Fermi Theory The Thomas-Fermi theory provides a functional form for the kinetic energy of a non-interacting electron gas in some known external potential V (r) (usually due to impurities)
Chapter 1 Thomas-Fermi Theory The Thomas-Fermi theory provides a functional form for the kinetic energy of a non-interacting electron gas in some known external potential V (r) (usually due to impurities)
Realization and characterization of a phase locked laser system for coherent spectroscopy of fiber-coupled cesium atoms
 Johannes Gutenberg-Universität Mainz - Institut für Physik Realization and characterization of a phase locked laser system for coherent spectroscopy of fiber-coupled cesium atoms Diplomarbeit von Melanie
Johannes Gutenberg-Universität Mainz - Institut für Physik Realization and characterization of a phase locked laser system for coherent spectroscopy of fiber-coupled cesium atoms Diplomarbeit von Melanie
Sample Exercise 6.1 Concepts of Wavelength and Frequency
 Sample Exercise 6.1 Concepts of Wavelength and Frequency Two electromagnetic waves are represented in the margin. (a) Which wave has the higher frequency? (b) If one wave represents visible light and the
Sample Exercise 6.1 Concepts of Wavelength and Frequency Two electromagnetic waves are represented in the margin. (a) Which wave has the higher frequency? (b) If one wave represents visible light and the
Chapter 29 Alternating-Current Circuits
 hapter 9 Alternating-urrent ircuits onceptual Problems A coil in an ac generator rotates at 6 Hz. How much time elapses between successive emf values of the coil? Determine the oncept Successive s are
hapter 9 Alternating-urrent ircuits onceptual Problems A coil in an ac generator rotates at 6 Hz. How much time elapses between successive emf values of the coil? Determine the oncept Successive s are
Chapter 22 The Hamiltonian and Lagrangian densities. from my book: Understanding Relativistic Quantum Field Theory. Hans de Vries
 Chapter 22 The Hamiltonian and Lagrangian densities from my book: Understanding Relativistic Quantum Field Theory Hans de Vries January 2, 2009 2 Chapter Contents 22 The Hamiltonian and Lagrangian densities
Chapter 22 The Hamiltonian and Lagrangian densities from my book: Understanding Relativistic Quantum Field Theory Hans de Vries January 2, 2009 2 Chapter Contents 22 The Hamiltonian and Lagrangian densities
FLAP P11.2 The quantum harmonic oscillator
 F L E X I B L E L E A R N I N G A P P R O A C H T O P H Y S I C S Module P. Opening items. Module introduction. Fast track questions.3 Ready to study? The harmonic oscillator. Classical description of
F L E X I B L E L E A R N I N G A P P R O A C H T O P H Y S I C S Module P. Opening items. Module introduction. Fast track questions.3 Ready to study? The harmonic oscillator. Classical description of
Blackbody Radiation References INTRODUCTION
 Blackbody Radiation References 1) R.A. Serway, R.J. Beichner: Physics for Scientists and Engineers with Modern Physics, 5 th Edition, Vol. 2, Ch.40, Saunders College Publishing (A Division of Harcourt
Blackbody Radiation References 1) R.A. Serway, R.J. Beichner: Physics for Scientists and Engineers with Modern Physics, 5 th Edition, Vol. 2, Ch.40, Saunders College Publishing (A Division of Harcourt
CHAPTER 9 ATOMIC STRUCTURE AND THE PERIODIC LAW
 CHAPTER 9 ATOMIC STRUCTURE AND THE PERIODIC LAW Quantum mechanics can account for the periodic structure of the elements, by any measure a major conceptual accomplishment for any theory. Although accurate
CHAPTER 9 ATOMIC STRUCTURE AND THE PERIODIC LAW Quantum mechanics can account for the periodic structure of the elements, by any measure a major conceptual accomplishment for any theory. Although accurate
Understanding Poles and Zeros
 MASSACHUSETTS INSTITUTE OF TECHNOLOGY DEPARTMENT OF MECHANICAL ENGINEERING 2.14 Analysis and Design of Feedback Control Systems Understanding Poles and Zeros 1 System Poles and Zeros The transfer function
MASSACHUSETTS INSTITUTE OF TECHNOLOGY DEPARTMENT OF MECHANICAL ENGINEERING 2.14 Analysis and Design of Feedback Control Systems Understanding Poles and Zeros 1 System Poles and Zeros The transfer function
Chemical group theory for quantum simulation
 Title JDWhitfield@gmail.com 1/19 Chemical group theory for quantum simulation James Daniel Whitfield U. Ghent September 28, 2015 Title JDWhitfield@gmail.com 2/19 1. Computational chemistry 2. Symmetry
Title JDWhitfield@gmail.com 1/19 Chemical group theory for quantum simulation James Daniel Whitfield U. Ghent September 28, 2015 Title JDWhitfield@gmail.com 2/19 1. Computational chemistry 2. Symmetry
Review of Statistical Mechanics
 Review of Statistical Mechanics 3. Microcanonical, Canonical, Grand Canonical Ensembles In statistical mechanics, we deal with a situation in which even the quantum state of the system is unknown. The
Review of Statistical Mechanics 3. Microcanonical, Canonical, Grand Canonical Ensembles In statistical mechanics, we deal with a situation in which even the quantum state of the system is unknown. The
- particle with kinetic energy E strikes a barrier with height U 0 > E and width L. - classically the particle cannot overcome the barrier
 Tunnel Effect: - particle with kinetic energy E strikes a barrier with height U 0 > E and width L - classically the particle cannot overcome the barrier - quantum mechanically the particle can penetrated
Tunnel Effect: - particle with kinetic energy E strikes a barrier with height U 0 > E and width L - classically the particle cannot overcome the barrier - quantum mechanically the particle can penetrated
Let s first see how precession works in quantitative detail. The system is illustrated below: ...
 lecture 20 Topics: Precession of tops Nutation Vectors in the body frame The free symmetric top in the body frame Euler s equations The free symmetric top ala Euler s The tennis racket theorem As you know,
lecture 20 Topics: Precession of tops Nutation Vectors in the body frame The free symmetric top in the body frame Euler s equations The free symmetric top ala Euler s The tennis racket theorem As you know,
Lecture 3: Optical Properties of Bulk and Nano. 5 nm
 Lecture 3: Optical Properties of Bulk and Nano 5 nm First H/W#1 is due Sept. 10 Course Info The Previous Lecture Origin frequency dependence of χ in real materials Lorentz model (harmonic oscillator model)
Lecture 3: Optical Properties of Bulk and Nano 5 nm First H/W#1 is due Sept. 10 Course Info The Previous Lecture Origin frequency dependence of χ in real materials Lorentz model (harmonic oscillator model)
How do we obtain the solution, if we are given F (t)? First we note that suppose someone did give us one solution of this equation
 1 Green s functions The harmonic oscillator equation is This has the solution mẍ + kx = 0 (1) x = A sin(ωt) + B cos(ωt), ω = k m where A, B are arbitrary constants reflecting the fact that we have two
1 Green s functions The harmonic oscillator equation is This has the solution mẍ + kx = 0 (1) x = A sin(ωt) + B cos(ωt), ω = k m where A, B are arbitrary constants reflecting the fact that we have two
LINEAR ALGEBRA W W L CHEN
 LINEAR ALGEBRA W W L CHEN c W W L Chen, 1997, 2008 This chapter is available free to all individuals, on understanding that it is not to be used for financial gain, and may be downloaded and/or photocopied,
LINEAR ALGEBRA W W L CHEN c W W L Chen, 1997, 2008 This chapter is available free to all individuals, on understanding that it is not to be used for financial gain, and may be downloaded and/or photocopied,
WAVES AND ELECTROMAGNETIC RADIATION
 WAVES AND ELECTROMAGNETIC RADIATION All waves are characterized by their wavelength, frequency and speed. Wavelength (lambda, ): the distance between any 2 successive crests or troughs. Frequency (nu,):
WAVES AND ELECTROMAGNETIC RADIATION All waves are characterized by their wavelength, frequency and speed. Wavelength (lambda, ): the distance between any 2 successive crests or troughs. Frequency (nu,):
Infrared Spectroscopy: Theory
 u Chapter 15 Infrared Spectroscopy: Theory An important tool of the organic chemist is Infrared Spectroscopy, or IR. IR spectra are acquired on a special instrument, called an IR spectrometer. IR is used
u Chapter 15 Infrared Spectroscopy: Theory An important tool of the organic chemist is Infrared Spectroscopy, or IR. IR spectra are acquired on a special instrument, called an IR spectrometer. IR is used
The Quantum Harmonic Oscillator Stephen Webb
 The Quantum Harmonic Oscillator Stephen Webb The Importance of the Harmonic Oscillator The quantum harmonic oscillator holds a unique importance in quantum mechanics, as it is both one of the few problems
The Quantum Harmonic Oscillator Stephen Webb The Importance of the Harmonic Oscillator The quantum harmonic oscillator holds a unique importance in quantum mechanics, as it is both one of the few problems
Feynman diagrams. 1 Aim of the game 2
 Feynman diagrams Contents 1 Aim of the game 2 2 Rules 2 2.1 Vertices................................ 3 2.2 Anti-particles............................. 3 2.3 Distinct diagrams...........................
Feynman diagrams Contents 1 Aim of the game 2 2 Rules 2 2.1 Vertices................................ 3 2.2 Anti-particles............................. 3 2.3 Distinct diagrams...........................
KE A = PE MAX 1/2M v 2 = k q1 q2 /R
 CHAPTER 13 NUCLEAR STRUCTURE NUCLEAR FORCE The nucleus is help firmly together by the nuclear or strong force, We can estimate the nuclear force by observing that protons residing about 1fm = 10-15m apart
CHAPTER 13 NUCLEAR STRUCTURE NUCLEAR FORCE The nucleus is help firmly together by the nuclear or strong force, We can estimate the nuclear force by observing that protons residing about 1fm = 10-15m apart
Fractional Revival of Rydberg Wave Packets in Twice-Kicked One-Dimensional Atoms
 Vol. 0 0) ACTA PHYSICA POLONICA A No. 3 Fractional Revival of Rydberg Wave Packets in Twice-Kicked One-Dimensional Atoms S. Chatterjee Department of Physics, Bidhannagar College, EB-, Sector-, Salt Lake,
Vol. 0 0) ACTA PHYSICA POLONICA A No. 3 Fractional Revival of Rydberg Wave Packets in Twice-Kicked One-Dimensional Atoms S. Chatterjee Department of Physics, Bidhannagar College, EB-, Sector-, Salt Lake,
2, 8, 20, 28, 50, 82, 126.
 Chapter 5 Nuclear Shell Model 5.1 Magic Numbers The binding energies predicted by the Liquid Drop Model underestimate the actual binding energies of magic nuclei for which either the number of neutrons
Chapter 5 Nuclear Shell Model 5.1 Magic Numbers The binding energies predicted by the Liquid Drop Model underestimate the actual binding energies of magic nuclei for which either the number of neutrons
Manual for SOA Exam FM/CAS Exam 2.
 Manual for SOA Exam FM/CAS Exam 2. Chapter 2. Cashflows. c 29. Miguel A. Arcones. All rights reserved. Extract from: Arcones Manual for the SOA Exam FM/CAS Exam 2, Financial Mathematics. Fall 29 Edition,
Manual for SOA Exam FM/CAS Exam 2. Chapter 2. Cashflows. c 29. Miguel A. Arcones. All rights reserved. Extract from: Arcones Manual for the SOA Exam FM/CAS Exam 2, Financial Mathematics. Fall 29 Edition,
Syllabus for Chem 359: Atomic and Molecular Spectroscopy
 Syllabus for Chem 359: Atomic and Molecular Spectroscopy Instructors: Dr. Reinhard Schweitzer- Stenner and Ms. Siobhan E. Toal Of#ice: Disque 605/Disque 306 Tel: (215) 895-2268 Email: rschweitzer- stenner@drexel.edu
Syllabus for Chem 359: Atomic and Molecular Spectroscopy Instructors: Dr. Reinhard Schweitzer- Stenner and Ms. Siobhan E. Toal Of#ice: Disque 605/Disque 306 Tel: (215) 895-2268 Email: rschweitzer- stenner@drexel.edu
Introduction to SME and Scattering Theory. Don Colladay. New College of Florida Sarasota, FL, 34243, U.S.A.
 June 2012 Introduction to SME and Scattering Theory Don Colladay New College of Florida Sarasota, FL, 34243, U.S.A. This lecture was given at the IUCSS summer school during June of 2012. It contains a
June 2012 Introduction to SME and Scattering Theory Don Colladay New College of Florida Sarasota, FL, 34243, U.S.A. This lecture was given at the IUCSS summer school during June of 2012. It contains a
2.2 Magic with complex exponentials
 2.2. MAGIC WITH COMPLEX EXPONENTIALS 97 2.2 Magic with complex exponentials We don t really know what aspects of complex variables you learned about in high school, so the goal here is to start more or
2.2. MAGIC WITH COMPLEX EXPONENTIALS 97 2.2 Magic with complex exponentials We don t really know what aspects of complex variables you learned about in high school, so the goal here is to start more or
7. DYNAMIC LIGHT SCATTERING 7.1 First order temporal autocorrelation function.
 7. DYNAMIC LIGHT SCATTERING 7. First order temporal autocorrelation function. Dynamic light scattering (DLS) studies the properties of inhomogeneous and dynamic media. A generic situation is illustrated
7. DYNAMIC LIGHT SCATTERING 7. First order temporal autocorrelation function. Dynamic light scattering (DLS) studies the properties of inhomogeneous and dynamic media. A generic situation is illustrated
Groups and Representations in Quantum Mechanics
 Chapter 6 Groups and Representations in Quantum Mechanics The universe is an enormous direct product of representations of symmetry groups. Steven Weinberg 1 This chapter is devoted to applying the mathematical
Chapter 6 Groups and Representations in Quantum Mechanics The universe is an enormous direct product of representations of symmetry groups. Steven Weinberg 1 This chapter is devoted to applying the mathematical
19.7. Applications of Differential Equations. Introduction. Prerequisites. Learning Outcomes. Learning Style
 Applications of Differential Equations 19.7 Introduction Blocks 19.2 to 19.6 have introduced several techniques for solving commonly-occurring firstorder and second-order ordinary differential equations.
Applications of Differential Equations 19.7 Introduction Blocks 19.2 to 19.6 have introduced several techniques for solving commonly-occurring firstorder and second-order ordinary differential equations.
arxiv:cond-mat/9301024v1 20 Jan 1993 ABSTRACT
 Anyons as Dirac Strings, the A x = 0 Gauge LPTB 93-1 John McCabe Laboratoire de Physique Théorique, 1 Université Bordeaux I 19 rue du Solarium, 33175 Gradignan FRANCE arxiv:cond-mat/930104v1 0 Jan 1993
Anyons as Dirac Strings, the A x = 0 Gauge LPTB 93-1 John McCabe Laboratoire de Physique Théorique, 1 Université Bordeaux I 19 rue du Solarium, 33175 Gradignan FRANCE arxiv:cond-mat/930104v1 0 Jan 1993
Power measurement in balanced 3 phase circuits and power factor improvement. 1 Power in Single Phase Circuits. Experiment no 1
 Experiment no 1 Power measurement in balanced 3 phase circuits and power factor improvement 1 Power in Single Phase Circuits Let v = m cos(ωt) = cos(ωt) is the voltage applied to a R-L circuit and i =
Experiment no 1 Power measurement in balanced 3 phase circuits and power factor improvement 1 Power in Single Phase Circuits Let v = m cos(ωt) = cos(ωt) is the voltage applied to a R-L circuit and i =
Solving Systems of Linear Equations
 LECTURE 5 Solving Systems of Linear Equations Recall that we introduced the notion of matrices as a way of standardizing the expression of systems of linear equations In today s lecture I shall show how
LECTURE 5 Solving Systems of Linear Equations Recall that we introduced the notion of matrices as a way of standardizing the expression of systems of linear equations In today s lecture I shall show how
Multipartite entanglement and sudden death in Quantum Optics: continuous variables domain
 Multipartite entanglement and sudden death in Quantum Optics: continuous variables domain Inst. de Física Marcelo Martinelli Lab. de Manipulação Coerente de Átomos e Luz Laboratório de Manipulação Coerente
Multipartite entanglement and sudden death in Quantum Optics: continuous variables domain Inst. de Física Marcelo Martinelli Lab. de Manipulação Coerente de Átomos e Luz Laboratório de Manipulação Coerente
MATRIX ALGEBRA AND SYSTEMS OF EQUATIONS
 MATRIX ALGEBRA AND SYSTEMS OF EQUATIONS Systems of Equations and Matrices Representation of a linear system The general system of m equations in n unknowns can be written a x + a 2 x 2 + + a n x n b a
MATRIX ALGEBRA AND SYSTEMS OF EQUATIONS Systems of Equations and Matrices Representation of a linear system The general system of m equations in n unknowns can be written a x + a 2 x 2 + + a n x n b a
Nuclear Magnetic Resonance
 Nuclear Magnetic Resonance Author: James Dragan Lab Partner: Stefan Evans Physics Department, Stony Brook University, Stony Brook, NY 794. (Dated: December 5, 23) We study the principles behind Nuclear
Nuclear Magnetic Resonance Author: James Dragan Lab Partner: Stefan Evans Physics Department, Stony Brook University, Stony Brook, NY 794. (Dated: December 5, 23) We study the principles behind Nuclear
F en = mω 0 2 x. We should regard this as a model of the response of an atom, rather than a classical model of the atom itself.
 The Electron Oscillator/Lorentz Atom Consider a simple model of a classical atom, in which the electron is harmonically bound to the nucleus n x e F en = mω 0 2 x origin resonance frequency Note: We should
The Electron Oscillator/Lorentz Atom Consider a simple model of a classical atom, in which the electron is harmonically bound to the nucleus n x e F en = mω 0 2 x origin resonance frequency Note: We should
Chapter 7. Lyapunov Exponents. 7.1 Maps
 Chapter 7 Lyapunov Exponents Lyapunov exponents tell us the rate of divergence of nearby trajectories a key component of chaotic dynamics. For one dimensional maps the exponent is simply the average
Chapter 7 Lyapunov Exponents Lyapunov exponents tell us the rate of divergence of nearby trajectories a key component of chaotic dynamics. For one dimensional maps the exponent is simply the average
Angle Modulation, II. Lecture topics FM bandwidth and Carson s rule. Spectral analysis of FM. Narrowband FM Modulation. Wideband FM Modulation
 Angle Modulation, II EE 179, Lecture 12, Handout #19 Lecture topics FM bandwidth and Carson s rule Spectral analysis of FM Narrowband FM Modulation Wideband FM Modulation EE 179, April 25, 2014 Lecture
Angle Modulation, II EE 179, Lecture 12, Handout #19 Lecture topics FM bandwidth and Carson s rule Spectral analysis of FM Narrowband FM Modulation Wideband FM Modulation EE 179, April 25, 2014 Lecture
Approximation Algorithms
 Approximation Algorithms or: How I Learned to Stop Worrying and Deal with NP-Completeness Ong Jit Sheng, Jonathan (A0073924B) March, 2012 Overview Key Results (I) General techniques: Greedy algorithms
Approximation Algorithms or: How I Learned to Stop Worrying and Deal with NP-Completeness Ong Jit Sheng, Jonathan (A0073924B) March, 2012 Overview Key Results (I) General techniques: Greedy algorithms
Basics of Quantum Physics - Part 1
 Czech Technical University in Prague Faculty of Nuclear Sciences and Physical Engineering, Department of Mathematics Time-periodic Quantum Systems Ph.D. Thesis Ondřej Lev Supervisor: Prof. Ing. Pavel Šťovíček
Czech Technical University in Prague Faculty of Nuclear Sciences and Physical Engineering, Department of Mathematics Time-periodic Quantum Systems Ph.D. Thesis Ondřej Lev Supervisor: Prof. Ing. Pavel Šťovíček
An Introduction to Hartree-Fock Molecular Orbital Theory
 An Introduction to Hartree-Fock Molecular Orbital Theory C. David Sherrill School of Chemistry and Biochemistry Georgia Institute of Technology June 2000 1 Introduction Hartree-Fock theory is fundamental
An Introduction to Hartree-Fock Molecular Orbital Theory C. David Sherrill School of Chemistry and Biochemistry Georgia Institute of Technology June 2000 1 Introduction Hartree-Fock theory is fundamental
Theory of EIT in an Ideal Three-Level System
 Chapter 3 Theory of EIT in an Ideal Three-Level System 3.1 Three-Level Systems So far the interaction of a two-level system (or series of two-level systems) with a near resonant, monochromatic field has
Chapter 3 Theory of EIT in an Ideal Three-Level System 3.1 Three-Level Systems So far the interaction of a two-level system (or series of two-level systems) with a near resonant, monochromatic field has
DYNAMIC ANALYSIS ON STEEL FIBRE
 International Journal of Civil Engineering and Technology (IJCIET) Volume 7, Issue 2, March-April 2016, pp. 179 184, Article ID: IJCIET_07_02_015 Available online at http://www.iaeme.com/ijciet/issues.asp?jtype=ijciet&vtype=7&itype=2
International Journal of Civil Engineering and Technology (IJCIET) Volume 7, Issue 2, March-April 2016, pp. 179 184, Article ID: IJCIET_07_02_015 Available online at http://www.iaeme.com/ijciet/issues.asp?jtype=ijciet&vtype=7&itype=2
Section 5 Molecular Electronic Spectroscopy (lecture 9 ish)
 Section 5 Molecular Electronic Spectroscopy (lecture 9 ish) Previously: Quantum theory of atoms / molecules Quantum Mechanics Vl Valence Molecular Electronic Spectroscopy Classification of electronic states
Section 5 Molecular Electronic Spectroscopy (lecture 9 ish) Previously: Quantum theory of atoms / molecules Quantum Mechanics Vl Valence Molecular Electronic Spectroscopy Classification of electronic states
