BERNOULLI NUMBERS AND POLYNOMIALS FROM A MORE GENERAL POINT OF VIEW
|
|
|
- Alisha Strickland
- 8 years ago
- Views:
Transcription
1 IT ENTE PER LE NUOVE TECNOLOGIE, L'ENERGIA E L'AMBIENTE Serie Innovazione BERNOULLI NUMBERS AND POLYNOMIALS FROM A MORE GENERAL POINT OF VIEW GIUSEPPE. DATTOLI ENEA - Divisione Fisica Applicata Centra Ricerche Frascati, Roma CLEMENTE CESARANO ULM University, Department of Matematics, ULM, Germany SILVERIA LORENZUTTA ENEA -P Divisione Fisica Applicata Centra Ricerche "Ezio Clementel", Bologna RT/INN/2000/11
2 Questo rapporto ё stato preparato da: Servizio Edizioni Scientifiche - ENEA, Centro Ricerche Frascati, C.P Frascati, Roma, Italia I contenuti tecnico-scientifici dei rapporti tecnici dell'enea rispecchiano I'opinione degli autori e non necessariamente quella dell'ente.
3 ISSN/ BERNOULLI NUMBERS AND POLYNOMIALS FROM A MORE GENERAL POINT OF VIEW Riassunto Si applica il metodo della funzione generatrice per introdurre nuove forme di numeri e polinomi di Bernoulli che vengono utilizzati per sviluppare e per calcolare somme parziali che coinvolgono polinomi a piu indici ed a piu variabili. Si sviluppano considerazioni analoghe per i polinomi ed i numeri di Eulero. BERNOULLI NUMBERS AND POLYNOMIALS FROM A MORE GENERAL POINT OF VIEW Abstract We apply the method of generating function, to introduce new forms of Bernoulli numbers and polynomials, which are exploited to derive further classes of partial sums involving generalized many index many variable polynomials. Analogous considerations are developed for the Euler numbers and polynomials. Key words: numeri di Bernoulli, polinomi di Bernoulli, polinomi di Hermite, polinomi di AppEl, somme parziali
4 INDICE 1 -INTRODUCTION p FINITE SUMS AND NEW CLASSES OF BERNOULLI NUMBERS p EULER POLYNOMIALS p CONCLUDING REMARKS p. 12 APPENDIX p. 15 REFERENCES p. 17
5 BERNOULLI NUMBERS AND POLYNOMIALS FROM A MORE GENERAL POINT OF VIEW 1 - INTRODUCTION In a previous paper [1] we have derived partial sums involving Hermite, Laguerre and Appell polynomials in terms of generalized Bernoulli polynomials. The new and interesting possibilities offered by this class of polynomials are better illustrated by an example relevant to the derivations of a partial sum involving two-index Hermite polynomials. To this aim we remind that: N-l n=0 (1) where B n (x) are Bernoulli polynomials defined by the generating function: TBnOO (2) or in terms of Bernoulli numbers B n as: Hermite polynomials with two variables and one parameter can be defined by means of the operational identity [2]: (4)
6 and the h m n (x,y x) are defined by the double sum: min(m,n) s m-s n-s l h mn (x,y x) = m!n! У (5) m ' tj s!(m-s)!(n-s)! s The identity (4) can be used to state that: and to introduce the following two variable one parameter Bernoulli polynomials: (6) h B r, s (x,y x)= JB r-qb s -kh q,k(x,y x). (7) It is easy to note that from Eq. (4) we get: e ЭхЭ У{В г (х)в 5 (у)}= ь В Г)8 (х,у х). (8) This new class of Bernoulli polynomials can be used to derive the following partial sum: M-1N-1 y r w s m=0n=0 (I В X z w -? ywj r> r., X Z M + -,. у w ywj (9) which is a consequence of Eqs. (1,6,7,8). The generating function of this last class of Bernoulli polynomials can be shown to be provided by: u v e ux + vy+xuv о» m n (10) This introductory examples shows that a wealth of implications is offered by the use of generalized forms of Bernoulli polynomials. In the forthcoming sections we will develop a
7 more systematic analysis which yields a deeper insight into the effectiveness of this type of generalizations. 2 - FINITE SUMS AND NEW CLASSES OF BERNOULLI NUMBERS In Ref. [1] we have touched on the following new class of numbers: '".f 21 n!b n _ 2s B s which are recognized as an Hermite convolution of Bernoulli numbsers on themselves. The generating functions of the B is provided by: H n 15 -z.-vh-n; (12) 1)(е 1 -: which suggests the following generalization with: t? ~ (e at -l)(e bt2 -l) n=0 xi: 1 B"(a,b) " = n ab ^ b У S \J s!(n-2s)! It is also evident that the generating function: 3 xt+yt 2 (eat _ 1)(ebt 2 _ 1} " t n /. (нв п (а,ь х,у)) (15) can be exploited to define the polynomials: B* (a,b x,y)= ip( B;_ (a,b)h ( y)) (16) H n H s s X) as also results from Eqs. (13) and (15) which are also expressed as:
8 10 * Пn! [n^2] n!a n - 2s b s цв н п nv (а,ь i х,у),yj = a b % s!(n-2s)! S KJ (17) yielding: H B n (a,b 0,0)= H B n (a,b) (18) Гп /91 V X,s.,n-2s are the K a where H n (x,y) = g!(n-2s)! п!1^=0 m P^ d e Feriet polynomials. The use of polynomials (16) is suggested by partial sums of the type: M-lN-l m=0n=0 1 (r + l)(r + 2)(r + 3) ~H B r+3 (У' w I x ' z + N w ) +H B r+3 ( У' W I X ' H B r+3 r+3 A further application of polynomials theorems. We find indeed: HB n (a,b x,y) is relevant to the multiplication HB n (mx, N 2 mn ~ 2 VVY mvl = -X X н в h 1 m TIT nr (20) m m^ and m-lm 1, k=l h=l т,-1 i ak bh u HB n a,b x +,y + -j m (21) and: p-1 q-1, 1 V 1 '% 1 k=0 h=c к h pq (22) can be proved by exploiting the procedure outlined in Appendix.
9 EULER POLYNOMIALS The examples we have provided yields an idea of the implications offered by this type of generalization. It is also evident that the considerations we have developed for Bernoulli polynomials can be extended to Euler polynomials [3]: In analogy of Ref. [1] and the results of previous sections, we introduce the following classes of Euler polynomials: 2 xt+yt2 ~ t n Х М Е ^» (24) and: m n It is easily realized that: y S E 2 ( X ) s=0 s!(n-2s)! (26) and: h E 'm > nv A >.yi m n /'щл/'^л t / ~ Zu 2-Л s=o r=o^sa r y c m-s l:: 'n-r I1 s,rv A >.y I l / \ А1 ) where E n (x) are the ordinary Euler polynomials and that the following theorems hold: _ s..., : s^ Z' w ) ( 28 > and (see appendix):
10 12 m-1 / /, \\ H E n (mx,py) = mn X (~l) k l H E n^ + ->^TJJ (29) As to the polynomials hem^c^yl't) w a can a so state l that: E m _ sn _ r (x,y t))z s w r (30) and: k=oh=o Цx pq 4 - CONCLUDING REMARKS The introduction of the Hermite-Euler polynomials given by Eq. (24) offers the possibility of speculating about alternative definitions as e.g. (t < л/тг): (е 1 +1)(е г +1) n=0 n - (32) The polynomials Е (х,у) are defined as: - 2 s ( X ) (33) s =o s! ( n - 2s >! - and are shown to satisfy the following differential equation: -E ( 2) n Эу (x,y) = ^TE ( 2) n Эх (x,y) (34) and the multiplication theorem: m-lm -1 ( f л и \Л Р 2 "]Г J (-1)^^(2)^1^^ (35)
11 13 The more general theorem relevant to Е '(mx,py) requires the introduction of a class of Euler polynomials analogous to those provided by Eq. (13) for the Bernoulli case. Before concluding this paper we want to emphasize that most of the identities holding for the ordinary Bernoulli or Euler polynomials can be extended to the generalized case. For example the identity [4]: B n (x + 1)-B n (x) = nx n - 1 (36) can be generalized as: B (x + l,y)- B (x,y) = nh _ (x,y) (37) H n H n n 1 which is a consequence of: y e 3x 2 Bn(x)=HB n (x,y). (38) For analogous reasons, we find: hb m, n (x + l,y + l T)- h B m>n (x,y + l T)- h B m>n (x + l,y T) +h B m,n<xy IT) = mnh m _ 1>n _ 1 (x,y I T) (39) and: HB* (a,b x + а,у + b) - H B^(a,b x,y) = n(n - l)(n - 2)H n _ 3 (x,y) (40) and HE ( n2) (x + l,y)+ H E ( 2) n (x,y) = 2H n (x,y). (41) We can also define the further generalized form: thus getting (n>n):
12 14 ({} {j)({} {}) ^ J ( { } ) (43) with: n=0 П ' ^ ( { } ) e 2 : i=i X i t. (44) The results if this paper show that the combination of operational rules and the properties of ordinary and generalized polynomials offer a wealth of possibilities to introduce new familes of Euler and Bernoulli polynomials which provides a powerful tool in applications.
13 15 APPENDIX The multiplication theorems are easily stated by exploiting the method of the generating functions and suitable manipulations. We note indeed that: m n (h-^m,n(p x 'qy ' r ) = (A.I) m=un=o m " П ' ( e ~* -1) The r.h.s. of the above relation can be more conveniently rewritten as: (e u -l)(e v -l) pq (e u P - by nothing that: up, p-l e - 1 r=0 we can rearrange (A.2) as: uveu P x+vqy+tuv P-l Я-1 } ~ - (up)m ( v q )n P^ X pq (A.4) which once confronted with (A.I) yields Eq. (22). A symilar procedure can be exploited to prove the multiplication theorems relevant to the Euler's generalized forms. We note indeed: 00 t n \ 2e mxt+pyt2 X ~7 (н E n (mx ' РУ)) = 1 n=0 n! e + 1 and handling the r.h.s. of the above equations, we find: (A- 5)
14 16 mt n=0 By noting that: yll/ h we obtain: n=0 n=0 (A.8) r~ (n-2r)!r! I and using the (26) we finally state the theorem (29)
15 17 REFERENCES [1 ] G. Dattoli, S. Lorenzutta and C. Cesarano, "Finite sums and generalization forms of Bernoulli polynomials", Rend. Di Matematica (1999). [2 ] G. Dattoli and A. Torre, " Theory and applications of generalized Bessel functions", ARACNE Rome (1996). [3 ] L.M. Milne-Thomson, "Calculus of finite differences", MacMillan & С Ltd., London (1951). [4] M. Abramovitz and I. Stegun, "Hand book of Mathematical Functions", Dover Pub. Inc. New York (1970).
16 Edito dall 1 Unita Comunicazione e Informazione Lungotevere Grande Ammiraglio Thaon di Revel, Roma Stampa: Centro Stampa Tecnografico - C. R. Frascati Indirizzo Internet: Finito di stampare nel mese di ottobre 2000
arxiv:1112.1570v1 [math-ph] 7 Dec 2011
![arxiv:1112.1570v1 [math-ph] 7 Dec 2011 arxiv:1112.1570v1 [math-ph] 7 Dec 2011](/thumbs/39/18196396.jpg) Umbral methods and operator ordering D. Babusci INFN - Laboratori Nazionali di Frascati, via E. Fermi, 40, IT 00044 Frascati (Roma), Italy arxiv:.570v [math-ph] 7 Dec 0 G. Dattoli ENEA - Centro Ricerche
Umbral methods and operator ordering D. Babusci INFN - Laboratori Nazionali di Frascati, via E. Fermi, 40, IT 00044 Frascati (Roma), Italy arxiv:.570v [math-ph] 7 Dec 0 G. Dattoli ENEA - Centro Ricerche
MICROCOPY RESOIUTION TEST CHART NBS 1010r; (ANSI and ISO TEST CHART No. 2)
 MICROCOPY RESOIUTION TEST CHART NBS 1010r; (ANSI and ISO TEST CHART No. 2) PHOTOORAPHIC SCIENCES CORPORATION 770 B«KET ROAD fl O. BOX 8 i n WEBST-.rt, NEW YORK U580 rr^p
MICROCOPY RESOIUTION TEST CHART NBS 1010r; (ANSI and ISO TEST CHART No. 2) PHOTOORAPHIC SCIENCES CORPORATION 770 B«KET ROAD fl O. BOX 8 i n WEBST-.rt, NEW YORK U580 rr^p
Rome, Prot. ENEN201O/4bS-00. Spett.le,
 Centro Ricerche Casaccia Unità Tecnica Modellistica Energetica Ambientale Il Responsabile 'IO...;,.,.. '. ~.,...~... -. -'0""........ '- RACCOMANDATA' A/R -- -. ( n1( I l ~ Rome, Prot. ENEN201O/4bS-00
Centro Ricerche Casaccia Unità Tecnica Modellistica Energetica Ambientale Il Responsabile 'IO...;,.,.. '. ~.,...~... -. -'0""........ '- RACCOMANDATA' A/R -- -. ( n1( I l ~ Rome, Prot. ENEN201O/4bS-00
WHAT ARE MATHEMATICAL PROOFS AND WHY THEY ARE IMPORTANT?
 WHAT ARE MATHEMATICAL PROOFS AND WHY THEY ARE IMPORTANT? introduction Many students seem to have trouble with the notion of a mathematical proof. People that come to a course like Math 216, who certainly
WHAT ARE MATHEMATICAL PROOFS AND WHY THEY ARE IMPORTANT? introduction Many students seem to have trouble with the notion of a mathematical proof. People that come to a course like Math 216, who certainly
ENEA Italian National Agency for New Technologies, Energy and Sustainable Economic Development International Fellowship Programme 2015
 AGENZIA NAZIONALE PER LE NUOVE TECNOLOGIE, L ENERGIA E LO SVILUPPO ECONOMICO SOSTENIBILE ENEA Italian National Agency for New Technologies, Energy and Sustainable Economic Development International Fellowship
AGENZIA NAZIONALE PER LE NUOVE TECNOLOGIE, L ENERGIA E LO SVILUPPO ECONOMICO SOSTENIBILE ENEA Italian National Agency for New Technologies, Energy and Sustainable Economic Development International Fellowship
Introduction to Algebraic Geometry. Bézout s Theorem and Inflection Points
 Introduction to Algebraic Geometry Bézout s Theorem and Inflection Points 1. The resultant. Let K be a field. Then the polynomial ring K[x] is a unique factorisation domain (UFD). Another example of a
Introduction to Algebraic Geometry Bézout s Theorem and Inflection Points 1. The resultant. Let K be a field. Then the polynomial ring K[x] is a unique factorisation domain (UFD). Another example of a
How To Factor By Grouping
 Lecture Notes Factoring by the AC-method page 1 Sample Problems 1. Completely factor each of the following. a) 4a 2 mn 15abm 2 6abmn + 10a 2 m 2 c) 162a + 162b 2ax 4 2bx 4 e) 3a 2 5a 2 b) a 2 x 3 b 2 x
Lecture Notes Factoring by the AC-method page 1 Sample Problems 1. Completely factor each of the following. a) 4a 2 mn 15abm 2 6abmn + 10a 2 m 2 c) 162a + 162b 2ax 4 2bx 4 e) 3a 2 5a 2 b) a 2 x 3 b 2 x
ORDINARY DIFFERENTIAL EQUATIONS
 ORDINARY DIFFERENTIAL EQUATIONS GABRIEL NAGY Mathematics Department, Michigan State University, East Lansing, MI, 48824. SEPTEMBER 4, 25 Summary. This is an introduction to ordinary differential equations.
ORDINARY DIFFERENTIAL EQUATIONS GABRIEL NAGY Mathematics Department, Michigan State University, East Lansing, MI, 48824. SEPTEMBER 4, 25 Summary. This is an introduction to ordinary differential equations.
Transient Voltage Suppressor SMBJ5.0 - SMBJ440CA
 Features: Glass passivated junction Low incremental surge resistance, excellent clamping capability 600W peak pulse power capability with a 10/1,000μs waveform, repetition rate (duty cycle): 0.01% Very
Features: Glass passivated junction Low incremental surge resistance, excellent clamping capability 600W peak pulse power capability with a 10/1,000μs waveform, repetition rate (duty cycle): 0.01% Very
9.2 Summation Notation
 9. Summation Notation 66 9. Summation Notation In the previous section, we introduced sequences and now we shall present notation and theorems concerning the sum of terms of a sequence. We begin with a
9. Summation Notation 66 9. Summation Notation In the previous section, we introduced sequences and now we shall present notation and theorems concerning the sum of terms of a sequence. We begin with a
Earthquake Hazard Zones: The relative risk of damage to Canadian buildings
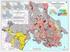 Earthquake Hazard Zones: The relative risk of damage to Canadian buildings by Paul Kovacs Executive Director, Institute for Catastrophic Loss Reduction Adjunct Research Professor, Economics, Univ. of Western
Earthquake Hazard Zones: The relative risk of damage to Canadian buildings by Paul Kovacs Executive Director, Institute for Catastrophic Loss Reduction Adjunct Research Professor, Economics, Univ. of Western
SECTION 10-2 Mathematical Induction
 73 0 Sequences and Series 6. Approximate e 0. using the first five terms of the series. Compare this approximation with your calculator evaluation of e 0.. 6. Approximate e 0.5 using the first five terms
73 0 Sequences and Series 6. Approximate e 0. using the first five terms of the series. Compare this approximation with your calculator evaluation of e 0.. 6. Approximate e 0.5 using the first five terms
LEVERRIER-FADEEV ALGORITHM AND CLASSICAL ORTHOGONAL POLYNOMIALS
 This is a reprint from Revista de la Academia Colombiana de Ciencias Vol 8 (106 (004, 39-47 LEVERRIER-FADEEV ALGORITHM AND CLASSICAL ORTHOGONAL POLYNOMIALS by J Hernández, F Marcellán & C Rodríguez LEVERRIER-FADEEV
This is a reprint from Revista de la Academia Colombiana de Ciencias Vol 8 (106 (004, 39-47 LEVERRIER-FADEEV ALGORITHM AND CLASSICAL ORTHOGONAL POLYNOMIALS by J Hernández, F Marcellán & C Rodríguez LEVERRIER-FADEEV
U.C. Berkeley CS276: Cryptography Handout 0.1 Luca Trevisan January, 2009. Notes on Algebra
 U.C. Berkeley CS276: Cryptography Handout 0.1 Luca Trevisan January, 2009 Notes on Algebra These notes contain as little theory as possible, and most results are stated without proof. Any introductory
U.C. Berkeley CS276: Cryptography Handout 0.1 Luca Trevisan January, 2009 Notes on Algebra These notes contain as little theory as possible, and most results are stated without proof. Any introductory
Using the ac Method to Factor
 4.6 Using the ac Method to Factor 4.6 OBJECTIVES 1. Use the ac test to determine factorability 2. Use the results of the ac test 3. Completely factor a trinomial In Sections 4.2 and 4.3 we used the trial-and-error
4.6 Using the ac Method to Factor 4.6 OBJECTIVES 1. Use the ac test to determine factorability 2. Use the results of the ac test 3. Completely factor a trinomial In Sections 4.2 and 4.3 we used the trial-and-error
By reversing the rules for multiplication of binomials from Section 4.6, we get rules for factoring polynomials in certain forms.
 SECTION 5.4 Special Factoring Techniques 317 5.4 Special Factoring Techniques OBJECTIVES 1 Factor a difference of squares. 2 Factor a perfect square trinomial. 3 Factor a difference of cubes. 4 Factor
SECTION 5.4 Special Factoring Techniques 317 5.4 Special Factoring Techniques OBJECTIVES 1 Factor a difference of squares. 2 Factor a perfect square trinomial. 3 Factor a difference of cubes. 4 Factor
Module MA3411: Abstract Algebra Galois Theory Appendix Michaelmas Term 2013
 Module MA3411: Abstract Algebra Galois Theory Appendix Michaelmas Term 2013 D. R. Wilkins Copyright c David R. Wilkins 1997 2013 Contents A Cyclotomic Polynomials 79 A.1 Minimum Polynomials of Roots of
Module MA3411: Abstract Algebra Galois Theory Appendix Michaelmas Term 2013 D. R. Wilkins Copyright c David R. Wilkins 1997 2013 Contents A Cyclotomic Polynomials 79 A.1 Minimum Polynomials of Roots of
ON THE EMBEDDING OF BIRKHOFF-WITT RINGS IN QUOTIENT FIELDS
 ON THE EMBEDDING OF BIRKHOFF-WITT RINGS IN QUOTIENT FIELDS DOV TAMARI 1. Introduction and general idea. In this note a natural problem arising in connection with so-called Birkhoff-Witt algebras of Lie
ON THE EMBEDDING OF BIRKHOFF-WITT RINGS IN QUOTIENT FIELDS DOV TAMARI 1. Introduction and general idea. In this note a natural problem arising in connection with so-called Birkhoff-Witt algebras of Lie
Hypermedia paradigm and Museum s didactics: a challenge for the future. Studies on Palazzo Pianetti s Application
 Federica Papa, University of Macerata (Italy) proposal What can UX Design offer to Museum s didactics? The State of the Art - growing importance of hypermediatexts in Cultural Heritage Communication; Cultural
Federica Papa, University of Macerata (Italy) proposal What can UX Design offer to Museum s didactics? The State of the Art - growing importance of hypermediatexts in Cultural Heritage Communication; Cultural
Reducibility of Second Order Differential Operators with Rational Coefficients
 Reducibility of Second Order Differential Operators with Rational Coefficients Joseph Geisbauer University of Arkansas-Fort Smith Advisor: Dr. Jill Guerra May 10, 2007 1. INTRODUCTION In this paper we
Reducibility of Second Order Differential Operators with Rational Coefficients Joseph Geisbauer University of Arkansas-Fort Smith Advisor: Dr. Jill Guerra May 10, 2007 1. INTRODUCTION In this paper we
Factoring Polynomials: Factoring by Grouping
 OpenStax-CNX module: m21901 1 Factoring Polynomials: Factoring by Grouping Wade Ellis Denny Burzynski This work is produced by OpenStax-CNX and licensed under the Creative Commons Attribution License 3.0
OpenStax-CNX module: m21901 1 Factoring Polynomials: Factoring by Grouping Wade Ellis Denny Burzynski This work is produced by OpenStax-CNX and licensed under the Creative Commons Attribution License 3.0
November 16, 2015. Interpolation, Extrapolation & Polynomial Approximation
 Interpolation, Extrapolation & Polynomial Approximation November 16, 2015 Introduction In many cases we know the values of a function f (x) at a set of points x 1, x 2,..., x N, but we don t have the analytic
Interpolation, Extrapolation & Polynomial Approximation November 16, 2015 Introduction In many cases we know the values of a function f (x) at a set of points x 1, x 2,..., x N, but we don t have the analytic
OPTIMAL SELECTION BASED ON RELATIVE RANK* (the "Secretary Problem")
 OPTIMAL SELECTION BASED ON RELATIVE RANK* (the "Secretary Problem") BY Y. S. CHOW, S. MORIGUTI, H. ROBBINS AND S. M. SAMUELS ABSTRACT n rankable persons appear sequentially in random order. At the ith
OPTIMAL SELECTION BASED ON RELATIVE RANK* (the "Secretary Problem") BY Y. S. CHOW, S. MORIGUTI, H. ROBBINS AND S. M. SAMUELS ABSTRACT n rankable persons appear sequentially in random order. At the ith
Some strong sufficient conditions for cyclic homogeneous polynomial inequalities of degree four in nonnegative variables
 Available online at www.tjnsa.com J. Nonlinear Sci. Appl. 6 (013), 74 85 Research Article Some strong sufficient conditions for cyclic homogeneous polynomial inequalities of degree four in nonnegative
Available online at www.tjnsa.com J. Nonlinear Sci. Appl. 6 (013), 74 85 Research Article Some strong sufficient conditions for cyclic homogeneous polynomial inequalities of degree four in nonnegative
CONTINUED FRACTIONS AND PELL S EQUATION. Contents 1. Continued Fractions 1 2. Solution to Pell s Equation 9 References 12
 CONTINUED FRACTIONS AND PELL S EQUATION SEUNG HYUN YANG Abstract. In this REU paper, I will use some important characteristics of continued fractions to give the complete set of solutions to Pell s equation.
CONTINUED FRACTIONS AND PELL S EQUATION SEUNG HYUN YANG Abstract. In this REU paper, I will use some important characteristics of continued fractions to give the complete set of solutions to Pell s equation.
Section 6.1 Factoring Expressions
 Section 6.1 Factoring Expressions The first method we will discuss, in solving polynomial equations, is the method of FACTORING. Before we jump into this process, you need to have some concept of what
Section 6.1 Factoring Expressions The first method we will discuss, in solving polynomial equations, is the method of FACTORING. Before we jump into this process, you need to have some concept of what
Probability Generating Functions
 page 39 Chapter 3 Probability Generating Functions 3 Preamble: Generating Functions Generating functions are widely used in mathematics, and play an important role in probability theory Consider a sequence
page 39 Chapter 3 Probability Generating Functions 3 Preamble: Generating Functions Generating functions are widely used in mathematics, and play an important role in probability theory Consider a sequence
LISTA RIVISTE MASTER 2013
 LISTA RIVISTE MASTER 2013 Fascie A+, A, B e P Le riviste classificate come P sono riviste di grande qualità e di rilevante impatto il cui fine principale e quello di diffondere le conoscenze scientifiche,
LISTA RIVISTE MASTER 2013 Fascie A+, A, B e P Le riviste classificate come P sono riviste di grande qualità e di rilevante impatto il cui fine principale e quello di diffondere le conoscenze scientifiche,
The Characteristic Polynomial
 Physics 116A Winter 2011 The Characteristic Polynomial 1 Coefficients of the characteristic polynomial Consider the eigenvalue problem for an n n matrix A, A v = λ v, v 0 (1) The solution to this problem
Physics 116A Winter 2011 The Characteristic Polynomial 1 Coefficients of the characteristic polynomial Consider the eigenvalue problem for an n n matrix A, A v = λ v, v 0 (1) The solution to this problem
Generating Functions Count
 2 Generating Functions Count 2.1 Counting from Polynomials to Power Series Consider the outcomes when a pair of dice are thrown and our interest is the sum of the numbers showing. One way to model the
2 Generating Functions Count 2.1 Counting from Polynomials to Power Series Consider the outcomes when a pair of dice are thrown and our interest is the sum of the numbers showing. One way to model the
DIFFERENTIABILITY OF COMPLEX FUNCTIONS. Contents
 DIFFERENTIABILITY OF COMPLEX FUNCTIONS Contents 1. Limit definition of a derivative 1 2. Holomorphic functions, the Cauchy-Riemann equations 3 3. Differentiability of real functions 5 4. A sufficient condition
DIFFERENTIABILITY OF COMPLEX FUNCTIONS Contents 1. Limit definition of a derivative 1 2. Holomorphic functions, the Cauchy-Riemann equations 3 3. Differentiability of real functions 5 4. A sufficient condition
Introduction. Appendix D Mathematical Induction D1
 Appendix D Mathematical Induction D D Mathematical Induction Use mathematical induction to prove a formula. Find a sum of powers of integers. Find a formula for a finite sum. Use finite differences to
Appendix D Mathematical Induction D D Mathematical Induction Use mathematical induction to prove a formula. Find a sum of powers of integers. Find a formula for a finite sum. Use finite differences to
k, then n = p2α 1 1 pα k
 Powers of Integers An integer n is a perfect square if n = m for some integer m. Taking into account the prime factorization, if m = p α 1 1 pα k k, then n = pα 1 1 p α k k. That is, n is a perfect square
Powers of Integers An integer n is a perfect square if n = m for some integer m. Taking into account the prime factorization, if m = p α 1 1 pα k k, then n = pα 1 1 p α k k. That is, n is a perfect square
Mathematical Induction
 Mathematical Induction (Handout March 8, 01) The Principle of Mathematical Induction provides a means to prove infinitely many statements all at once The principle is logical rather than strictly mathematical,
Mathematical Induction (Handout March 8, 01) The Principle of Mathematical Induction provides a means to prove infinitely many statements all at once The principle is logical rather than strictly mathematical,
x(x + 5) x 2 25 (x + 5)(x 5) = x 6(x 4) x ( x 4) + 3
 CORE 4 Summary Notes Rational Expressions Factorise all expressions where possible Cancel any factors common to the numerator and denominator x + 5x x(x + 5) x 5 (x + 5)(x 5) x x 5 To add or subtract -
CORE 4 Summary Notes Rational Expressions Factorise all expressions where possible Cancel any factors common to the numerator and denominator x + 5x x(x + 5) x 5 (x + 5)(x 5) x x 5 To add or subtract -
Quotient Rings and Field Extensions
 Chapter 5 Quotient Rings and Field Extensions In this chapter we describe a method for producing field extension of a given field. If F is a field, then a field extension is a field K that contains F.
Chapter 5 Quotient Rings and Field Extensions In this chapter we describe a method for producing field extension of a given field. If F is a field, then a field extension is a field K that contains F.
SOLVING LINEAR SYSTEMS
 SOLVING LINEAR SYSTEMS Linear systems Ax = b occur widely in applied mathematics They occur as direct formulations of real world problems; but more often, they occur as a part of the numerical analysis
SOLVING LINEAR SYSTEMS Linear systems Ax = b occur widely in applied mathematics They occur as direct formulations of real world problems; but more often, they occur as a part of the numerical analysis
1.5. Factorisation. Introduction. Prerequisites. Learning Outcomes. Learning Style
 Factorisation 1.5 Introduction In Block 4 we showed the way in which brackets were removed from algebraic expressions. Factorisation, which can be considered as the reverse of this process, is dealt with
Factorisation 1.5 Introduction In Block 4 we showed the way in which brackets were removed from algebraic expressions. Factorisation, which can be considered as the reverse of this process, is dealt with
1. Oblast rozvoj spolků a SU UK 1.1. Zvyšování kvalifikace Školení Zapojení do projektů Poradenství 1.2. Financování 1.2.1.
 1. O b l a s t r o z v o j s p o l k a S U U K 1. 1. Z v y š o v á n í k v a l i f i k a c e Š k o l e n í o S t u d e n t s k á u n i e U n i v e r z i t y K a r l o v y ( d á l e j e n S U U K ) z í
1. O b l a s t r o z v o j s p o l k a S U U K 1. 1. Z v y š o v á n í k v a l i f i k a c e Š k o l e n í o S t u d e n t s k á u n i e U n i v e r z i t y K a r l o v y ( d á l e j e n S U U K ) z í
Matrix Algebra. Some Basic Matrix Laws. Before reading the text or the following notes glance at the following list of basic matrix algebra laws.
 Matrix Algebra A. Doerr Before reading the text or the following notes glance at the following list of basic matrix algebra laws. Some Basic Matrix Laws Assume the orders of the matrices are such that
Matrix Algebra A. Doerr Before reading the text or the following notes glance at the following list of basic matrix algebra laws. Some Basic Matrix Laws Assume the orders of the matrices are such that
Analysis-suitable T-splines of arbitrary degree: definition and properties
 Mathematical Models and Methods in Applied Sciences c World Scientific Publishing Company Analysis-suitable T-splines of arbitrary degree: definition and properties L. Beirão da Veiga, A. Buffa, G. Sangalli,
Mathematical Models and Methods in Applied Sciences c World Scientific Publishing Company Analysis-suitable T-splines of arbitrary degree: definition and properties L. Beirão da Veiga, A. Buffa, G. Sangalli,
Section 1. Finding Common Terms
 Worksheet 2.1 Factors of Algebraic Expressions Section 1 Finding Common Terms In worksheet 1.2 we talked about factors of whole numbers. Remember, if a b = ab then a is a factor of ab and b is a factor
Worksheet 2.1 Factors of Algebraic Expressions Section 1 Finding Common Terms In worksheet 1.2 we talked about factors of whole numbers. Remember, if a b = ab then a is a factor of ab and b is a factor
FACTORING LARGE NUMBERS, A GREAT WAY TO SPEND A BIRTHDAY
 FACTORING LARGE NUMBERS, A GREAT WAY TO SPEND A BIRTHDAY LINDSEY R. BOSKO I would like to acknowledge the assistance of Dr. Michael Singer. His guidance and feedback were instrumental in completing this
FACTORING LARGE NUMBERS, A GREAT WAY TO SPEND A BIRTHDAY LINDSEY R. BOSKO I would like to acknowledge the assistance of Dr. Michael Singer. His guidance and feedback were instrumental in completing this
CORPORATE VALUATIONS AND THE MERTON MODEL
 UNIVERSITÀ DEGLI STUDI ROMA TRE DIPARTIMENTO DI ECONOMIA CORPORATE VALUATIONS AND THE MERTON MODEL Andrea Gheno Working Paper n 55, 2005 UNIVERSITÀ DEGLI STUDI ROMA TRE DIPARTIMENTO DI ECONOMIA Working
UNIVERSITÀ DEGLI STUDI ROMA TRE DIPARTIMENTO DI ECONOMIA CORPORATE VALUATIONS AND THE MERTON MODEL Andrea Gheno Working Paper n 55, 2005 UNIVERSITÀ DEGLI STUDI ROMA TRE DIPARTIMENTO DI ECONOMIA Working
ECG590I Asset Pricing. Lecture 2: Present Value 1
 ECG59I Asset Pricing. Lecture 2: Present Value 1 2 Present Value If you have to decide between receiving 1$ now or 1$ one year from now, then you would rather have your money now. If you have to decide
ECG59I Asset Pricing. Lecture 2: Present Value 1 2 Present Value If you have to decide between receiving 1$ now or 1$ one year from now, then you would rather have your money now. If you have to decide
Pricing of Limit Orders in the Electronic Security Trading System Xetra
 Pricing of Limit Orders in the Electronic Security Trading System Xetra Li Xihao Bielefeld Graduate School of Economics and Management Bielefeld University, P.O. Box 100 131 D-33501 Bielefeld, Germany
Pricing of Limit Orders in the Electronic Security Trading System Xetra Li Xihao Bielefeld Graduate School of Economics and Management Bielefeld University, P.O. Box 100 131 D-33501 Bielefeld, Germany
On closed-form solutions to a class of ordinary differential equations
 International Journal of Advanced Mathematical Sciences, 2 (1 (2014 57-70 c Science Publishing Corporation www.sciencepubco.com/index.php/ijams doi: 10.14419/ijams.v2i1.1556 Research Paper On closed-form
International Journal of Advanced Mathematical Sciences, 2 (1 (2014 57-70 c Science Publishing Corporation www.sciencepubco.com/index.php/ijams doi: 10.14419/ijams.v2i1.1556 Research Paper On closed-form
THREE DIMENSIONAL GEOMETRY
 Chapter 8 THREE DIMENSIONAL GEOMETRY 8.1 Introduction In this chapter we present a vector algebra approach to three dimensional geometry. The aim is to present standard properties of lines and planes,
Chapter 8 THREE DIMENSIONAL GEOMETRY 8.1 Introduction In this chapter we present a vector algebra approach to three dimensional geometry. The aim is to present standard properties of lines and planes,
The Membrane Equation
 The Membrane Equation Professor David Heeger September 5, 2000 RC Circuits Figure 1A shows an RC (resistor, capacitor) equivalent circuit model for a patch of passive neural membrane. The capacitor represents
The Membrane Equation Professor David Heeger September 5, 2000 RC Circuits Figure 1A shows an RC (resistor, capacitor) equivalent circuit model for a patch of passive neural membrane. The capacitor represents
So far, we have looked at homogeneous equations
 Chapter 3.6: equations Non-homogeneous So far, we have looked at homogeneous equations L[y] = y + p(t)y + q(t)y = 0. Homogeneous means that the right side is zero. Linear homogeneous equations satisfy
Chapter 3.6: equations Non-homogeneous So far, we have looked at homogeneous equations L[y] = y + p(t)y + q(t)y = 0. Homogeneous means that the right side is zero. Linear homogeneous equations satisfy
Corso: Mastering Microsoft Project 2010 Codice PCSNET: MSPJ-11 Cod. Vendor: 50413 Durata: 3
 Corso: Mastering Microsoft Project 2010 Codice PCSNET: MSPJ-11 Cod. Vendor: 50413 Durata: 3 Obiettivi Comprendere la disciplina del project management in quanto si applica all'utilizzo di Project. Apprendere
Corso: Mastering Microsoft Project 2010 Codice PCSNET: MSPJ-11 Cod. Vendor: 50413 Durata: 3 Obiettivi Comprendere la disciplina del project management in quanto si applica all'utilizzo di Project. Apprendere
Introduction to Partial Differential Equations. John Douglas Moore
 Introduction to Partial Differential Equations John Douglas Moore May 2, 2003 Preface Partial differential equations are often used to construct models of the most basic theories underlying physics and
Introduction to Partial Differential Equations John Douglas Moore May 2, 2003 Preface Partial differential equations are often used to construct models of the most basic theories underlying physics and
Some Polynomial Theorems. John Kennedy Mathematics Department Santa Monica College 1900 Pico Blvd. Santa Monica, CA 90405 rkennedy@ix.netcom.
 Some Polynomial Theorems by John Kennedy Mathematics Department Santa Monica College 1900 Pico Blvd. Santa Monica, CA 90405 rkennedy@ix.netcom.com This paper contains a collection of 31 theorems, lemmas,
Some Polynomial Theorems by John Kennedy Mathematics Department Santa Monica College 1900 Pico Blvd. Santa Monica, CA 90405 rkennedy@ix.netcom.com This paper contains a collection of 31 theorems, lemmas,
How To Solve Power Series
 Methods and Applications of Power Series By Jay A. Leavitt Power series in the past played a minor role in the numerical solutions of ordinary and partial differential equations. There have been good reasons.
Methods and Applications of Power Series By Jay A. Leavitt Power series in the past played a minor role in the numerical solutions of ordinary and partial differential equations. There have been good reasons.
Curriculum Vitae et Studiorum Dossier n. 48773. Cinzia Di Giusto
 Curriculum Vitae et Studiorum Dossier n. 48773 Cinzia Di Giusto December 31, 2010 Personal Information: Date of birth: Nationality: Di Giusto, Cinzia Oct 17, 1979 in Udine, Italy Italian Home address:
Curriculum Vitae et Studiorum Dossier n. 48773 Cinzia Di Giusto December 31, 2010 Personal Information: Date of birth: Nationality: Di Giusto, Cinzia Oct 17, 1979 in Udine, Italy Italian Home address:
 C o a t i a n P u b l i c D e b tm a n a g e m e n t a n d C h a l l e n g e s o f M a k e t D e v e l o p m e n t Z a g e bo 8 t h A p i l 2 0 1 1 h t t pdd w w wp i j fp h D p u b l i c2 d e b td S t
C o a t i a n P u b l i c D e b tm a n a g e m e n t a n d C h a l l e n g e s o f M a k e t D e v e l o p m e n t Z a g e bo 8 t h A p i l 2 0 1 1 h t t pdd w w wp i j fp h D p u b l i c2 d e b td S t
Chapter 4, Arithmetic in F [x] Polynomial arithmetic and the division algorithm.
![Chapter 4, Arithmetic in F [x] Polynomial arithmetic and the division algorithm. Chapter 4, Arithmetic in F [x] Polynomial arithmetic and the division algorithm.](/thumbs/26/8276997.jpg) Chapter 4, Arithmetic in F [x] Polynomial arithmetic and the division algorithm. We begin by defining the ring of polynomials with coefficients in a ring R. After some preliminary results, we specialize
Chapter 4, Arithmetic in F [x] Polynomial arithmetic and the division algorithm. We begin by defining the ring of polynomials with coefficients in a ring R. After some preliminary results, we specialize
Tim Kerins. Leaving Certificate Honours Maths - Algebra. Tim Kerins. the date
 Leaving Certificate Honours Maths - Algebra the date Chapter 1 Algebra This is an important portion of the course. As well as generally accounting for 2 3 questions in examination it is the basis for many
Leaving Certificate Honours Maths - Algebra the date Chapter 1 Algebra This is an important portion of the course. As well as generally accounting for 2 3 questions in examination it is the basis for many
Elementary Differential Equations and Boundary Value Problems. 10th Edition International Student Version
 Brochure More information from http://www.researchandmarkets.com/reports/3148843/ Elementary Differential Equations and Boundary Value Problems. 10th Edition International Student Version Description:
Brochure More information from http://www.researchandmarkets.com/reports/3148843/ Elementary Differential Equations and Boundary Value Problems. 10th Edition International Student Version Description:
Revised Version of Chapter 23. We learned long ago how to solve linear congruences. ax c (mod m)
 Chapter 23 Squares Modulo p Revised Version of Chapter 23 We learned long ago how to solve linear congruences ax c (mod m) (see Chapter 8). It s now time to take the plunge and move on to quadratic equations.
Chapter 23 Squares Modulo p Revised Version of Chapter 23 We learned long ago how to solve linear congruences ax c (mod m) (see Chapter 8). It s now time to take the plunge and move on to quadratic equations.
SECTION 0.6: POLYNOMIAL, RATIONAL, AND ALGEBRAIC EXPRESSIONS
 (Section 0.6: Polynomial, Rational, and Algebraic Expressions) 0.6.1 SECTION 0.6: POLYNOMIAL, RATIONAL, AND ALGEBRAIC EXPRESSIONS LEARNING OBJECTIVES Be able to identify polynomial, rational, and algebraic
(Section 0.6: Polynomial, Rational, and Algebraic Expressions) 0.6.1 SECTION 0.6: POLYNOMIAL, RATIONAL, AND ALGEBRAIC EXPRESSIONS LEARNING OBJECTIVES Be able to identify polynomial, rational, and algebraic
HOMEWORK 5 SOLUTIONS. n!f n (1) lim. ln x n! + xn x. 1 = G n 1 (x). (2) k + 1 n. (n 1)!
 Math 7 Fall 205 HOMEWORK 5 SOLUTIONS Problem. 2008 B2 Let F 0 x = ln x. For n 0 and x > 0, let F n+ x = 0 F ntdt. Evaluate n!f n lim n ln n. By directly computing F n x for small n s, we obtain the following
Math 7 Fall 205 HOMEWORK 5 SOLUTIONS Problem. 2008 B2 Let F 0 x = ln x. For n 0 and x > 0, let F n+ x = 0 F ntdt. Evaluate n!f n lim n ln n. By directly computing F n x for small n s, we obtain the following
CM2202: Scientific Computing and Multimedia Applications General Maths: 2. Algebra - Factorisation
 CM2202: Scientific Computing and Multimedia Applications General Maths: 2. Algebra - Factorisation Prof. David Marshall School of Computer Science & Informatics Factorisation Factorisation is a way of
CM2202: Scientific Computing and Multimedia Applications General Maths: 2. Algebra - Factorisation Prof. David Marshall School of Computer Science & Informatics Factorisation Factorisation is a way of
Ministry of Economic Development
 Ministry of Economic Development Department of Communications Contribution of ICT to economic growth in Italy: Input Output analysis DEPARTMENT OF COMMUNICATIONS Relatore: n Claudio Di Carlo claudio.dicarlo@sviluppoeconomico.gov.it
Ministry of Economic Development Department of Communications Contribution of ICT to economic growth in Italy: Input Output analysis DEPARTMENT OF COMMUNICATIONS Relatore: n Claudio Di Carlo claudio.dicarlo@sviluppoeconomico.gov.it
Methods for Finding Bases
 Methods for Finding Bases Bases for the subspaces of a matrix Row-reduction methods can be used to find bases. Let us now look at an example illustrating how to obtain bases for the row space, null space,
Methods for Finding Bases Bases for the subspaces of a matrix Row-reduction methods can be used to find bases. Let us now look at an example illustrating how to obtain bases for the row space, null space,
Ministero dell Economia e delle Finanze
 Dear Investor, the Treasury is pleased to come back to you with the 0 calendar of government bond auctions, which can be a useful instrument to assist you in your investment decisions. For every month,
Dear Investor, the Treasury is pleased to come back to you with the 0 calendar of government bond auctions, which can be a useful instrument to assist you in your investment decisions. For every month,
FIRST YEAR CALCULUS. Chapter 7 CONTINUITY. It is a parabola, and we can draw this parabola without lifting our pencil from the paper.
 FIRST YEAR CALCULUS WWLCHENW L c WWWL W L Chen, 1982, 2008. 2006. This chapter originates from material used by the author at Imperial College, University of London, between 1981 and 1990. It It is is
FIRST YEAR CALCULUS WWLCHENW L c WWWL W L Chen, 1982, 2008. 2006. This chapter originates from material used by the author at Imperial College, University of London, between 1981 and 1990. It It is is
( ) FACTORING. x In this polynomial the only variable in common to all is x.
 FACTORING Factoring is similar to breaking up a number into its multiples. For example, 10=5*. The multiples are 5 and. In a polynomial it is the same way, however, the procedure is somewhat more complicated
FACTORING Factoring is similar to breaking up a number into its multiples. For example, 10=5*. The multiples are 5 and. In a polynomial it is the same way, however, the procedure is somewhat more complicated
General Theory of Differential Equations Sections 2.8, 3.1-3.2, 4.1
 A B I L E N E C H R I S T I A N U N I V E R S I T Y Department of Mathematics General Theory of Differential Equations Sections 2.8, 3.1-3.2, 4.1 Dr. John Ehrke Department of Mathematics Fall 2012 Questions
A B I L E N E C H R I S T I A N U N I V E R S I T Y Department of Mathematics General Theory of Differential Equations Sections 2.8, 3.1-3.2, 4.1 Dr. John Ehrke Department of Mathematics Fall 2012 Questions
Introduction to Finite Fields (cont.)
 Chapter 6 Introduction to Finite Fields (cont.) 6.1 Recall Theorem. Z m is a field m is a prime number. Theorem (Subfield Isomorphic to Z p ). Every finite field has the order of a power of a prime number
Chapter 6 Introduction to Finite Fields (cont.) 6.1 Recall Theorem. Z m is a field m is a prime number. Theorem (Subfield Isomorphic to Z p ). Every finite field has the order of a power of a prime number
1.3 Polynomials and Factoring
 1.3 Polynomials and Factoring Polynomials Constant: a number, such as 5 or 27 Variable: a letter or symbol that represents a value. Term: a constant, variable, or the product or a constant and variable.
1.3 Polynomials and Factoring Polynomials Constant: a number, such as 5 or 27 Variable: a letter or symbol that represents a value. Term: a constant, variable, or the product or a constant and variable.
Taylor and Maclaurin Series
 Taylor and Maclaurin Series In the preceding section we were able to find power series representations for a certain restricted class of functions. Here we investigate more general problems: Which functions
Taylor and Maclaurin Series In the preceding section we were able to find power series representations for a certain restricted class of functions. Here we investigate more general problems: Which functions
Reference: Introduction to Partial Differential Equations by G. Folland, 1995, Chap. 3.
 5 Potential Theory Reference: Introduction to Partial Differential Equations by G. Folland, 995, Chap. 3. 5. Problems of Interest. In what follows, we consider Ω an open, bounded subset of R n with C 2
5 Potential Theory Reference: Introduction to Partial Differential Equations by G. Folland, 995, Chap. 3. 5. Problems of Interest. In what follows, we consider Ω an open, bounded subset of R n with C 2
0.4 FACTORING POLYNOMIALS
 36_.qxd /3/5 :9 AM Page -9 SECTION. Factoring Polynomials -9. FACTORING POLYNOMIALS Use special products and factorization techniques to factor polynomials. Find the domains of radical expressions. Use
36_.qxd /3/5 :9 AM Page -9 SECTION. Factoring Polynomials -9. FACTORING POLYNOMIALS Use special products and factorization techniques to factor polynomials. Find the domains of radical expressions. Use
4. CLASSES OF RINGS 4.1. Classes of Rings class operator A-closed Example 1: product Example 2:
 4. CLASSES OF RINGS 4.1. Classes of Rings Normally we associate, with any property, a set of objects that satisfy that property. But problems can arise when we allow sets to be elements of larger sets
4. CLASSES OF RINGS 4.1. Classes of Rings Normally we associate, with any property, a set of objects that satisfy that property. But problems can arise when we allow sets to be elements of larger sets
4. FIRST STEPS IN THE THEORY 4.1. A
 4. FIRST STEPS IN THE THEORY 4.1. A Catalogue of All Groups: The Impossible Dream The fundamental problem of group theory is to systematically explore the landscape and to chart what lies out there. We
4. FIRST STEPS IN THE THEORY 4.1. A Catalogue of All Groups: The Impossible Dream The fundamental problem of group theory is to systematically explore the landscape and to chart what lies out there. We
Continuous Groups, Lie Groups, and Lie Algebras
 Chapter 7 Continuous Groups, Lie Groups, and Lie Algebras Zeno was concerned with three problems... These are the problem of the infinitesimal, the infinite, and continuity... Bertrand Russell The groups
Chapter 7 Continuous Groups, Lie Groups, and Lie Algebras Zeno was concerned with three problems... These are the problem of the infinitesimal, the infinite, and continuity... Bertrand Russell The groups
SCHWEITZER ENGINEERING LABORATORIES, COMERCIAL LTDA.
 Pocket book of Electrical Engineering Formulas Content 1. Elementary Algebra and Geometry 1. Fundamental Properties (real numbers) 1 2. Exponents 2 3. Fractional Exponents 2 4. Irrational Exponents 2 5.
Pocket book of Electrical Engineering Formulas Content 1. Elementary Algebra and Geometry 1. Fundamental Properties (real numbers) 1 2. Exponents 2 3. Fractional Exponents 2 4. Irrational Exponents 2 5.
a 11 x 1 + a 12 x 2 + + a 1n x n = b 1 a 21 x 1 + a 22 x 2 + + a 2n x n = b 2.
 Chapter 1 LINEAR EQUATIONS 1.1 Introduction to linear equations A linear equation in n unknowns x 1, x,, x n is an equation of the form a 1 x 1 + a x + + a n x n = b, where a 1, a,..., a n, b are given
Chapter 1 LINEAR EQUATIONS 1.1 Introduction to linear equations A linear equation in n unknowns x 1, x,, x n is an equation of the form a 1 x 1 + a x + + a n x n = b, where a 1, a,..., a n, b are given
Victims Compensation Claim Status of All Pending Claims and Claims Decided Within the Last Three Years
 Claim#:021914-174 Initials: J.T. Last4SSN: 6996 DOB: 5/3/1970 Crime Date: 4/30/2013 Status: Claim is currently under review. Decision expected within 7 days Claim#:041715-334 Initials: M.S. Last4SSN: 2957
Claim#:021914-174 Initials: J.T. Last4SSN: 6996 DOB: 5/3/1970 Crime Date: 4/30/2013 Status: Claim is currently under review. Decision expected within 7 days Claim#:041715-334 Initials: M.S. Last4SSN: 2957
MBA Jump Start Program
 MBA Jump Start Program Module 2: Mathematics Thomas Gilbert Mathematics Module Online Appendix: Basic Mathematical Concepts 2 1 The Number Spectrum Generally we depict numbers increasing from left to right
MBA Jump Start Program Module 2: Mathematics Thomas Gilbert Mathematics Module Online Appendix: Basic Mathematical Concepts 2 1 The Number Spectrum Generally we depict numbers increasing from left to right
arxiv:math/0601660v3 [math.nt] 25 Feb 2006
![arxiv:math/0601660v3 [math.nt] 25 Feb 2006 arxiv:math/0601660v3 [math.nt] 25 Feb 2006](/thumbs/40/21422467.jpg) NOTES Edited by William Adkins arxiv:math/666v3 [math.nt] 25 Feb 26 A Short Proof of the Simple Continued Fraction Expansion of e Henry Cohn. INTRODUCTION. In [3], Euler analyzed the Riccati equation to
NOTES Edited by William Adkins arxiv:math/666v3 [math.nt] 25 Feb 26 A Short Proof of the Simple Continued Fraction Expansion of e Henry Cohn. INTRODUCTION. In [3], Euler analyzed the Riccati equation to
Nuovi domini di primo livello - Registra nuove estensioni con FabsWeb_HOST
 Oltre 700 nuove estensioni per domini personalizzati Il conto alla rovescia è terminato! Finalmente più di 700 nuove estensioni di dominio gtld stanno per arrivare sul mercato e sono destinate a rivoluzionare
Oltre 700 nuove estensioni per domini personalizzati Il conto alla rovescia è terminato! Finalmente più di 700 nuove estensioni di dominio gtld stanno per arrivare sul mercato e sono destinate a rivoluzionare
Exercise Set 3. Similar triangles. Parallel lines
 Exercise Set 3. Similar triangles Parallel lines Note: The exercises marked with are more difficult and go beyond the course/examination requirements. (1) Let ABC be a triangle with AB = AC. Let D be an
Exercise Set 3. Similar triangles Parallel lines Note: The exercises marked with are more difficult and go beyond the course/examination requirements. (1) Let ABC be a triangle with AB = AC. Let D be an
The Mean Value Theorem
 The Mean Value Theorem THEOREM (The Extreme Value Theorem): If f is continuous on a closed interval [a, b], then f attains an absolute maximum value f(c) and an absolute minimum value f(d) at some numbers
The Mean Value Theorem THEOREM (The Extreme Value Theorem): If f is continuous on a closed interval [a, b], then f attains an absolute maximum value f(c) and an absolute minimum value f(d) at some numbers
Solutions to Homework 10
 Solutions to Homework 1 Section 7., exercise # 1 (b,d): (b) Compute the value of R f dv, where f(x, y) = y/x and R = [1, 3] [, 4]. Solution: Since f is continuous over R, f is integrable over R. Let x
Solutions to Homework 1 Section 7., exercise # 1 (b,d): (b) Compute the value of R f dv, where f(x, y) = y/x and R = [1, 3] [, 4]. Solution: Since f is continuous over R, f is integrable over R. Let x
Linear Maps. Isaiah Lankham, Bruno Nachtergaele, Anne Schilling (February 5, 2007)
 MAT067 University of California, Davis Winter 2007 Linear Maps Isaiah Lankham, Bruno Nachtergaele, Anne Schilling (February 5, 2007) As we have discussed in the lecture on What is Linear Algebra? one of
MAT067 University of California, Davis Winter 2007 Linear Maps Isaiah Lankham, Bruno Nachtergaele, Anne Schilling (February 5, 2007) As we have discussed in the lecture on What is Linear Algebra? one of
Understanding Basic Calculus
 Understanding Basic Calculus S.K. Chung Dedicated to all the people who have helped me in my life. i Preface This book is a revised and expanded version of the lecture notes for Basic Calculus and other
Understanding Basic Calculus S.K. Chung Dedicated to all the people who have helped me in my life. i Preface This book is a revised and expanded version of the lecture notes for Basic Calculus and other
On the irreducibility of certain polynomials with coefficients as products of terms in an arithmetic progression
 On the irreducibility of certain polynomials with coefficients as products of terms in an arithmetic progression Carrie E. Finch and N. Saradha Abstract We prove the irreducibility of ceratin polynomials
On the irreducibility of certain polynomials with coefficients as products of terms in an arithmetic progression Carrie E. Finch and N. Saradha Abstract We prove the irreducibility of ceratin polynomials
Certainty Equivalent in Capital Markets
 Certainty Equivalent in Capital Markets Lutz Kruschwitz Freie Universität Berlin and Andreas Löffler 1 Universität Hannover version from January 23, 2003 1 Corresponding author, Königsworther Platz 1,
Certainty Equivalent in Capital Markets Lutz Kruschwitz Freie Universität Berlin and Andreas Löffler 1 Universität Hannover version from January 23, 2003 1 Corresponding author, Königsworther Platz 1,
Maximum Hitting Time for Random Walks on Graphs. Graham Brightwell, Cambridge University Peter Winkler*, Emory University
 Maximum Hitting Time for Random Walks on Graphs Graham Brightwell, Cambridge University Peter Winkler*, Emory University Abstract. For x and y vertices of a connected graph G, let T G (x, y denote the
Maximum Hitting Time for Random Walks on Graphs Graham Brightwell, Cambridge University Peter Winkler*, Emory University Abstract. For x and y vertices of a connected graph G, let T G (x, y denote the
n k=1 k=0 1/k! = e. Example 6.4. The series 1/k 2 converges in R. Indeed, if s n = n then k=1 1/k, then s 2n s n = 1 n + 1 +...
 6 Series We call a normed space (X, ) a Banach space provided that every Cauchy sequence (x n ) in X converges. For example, R with the norm = is an example of Banach space. Now let (x n ) be a sequence
6 Series We call a normed space (X, ) a Banach space provided that every Cauchy sequence (x n ) in X converges. For example, R with the norm = is an example of Banach space. Now let (x n ) be a sequence
Mathematics 31 Pre-calculus and Limits
 Mathematics 31 Pre-calculus and Limits Overview After completing this section, students will be epected to have acquired reliability and fluency in the algebraic skills of factoring, operations with radicals
Mathematics 31 Pre-calculus and Limits Overview After completing this section, students will be epected to have acquired reliability and fluency in the algebraic skills of factoring, operations with radicals
Second Order Differential Equations with Hypergeometric Solutions of Degree Three
 Second Order Differential Equations with Hypergeometric Solutions of Degree Three Vijay Jung Kunwar, Mark van Hoeij Department of Mathematics, Florida State University Tallahassee, FL 0-07, USA vkunwar@mathfsuedu,
Second Order Differential Equations with Hypergeometric Solutions of Degree Three Vijay Jung Kunwar, Mark van Hoeij Department of Mathematics, Florida State University Tallahassee, FL 0-07, USA vkunwar@mathfsuedu,
COPIA TRATTA DA GURITEL GAZZETTA UFFICIALE ON-LINE
 Supplemento ordinario alla Gazzetta Ufficiale,, n. 288 del 12 dicembre 2006 - Serie generale Spediz. abb. post. 45% - art. 2, comma 20/b Legge 23-12-1996, n. 662 - Filiale di Roma SI PUBBLICA TUTTI PARTE
Supplemento ordinario alla Gazzetta Ufficiale,, n. 288 del 12 dicembre 2006 - Serie generale Spediz. abb. post. 45% - art. 2, comma 20/b Legge 23-12-1996, n. 662 - Filiale di Roma SI PUBBLICA TUTTI PARTE
The Australian Journal of Mathematical Analysis and Applications
 The Australian Journal of Mathematical Analysis and Applications Volume 7, Issue, Article 11, pp. 1-14, 011 SOME HOMOGENEOUS CYCLIC INEQUALITIES OF THREE VARIABLES OF DEGREE THREE AND FOUR TETSUYA ANDO
The Australian Journal of Mathematical Analysis and Applications Volume 7, Issue, Article 11, pp. 1-14, 011 SOME HOMOGENEOUS CYCLIC INEQUALITIES OF THREE VARIABLES OF DEGREE THREE AND FOUR TETSUYA ANDO
3. INNER PRODUCT SPACES
 . INNER PRODUCT SPACES.. Definition So far we have studied abstract vector spaces. These are a generalisation of the geometric spaces R and R. But these have more structure than just that of a vector space.
. INNER PRODUCT SPACES.. Definition So far we have studied abstract vector spaces. These are a generalisation of the geometric spaces R and R. But these have more structure than just that of a vector space.
CHAPTER II THE LIMIT OF A SEQUENCE OF NUMBERS DEFINITION OF THE NUMBER e.
 CHAPTER II THE LIMIT OF A SEQUENCE OF NUMBERS DEFINITION OF THE NUMBER e. This chapter contains the beginnings of the most important, and probably the most subtle, notion in mathematical analysis, i.e.,
CHAPTER II THE LIMIT OF A SEQUENCE OF NUMBERS DEFINITION OF THE NUMBER e. This chapter contains the beginnings of the most important, and probably the most subtle, notion in mathematical analysis, i.e.,
How To Learn Math At A Junior High
 MATH DEPARTMENT COURSE DESCRIPTIONS The Mathematics Department provides a challenging curriculum that strives to meet the needs of a diverse student body by: Helping the student realize that the analytical
MATH DEPARTMENT COURSE DESCRIPTIONS The Mathematics Department provides a challenging curriculum that strives to meet the needs of a diverse student body by: Helping the student realize that the analytical
SOL Warm-Up Graphing Calculator Active
 A.2a (a) Using laws of exponents to simplify monomial expressions and ratios of monomial expressions 1. Which expression is equivalent to (5x 2 )(4x 5 )? A 9x 7 B 9x 10 C 20x 7 D 20x 10 2. Which expression
A.2a (a) Using laws of exponents to simplify monomial expressions and ratios of monomial expressions 1. Which expression is equivalent to (5x 2 )(4x 5 )? A 9x 7 B 9x 10 C 20x 7 D 20x 10 2. Which expression
