Black-Scholes Option Pricing Model
|
|
|
- Augustus Bell
- 8 years ago
- Views:
Transcription
1 Black-Scholes Option Pricing Model Nathan Coelen June 6, 22 1 Introduction Finance is one of the most rapidly changing and fastest growing areas in the corporate business world. Because of this rapid change, modern financial instruments have become extremely complex. New mathematical models are essential to implement and price these new financial instruments. The world of corporate finance once managed by business students is now controlled by mathematicians and computer scientists. In the early 197 s, Myron Scholes, Robert Merton, and Fisher Black made an important breakthrough in the pricing of complex financial instruments by developing what has become known as the Black-Scholes model. In 1997, the importance of their model was recognized world wide when Myron Scholes and Robert Merton received the Nobel Prize for Economics. Unfortunately, Fisher Black died in 1995, or he would have also received the award [Hull, 2]. The Black-Scholes model displayed the importance that mathematics plays in the field of finance. It also led to the growth and success of the new field of mathematical finance or financial engineering. In this paper, we will derive the Black-Scholes partial differential equation and ultimately solve the equation for a European call option. First, we will discuss basic financial terms, such as stock and option, and review the arbitrage pricing theory. We will then derive a model for the movement of a stock, which will include a random component, Brownian motion. Then, we will discuss some basic concepts of stochastic calculus that will be applied to our stock model. From this model, we will derive the Black-Scholes partial differential equation, and I will use boundary conditions for a European call option to solve the equation. 1
2 2 Definitions Financial assets are claims on some issuer, such as the federal government or a corporation, such as Microsoft. Financial assets also include real assets such as real estate, but we will be primarily concerned with common stock. Common stock represents an ownership in a corporation. Stocks provide a claim to the corporation s income and assets. A person who buys a financial asset in hopes that it will increase in value has taken a long position. A person who sells a stock before he/she owns it hoping that it decreases in value is said to be short an asset. People who take short positions borrow the asset from large financial institutions, sell the asset, and buy the asset back at a later time. A derivative is a financial instrument whose value depends on the value of other basic assets, such as common stock. In recent years, derivatives have become increasingly complex and important in the world of finance. Many individuals and corporations use derivatives to hedge against risk. The derivative asset we will be most interested in is a European call option. A call option gives the owner the right to buy the underlying asset on a certain date for a certain price. The specified price is known as the exercise or strike price and will be denoted by E. The specified date is known as the expiration date or day until maturity. European options can be exercised only on the expiration date itself. Another common option is a put option, which gives the owner the right to sell the underlying asset on a certain date for a certain price. For example, consider a July European call option contract on Microsoft with strike price $7. When the contract expires in July, if the price of Microsoft stock is $72 the owner will exercise the option and realize a profit of $2. He will buy the stock for $7 from the seller of the option and immediately sell the stock for $72. On the other hand, if a share of Microsoft is worth $69 the owner of the option will not exercise the option and it will expire worthless. In this case, the buyer would lose the purchase price of the option. 3 Arbitrage One of the most fundamental theories to the world of finance is the arbitrage pricing theory. The theory states that two otherwise identical assets cannot sell at different prices. This also means that there are no opportunities to 2
3 make an instantaneous risk-free profit. Here we are assuming the risk-free rate to be that of a bank account or a government bond, such as a Treasury bill. To illustrate the concept of arbitrage, consider a simple example of a stock that is traded in the U.S. and in London. In the U.S. the price of the stock is $15 and the asset sells for 1 in London, while the exchange rate is $1.6 per pound. A person could make an instantaneous profit by simultaneously buying 1 shares of stock in New York and selling them in London. An instantaneous profit of is realized without risk. 4 Hedging 1 (($1.6 1) $15) = $1 Three types of traders are attracted to derivative securities: speculators, arbitrageurs, and hedgers. Speculators take long or short positions in derivatives to increase their exposure to the market. They are betting that the underlying asset will go up or go down. Arbitrageurs find mispriced securities and instantaneously lock in a profit by adopting certain trading strategies like those discussed above. The last group is hedgers who take positions in derivative securities opposite those taken in the underlying asset in order to help manage risk. For example, consider an investor who owns 1 shares of Microsoft which is currently priced $62. The person is worried that the stock might decline sharply in the next two months. The person could buy put options on Microsoft to sell 1 shares at a price of $6. The person would pay the price of the options, but this would ensure that he could sell the stock for $6 at expiration if the stock declines sharply. One very important hedging strategy is delta hedging. The delta,, of the option is defined as the change of the option price with respect to the change in the price of the underlying asset. In other words, delta is the first derivative of the option price with respect to the stock price: = For example, suppose that the delta of a call option is.6, the price of a stock is $1 and the price of a call option is $1. Imagine an investor who 3
4 has sold 1 call option. The call option gives the buyer the right to buy 1 shares, since each option contract is for 1 shares. The seller s position could be hedged by buying.6 1 = 6 shares. The gain (loss) on the option position would then tend to be offset by the loss (gain) on the stock position. If the stock price goes up by $1 (producing a gain of $6 on the shares purchased) the option price would tend to go up by.6 $1 = $.6 (producing a loss of $.6 * 1 = $6 on the call option written)[hull, 2]. 5 Stock Price Model Most people agree that stock prices move randomly because of the efficient market hypothesis. There are different forms of this hypothesis, but all say the same two things. First, the history of the stock is fully reflected in the present price. Second, markets respond immediately to new information about the stock. With the previous two assumptions, changes in a stock price follow a Markov process. A Markov process is a stochastic process where only the present value of the variable is relevant for predicting the future. So, our stock model states that our predictions for the future price of the stock should be unaffected by the price one week, one month, or one year ago. As stated above, a Markov process is a stochastic process. In the real world, stock prices are restricted to discrete values, and changes in the stock price can only be realized during specified trading hours. Nevertheless, the continuous-variable, continuous-time model proves to more useful than a discrete model. Another important observation is to note that the absolute change in the price of a stock is by itself, not a useful quality. For example, an increase of one dollar in a stock is much more significant on a stock worth $1 than a stock worth $1. The relative change of the price of a stock is information that is more valuable. The relative change will be defined as the change in the price divided by the original price. Now consider the price of a stock S at time t. Consider a small time interval dt during which the the price of the underlying asset S changes by an amount ds. The most common model separates the return on the asset, ds/s into two parts. The first part is completely deterministic, and it is usually the risk free interest rate on a Treasury bill issued by the government. 4
5 This part yields a contribution of µdt to ds/s. Here µ is a measure of the average rate of growth of the stock, also known as the drift. In this model µ is assumed to be the risk free interest rate on a bond, but it can also be represented as a function of S and t. The second part of the model accounts for the random changes in the stock price due to external effects, such as unanticipated news. It is best modelled by a random sample drawn from a normal distribution with mean zero and contributes σdb to ds/s. In this formula σ is defined as the volatility of the stock, which measures the standard deviation of the returns. Like the term µ, σ can be represented as a function of S and t. The B in db denotes Brownian motion, which will be described in the next section. It is important to note that µ and σ can be estimated for individual stocks using statistical analysis of historical prices. This is not of interest for our model. It is only important that µ and σ are functions of S and t. Putting this information together, we obtain the stochastic differential equation ds/s = µdt + σdb (1) Notice that if the volatility is zero the model implies ds/s = µdt When µ is constant this equation can be solved so that S = S e µt (2) where S is the price of the stock at t and S is the price of the stock at t =. This equation shows that when the variance of an asset is zero, the asset grows at a continuously compunded rate of µ per unit of time. 5
6 6 Brownian Motion The term that has not been discussed so far is the random term, db. Brownian motion, which was originally used as a model for stock price movements in 19 by L. Bachelier[Klebaner, 1998], is a stochastic process B(t) characterized by the following three properties: 1. Normal Increments: B(t) B(s) has a normal distribution with mean and variance t s. Notice if s = that B(t) B() has normal distribution with mean and variance t. 2. Independence of Increments: B(t) B(s) is independent of the past. 3. Continuity of Paths: B(t), t > are continuous functions of t. These three properties alone define Brownian motion, but they also show why Brownian motion is used to model stock prices. Property 2 shows stock price changes will be independent of past price movements. This was an important assumption we made in our stock price model. An occurence of Brownian motion from time to T is called a path of the process on the interval [,T]. There are five important properties of Brownian motion paths. The path B(t), < t < T 1. is a continuous function of t, 2. is not monotonic on any interval, no matter how small the interval is, 3. is not differentiable at any point, 4. has infinite variation on any interval no matter how small it is, 5. has quadratic variation on [,t] equal to t, for any t. Together properties 1 and 3 state that although Brownian motion paths are continuous, the B(t) over interval t is much larger than t as t. Properties 4 and 5 show the distinction between functions of Brownian motion and normal, smooth functions. The variation of a function over the interval [a,b] is defined as n V g ([a, b]) = sup g(t i ) g(t i 1 ) i=1 6
7 where the supremum is taken over partitions: a = t < t 1 <... < t n = b. (3) It can be seen that smooth functions are of finite variation while Brownian motion is of infinite variation. Quadratic variation plays a very important role with Brownian motion and stochastic calculus. Quadratic variation is defined for a function g over the interval [,t] as n [g, g](t) = lim (g(t i ) g(t i 1 )) 2, i=1 where the limit is taken over partitions: = t < t 1 <... < t n = t. (4) Quadratic variation plays no role in standard calculus due to the fact that continuous functions of finite variation have quadratic variation of. 7 Stochastic Calculus In this section I will introduce stochastic integrals with respect to Brownian motion. These stochastic integrals are commonly called Itô integrals. In order to procede with the derivation of the Black-Scholes formula we need to define thestochastic integral X(t)dB(t). If X(t) is a constant, c, then cdb(t) = c(b(t ) B()). The integral over(, T ] should be the sum of integrals over subintervals [, a 1 ), (a 1, a 2 ), (a 2, a 3 ),..., (a n 1, T ]. So if X(t) takes values c i on each subinterval then the integral of X with respect to B is easily defined. First we consider the integrals of simple processes e(t) which depend on t and not on B(t). A simple deterministic process e(t) is a process for which there exist times = t < t 1 < t 2 <... < t n = T and constants c, c 1, c 2, c n 1, such that { c if t = e(t) = c i if t i < t t i+1, i =,..., n 1. Therefore, the Itô integral e(t)db(t) = X(t)dB(t) is defined as the sum n 1 i= c i (B(t i+1 ) B(t i )). 7
8 The Itô integral of simple processes is a random variable with the following four properties. 1. Linearity. If X(t) and Y (t) are simple processes and α and β are constants then (αx(t) + βy (t))db(t) = α X(t)dB(t) + β Y (t)db(t). 2. The integral of the indicator function of an interval I [a,b] (t) = 1 when t [a, b], and zero otherwise is just B(b) B(a), < a < b < T, 3. Zero mean property. E 4. Isometry Property. I [a,b] (t)db(t) = B(b) B(a). X(t)dB(t) =. ( 2 E X(t)dB(t)) = E(X 2 (t))dt. The definition of the Itô integral can be extended to processes X(t) that can be approximated by sequences e n of simple processes in the sense that as n. In that case, we define ( ) T E e n (t) X(t) 2 dt X(t)dB = n lim e n tdb. This is a sound definition beecause the limit does not depend on the approximating sequence. Also the integral that arises this way still satisfies properties 1-4 above. Now that we have defined the Itô integral of simple processes we wish to define Itô integral of other processes. It can be shown that if a general predictable process satifies certain conditions, the eneral process is a limit in probability of siple predictable processes we discussed earlier. The Itô integral of general predictable processes is defined as a limit of integrals of 8
9 simple processes. If X(t) is a predictable process such that X2 (t)dt < then the Itô integral X(t)dB(t) is defined and satisfies the above four properties. For example, we find the B(t)dB(t). We let = t < t 1 < t 2 <... < t n = T be a partition of [,T] then e n (t) = n 1 i= B(t i )I [ti,t i+1 ](t). Then for any n, e n (t) is a simple process. We can take a sequence of the partitions such that max i (t i+1 t i ) as n. The Itô integral of this simple function is given by e n (t)db(t) = n 1 i= B(t i )(B(t i+1 ) B(t i )). We now show that this sequence of integrals converges in probability and identify the limit. Adding and subtracting B 2 (t i+1 ), we obtain B(t i )(B(t i+1 ) B(t i )) = 1 2 and e n (t)db(t) = 1 2 n 1 i= ( B 2 (t i+1 ) B 2 (t i ) (B(t i+1 ) B(t i )) 2). ( B 2 (t i+1 ) B 2 (t i ) ) 1 2 = 1 2 B2 (T ) 1 2 B2 () 1 2 n 1 i= n 1 i= (B(t i+1 ) B(t i )) 2 (5) (B(t i+1 ) B(t i )) 2, since the first sum is a telescpopic sum. Notice that from property 5 of Brownian motion patht the second sum converges to the limit T. Therefore, en (t)db(t) converges, and the limit is B(t)dB(t) = lim e n (t)db(t) = 1 n 2 B2 (T ) 1 2 T. This example illustrates the difference between deterministic calculus and stochastic calculus. The quadratic variation of continuous functions, x(t), of finite variation we work with in standard calculus is. Therefore, if we were calculating the integral of x(t)dx(t) the same way as above the term 9
10 n 1 i= (x(t i+1 ) x(t i )) 2 would converge to the limit. So the x(t)dx(t) simply equals 1 2 x2 (T ). Now we need to discuss one of the main tools of stochastic calculus, Itô s formula, which is the stochastic counterpart of the chain rule in Calculus. Recall that Brownian motion has quadratic variation on [,t] equal to t, for any t. This can also be expressed as the following t (db(s)) 2 = t ds = t or in differential notation (db(t)) 2 = dt. Using this property and applying Taylor s formula, Itô s formula states that if f(x) is twice differentiable function then for any t f(b(t)) = f() + t f (B(s))dB(s) Note that Itô s formula in differential notation becomes t d(f(b(t)) = f B(t))dB(t) f (B(t))dt. f (B(s))ds. Next we define an Itô process. Let Y (t) be an Itô integral process Y (t) = t X(s)dB(s). An Itô process is an Itô integral plus an adapted continuous process of finite variation. Process Y is called an Itô process if for any t T it can be represented as Y (t) = Y () + t µ(s)ds + t σ(s)dbs. More generally, if Y is an Itô process represented above then it has a stochastic differential on [o, T ] dy (t) = µ(t)dt + σ(t)db(t) (6) for t T. The function µ is often called the drift coefficient and the function σ is called the diffusion coefficient. One last very important case for us to consider is for functions of the form f(x(t), t). If f(x, t) is a twice continuously differentiable in x, and 1
11 continuously differentiable in t and X(t) represnets an Itô process, then df(x(t), t) = f x f (X(t), t)dx(t) + (X(t), t)dt (7) t σ2 (X(t), t) 2 f (X(t), t)dt. x2 We will use this case of Itô s formula to compute the Black-Scholes partial differential equation in the next section. 8 Derivation of the Equation In the next two sections, the price of a derivative security, V (S, t) is derived. The model for a stock we derived in section 5 satisfies a Itô process defined in equation 6. Therefore, we let the function V (S, t) be twice differentiable in S and differentiable in t. Applying equation 7 from above we have dv (S, t) = ds + t dt σ2 S 2 2 V dt. (8) 2 Plugging into equation 8 for ds with Equation 1 we have dv (S, t) = This simplifies to (µsdt + σsdb) + t dt σ2 S 2 2 V 2 dt. dv (S, t) = σsdb + (µs + t σ2 S 2 2 V )dt. (9) 2 Now set up a portfolio long one option, V, and short an amount stock. Note from above that this portfolio is hedged. The value of this portfolio, π, is π = V S. (1) The change, dπ, in the value of this portfolio over a small time interval dt is given by dπ = dv ds. (11) 11
12 Now plugging equations 9 and 1 into equation 11 for dv and ds we get dπ = σsdb This simplifies to db + (µs + t σ2 S 2 2 V 2 )dt (µsdt + σsdb) (12) dπ = ( t σ2 S 2 2 V )dt. (13) 2 It is important to note that this portfolio is completely riskless because it does not contain the random Brownian motion term. Since this portfolio contains no risk it must earn the same as other short-term risk-free securities. If it earned more than this, arbitrageurs could make a profit by shorting the riskfree securities and using the proceeds to buy this portfolio. If the portfolio earned less arbitrageurs could make a riskless profit by shorting the portfolio and buying the risk-free securities. It follows for a riskless portfolio that dπ = rπdt (14) where r is the risk free interst rate. Substituting for dπ and π from equations 13 and 1 yields ( t σ2 S 2 2 V 2 )dt = r(v S )dt. (15) Further simplification yields the Black-Scholes differential equation t σ2 S 2 2 V 2 + rs rv =. (16) 9 Solution for a European Call In order to solve the Black-Scholes equation derived in the last section we need to consider final and boundary conditions, or else the partial differential equation does not have a unique solution. For this project we will concern ourselves with a European call, C(S, t) with exercise price E and expiry date T. 12
13 The final condition at time t = T can be derived from the definition of a call option. If at expiration S > E the call option will be worth S E because the buyer of the option can buy the stock for E and immediately sell it for S. If at expiration S < E the option will not be exercised and it will expire worthless. At t = T, the value of the option is known for certain to be the payoff C(S, T ) = max(s E, ). (17) This is the final condition for our differential equation. In order to find boundary conditions we consider the value of C when S = and as S. If S = then it is easy to see from equation 1 that ds =, and therefore, S will never change. If at expiry S = then from equation 17 the payoff must be. Consequently, when S = we have C(, T ) =. (18) Now when S it becomes more and more likely the option will be exercised and the payoff will be S E. The exercise price becomes less and less important as S, so the value of the option is equivalent to C(S, T ) S as S. (19) In order to solve the Black-Scholes equation we need to transform the equation into an equation we can work with. The first step is to get rid of the S and S 2 terms in equation 16. In order to do this consider the change of variables S = Ee x (2) t = T τ 1 2 σ2 (21) V = Ev(x, τ). (22) Using the Chain rule from Calculus for transforming partial derivatives for functions of two variables we have = x x + τ 13 τ (23)
14 t = x x t + τ Looking at equations 2, 21, and 22 it can be shown that τ t. (24) τ = 1 x σ2 = t 2 t x = 1 S τ = Plugging these into equation 23 and equation 24 yields = E S x (25) t = 1 2 σ2 E (26) 2 V 2 = E S 2 2 v x 2 E S 2 x. (27) Substituting equation 25, equation 26, and equation 27 into the Black- Scholes partial differential equation gives the differential equation where τ = 2 v x 2 + (k 1) x k = r 1 2 σ2. kv (28) The initial condition C(S, T ) = max(s E, ) is transformed into v(x, ) = max(e x 1, ). Now we apply another change of variable and let Then by simple differntiation we have v = e αx+βτ u(x, τ). (29) τ = βe αx+βτ αx+βτ u u + e τ 14
15 x = αeαx+βτ αx+βτ u u + e x 2 v x 2 = α(αe αx+βτ u + e αx+βτ u x Substituting these partials into equation 28 yields ) + αeαx+βτ u x + eαx+βτ 2 u x 2. βu + u τ = α2 u + 2α u x + 2 u u + (k 1)(αu + x2 x ) ku. We can get rid of the of the u terms and the u terms by carefully choosing x values of α and β such that and β = α 2 + (k 1)α k 2α + k 1 =. We can rearrange these equations so they can be written α = 1 2 (k 1) and β = 1 4 (k + 1)2. We now have the tranformation from v to u is v = e 1 2 (k 1)x 1 4 (k+1)2τ u(x, τ) resulting in the simple diffusion equation du dτ = d2 u for < x <, τ >. (3) dx2 Our initial condition has now been changed as well to u (x) = u(x, ) = max(e 1 2 (k+1)x e 1 2 (k 1)x, ). (31) The solution to the simple diffusion equation obtained above is well-known to be u(x, τ) = 1 2π 15 u (s)e (x s)2 4τ ds (32)
16 where u (x, ) is given by equation 31. In order to solve this integral it is very convenient to make a change of variable y = s x 2τ so that u(x, τ) = 1 2π u (y 2τ + x)e y2 2 dy. Substituting our intitial condition into this equation results in u(x, τ) = 1 2π 1 2π e 1 2 (k+1)(y x 2τ e 1 2 (k 1)(y x 2τ 2τ+x) e y2 2 dy 2τ+x) e y2 2 dy. In order to solve this we will solve each integral separately. The first integral can be solved by completing the square in the exponent. The exponent of the first integral is 1 2 y (k + 1)(x + y 2π). Factoring out the 1 2 gives us 1 2 (y2 [k + 1]y 2τ [k + 1]x). Separating out the term that is not a function of y, and adding and subtracting terms to set up a perfect square yields 1 2 (k + 1)x 1 y 2 [k + 1]y ( ) 2 ( ) 2 [k + 1] 2τ [k + 1] 2τ 2τ which can be written 1 2 (k + 1)x 1 ( y [k + 1] ) 2 2τ + 1 ( ) 2 [k + 1] 2τ
17 and simplified to 1 2 (k + 1)x 1 ( y [k + 1] ) 2 2τ + (k + 1)2 τ Thus the first integral reduces to Now substituting results in I 1 = e 1 2 (k+1)x 2π e 1 4 (k+1)2τ e 1 2(y 1 2 [k+1] 2τ) 2 dy. x 2τ z = y 1 2 [k + 1] 2τ I 1 = e 1 2 (k+1)x (k + 1)2 τ 2π = e 1 2 (k+1)x (k + 1)2 τ 2π x 1 2τ 2 (k+1) 2τ N(d1) e 1 2 z2 dz where and d 1 = x 2τ (k + 1) 2τ N(x) = 1 2π x e 1 2 y2 dy is the cumulative distribution function for the normal distribution. The calculation of the second integral I 2 is identical to that of I 1, except that (k-1) replaces (k+1) throughout. Finally, we work our way backwards with v(x, τ) = e 1 2 (k 1)x 1 4 (k+1)2τ u(x, τ) and then substituting the inverse transformations ( ) S x = log E 17
18 τ = 1 2 σ2 (T t) we finally obtain the desired result where and 1 Conclusion C = Ev(x, τ) C(S, t) = SN(d 1 ) Ee r(t t) N(d 2 ) d 1 = log( S E ) + (r σ2 )(T t) σ T t d 2 = log( S ) + (r 1 E 2 σ2 )(T t) σ. T t The important point to note about the derivation of the Black-Scholes differential equation is that we never specified a specific type of derivative security we were trying to find the price for until we set up boundary conditions for a European call. This means that a person can use the Black-Scholes differential equation to solve for the price of any type of option only by changing the boundary conditions. The Black-Scholes model truly revolutionized the world of finance. For the first time the model has given traders, hedgers, and investors a standard way to value options. The model also has also caused a huge growth in the importance of financial engineering in the world of finance. Today, mathematicians are building models to maximize portfolio returns while minimizing risk. They are also building sophisticated computer programs to search for inefficiencies in the market. The world of finance is becoming built on mathematics and the Black-Scholes model was the beginning of this mathematical revolution. 18
19 Bibliography [Baxter, 1996] Baxter, Martin and Andrew Rennie. Financial Calculus: An Introduction to Derivative Pricing. Cambridge, England: Cambridge University Press, [Hull, 2] Hull, John C. Options, Futures, and Other Derivatives. Upper Saddle River, New Jersey: Prentice Hall, 2. [Klebaner, 1998] Klebaner, Fima C. Introduction to Stochastic Calculus with Applications. London:Imperial College Press, [Ross, 1999] Ross, Sheldon. An Introduction to Mathematical Finance. Cambridge, England: Cambridge University Press, [Wilmott, 1995] Wilmott, Paul, Sam Howison, and Jeff Dewynne. The Mathematics of Financial Derivatives. Cambridge, England: Cambridge University Press,
Black-Scholes Equation for Option Pricing
 Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
Does Black-Scholes framework for Option Pricing use Constant Volatilities and Interest Rates? New Solution for a New Problem
 Does Black-Scholes framework for Option Pricing use Constant Volatilities and Interest Rates? New Solution for a New Problem Gagan Deep Singh Assistant Vice President Genpact Smart Decision Services Financial
Does Black-Scholes framework for Option Pricing use Constant Volatilities and Interest Rates? New Solution for a New Problem Gagan Deep Singh Assistant Vice President Genpact Smart Decision Services Financial
Finite Differences Schemes for Pricing of European and American Options
 Finite Differences Schemes for Pricing of European and American Options Margarida Mirador Fernandes IST Technical University of Lisbon Lisbon, Portugal November 009 Abstract Starting with the Black-Scholes
Finite Differences Schemes for Pricing of European and American Options Margarida Mirador Fernandes IST Technical University of Lisbon Lisbon, Portugal November 009 Abstract Starting with the Black-Scholes
第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model
 1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
Option pricing. Vinod Kothari
 Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
Moreover, under the risk neutral measure, it must be the case that (5) r t = µ t.
 LECTURE 7: BLACK SCHOLES THEORY 1. Introduction: The Black Scholes Model In 1973 Fisher Black and Myron Scholes ushered in the modern era of derivative securities with a seminal paper 1 on the pricing
LECTURE 7: BLACK SCHOLES THEORY 1. Introduction: The Black Scholes Model In 1973 Fisher Black and Myron Scholes ushered in the modern era of derivative securities with a seminal paper 1 on the pricing
The Black-Scholes Formula
 FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
CS 522 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options
 CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
Lecture 12: The Black-Scholes Model Steven Skiena. http://www.cs.sunysb.edu/ skiena
 Lecture 12: The Black-Scholes Model Steven Skiena Department of Computer Science State University of New York Stony Brook, NY 11794 4400 http://www.cs.sunysb.edu/ skiena The Black-Scholes-Merton Model
Lecture 12: The Black-Scholes Model Steven Skiena Department of Computer Science State University of New York Stony Brook, NY 11794 4400 http://www.cs.sunysb.edu/ skiena The Black-Scholes-Merton Model
How To Price A Call Option
 Now by Itô s formula But Mu f and u g in Ū. Hence τ θ u(x) =E( Mu(X) ds + u(x(τ θ))) 0 τ θ u(x) E( f(x) ds + g(x(τ θ))) = J x (θ). 0 But since u(x) =J x (θ ), we consequently have u(x) =J x (θ ) = min
Now by Itô s formula But Mu f and u g in Ū. Hence τ θ u(x) =E( Mu(X) ds + u(x(τ θ))) 0 τ θ u(x) E( f(x) ds + g(x(τ θ))) = J x (θ). 0 But since u(x) =J x (θ ), we consequently have u(x) =J x (θ ) = min
Call Price as a Function of the Stock Price
 Call Price as a Function of the Stock Price Intuitively, the call price should be an increasing function of the stock price. This relationship allows one to develop a theory of option pricing, derived
Call Price as a Function of the Stock Price Intuitively, the call price should be an increasing function of the stock price. This relationship allows one to develop a theory of option pricing, derived
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com
 Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Mathematical Finance
 Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
Lectures. Sergei Fedotov. 20912 - Introduction to Financial Mathematics. No tutorials in the first week
 Lectures Sergei Fedotov 20912 - Introduction to Financial Mathematics No tutorials in the first week Sergei Fedotov (University of Manchester) 20912 2010 1 / 1 Lecture 1 1 Introduction Elementary economics
Lectures Sergei Fedotov 20912 - Introduction to Financial Mathematics No tutorials in the first week Sergei Fedotov (University of Manchester) 20912 2010 1 / 1 Lecture 1 1 Introduction Elementary economics
The Black-Scholes-Merton Approach to Pricing Options
 he Black-Scholes-Merton Approach to Pricing Options Paul J Atzberger Comments should be sent to: atzberg@mathucsbedu Introduction In this article we shall discuss the Black-Scholes-Merton approach to determining
he Black-Scholes-Merton Approach to Pricing Options Paul J Atzberger Comments should be sent to: atzberg@mathucsbedu Introduction In this article we shall discuss the Black-Scholes-Merton approach to determining
FINANCIAL ECONOMICS OPTION PRICING
 OPTION PRICING Options are contingency contracts that specify payoffs if stock prices reach specified levels. A call option is the right to buy a stock at a specified price, X, called the strike price.
OPTION PRICING Options are contingency contracts that specify payoffs if stock prices reach specified levels. A call option is the right to buy a stock at a specified price, X, called the strike price.
Hedging. An Undergraduate Introduction to Financial Mathematics. J. Robert Buchanan. J. Robert Buchanan Hedging
 Hedging An Undergraduate Introduction to Financial Mathematics J. Robert Buchanan 2010 Introduction Definition Hedging is the practice of making a portfolio of investments less sensitive to changes in
Hedging An Undergraduate Introduction to Financial Mathematics J. Robert Buchanan 2010 Introduction Definition Hedging is the practice of making a portfolio of investments less sensitive to changes in
Review of Basic Options Concepts and Terminology
 Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
Alternative Price Processes for Black-Scholes: Empirical Evidence and Theory
 Alternative Price Processes for Black-Scholes: Empirical Evidence and Theory Samuel W. Malone April 19, 2002 This work is supported by NSF VIGRE grant number DMS-9983320. Page 1 of 44 1 Introduction This
Alternative Price Processes for Black-Scholes: Empirical Evidence and Theory Samuel W. Malone April 19, 2002 This work is supported by NSF VIGRE grant number DMS-9983320. Page 1 of 44 1 Introduction This
On Market-Making and Delta-Hedging
 On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
Monte Carlo Methods in Finance
 Author: Yiyang Yang Advisor: Pr. Xiaolin Li, Pr. Zari Rachev Department of Applied Mathematics and Statistics State University of New York at Stony Brook October 2, 2012 Outline Introduction 1 Introduction
Author: Yiyang Yang Advisor: Pr. Xiaolin Li, Pr. Zari Rachev Department of Applied Mathematics and Statistics State University of New York at Stony Brook October 2, 2012 Outline Introduction 1 Introduction
Lecture 6: Option Pricing Using a One-step Binomial Tree. Friday, September 14, 12
 Lecture 6: Option Pricing Using a One-step Binomial Tree An over-simplified model with surprisingly general extensions a single time step from 0 to T two types of traded securities: stock S and a bond
Lecture 6: Option Pricing Using a One-step Binomial Tree An over-simplified model with surprisingly general extensions a single time step from 0 to T two types of traded securities: stock S and a bond
Numerical Methods for Option Pricing
 Chapter 9 Numerical Methods for Option Pricing Equation (8.26) provides a way to evaluate option prices. For some simple options, such as the European call and put options, one can integrate (8.26) directly
Chapter 9 Numerical Methods for Option Pricing Equation (8.26) provides a way to evaluate option prices. For some simple options, such as the European call and put options, one can integrate (8.26) directly
QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS
 QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS L. M. Dieng ( Department of Physics, CUNY/BCC, New York, New York) Abstract: In this work, we expand the idea of Samuelson[3] and Shepp[,5,6] for
QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS L. M. Dieng ( Department of Physics, CUNY/BCC, New York, New York) Abstract: In this work, we expand the idea of Samuelson[3] and Shepp[,5,6] for
An Introduction to Exotic Options
 An Introduction to Exotic Options Jeff Casey Jeff Casey is entering his final semester of undergraduate studies at Ball State University. He is majoring in Financial Mathematics and has been a math tutor
An Introduction to Exotic Options Jeff Casey Jeff Casey is entering his final semester of undergraduate studies at Ball State University. He is majoring in Financial Mathematics and has been a math tutor
Chapter 11 Options. Main Issues. Introduction to Options. Use of Options. Properties of Option Prices. Valuation Models of Options.
 Chapter 11 Options Road Map Part A Introduction to finance. Part B Valuation of assets, given discount rates. Part C Determination of risk-adjusted discount rate. Part D Introduction to derivatives. Forwards
Chapter 11 Options Road Map Part A Introduction to finance. Part B Valuation of assets, given discount rates. Part C Determination of risk-adjusted discount rate. Part D Introduction to derivatives. Forwards
The Black-Scholes pricing formulas
 The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
1 The Black-Scholes Formula
 1 The Black-Scholes Formula In 1973 Fischer Black and Myron Scholes published a formula - the Black-Scholes formula - for computing the theoretical price of a European call option on a stock. Their paper,
1 The Black-Scholes Formula In 1973 Fischer Black and Myron Scholes published a formula - the Black-Scholes formula - for computing the theoretical price of a European call option on a stock. Their paper,
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price
 On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
Understanding Options and Their Role in Hedging via the Greeks
 Understanding Options and Their Role in Hedging via the Greeks Bradley J. Wogsland Department of Physics and Astronomy, University of Tennessee, Knoxville, TN 37996-1200 Options are priced assuming that
Understanding Options and Their Role in Hedging via the Greeks Bradley J. Wogsland Department of Physics and Astronomy, University of Tennessee, Knoxville, TN 37996-1200 Options are priced assuming that
1 IEOR 4700: Introduction to stochastic integration
 Copyright c 7 by Karl Sigman 1 IEOR 47: Introduction to stochastic integration 1.1 Riemann-Stieltjes integration Recall from calculus how the Riemann integral b a h(t)dt is defined for a continuous function
Copyright c 7 by Karl Sigman 1 IEOR 47: Introduction to stochastic integration 1.1 Riemann-Stieltjes integration Recall from calculus how the Riemann integral b a h(t)dt is defined for a continuous function
Binomial lattice model for stock prices
 Copyright c 2007 by Karl Sigman Binomial lattice model for stock prices Here we model the price of a stock in discrete time by a Markov chain of the recursive form S n+ S n Y n+, n 0, where the {Y i }
Copyright c 2007 by Karl Sigman Binomial lattice model for stock prices Here we model the price of a stock in discrete time by a Markov chain of the recursive form S n+ S n Y n+, n 0, where the {Y i }
Lecture 15. Sergei Fedotov. 20912 - Introduction to Financial Mathematics. Sergei Fedotov (University of Manchester) 20912 2010 1 / 6
 Lecture 15 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 6 Lecture 15 1 Black-Scholes Equation and Replicating Portfolio 2 Static
Lecture 15 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 6 Lecture 15 1 Black-Scholes Equation and Replicating Portfolio 2 Static
Call and Put. Options. American and European Options. Option Terminology. Payoffs of European Options. Different Types of Options
 Call and Put Options A call option gives its holder the right to purchase an asset for a specified price, called the strike price, on or before some specified expiration date. A put option gives its holder
Call and Put Options A call option gives its holder the right to purchase an asset for a specified price, called the strike price, on or before some specified expiration date. A put option gives its holder
Jorge Cruz Lopez - Bus 316: Derivative Securities. Week 11. The Black-Scholes Model: Hull, Ch. 13.
 Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
BINOMIAL OPTION PRICING
 Darden Graduate School of Business Administration University of Virginia BINOMIAL OPTION PRICING Binomial option pricing is a simple but powerful technique that can be used to solve many complex option-pricing
Darden Graduate School of Business Administration University of Virginia BINOMIAL OPTION PRICING Binomial option pricing is a simple but powerful technique that can be used to solve many complex option-pricing
Lecture Notes: Basic Concepts in Option Pricing - The Black and Scholes Model
 Brunel University Msc., EC5504, Financial Engineering Prof Menelaos Karanasos Lecture Notes: Basic Concepts in Option Pricing - The Black and Scholes Model Recall that the price of an option is equal to
Brunel University Msc., EC5504, Financial Engineering Prof Menelaos Karanasos Lecture Notes: Basic Concepts in Option Pricing - The Black and Scholes Model Recall that the price of an option is equal to
THE BLACK-SCHOLES MODEL AND EXTENSIONS
 THE BLAC-SCHOLES MODEL AND EXTENSIONS EVAN TURNER Abstract. This paper will derive the Black-Scholes pricing model of a European option by calculating the expected value of the option. We will assume that
THE BLAC-SCHOLES MODEL AND EXTENSIONS EVAN TURNER Abstract. This paper will derive the Black-Scholes pricing model of a European option by calculating the expected value of the option. We will assume that
Figure S9.1 Profit from long position in Problem 9.9
 Problem 9.9 Suppose that a European call option to buy a share for $100.00 costs $5.00 and is held until maturity. Under what circumstances will the holder of the option make a profit? Under what circumstances
Problem 9.9 Suppose that a European call option to buy a share for $100.00 costs $5.00 and is held until maturity. Under what circumstances will the holder of the option make a profit? Under what circumstances
Pricing Barrier Options under Local Volatility
 Abstract Pricing Barrier Options under Local Volatility Artur Sepp Mail: artursepp@hotmail.com, Web: www.hot.ee/seppar 16 November 2002 We study pricing under the local volatility. Our research is mainly
Abstract Pricing Barrier Options under Local Volatility Artur Sepp Mail: artursepp@hotmail.com, Web: www.hot.ee/seppar 16 November 2002 We study pricing under the local volatility. Our research is mainly
Notes on Black-Scholes Option Pricing Formula
 . Notes on Black-Scholes Option Pricing Formula by De-Xing Guan March 2006 These notes are a brief introduction to the Black-Scholes formula, which prices the European call options. The essential reading
. Notes on Black-Scholes Option Pricing Formula by De-Xing Guan March 2006 These notes are a brief introduction to the Black-Scholes formula, which prices the European call options. The essential reading
Valuation of American Options
 Valuation of American Options Among the seminal contributions to the mathematics of finance is the paper F. Black and M. Scholes, The pricing of options and corporate liabilities, Journal of Political
Valuation of American Options Among the seminal contributions to the mathematics of finance is the paper F. Black and M. Scholes, The pricing of options and corporate liabilities, Journal of Political
Lecture 1: Stochastic Volatility and Local Volatility
 Lecture 1: Stochastic Volatility and Local Volatility Jim Gatheral, Merrill Lynch Case Studies in Financial Modelling Course Notes, Courant Institute of Mathematical Sciences, Fall Term, 2002 Abstract
Lecture 1: Stochastic Volatility and Local Volatility Jim Gatheral, Merrill Lynch Case Studies in Financial Modelling Course Notes, Courant Institute of Mathematical Sciences, Fall Term, 2002 Abstract
Stochastic Processes and Advanced Mathematical Finance. Solution of the Black-Scholes Equation
 Steven R. Dunbar Department of Mathematics 03 Avery Hall University of Nebraska-Lincoln Lincoln, NE 68588-0130 http://www.math.unl.edu Voice: 40-47-3731 Fax: 40-47-8466 Stochastic Processes and Advanced
Steven R. Dunbar Department of Mathematics 03 Avery Hall University of Nebraska-Lincoln Lincoln, NE 68588-0130 http://www.math.unl.edu Voice: 40-47-3731 Fax: 40-47-8466 Stochastic Processes and Advanced
BINOMIAL OPTIONS PRICING MODEL. Mark Ioffe. Abstract
 BINOMIAL OPTIONS PRICING MODEL Mark Ioffe Abstract Binomial option pricing model is a widespread numerical method of calculating price of American options. In terms of applied mathematics this is simple
BINOMIAL OPTIONS PRICING MODEL Mark Ioffe Abstract Binomial option pricing model is a widespread numerical method of calculating price of American options. In terms of applied mathematics this is simple
Options Pricing. This is sometimes referred to as the intrinsic value of the option.
 Options Pricing We will use the example of a call option in discussing the pricing issue. Later, we will turn our attention to the Put-Call Parity Relationship. I. Preliminary Material Recall the payoff
Options Pricing We will use the example of a call option in discussing the pricing issue. Later, we will turn our attention to the Put-Call Parity Relationship. I. Preliminary Material Recall the payoff
Lecture 4: The Black-Scholes model
 OPTIONS and FUTURES Lecture 4: The Black-Scholes model Philip H. Dybvig Washington University in Saint Louis Black-Scholes option pricing model Lognormal price process Call price Put price Using Black-Scholes
OPTIONS and FUTURES Lecture 4: The Black-Scholes model Philip H. Dybvig Washington University in Saint Louis Black-Scholes option pricing model Lognormal price process Call price Put price Using Black-Scholes
Jung-Soon Hyun and Young-Hee Kim
 J. Korean Math. Soc. 43 (2006), No. 4, pp. 845 858 TWO APPROACHES FOR STOCHASTIC INTEREST RATE OPTION MODEL Jung-Soon Hyun and Young-Hee Kim Abstract. We present two approaches of the stochastic interest
J. Korean Math. Soc. 43 (2006), No. 4, pp. 845 858 TWO APPROACHES FOR STOCHASTIC INTEREST RATE OPTION MODEL Jung-Soon Hyun and Young-Hee Kim Abstract. We present two approaches of the stochastic interest
The Intuition Behind Option Valuation: A Teaching Note
 The Intuition Behind Option Valuation: A Teaching Note Thomas Grossman Haskayne School of Business University of Calgary Steve Powell Tuck School of Business Dartmouth College Kent L Womack Tuck School
The Intuition Behind Option Valuation: A Teaching Note Thomas Grossman Haskayne School of Business University of Calgary Steve Powell Tuck School of Business Dartmouth College Kent L Womack Tuck School
Pricing of an Exotic Forward Contract
 Pricing of an Exotic Forward Contract Jirô Akahori, Yuji Hishida and Maho Nishida Dept. of Mathematical Sciences, Ritsumeikan University 1-1-1 Nojihigashi, Kusatsu, Shiga 525-8577, Japan E-mail: {akahori,
Pricing of an Exotic Forward Contract Jirô Akahori, Yuji Hishida and Maho Nishida Dept. of Mathematical Sciences, Ritsumeikan University 1-1-1 Nojihigashi, Kusatsu, Shiga 525-8577, Japan E-mail: {akahori,
Options: Valuation and (No) Arbitrage
 Prof. Alex Shapiro Lecture Notes 15 Options: Valuation and (No) Arbitrage I. Readings and Suggested Practice Problems II. Introduction: Objectives and Notation III. No Arbitrage Pricing Bound IV. The Binomial
Prof. Alex Shapiro Lecture Notes 15 Options: Valuation and (No) Arbitrage I. Readings and Suggested Practice Problems II. Introduction: Objectives and Notation III. No Arbitrage Pricing Bound IV. The Binomial
A SNOWBALL CURRENCY OPTION
 J. KSIAM Vol.15, No.1, 31 41, 011 A SNOWBALL CURRENCY OPTION GYOOCHEOL SHIM 1 1 GRADUATE DEPARTMENT OF FINANCIAL ENGINEERING, AJOU UNIVERSITY, SOUTH KOREA E-mail address: gshim@ajou.ac.kr ABSTRACT. I introduce
J. KSIAM Vol.15, No.1, 31 41, 011 A SNOWBALL CURRENCY OPTION GYOOCHEOL SHIM 1 1 GRADUATE DEPARTMENT OF FINANCIAL ENGINEERING, AJOU UNIVERSITY, SOUTH KOREA E-mail address: gshim@ajou.ac.kr ABSTRACT. I introduce
CHAPTER 21: OPTION VALUATION
 CHAPTER 21: OPTION VALUATION PROBLEM SETS 1. The value of a put option also increases with the volatility of the stock. We see this from the put-call parity theorem as follows: P = C S + PV(X) + PV(Dividends)
CHAPTER 21: OPTION VALUATION PROBLEM SETS 1. The value of a put option also increases with the volatility of the stock. We see this from the put-call parity theorem as follows: P = C S + PV(X) + PV(Dividends)
Risk/Arbitrage Strategies: An Application to Stock Option Portfolio Management
 Risk/Arbitrage Strategies: An Application to Stock Option Portfolio Management Vincenzo Bochicchio, Niklaus Bühlmann, Stephane Junod and Hans-Fredo List Swiss Reinsurance Company Mythenquai 50/60, CH-8022
Risk/Arbitrage Strategies: An Application to Stock Option Portfolio Management Vincenzo Bochicchio, Niklaus Bühlmann, Stephane Junod and Hans-Fredo List Swiss Reinsurance Company Mythenquai 50/60, CH-8022
CHAPTER 21: OPTION VALUATION
 CHAPTER 21: OPTION VALUATION 1. Put values also must increase as the volatility of the underlying stock increases. We see this from the parity relation as follows: P = C + PV(X) S 0 + PV(Dividends). Given
CHAPTER 21: OPTION VALUATION 1. Put values also must increase as the volatility of the underlying stock increases. We see this from the parity relation as follows: P = C + PV(X) S 0 + PV(Dividends). Given
Merton-Black-Scholes model for option pricing. Peter Denteneer. 22 oktober 2009
 Merton-Black-Scholes model for option pricing Instituut{Lorentz voor Theoretische Natuurkunde, LION, Universiteit Leiden 22 oktober 2009 With inspiration from: J. Tinbergen, T.C. Koopmans, E. Majorana,
Merton-Black-Scholes model for option pricing Instituut{Lorentz voor Theoretische Natuurkunde, LION, Universiteit Leiden 22 oktober 2009 With inspiration from: J. Tinbergen, T.C. Koopmans, E. Majorana,
A Genetic Algorithm to Price an European Put Option Using the Geometric Mean Reverting Model
 Applied Mathematical Sciences, vol 8, 14, no 143, 715-7135 HIKARI Ltd, wwwm-hikaricom http://dxdoiorg/11988/ams144644 A Genetic Algorithm to Price an European Put Option Using the Geometric Mean Reverting
Applied Mathematical Sciences, vol 8, 14, no 143, 715-7135 HIKARI Ltd, wwwm-hikaricom http://dxdoiorg/11988/ams144644 A Genetic Algorithm to Price an European Put Option Using the Geometric Mean Reverting
Research on Option Trading Strategies
 Research on Option Trading Strategies An Interactive Qualifying Project Report: Submitted to the Faculty of the WORCESTER POLYTECHNIC INSTITUTE In partial fulfillment of the requirements for the Degree
Research on Option Trading Strategies An Interactive Qualifying Project Report: Submitted to the Faculty of the WORCESTER POLYTECHNIC INSTITUTE In partial fulfillment of the requirements for the Degree
EXP 481 -- Capital Markets Option Pricing. Options: Definitions. Arbitrage Restrictions on Call Prices. Arbitrage Restrictions on Call Prices 1) C > 0
 EXP 481 -- Capital Markets Option Pricing imple arbitrage relations Payoffs to call options Black-choles model Put-Call Parity Implied Volatility Options: Definitions A call option gives the buyer the
EXP 481 -- Capital Markets Option Pricing imple arbitrage relations Payoffs to call options Black-choles model Put-Call Parity Implied Volatility Options: Definitions A call option gives the buyer the
Valuing Stock Options: The Black-Scholes-Merton Model. Chapter 13
 Valuing Stock Options: The Black-Scholes-Merton Model Chapter 13 Fundamentals of Futures and Options Markets, 8th Ed, Ch 13, Copyright John C. Hull 2013 1 The Black-Scholes-Merton Random Walk Assumption
Valuing Stock Options: The Black-Scholes-Merton Model Chapter 13 Fundamentals of Futures and Options Markets, 8th Ed, Ch 13, Copyright John C. Hull 2013 1 The Black-Scholes-Merton Random Walk Assumption
Hedging of Financial Derivatives and Portfolio Insurance
 Hedging of Financial Derivatives and Portfolio Insurance Gasper Godson Mwanga African Institute for Mathematical Sciences 6, Melrose Road, 7945 Muizenberg, Cape Town South Africa. e-mail: gasper@aims.ac.za,
Hedging of Financial Derivatives and Portfolio Insurance Gasper Godson Mwanga African Institute for Mathematical Sciences 6, Melrose Road, 7945 Muizenberg, Cape Town South Africa. e-mail: gasper@aims.ac.za,
The Behavior of Bonds and Interest Rates. An Impossible Bond Pricing Model. 780 w Interest Rate Models
 780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
EC3070 FINANCIAL DERIVATIVES
 BINOMIAL OPTION PRICING MODEL A One-Step Binomial Model The Binomial Option Pricing Model is a simple device that is used for determining the price c τ 0 that should be attributed initially to a call option
BINOMIAL OPTION PRICING MODEL A One-Step Binomial Model The Binomial Option Pricing Model is a simple device that is used for determining the price c τ 0 that should be attributed initially to a call option
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies
 Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Chapter 8 Financial Options and Applications in Corporate Finance ANSWERS TO END-OF-CHAPTER QUESTIONS
 Chapter 8 Financial Options and Applications in Corporate Finance ANSWERS TO END-OF-CHAPTER QUESTIONS 8-1 a. An option is a contract which gives its holder the right to buy or sell an asset at some predetermined
Chapter 8 Financial Options and Applications in Corporate Finance ANSWERS TO END-OF-CHAPTER QUESTIONS 8-1 a. An option is a contract which gives its holder the right to buy or sell an asset at some predetermined
14 Greeks Letters and Hedging
 ECG590I Asset Pricing. Lecture 14: Greeks Letters and Hedging 1 14 Greeks Letters and Hedging 14.1 Illustration We consider the following example through out this section. A financial institution sold
ECG590I Asset Pricing. Lecture 14: Greeks Letters and Hedging 1 14 Greeks Letters and Hedging 14.1 Illustration We consider the following example through out this section. A financial institution sold
CHAPTER 15. Option Valuation
 CHAPTER 15 Option Valuation Just what is an option worth? Actually, this is one of the more difficult questions in finance. Option valuation is an esoteric area of finance since it often involves complex
CHAPTER 15 Option Valuation Just what is an option worth? Actually, this is one of the more difficult questions in finance. Option valuation is an esoteric area of finance since it often involves complex
INTRODUCTION TO OPTIONS MARKETS QUESTIONS
 INTRODUCTION TO OPTIONS MARKETS QUESTIONS 1. What is the difference between a put option and a call option? 2. What is the difference between an American option and a European option? 3. Why does an option
INTRODUCTION TO OPTIONS MARKETS QUESTIONS 1. What is the difference between a put option and a call option? 2. What is the difference between an American option and a European option? 3. Why does an option
1 The Black-Scholes model: extensions and hedging
 1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
Pricing Barrier Option Using Finite Difference Method and MonteCarlo Simulation
 Pricing Barrier Option Using Finite Difference Method and MonteCarlo Simulation Yoon W. Kwon CIMS 1, Math. Finance Suzanne A. Lewis CIMS, Math. Finance May 9, 000 1 Courant Institue of Mathematical Science,
Pricing Barrier Option Using Finite Difference Method and MonteCarlo Simulation Yoon W. Kwon CIMS 1, Math. Finance Suzanne A. Lewis CIMS, Math. Finance May 9, 000 1 Courant Institue of Mathematical Science,
Finance 400 A. Penati - G. Pennacchi. Option Pricing
 Finance 400 A. Penati - G. Pennacchi Option Pricing Earlier we derived general pricing relationships for contingent claims in terms of an equilibrium stochastic discount factor or in terms of elementary
Finance 400 A. Penati - G. Pennacchi Option Pricing Earlier we derived general pricing relationships for contingent claims in terms of an equilibrium stochastic discount factor or in terms of elementary
Fundamentals of Futures and Options (a summary)
 Fundamentals of Futures and Options (a summary) Roger G. Clarke, Harindra de Silva, CFA, and Steven Thorley, CFA Published 2013 by the Research Foundation of CFA Institute Summary prepared by Roger G.
Fundamentals of Futures and Options (a summary) Roger G. Clarke, Harindra de Silva, CFA, and Steven Thorley, CFA Published 2013 by the Research Foundation of CFA Institute Summary prepared by Roger G.
Pricing Options with Discrete Dividends by High Order Finite Differences and Grid Stretching
 Pricing Options with Discrete Dividends by High Order Finite Differences and Grid Stretching Kees Oosterlee Numerical analysis group, Delft University of Technology Joint work with Coen Leentvaar, Ariel
Pricing Options with Discrete Dividends by High Order Finite Differences and Grid Stretching Kees Oosterlee Numerical analysis group, Delft University of Technology Joint work with Coen Leentvaar, Ariel
Pricing American Options without Expiry Date
 Pricing American Options without Expiry Date Carisa K. W. Yu Department of Applied Mathematics The Hong Kong Polytechnic University Hung Hom, Hong Kong E-mail: carisa.yu@polyu.edu.hk Abstract This paper
Pricing American Options without Expiry Date Carisa K. W. Yu Department of Applied Mathematics The Hong Kong Polytechnic University Hung Hom, Hong Kong E-mail: carisa.yu@polyu.edu.hk Abstract This paper
11 Option. Payoffs and Option Strategies. Answers to Questions and Problems
 11 Option Payoffs and Option Strategies Answers to Questions and Problems 1. Consider a call option with an exercise price of $80 and a cost of $5. Graph the profits and losses at expiration for various
11 Option Payoffs and Option Strategies Answers to Questions and Problems 1. Consider a call option with an exercise price of $80 and a cost of $5. Graph the profits and losses at expiration for various
ARBITRAGE-FREE OPTION PRICING MODELS. Denis Bell. University of North Florida
 ARBITRAGE-FREE OPTION PRICING MODELS Denis Bell University of North Florida Modelling Stock Prices Example American Express In mathematical finance, it is customary to model a stock price by an (Ito) stochatic
ARBITRAGE-FREE OPTION PRICING MODELS Denis Bell University of North Florida Modelling Stock Prices Example American Express In mathematical finance, it is customary to model a stock price by an (Ito) stochatic
The interest volatility surface
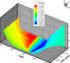 The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
Asian Option Pricing Formula for Uncertain Financial Market
 Sun and Chen Journal of Uncertainty Analysis and Applications (215) 3:11 DOI 1.1186/s4467-15-35-7 RESEARCH Open Access Asian Option Pricing Formula for Uncertain Financial Market Jiajun Sun 1 and Xiaowei
Sun and Chen Journal of Uncertainty Analysis and Applications (215) 3:11 DOI 1.1186/s4467-15-35-7 RESEARCH Open Access Asian Option Pricing Formula for Uncertain Financial Market Jiajun Sun 1 and Xiaowei
Lecture 11: The Greeks and Risk Management
 Lecture 11: The Greeks and Risk Management This lecture studies market risk management from the perspective of an options trader. First, we show how to describe the risk characteristics of derivatives.
Lecture 11: The Greeks and Risk Management This lecture studies market risk management from the perspective of an options trader. First, we show how to describe the risk characteristics of derivatives.
The Binomial Option Pricing Model André Farber
 1 Solvay Business School Université Libre de Bruxelles The Binomial Option Pricing Model André Farber January 2002 Consider a non-dividend paying stock whose price is initially S 0. Divide time into small
1 Solvay Business School Université Libre de Bruxelles The Binomial Option Pricing Model André Farber January 2002 Consider a non-dividend paying stock whose price is initially S 0. Divide time into small
LECTURE 15: AMERICAN OPTIONS
 LECTURE 15: AMERICAN OPTIONS 1. Introduction All of the options that we have considered thus far have been of the European variety: exercise is permitted only at the termination of the contract. These
LECTURE 15: AMERICAN OPTIONS 1. Introduction All of the options that we have considered thus far have been of the European variety: exercise is permitted only at the termination of the contract. These
Derivative Users Traders of derivatives can be categorized as hedgers, speculators, or arbitrageurs.
 OPTIONS THEORY Introduction The Financial Manager must be knowledgeable about derivatives in order to manage the price risk inherent in financial transactions. Price risk refers to the possibility of loss
OPTIONS THEORY Introduction The Financial Manager must be knowledgeable about derivatives in order to manage the price risk inherent in financial transactions. Price risk refers to the possibility of loss
An Introduction to Modeling Stock Price Returns With a View Towards Option Pricing
 An Introduction to Modeling Stock Price Returns With a View Towards Option Pricing Kyle Chauvin August 21, 2006 This work is the product of a summer research project at the University of Kansas, conducted
An Introduction to Modeling Stock Price Returns With a View Towards Option Pricing Kyle Chauvin August 21, 2006 This work is the product of a summer research project at the University of Kansas, conducted
GAMMA.0279 THETA 8.9173 VEGA 9.9144 RHO 3.5985
 14 Option Sensitivities and Option Hedging Answers to Questions and Problems 1. Consider Call A, with: X $70; r 0.06; T t 90 days; 0.4; and S $60. Compute the price, DELTA, GAMMA, THETA, VEGA, and RHO
14 Option Sensitivities and Option Hedging Answers to Questions and Problems 1. Consider Call A, with: X $70; r 0.06; T t 90 days; 0.4; and S $60. Compute the price, DELTA, GAMMA, THETA, VEGA, and RHO
Exam MFE Spring 2007 FINAL ANSWER KEY 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D
 Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
where N is the standard normal distribution function,
 The Black-Scholes-Merton formula (Hull 13.5 13.8) Assume S t is a geometric Brownian motion w/drift. Want market value at t = 0 of call option. European call option with expiration at time T. Payout at
The Black-Scholes-Merton formula (Hull 13.5 13.8) Assume S t is a geometric Brownian motion w/drift. Want market value at t = 0 of call option. European call option with expiration at time T. Payout at
Other variables as arguments besides S. Want those other variables to be observables.
 Valuation of options before expiration Need to distinguish between American and European options. Consider European options with time t until expiration. Value now of receiving c T at expiration? (Value
Valuation of options before expiration Need to distinguish between American and European options. Consider European options with time t until expiration. Value now of receiving c T at expiration? (Value
Hedging Options In The Incomplete Market With Stochastic Volatility. Rituparna Sen Sunday, Nov 15
 Hedging Options In The Incomplete Market With Stochastic Volatility Rituparna Sen Sunday, Nov 15 1. Motivation This is a pure jump model and hence avoids the theoretical drawbacks of continuous path models.
Hedging Options In The Incomplete Market With Stochastic Volatility Rituparna Sen Sunday, Nov 15 1. Motivation This is a pure jump model and hence avoids the theoretical drawbacks of continuous path models.
Option Valuation. Chapter 21
 Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Stochastic Processes and Advanced Mathematical Finance. Multiperiod Binomial Tree Models
 Steven R. Dunbar Department of Mathematics 203 Avery Hall University of Nebraska-Lincoln Lincoln, NE 68588-0130 http://www.math.unl.edu Voice: 402-472-3731 Fax: 402-472-8466 Stochastic Processes and Advanced
Steven R. Dunbar Department of Mathematics 203 Avery Hall University of Nebraska-Lincoln Lincoln, NE 68588-0130 http://www.math.unl.edu Voice: 402-472-3731 Fax: 402-472-8466 Stochastic Processes and Advanced
Chapter 2: Binomial Methods and the Black-Scholes Formula
 Chapter 2: Binomial Methods and the Black-Scholes Formula 2.1 Binomial Trees We consider a financial market consisting of a bond B t = B(t), a stock S t = S(t), and a call-option C t = C(t), where the
Chapter 2: Binomial Methods and the Black-Scholes Formula 2.1 Binomial Trees We consider a financial market consisting of a bond B t = B(t), a stock S t = S(t), and a call-option C t = C(t), where the
Hedging Barriers. Liuren Wu. Zicklin School of Business, Baruch College (http://faculty.baruch.cuny.edu/lwu/)
 Hedging Barriers Liuren Wu Zicklin School of Business, Baruch College (http://faculty.baruch.cuny.edu/lwu/) Based on joint work with Peter Carr (Bloomberg) Modeling and Hedging Using FX Options, March
Hedging Barriers Liuren Wu Zicklin School of Business, Baruch College (http://faculty.baruch.cuny.edu/lwu/) Based on joint work with Peter Carr (Bloomberg) Modeling and Hedging Using FX Options, March
Options pricing in discrete systems
 UNIVERZA V LJUBLJANI, FAKULTETA ZA MATEMATIKO IN FIZIKO Options pricing in discrete systems Seminar II Mentor: prof. Dr. Mihael Perman Author: Gorazd Gotovac //2008 Abstract This paper is a basic introduction
UNIVERZA V LJUBLJANI, FAKULTETA ZA MATEMATIKO IN FIZIKO Options pricing in discrete systems Seminar II Mentor: prof. Dr. Mihael Perman Author: Gorazd Gotovac //2008 Abstract This paper is a basic introduction
Option Premium = Intrinsic. Speculative Value. Value
 Chapters 4/ Part Options: Basic Concepts Options Call Options Put Options Selling Options Reading The Wall Street Journal Combinations of Options Valuing Options An Option-Pricing Formula Investment in
Chapters 4/ Part Options: Basic Concepts Options Call Options Put Options Selling Options Reading The Wall Street Journal Combinations of Options Valuing Options An Option-Pricing Formula Investment in
American and European. Put Option
 American and European Put Option Analytical Finance I Kinda Sumlaji 1 Table of Contents: 1. Introduction... 3 2. Option Style... 4 3. Put Option 4 3.1 Definition 4 3.2 Payoff at Maturity... 4 3.3 Example
American and European Put Option Analytical Finance I Kinda Sumlaji 1 Table of Contents: 1. Introduction... 3 2. Option Style... 4 3. Put Option 4 3.1 Definition 4 3.2 Payoff at Maturity... 4 3.3 Example
Black Scholes Merton Approach To Modelling Financial Derivatives Prices Tomas Sinkariovas 0802869. Words: 3441
 Black Scholes Merton Approach To Modelling Financial Derivatives Prices Tomas Sinkariovas 0802869 Words: 3441 1 1. Introduction In this paper I present Black, Scholes (1973) and Merton (1973) (BSM) general
Black Scholes Merton Approach To Modelling Financial Derivatives Prices Tomas Sinkariovas 0802869 Words: 3441 1 1. Introduction In this paper I present Black, Scholes (1973) and Merton (1973) (BSM) general
Chapter 5 Financial Forwards and Futures
 Chapter 5 Financial Forwards and Futures Question 5.1. Four different ways to sell a share of stock that has a price S(0) at time 0. Question 5.2. Description Get Paid at Lose Ownership of Receive Payment
Chapter 5 Financial Forwards and Futures Question 5.1. Four different ways to sell a share of stock that has a price S(0) at time 0. Question 5.2. Description Get Paid at Lose Ownership of Receive Payment
Example 1. Consider the following two portfolios: 2. Buy one c(s(t), 20, τ, r) and sell one c(s(t), 10, τ, r).
 Chapter 4 Put-Call Parity 1 Bull and Bear Financial analysts use words such as bull and bear to describe the trend in stock markets. Generally speaking, a bull market is characterized by rising prices.
Chapter 4 Put-Call Parity 1 Bull and Bear Financial analysts use words such as bull and bear to describe the trend in stock markets. Generally speaking, a bull market is characterized by rising prices.
CHAPTER 5 American Options
 CHAPTER 5 American Options The distinctive feature of an American option is its early exercise privilege, that is, the holder can exercise the option prior to the date of expiration. Since the additional
CHAPTER 5 American Options The distinctive feature of an American option is its early exercise privilege, that is, the holder can exercise the option prior to the date of expiration. Since the additional
