Moreover, under the risk neutral measure, it must be the case that (5) r t = µ t.
|
|
|
- Philomena Hudson
- 7 years ago
- Views:
Transcription
1 LECTURE 7: BLACK SCHOLES THEORY 1. Introduction: The Black Scholes Model In 1973 Fisher Black and Myron Scholes ushered in the modern era of derivative securities with a seminal paper 1 on the pricing and hedging of European call and put options. In this paper the famous Black-Scholes formula made its debut, and the Itô calculus was unleashed upon the world of finance. 2 In this lecture we shall explain the Black-Scholes argument in its original setting, the pricing and hedging of European contingent claims. In subsequent lectures, we will see how to use the Black Scholes model in conjunction with the Itô calculus to price and hedge all manner of exotic derivative securities. In its simplest form, the Black Scholes Merton model involves only two underlying assets, a riskless asset Cash Bond and a risky asset Stock. 3 The asset Cash Bond appreciates at the short rate, or riskless rate of return r t, which at least for now is assumed to be nonrandom, although possibly time varying. Thus, the price B t of the Cash Bond at time t is assumed to satisfy the differential equation db t 1 = r t B t, dt whose unique solution for the value B = 1 is as the reader will now check 2 B t = exp r s ds. The share price S t of the risky asset Stock at time t is assumed to follow a stochastic differential equation SDE of the form 3 ds t = µ t S t dt + σs t dw t, where {W t } t is a standard Brownian motion, µ t is a nonrandom but not necessarily constant function of t, and σ > is a constant called the volatility of the Stock. Proposition 1. If the drift coefficient function µ t is bounded, then the SDE 3 has a unique solution with initial condition S, and it is given by 4 S t = S exp σw t σ 2 t/2 + µ s ds Moreover, under the risk neutral measure, it must be the case that 5 r t = µ t. 1 The pricing of options and corporate liabilities in Journal of Political Economy, volume 81, pages In fact, the use of the Wiener process in financial models dates back to the early years of the 2th century, in Bachelier s thesis. However, the success of the Black-Scholes model assured that the Itô calculus would have a permanent place in the world of mathematical finance. 3 Keep in mind that the designation of a riskless asset is somewhat arbitrary. Recall that any tradeable asset whose value at the termination time T is strictly positive may be used as numeraire, in which case it becomes the riskless asset, with rate of return r =. This is the principle of numeraire invariance. Note, however, that the equilibrium measure depends on the choice of riskless asset. More on this when we talk about the Cameron Martin Girsanov theorem later in the course. 1
2 Proof. As in many arguments to follow, the magical incantation is Itô s formula. Consider first the formula 4 for the share price of Stock; to see that this defines a solution to the SDE 3, apply Itô s formula to the function ux, t = exp σx σ 2 t/2 + µ s ds. To see that the solution is unique, check your favorite reference on the theory of SDEs. Finally, to see that the drift coefficient µ t must coincide with the riskless rate of return r t in the risk neutral world, recall that under the risk neutral measure the discounted share price of Stock must be a martingale. The appropriate discount factor is B t, so the discounted share price of Stock is St = S t /B t = S exp σw t σ 2 t/2 + µ s r s ds. Applying Itô s formula once more, one finds that ds t = σs t dw t + S t µ t r t dt. In order that S t be a martingale, it is necessary that the dt term be ; this implies that µ t = r t. Corollary 1. Under the risk neutral measure, the log of the discounted stock price at time t is normally distributed with mean log S σ 2 t/2 and variance σ 2 T. 2. The Black Scholes Formula for the Price of a European Call Option Recall that a European Call on the asset Stock with strike K and expiration date T is a contract that allows the owner to purchase one share of Stock at price K at time T. Thus, the value of the Call at time T is S T K +. According to the Fundamental Theorem of Arbitrage Pricing, 4 the price of the asset Call at time t = must be the discounted expectation, under the risk neutral measure, of the value at time t = T, which, by Proposition 1, is 6 CS, = CS, ; K, T = ES T K/B T + where ST has the distribution specified in Corollary 1. A routine calculation, using integration by parts, shows that Cx, T ; K may be rewritten as 7 Cx, ; K, T = xφz K B T Φz σ T, where z = logxb t/k + σ 2 t/2 σ T and Φ is the cumulative normal distribution function. And that s all there is to the Black Scholes formula! There are other derivations, some with pedagogical merit, others just long and painful, most based somehow on the Itô formula. For the cultural enlightenment of the reader, we shall present another argument, based on PDE theory, in the next section. Before we leave this derivation, though, we should observe that it works essentially unchanged for all European style derivative securities. Let F : R R be a function of polynomial 4 We only proved this in the case of discrete time, finite scenario markets. However, as we mentioned at the time, the theorem is true in much greater generality, and the essential idea of the proof remains the same.
3 growth, and consider the derivative security whose value at t = T is F S T. Then the value at time t = of this derivative security is 8 V = EB 1 T F B T S T, where S T has the lognormal distribution specified in Corollary 1 above. 3. The Black Scholes PDE Next, another derivation of the Black Scholes formula. This one proceeds by finding a PDE for the price function Cx, T = Cx, T ; K and then verifying that the function defined by 7 solves the PDE. It has the disadvantage that the issues of uniqueness and smoothness of solutions to the PDE must be tackled separately and we won t do this here. For the sake of simplicity, we shall only consider the case where the short rate is constant, that is, r t r. Let CS t, t be the price of the Call at time t when the share price of Stock is S t. By the Fundamental Theorem of Arbitrage Pricing, the discounted Call price must be a martingale under the risk neutral measure. Now the Itô formula 5 implies that the Call price must satisfy the SDE B t dbt 1 CS t, t = C x S t, t ds t C xxs t, tσ 2 S 2 t dt + C t S t, t dt r t CS t, t dt = C x S t, tσs t dw t + {C x S t, tr t S t + σ2 St C xxs t, t + C t S t, t r t CS t, t} dt. Here C x, C xx, C t, etc. represent partial derivatives of the call price function Cx, t with respect to the indicated variables. Multiplying by the discount factor Bt 1, one sees that the discounted Call price can be a martingale only if the dt term on the right side vanishes. Thus, the call price function Cx, t must satisfy the Black Scholes PDE: 1 r t Cx, t + C t x, t + r t xc x x, t + σ2 x 2 with the terminal condition 11 Cx, T = x K +. 2 C xxx, t = It may now be verified by differentiation that the function defined by the Black Scholes formula 7 solves the Black Scholes PDE 1, and converges to the terminal value as t T. This isn t an especially enlightening way to spend one s time. What might make a nice Exercise, though, is to check that 7 solves the Black Scholes PDE 1 by using a computer package that does symbolic differentiation. Observe that nowhere in this argument did we use the specific form of the terminal payoff, except in determining the terminal condition 11. Thus, the argument applies, unchanged, to the price function Cx, t for any derivative security whose terminal payoff is a function of the terminal Stock price S T ; and so the Black Scholes PDE 1 must hold for the price function of any such derivative security. 5 Notice that, to use the Itô formula, we must know a priori that the function Cx, t is twice differentiable in x and once differentiable in t. But we don t know this! So the argument of this section does not give a complete proof of the Black Scholes formula. However, since we already know that the Black Scholes formula is true, by the argument of the preceding section, we know that Cx, t is infinitely differentiable; hence, the argument of this section does at least give a proof of the Black Scholes PDE 1.
4 4. Hedging in Continuous Time Can one hedge a call option in the traded assets Cash Bond and Stock? If so, how does one do it? The answer comes by examination of the SDE 9 satisfied by the call price function CS t, t. To obtain this SDE, we once again invoke the Itô formula to get dcs t, t = C x S t, t ds t + C t S t, t + σ2 St C xxs t, t dt = C x S t, t ds t + r t S t C x S t, t + r t CS t, t dt = C x S t, t ds t + S tc x S t, t + CS t, t db t B t B t Observe that the second equality follows from the first because the call price function Cx, t must satisfy the Black Scholes PDE 1; and the third equality follows from the second because the Cash Bond price B t satisfies the ODE 1. Equation 12 shows that the instantaneous fluctuation in the price of the Call at any time t is a linear combination of the instantaneous fluctuations in the share prices of Stock and Cash Bond. The coefficients in this linear combination are expressions involving the function Cx, t and its first partial derivative C x x, t; since the call price function Cx, t is explicitly given by the Black Scholes formula 7, these coefficients may be computed to any desired degree of accuracy, at any time t. Thus, the formula 12 tells us how to replicate a European Call by holding a time dependent portfolio in Cash Bond and Stock: Hedging Strategy: At time t T, hold 13 C x S t, t S t C x S t, t + CS t, t/b t shares of Stock and shares of Cash Bond. There is only one problem 6 : how do we know that, if we start with CS, dollars at time t = and invest it according to the Hedging Strategy, we will have enough assets at time t > to buy the number of shares of Stock and Cash Bond required? This is, after all, what we would like a hedging strategy to do: if someone pays us CS, dollars 7 at time t = for a Call option, we would like to arrange things so that there is absolutely no risk to us of having to pump in any of our own money later to cover the Call at expiration T. Definition 1. A portfolio in the assets Cash Bond and Stock consists of a pair of adapted processes {α t } t T and {β t } t T, representing the number of shares of Cash Bond and Stock that are owned or shorted at times t T. The portfolio is said to be self financing if, with probability 1, for every t [, T ], 14 α t B t + β t S t = α B + β S + α s db s + β s ds s A portfolio {α t, β t } t T replicates a derivative security whose value at t = T is V T if, with probability 1, 15 V T = α T B T + β T S T. 6 Actually, there is another problem: the Hedging Strategy entails buying and selling infinitely many shares of Stock between times t = and t = T, because of the wild fluctuations in the Stock price. If there were transaction costs for buying and selling shares of Stock, the Hedging Strategy would be quite expensive to follow! More on this later. 7 plus a transaction fee that s how we make a living
5 The equation 14 states that the value α t B t + β t S t of the portfolio at any time t should be the initial value α B + β S plus the accumulated changes in value due to fluctuations db s and ds s in the values of the assets held at times up to t. A self financing portfolio that replicates a derivative security is called a hedge or a hedging strategy. Proposition 2. The portfolio defined by equations 13 is a hedge for the Call. Proof. The strategy 13 specifies the portfolio β t = C x S t, t The value of this portfolio at any time t T is and α t = S t C x S t, t + CS t, t/b t 18 α t B t + β t S t = S t C x S t, t + CS t, t + C x x, ts t = CS t, t. In particular, setting t = T, one sees that the portfolio replicates the Call. To see that the portfolio is self financing, integrate the stochastic differential equation 12 for the value of the call to obtain 19 CS t, t = CS, + α s db s + β s ds s. Substituting CS t, t = α t B t + β t S t and CS, = α B + β S, by 18, yields The Arbitrage Argument It is possible to avoid the use of the Fundamental Theorem and risk neutral measures in the derivation of the Black Scholes formula altogether by resorting to an arbitrage argument. Although this argument is somewhat circuitous, it is instructive. Assume that the price process of the Cash Bond obeys 1, and that the share price process of Stock obeys the SDE 3. 8 Since we have not assumed that the underlying probability is risk neutral, it is no longer necessary that µ t = r t. Consider the problem of pricing a derivative security whose payoff at expiration t = T is F S T, for some measurable function F of polynomial growth. Let V be the t = price of this derivative security. Theorem 1. Let Cx, t, for t T and x R +, be the unique 9 solution of the Black Scholes PDE 1 that satisfies the terminal condition Cx, T = F x. In the absence of arbitrage, 2 V = CS,. Note: The Black Scholes PDE does not involve the drift coefficient µ t that appears in the SDE 3, and so the function Cx, t does not depend in any way on the function µ t. The volatility parameter σ and the short rate r t do influence Cx, t, but unlike µ t these parameters are observable in particular, the volatility σ is determined by the quadratic variation of the Stock price process. Thus, two different investors could have two different drift functions in their models for the Stock price process, but, according to the theorem, would price the derivative security the same way. 8 Since we do not assume the existence of a risk neutral measure, the underlying probability measure implied by the SDE 3 must be given a new interpretation. It seems that most authors implicitly take this probability measure to be subjective, that is, an expression of the trader s prior opinions about the future behavior of the Stock price. 9 Uniqueness of solutions is proved by the maximum principle. Consult a book on the theory of PDEs for this.
6 Proof. Sketch. Define a portfolio α t, β t t T by equations By the same argument as in the proof of Proposition 2, the portfolio α t, β t replicates the derivative security, since the terminal condition assures that Cx, T = F x. Moreover, the Itô formula applies, as earlier, to give the SDE 12 here we use the assumption that the function Cx, t satisfies the Black Scholes PDE. Now 12 may be integrated as in the proof of Proposition 2 to give 19, which implies, again by the same argument as in the proof of Proposition 2, to show that the portfolio α t, β t is self financing. Thus, the portfolio α t, β t hedges the derivative security. Now the arbitrage. Suppose that V < CS, ; in this case, one would at time t = buy 1 derivative security; short the portfolio α, β ; and invest the proceeds CS, V in Cash Bond. In the trading period < t < T one would dynamically update the short position α, β to α t, β t, so that at the expiration time t = T the short position would cancel the payoff from the 1 derivative security bought at t =. Note that no further infusion of assets would be needed for this dynamic updating, because the portfolio α, β is self financing. At the expiration time t = T, the net proceeds would be CS, V B T >, with probability one. Since the position at time t = was flat, this is an arbitrage. Consequently, V cannot be less than CS,. The same argument, reversed, shows that V cannot be more than CS,. 6. Exercises In problems 1 2, assume that there are tradable assets Cash Bond and Stock whose price processes obey the differential equations db t = r t B t dt and ds t = S t r t dt + σ dw t, where W t is a standard Wiener process Brownian motion and r t is the short rate. Assume that the short rate r t is a continuous, non-random function of t. Problem 1: Consider a contingent claim that pays S n T A Show that the value of this contingent claim at time t T is ht, T S n t at time T, where n is a positive integer. for some function h of t, T. Hint: Use the fact that the process S t is given by 4. You should not need the Itô formula if you start from 4. B Derive an ordinary differential equation for ht, T in the variable t, and solve it. Hint: Your ordinary differential equation should be first-order, and it should involve only the short rate r t. Problem 2: Let CS t, t; K, T be the price at time t of a European call option on the tradable asset S t with strike price K and exercise time T.
7 A Show that the price function C satisfies the following symmetry properties:for any positive constant a, CS, t; K, T = CS, ; K, T t CaS, t; ak, T = acs, t; K, T a >. B Use the result of part A to derive an identity relating the partial derivatives C S and C K. C Find a PDE in the variables K, T for the function Cx, ; K, T. The equation should involve first and second partial derivatives.
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price
 On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
LECTURE 9: A MODEL FOR FOREIGN EXCHANGE
 LECTURE 9: A MODEL FOR FOREIGN EXCHANGE 1. Foreign Exchange Contracts There was a time, not so long ago, when a U. S. dollar would buy you precisely.4 British pounds sterling 1, and a British pound sterling
LECTURE 9: A MODEL FOR FOREIGN EXCHANGE 1. Foreign Exchange Contracts There was a time, not so long ago, when a U. S. dollar would buy you precisely.4 British pounds sterling 1, and a British pound sterling
CS 522 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options
 CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
The Black-Scholes Formula
 FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
LECTURE 15: AMERICAN OPTIONS
 LECTURE 15: AMERICAN OPTIONS 1. Introduction All of the options that we have considered thus far have been of the European variety: exercise is permitted only at the termination of the contract. These
LECTURE 15: AMERICAN OPTIONS 1. Introduction All of the options that we have considered thus far have been of the European variety: exercise is permitted only at the termination of the contract. These
ARBITRAGE-FREE OPTION PRICING MODELS. Denis Bell. University of North Florida
 ARBITRAGE-FREE OPTION PRICING MODELS Denis Bell University of North Florida Modelling Stock Prices Example American Express In mathematical finance, it is customary to model a stock price by an (Ito) stochatic
ARBITRAGE-FREE OPTION PRICING MODELS Denis Bell University of North Florida Modelling Stock Prices Example American Express In mathematical finance, it is customary to model a stock price by an (Ito) stochatic
Pricing of an Exotic Forward Contract
 Pricing of an Exotic Forward Contract Jirô Akahori, Yuji Hishida and Maho Nishida Dept. of Mathematical Sciences, Ritsumeikan University 1-1-1 Nojihigashi, Kusatsu, Shiga 525-8577, Japan E-mail: {akahori,
Pricing of an Exotic Forward Contract Jirô Akahori, Yuji Hishida and Maho Nishida Dept. of Mathematical Sciences, Ritsumeikan University 1-1-1 Nojihigashi, Kusatsu, Shiga 525-8577, Japan E-mail: {akahori,
How To Price A Call Option
 Now by Itô s formula But Mu f and u g in Ū. Hence τ θ u(x) =E( Mu(X) ds + u(x(τ θ))) 0 τ θ u(x) E( f(x) ds + g(x(τ θ))) = J x (θ). 0 But since u(x) =J x (θ ), we consequently have u(x) =J x (θ ) = min
Now by Itô s formula But Mu f and u g in Ū. Hence τ θ u(x) =E( Mu(X) ds + u(x(τ θ))) 0 τ θ u(x) E( f(x) ds + g(x(τ θ))) = J x (θ). 0 But since u(x) =J x (θ ), we consequently have u(x) =J x (θ ) = min
Lecture 6: Option Pricing Using a One-step Binomial Tree. Friday, September 14, 12
 Lecture 6: Option Pricing Using a One-step Binomial Tree An over-simplified model with surprisingly general extensions a single time step from 0 to T two types of traded securities: stock S and a bond
Lecture 6: Option Pricing Using a One-step Binomial Tree An over-simplified model with surprisingly general extensions a single time step from 0 to T two types of traded securities: stock S and a bond
Mathematical Finance
 Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
The Black-Scholes pricing formulas
 The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
THE FUNDAMENTAL THEOREM OF ARBITRAGE PRICING
 THE FUNDAMENTAL THEOREM OF ARBITRAGE PRICING 1. Introduction The Black-Scholes theory, which is the main subject of this course and its sequel, is based on the Efficient Market Hypothesis, that arbitrages
THE FUNDAMENTAL THEOREM OF ARBITRAGE PRICING 1. Introduction The Black-Scholes theory, which is the main subject of this course and its sequel, is based on the Efficient Market Hypothesis, that arbitrages
Black-Scholes Option Pricing Model
 Black-Scholes Option Pricing Model Nathan Coelen June 6, 22 1 Introduction Finance is one of the most rapidly changing and fastest growing areas in the corporate business world. Because of this rapid change,
Black-Scholes Option Pricing Model Nathan Coelen June 6, 22 1 Introduction Finance is one of the most rapidly changing and fastest growing areas in the corporate business world. Because of this rapid change,
Does Black-Scholes framework for Option Pricing use Constant Volatilities and Interest Rates? New Solution for a New Problem
 Does Black-Scholes framework for Option Pricing use Constant Volatilities and Interest Rates? New Solution for a New Problem Gagan Deep Singh Assistant Vice President Genpact Smart Decision Services Financial
Does Black-Scholes framework for Option Pricing use Constant Volatilities and Interest Rates? New Solution for a New Problem Gagan Deep Singh Assistant Vice President Genpact Smart Decision Services Financial
Lecture 12: The Black-Scholes Model Steven Skiena. http://www.cs.sunysb.edu/ skiena
 Lecture 12: The Black-Scholes Model Steven Skiena Department of Computer Science State University of New York Stony Brook, NY 11794 4400 http://www.cs.sunysb.edu/ skiena The Black-Scholes-Merton Model
Lecture 12: The Black-Scholes Model Steven Skiena Department of Computer Science State University of New York Stony Brook, NY 11794 4400 http://www.cs.sunysb.edu/ skiena The Black-Scholes-Merton Model
Binomial lattice model for stock prices
 Copyright c 2007 by Karl Sigman Binomial lattice model for stock prices Here we model the price of a stock in discrete time by a Markov chain of the recursive form S n+ S n Y n+, n 0, where the {Y i }
Copyright c 2007 by Karl Sigman Binomial lattice model for stock prices Here we model the price of a stock in discrete time by a Markov chain of the recursive form S n+ S n Y n+, n 0, where the {Y i }
Jung-Soon Hyun and Young-Hee Kim
 J. Korean Math. Soc. 43 (2006), No. 4, pp. 845 858 TWO APPROACHES FOR STOCHASTIC INTEREST RATE OPTION MODEL Jung-Soon Hyun and Young-Hee Kim Abstract. We present two approaches of the stochastic interest
J. Korean Math. Soc. 43 (2006), No. 4, pp. 845 858 TWO APPROACHES FOR STOCHASTIC INTEREST RATE OPTION MODEL Jung-Soon Hyun and Young-Hee Kim Abstract. We present two approaches of the stochastic interest
Option Pricing. 1 Introduction. Mrinal K. Ghosh
 Option Pricing Mrinal K. Ghosh 1 Introduction We first introduce the basic terminology in option pricing. Option: An option is the right, but not the obligation to buy (or sell) an asset under specified
Option Pricing Mrinal K. Ghosh 1 Introduction We first introduce the basic terminology in option pricing. Option: An option is the right, but not the obligation to buy (or sell) an asset under specified
第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model
 1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com
 Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Betting on Volatility: A Delta Hedging Approach. Liang Zhong
 Betting on Volatility: A Delta Hedging Approach Liang Zhong Department of Mathematics, KTH, Stockholm, Sweden April, 211 Abstract In the financial market, investors prefer to estimate the stock price
Betting on Volatility: A Delta Hedging Approach Liang Zhong Department of Mathematics, KTH, Stockholm, Sweden April, 211 Abstract In the financial market, investors prefer to estimate the stock price
FINANCIAL ECONOMICS OPTION PRICING
 OPTION PRICING Options are contingency contracts that specify payoffs if stock prices reach specified levels. A call option is the right to buy a stock at a specified price, X, called the strike price.
OPTION PRICING Options are contingency contracts that specify payoffs if stock prices reach specified levels. A call option is the right to buy a stock at a specified price, X, called the strike price.
Black-Scholes Equation for Option Pricing
 Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
7: The CRR Market Model
 Ben Goldys and Marek Rutkowski School of Mathematics and Statistics University of Sydney MATH3075/3975 Financial Mathematics Semester 2, 2015 Outline We will examine the following issues: 1 The Cox-Ross-Rubinstein
Ben Goldys and Marek Rutkowski School of Mathematics and Statistics University of Sydney MATH3075/3975 Financial Mathematics Semester 2, 2015 Outline We will examine the following issues: 1 The Cox-Ross-Rubinstein
Understanding Options and Their Role in Hedging via the Greeks
 Understanding Options and Their Role in Hedging via the Greeks Bradley J. Wogsland Department of Physics and Astronomy, University of Tennessee, Knoxville, TN 37996-1200 Options are priced assuming that
Understanding Options and Their Role in Hedging via the Greeks Bradley J. Wogsland Department of Physics and Astronomy, University of Tennessee, Knoxville, TN 37996-1200 Options are priced assuming that
Option Pricing. Chapter 4 Including dividends in the BS model. Stefan Ankirchner. University of Bonn. last update: 6th November 2013
 Option Pricing Chapter 4 Including dividends in the BS model Stefan Ankirchner University of Bonn last update: 6th November 2013 Stefan Ankirchner Option Pricing 1 Dividend payments So far: we assumed
Option Pricing Chapter 4 Including dividends in the BS model Stefan Ankirchner University of Bonn last update: 6th November 2013 Stefan Ankirchner Option Pricing 1 Dividend payments So far: we assumed
Martingale Pricing Applied to Options, Forwards and Futures
 IEOR E4706: Financial Engineering: Discrete-Time Asset Pricing Fall 2005 c 2005 by Martin Haugh Martingale Pricing Applied to Options, Forwards and Futures We now apply martingale pricing theory to the
IEOR E4706: Financial Engineering: Discrete-Time Asset Pricing Fall 2005 c 2005 by Martin Haugh Martingale Pricing Applied to Options, Forwards and Futures We now apply martingale pricing theory to the
Option pricing. Vinod Kothari
 Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
Computational Finance Options
 1 Options 1 1 Options Computational Finance Options An option gives the holder of the option the right, but not the obligation to do something. Conversely, if you sell an option, you may be obliged to
1 Options 1 1 Options Computational Finance Options An option gives the holder of the option the right, but not the obligation to do something. Conversely, if you sell an option, you may be obliged to
1 The Black-Scholes model: extensions and hedging
 1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
THE BLACK-SCHOLES MODEL AND EXTENSIONS
 THE BLAC-SCHOLES MODEL AND EXTENSIONS EVAN TURNER Abstract. This paper will derive the Black-Scholes pricing model of a European option by calculating the expected value of the option. We will assume that
THE BLAC-SCHOLES MODEL AND EXTENSIONS EVAN TURNER Abstract. This paper will derive the Black-Scholes pricing model of a European option by calculating the expected value of the option. We will assume that
Likewise, the payoff of the better-of-two note may be decomposed as follows: Price of gold (US$/oz) 375 400 425 450 475 500 525 550 575 600 Oil price
 Exchange Options Consider the Double Index Bull (DIB) note, which is suited to investors who believe that two indices will rally over a given term. The note typically pays no coupons and has a redemption
Exchange Options Consider the Double Index Bull (DIB) note, which is suited to investors who believe that two indices will rally over a given term. The note typically pays no coupons and has a redemption
QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS
 QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS L. M. Dieng ( Department of Physics, CUNY/BCC, New York, New York) Abstract: In this work, we expand the idea of Samuelson[3] and Shepp[,5,6] for
QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS L. M. Dieng ( Department of Physics, CUNY/BCC, New York, New York) Abstract: In this work, we expand the idea of Samuelson[3] and Shepp[,5,6] for
One Period Binomial Model
 FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 One Period Binomial Model These notes consider the one period binomial model to exactly price an option. We will consider three different methods of pricing
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 One Period Binomial Model These notes consider the one period binomial model to exactly price an option. We will consider three different methods of pricing
Review of Basic Options Concepts and Terminology
 Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
Hedging Options In The Incomplete Market With Stochastic Volatility. Rituparna Sen Sunday, Nov 15
 Hedging Options In The Incomplete Market With Stochastic Volatility Rituparna Sen Sunday, Nov 15 1. Motivation This is a pure jump model and hence avoids the theoretical drawbacks of continuous path models.
Hedging Options In The Incomplete Market With Stochastic Volatility Rituparna Sen Sunday, Nov 15 1. Motivation This is a pure jump model and hence avoids the theoretical drawbacks of continuous path models.
Session X: Lecturer: Dr. Jose Olmo. Module: Economics of Financial Markets. MSc. Financial Economics. Department of Economics, City University, London
 Session X: Options: Hedging, Insurance and Trading Strategies Lecturer: Dr. Jose Olmo Module: Economics of Financial Markets MSc. Financial Economics Department of Economics, City University, London Option
Session X: Options: Hedging, Insurance and Trading Strategies Lecturer: Dr. Jose Olmo Module: Economics of Financial Markets MSc. Financial Economics Department of Economics, City University, London Option
Call Price as a Function of the Stock Price
 Call Price as a Function of the Stock Price Intuitively, the call price should be an increasing function of the stock price. This relationship allows one to develop a theory of option pricing, derived
Call Price as a Function of the Stock Price Intuitively, the call price should be an increasing function of the stock price. This relationship allows one to develop a theory of option pricing, derived
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies
 Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Lecture. S t = S t δ[s t ].
![Lecture. S t = S t δ[s t ]. Lecture. S t = S t δ[s t ].](/thumbs/28/12512709.jpg) Lecture In real life the vast majority of all traded options are written on stocks having at least one dividend left before the date of expiration of the option. Thus the study of dividends is important
Lecture In real life the vast majority of all traded options are written on stocks having at least one dividend left before the date of expiration of the option. Thus the study of dividends is important
1 IEOR 4700: Introduction to stochastic integration
 Copyright c 7 by Karl Sigman 1 IEOR 47: Introduction to stochastic integration 1.1 Riemann-Stieltjes integration Recall from calculus how the Riemann integral b a h(t)dt is defined for a continuous function
Copyright c 7 by Karl Sigman 1 IEOR 47: Introduction to stochastic integration 1.1 Riemann-Stieltjes integration Recall from calculus how the Riemann integral b a h(t)dt is defined for a continuous function
Caput Derivatives: October 30, 2003
 Caput Derivatives: October 30, 2003 Exam + Answers Total time: 2 hours and 30 minutes. Note 1: You are allowed to use books, course notes, and a calculator. Question 1. [20 points] Consider an investor
Caput Derivatives: October 30, 2003 Exam + Answers Total time: 2 hours and 30 minutes. Note 1: You are allowed to use books, course notes, and a calculator. Question 1. [20 points] Consider an investor
Private Equity Fund Valuation and Systematic Risk
 An Equilibrium Approach and Empirical Evidence Axel Buchner 1, Christoph Kaserer 2, Niklas Wagner 3 Santa Clara University, March 3th 29 1 Munich University of Technology 2 Munich University of Technology
An Equilibrium Approach and Empirical Evidence Axel Buchner 1, Christoph Kaserer 2, Niklas Wagner 3 Santa Clara University, March 3th 29 1 Munich University of Technology 2 Munich University of Technology
More Exotic Options. 1 Barrier Options. 2 Compound Options. 3 Gap Options
 More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options Definition; Some types The payoff of a Barrier option is path
More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options Definition; Some types The payoff of a Barrier option is path
Lecture 6 Black-Scholes PDE
 Lecture 6 Black-Scholes PDE Lecture Notes by Andrzej Palczewski Computational Finance p. 1 Pricing function Let the dynamics of underlining S t be given in the risk-neutral measure Q by If the contingent
Lecture 6 Black-Scholes PDE Lecture Notes by Andrzej Palczewski Computational Finance p. 1 Pricing function Let the dynamics of underlining S t be given in the risk-neutral measure Q by If the contingent
Lectures. Sergei Fedotov. 20912 - Introduction to Financial Mathematics. No tutorials in the first week
 Lectures Sergei Fedotov 20912 - Introduction to Financial Mathematics No tutorials in the first week Sergei Fedotov (University of Manchester) 20912 2010 1 / 1 Lecture 1 1 Introduction Elementary economics
Lectures Sergei Fedotov 20912 - Introduction to Financial Mathematics No tutorials in the first week Sergei Fedotov (University of Manchester) 20912 2010 1 / 1 Lecture 1 1 Introduction Elementary economics
where N is the standard normal distribution function,
 The Black-Scholes-Merton formula (Hull 13.5 13.8) Assume S t is a geometric Brownian motion w/drift. Want market value at t = 0 of call option. European call option with expiration at time T. Payout at
The Black-Scholes-Merton formula (Hull 13.5 13.8) Assume S t is a geometric Brownian motion w/drift. Want market value at t = 0 of call option. European call option with expiration at time T. Payout at
Example 1. Consider the following two portfolios: 2. Buy one c(s(t), 20, τ, r) and sell one c(s(t), 10, τ, r).
 Chapter 4 Put-Call Parity 1 Bull and Bear Financial analysts use words such as bull and bear to describe the trend in stock markets. Generally speaking, a bull market is characterized by rising prices.
Chapter 4 Put-Call Parity 1 Bull and Bear Financial analysts use words such as bull and bear to describe the trend in stock markets. Generally speaking, a bull market is characterized by rising prices.
Lecture 15. Sergei Fedotov. 20912 - Introduction to Financial Mathematics. Sergei Fedotov (University of Manchester) 20912 2010 1 / 6
 Lecture 15 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 6 Lecture 15 1 Black-Scholes Equation and Replicating Portfolio 2 Static
Lecture 15 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 6 Lecture 15 1 Black-Scholes Equation and Replicating Portfolio 2 Static
Barrier Options. Peter Carr
 Barrier Options Peter Carr Head of Quantitative Financial Research, Bloomberg LP, New York Director of the Masters Program in Math Finance, Courant Institute, NYU March 14th, 2008 What are Barrier Options?
Barrier Options Peter Carr Head of Quantitative Financial Research, Bloomberg LP, New York Director of the Masters Program in Math Finance, Courant Institute, NYU March 14th, 2008 What are Barrier Options?
Two-State Option Pricing
 Rendleman and Bartter [1] present a simple two-state model of option pricing. The states of the world evolve like the branches of a tree. Given the current state, there are two possible states next period.
Rendleman and Bartter [1] present a simple two-state model of option pricing. The states of the world evolve like the branches of a tree. Given the current state, there are two possible states next period.
Options 1 OPTIONS. Introduction
 Options 1 OPTIONS Introduction A derivative is a financial instrument whose value is derived from the value of some underlying asset. A call option gives one the right to buy an asset at the exercise or
Options 1 OPTIONS Introduction A derivative is a financial instrument whose value is derived from the value of some underlying asset. A call option gives one the right to buy an asset at the exercise or
Finite Differences Schemes for Pricing of European and American Options
 Finite Differences Schemes for Pricing of European and American Options Margarida Mirador Fernandes IST Technical University of Lisbon Lisbon, Portugal November 009 Abstract Starting with the Black-Scholes
Finite Differences Schemes for Pricing of European and American Options Margarida Mirador Fernandes IST Technical University of Lisbon Lisbon, Portugal November 009 Abstract Starting with the Black-Scholes
Numerical methods for American options
 Lecture 9 Numerical methods for American options Lecture Notes by Andrzej Palczewski Computational Finance p. 1 American options The holder of an American option has the right to exercise it at any moment
Lecture 9 Numerical methods for American options Lecture Notes by Andrzej Palczewski Computational Finance p. 1 American options The holder of an American option has the right to exercise it at any moment
Black Scholes Merton Approach To Modelling Financial Derivatives Prices Tomas Sinkariovas 0802869. Words: 3441
 Black Scholes Merton Approach To Modelling Financial Derivatives Prices Tomas Sinkariovas 0802869 Words: 3441 1 1. Introduction In this paper I present Black, Scholes (1973) and Merton (1973) (BSM) general
Black Scholes Merton Approach To Modelling Financial Derivatives Prices Tomas Sinkariovas 0802869 Words: 3441 1 1. Introduction In this paper I present Black, Scholes (1973) and Merton (1973) (BSM) general
Numerical Methods for Option Pricing
 Chapter 9 Numerical Methods for Option Pricing Equation (8.26) provides a way to evaluate option prices. For some simple options, such as the European call and put options, one can integrate (8.26) directly
Chapter 9 Numerical Methods for Option Pricing Equation (8.26) provides a way to evaluate option prices. For some simple options, such as the European call and put options, one can integrate (8.26) directly
Option Pricing. Chapter 11 Options on Futures. Stefan Ankirchner. University of Bonn. last update: 13/01/2014 at 14:25
 Option Pricing Chapter 11 Options on Futures Stefan Ankirchner University of Bonn last update: 13/01/2014 at 14:25 Stefan Ankirchner Option Pricing 1 Agenda Forward contracts Definition Determining forward
Option Pricing Chapter 11 Options on Futures Stefan Ankirchner University of Bonn last update: 13/01/2014 at 14:25 Stefan Ankirchner Option Pricing 1 Agenda Forward contracts Definition Determining forward
Asian Option Pricing Formula for Uncertain Financial Market
 Sun and Chen Journal of Uncertainty Analysis and Applications (215) 3:11 DOI 1.1186/s4467-15-35-7 RESEARCH Open Access Asian Option Pricing Formula for Uncertain Financial Market Jiajun Sun 1 and Xiaowei
Sun and Chen Journal of Uncertainty Analysis and Applications (215) 3:11 DOI 1.1186/s4467-15-35-7 RESEARCH Open Access Asian Option Pricing Formula for Uncertain Financial Market Jiajun Sun 1 and Xiaowei
Valuation of the Surrender Option Embedded in Equity-Linked Life Insurance. Brennan Schwartz (1976,1979) Brennan Schwartz
 Valuation of the Surrender Option Embedded in Equity-Linked Life Insurance Brennan Schwartz (976,979) Brennan Schwartz 04 2005 6. Introduction Compared to traditional insurance products, one distinguishing
Valuation of the Surrender Option Embedded in Equity-Linked Life Insurance Brennan Schwartz (976,979) Brennan Schwartz 04 2005 6. Introduction Compared to traditional insurance products, one distinguishing
Monte Carlo Methods in Finance
 Author: Yiyang Yang Advisor: Pr. Xiaolin Li, Pr. Zari Rachev Department of Applied Mathematics and Statistics State University of New York at Stony Brook October 2, 2012 Outline Introduction 1 Introduction
Author: Yiyang Yang Advisor: Pr. Xiaolin Li, Pr. Zari Rachev Department of Applied Mathematics and Statistics State University of New York at Stony Brook October 2, 2012 Outline Introduction 1 Introduction
1 Portfolio mean and variance
 Copyright c 2005 by Karl Sigman Portfolio mean and variance Here we study the performance of a one-period investment X 0 > 0 (dollars) shared among several different assets. Our criterion for measuring
Copyright c 2005 by Karl Sigman Portfolio mean and variance Here we study the performance of a one-period investment X 0 > 0 (dollars) shared among several different assets. Our criterion for measuring
Jorge Cruz Lopez - Bus 316: Derivative Securities. Week 11. The Black-Scholes Model: Hull, Ch. 13.
 Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
Options Markets: Introduction
 Options Markets: Introduction Chapter 20 Option Contracts call option = contract that gives the holder the right to purchase an asset at a specified price, on or before a certain date put option = contract
Options Markets: Introduction Chapter 20 Option Contracts call option = contract that gives the holder the right to purchase an asset at a specified price, on or before a certain date put option = contract
A Martingale System Theorem for Stock Investments
 A Martingale System Theorem for Stock Investments Robert J. Vanderbei April 26, 1999 DIMACS New Market Models Workshop 1 Beginning Middle End Controversial Remarks Outline DIMACS New Market Models Workshop
A Martingale System Theorem for Stock Investments Robert J. Vanderbei April 26, 1999 DIMACS New Market Models Workshop 1 Beginning Middle End Controversial Remarks Outline DIMACS New Market Models Workshop
OPTION PRICING FOR WEIGHTED AVERAGE OF ASSET PRICES
 OPTION PRICING FOR WEIGHTED AVERAGE OF ASSET PRICES Hiroshi Inoue 1, Masatoshi Miyake 2, Satoru Takahashi 1 1 School of Management, T okyo University of Science, Kuki-shi Saitama 346-8512, Japan 2 Department
OPTION PRICING FOR WEIGHTED AVERAGE OF ASSET PRICES Hiroshi Inoue 1, Masatoshi Miyake 2, Satoru Takahashi 1 1 School of Management, T okyo University of Science, Kuki-shi Saitama 346-8512, Japan 2 Department
Notes on Black-Scholes Option Pricing Formula
 . Notes on Black-Scholes Option Pricing Formula by De-Xing Guan March 2006 These notes are a brief introduction to the Black-Scholes formula, which prices the European call options. The essential reading
. Notes on Black-Scholes Option Pricing Formula by De-Xing Guan March 2006 These notes are a brief introduction to the Black-Scholes formula, which prices the European call options. The essential reading
Lecture 21 Options Pricing
 Lecture 21 Options Pricing Readings BM, chapter 20 Reader, Lecture 21 M. Spiegel and R. Stanton, 2000 1 Outline Last lecture: Examples of options Derivatives and risk (mis)management Replication and Put-call
Lecture 21 Options Pricing Readings BM, chapter 20 Reader, Lecture 21 M. Spiegel and R. Stanton, 2000 1 Outline Last lecture: Examples of options Derivatives and risk (mis)management Replication and Put-call
Continued Fractions and the Euclidean Algorithm
 Continued Fractions and the Euclidean Algorithm Lecture notes prepared for MATH 326, Spring 997 Department of Mathematics and Statistics University at Albany William F Hammond Table of Contents Introduction
Continued Fractions and the Euclidean Algorithm Lecture notes prepared for MATH 326, Spring 997 Department of Mathematics and Statistics University at Albany William F Hammond Table of Contents Introduction
Option Values. Option Valuation. Call Option Value before Expiration. Determinants of Call Option Values
 Option Values Option Valuation Intrinsic value profit that could be made if the option was immediately exercised Call: stock price exercise price : S T X i i k i X S Put: exercise price stock price : X
Option Values Option Valuation Intrinsic value profit that could be made if the option was immediately exercised Call: stock price exercise price : S T X i i k i X S Put: exercise price stock price : X
1 The Black-Scholes Formula
 1 The Black-Scholes Formula In 1973 Fischer Black and Myron Scholes published a formula - the Black-Scholes formula - for computing the theoretical price of a European call option on a stock. Their paper,
1 The Black-Scholes Formula In 1973 Fischer Black and Myron Scholes published a formula - the Black-Scholes formula - for computing the theoretical price of a European call option on a stock. Their paper,
Chapter 2: Binomial Methods and the Black-Scholes Formula
 Chapter 2: Binomial Methods and the Black-Scholes Formula 2.1 Binomial Trees We consider a financial market consisting of a bond B t = B(t), a stock S t = S(t), and a call-option C t = C(t), where the
Chapter 2: Binomial Methods and the Black-Scholes Formula 2.1 Binomial Trees We consider a financial market consisting of a bond B t = B(t), a stock S t = S(t), and a call-option C t = C(t), where the
BINOMIAL OPTIONS PRICING MODEL. Mark Ioffe. Abstract
 BINOMIAL OPTIONS PRICING MODEL Mark Ioffe Abstract Binomial option pricing model is a widespread numerical method of calculating price of American options. In terms of applied mathematics this is simple
BINOMIAL OPTIONS PRICING MODEL Mark Ioffe Abstract Binomial option pricing model is a widespread numerical method of calculating price of American options. In terms of applied mathematics this is simple
Power-law Price-impact Models and Stock Pinning near Option Expiration Dates. Marco Avellaneda Gennady Kasyan Michael D. Lipkin
 Power-law Price-impact Models and Stock Pinning near Option Expiration Dates Marco Avellaneda Gennady Kasyan Michael D. Lipkin PDE in Finance, Stockholm, August 7 Summary Empirical evidence of stock pinning
Power-law Price-impact Models and Stock Pinning near Option Expiration Dates Marco Avellaneda Gennady Kasyan Michael D. Lipkin PDE in Finance, Stockholm, August 7 Summary Empirical evidence of stock pinning
Option Pricing. Chapter 12 - Local volatility models - Stefan Ankirchner. University of Bonn. last update: 13th January 2014
 Option Pricing Chapter 12 - Local volatility models - Stefan Ankirchner University of Bonn last update: 13th January 2014 Stefan Ankirchner Option Pricing 1 Agenda The volatility surface Local volatility
Option Pricing Chapter 12 - Local volatility models - Stefan Ankirchner University of Bonn last update: 13th January 2014 Stefan Ankirchner Option Pricing 1 Agenda The volatility surface Local volatility
2. Exercising the option - buying or selling asset by using option. 3. Strike (or exercise) price - price at which asset may be bought or sold
 Chapter 21 : Options-1 CHAPTER 21. OPTIONS Contents I. INTRODUCTION BASIC TERMS II. VALUATION OF OPTIONS A. Minimum Values of Options B. Maximum Values of Options C. Determinants of Call Value D. Black-Scholes
Chapter 21 : Options-1 CHAPTER 21. OPTIONS Contents I. INTRODUCTION BASIC TERMS II. VALUATION OF OPTIONS A. Minimum Values of Options B. Maximum Values of Options C. Determinants of Call Value D. Black-Scholes
The interest volatility surface
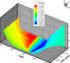 The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
Lecture 5: Put - Call Parity
 Lecture 5: Put - Call Parity Reading: J.C.Hull, Chapter 9 Reminder: basic assumptions 1. There are no arbitrage opportunities, i.e. no party can get a riskless profit. 2. Borrowing and lending are possible
Lecture 5: Put - Call Parity Reading: J.C.Hull, Chapter 9 Reminder: basic assumptions 1. There are no arbitrage opportunities, i.e. no party can get a riskless profit. 2. Borrowing and lending are possible
LOGNORMAL MODEL FOR STOCK PRICES
 LOGNORMAL MODEL FOR STOCK PRICES MICHAEL J. SHARPE MATHEMATICS DEPARTMENT, UCSD 1. INTRODUCTION What follows is a simple but important model that will be the basis for a later study of stock prices as
LOGNORMAL MODEL FOR STOCK PRICES MICHAEL J. SHARPE MATHEMATICS DEPARTMENT, UCSD 1. INTRODUCTION What follows is a simple but important model that will be the basis for a later study of stock prices as
Simulating Stochastic Differential Equations
 Monte Carlo Simulation: IEOR E473 Fall 24 c 24 by Martin Haugh Simulating Stochastic Differential Equations 1 Brief Review of Stochastic Calculus and Itô s Lemma Let S t be the time t price of a particular
Monte Carlo Simulation: IEOR E473 Fall 24 c 24 by Martin Haugh Simulating Stochastic Differential Equations 1 Brief Review of Stochastic Calculus and Itô s Lemma Let S t be the time t price of a particular
Chapter 1: Financial Markets and Financial Derivatives
 Chapter 1: Financial Markets and Financial Derivatives 1.1 Financial Markets Financial markets are markets for financial instruments, in which buyers and sellers find each other and create or exchange
Chapter 1: Financial Markets and Financial Derivatives 1.1 Financial Markets Financial markets are markets for financial instruments, in which buyers and sellers find each other and create or exchange
MTH6120 Further Topics in Mathematical Finance Lesson 2
 MTH6120 Further Topics in Mathematical Finance Lesson 2 Contents 1.2.3 Non-constant interest rates....................... 15 1.3 Arbitrage and Black-Scholes Theory....................... 16 1.3.1 Informal
MTH6120 Further Topics in Mathematical Finance Lesson 2 Contents 1.2.3 Non-constant interest rates....................... 15 1.3 Arbitrage and Black-Scholes Theory....................... 16 1.3.1 Informal
From Binomial Trees to the Black-Scholes Option Pricing Formulas
 Lecture 4 From Binomial Trees to the Black-Scholes Option Pricing Formulas In this lecture, we will extend the example in Lecture 2 to a general setting of binomial trees, as an important model for a single
Lecture 4 From Binomial Trees to the Black-Scholes Option Pricing Formulas In this lecture, we will extend the example in Lecture 2 to a general setting of binomial trees, as an important model for a single
Option Valuation. Chapter 21
 Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Black-Scholes-Merton approach merits and shortcomings
 Black-Scholes-Merton approach merits and shortcomings Emilia Matei 1005056 EC372 Term Paper. Topic 3 1. Introduction The Black-Scholes and Merton method of modelling derivatives prices was first introduced
Black-Scholes-Merton approach merits and shortcomings Emilia Matei 1005056 EC372 Term Paper. Topic 3 1. Introduction The Black-Scholes and Merton method of modelling derivatives prices was first introduced
a. What is the portfolio of the stock and the bond that replicates the option?
 Practice problems for Lecture 2. Answers. 1. A Simple Option Pricing Problem in One Period Riskless bond (interest rate is 5%): 1 15 Stock: 5 125 5 Derivative security (call option with a strike of 8):?
Practice problems for Lecture 2. Answers. 1. A Simple Option Pricing Problem in One Period Riskless bond (interest rate is 5%): 1 15 Stock: 5 125 5 Derivative security (call option with a strike of 8):?
Exam MFE Spring 2007 FINAL ANSWER KEY 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D
 Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
ONE PERIOD MODELS t = TIME = 0 or 1
 BASIC INSTRUMENTS: * S t : STOCK * B t : RISKLESS BOND ONE PERIOD MODELS t = TIME = 0 or 1 B 0 = 1 B 1 = 1 + r or e r * FORWARD CONTRACT: AGREEMENT TO SWAP $$ FOR STOCK - AGREEMENT TIME: t = 0 - AGREEMENT
BASIC INSTRUMENTS: * S t : STOCK * B t : RISKLESS BOND ONE PERIOD MODELS t = TIME = 0 or 1 B 0 = 1 B 1 = 1 + r or e r * FORWARD CONTRACT: AGREEMENT TO SWAP $$ FOR STOCK - AGREEMENT TIME: t = 0 - AGREEMENT
Hedging. An Undergraduate Introduction to Financial Mathematics. J. Robert Buchanan. J. Robert Buchanan Hedging
 Hedging An Undergraduate Introduction to Financial Mathematics J. Robert Buchanan 2010 Introduction Definition Hedging is the practice of making a portfolio of investments less sensitive to changes in
Hedging An Undergraduate Introduction to Financial Mathematics J. Robert Buchanan 2010 Introduction Definition Hedging is the practice of making a portfolio of investments less sensitive to changes in
Hedging Variable Annuity Guarantees
 p. 1/4 Hedging Variable Annuity Guarantees Actuarial Society of Hong Kong Hong Kong, July 30 Phelim P Boyle Wilfrid Laurier University Thanks to Yan Liu and Adam Kolkiewicz for useful discussions. p. 2/4
p. 1/4 Hedging Variable Annuity Guarantees Actuarial Society of Hong Kong Hong Kong, July 30 Phelim P Boyle Wilfrid Laurier University Thanks to Yan Liu and Adam Kolkiewicz for useful discussions. p. 2/4
COMPLETE MARKETS DO NOT ALLOW FREE CASH FLOW STREAMS
 COMPLETE MARKETS DO NOT ALLOW FREE CASH FLOW STREAMS NICOLE BÄUERLE AND STEFANIE GRETHER Abstract. In this short note we prove a conjecture posed in Cui et al. 2012): Dynamic mean-variance problems in
COMPLETE MARKETS DO NOT ALLOW FREE CASH FLOW STREAMS NICOLE BÄUERLE AND STEFANIE GRETHER Abstract. In this short note we prove a conjecture posed in Cui et al. 2012): Dynamic mean-variance problems in
European Call Option Pricing using the Adomian Decomposition Method
 Advances in Dynamical Systems and Applications ISSN 0973-5321, Volume 9, Number 1, pp. 75 85 (2014) http://campus.mst.edu/adsa European Call Option Pricing using the Adomian Decomposition Method Martin
Advances in Dynamical Systems and Applications ISSN 0973-5321, Volume 9, Number 1, pp. 75 85 (2014) http://campus.mst.edu/adsa European Call Option Pricing using the Adomian Decomposition Method Martin
LogNormal stock-price models in Exams MFE/3 and C/4
 Making sense of... LogNormal stock-price models in Exams MFE/3 and C/4 James W. Daniel Austin Actuarial Seminars http://www.actuarialseminars.com June 26, 2008 c Copyright 2007 by James W. Daniel; reproduction
Making sense of... LogNormal stock-price models in Exams MFE/3 and C/4 James W. Daniel Austin Actuarial Seminars http://www.actuarialseminars.com June 26, 2008 c Copyright 2007 by James W. Daniel; reproduction
Chapter 5 Financial Forwards and Futures
 Chapter 5 Financial Forwards and Futures Question 5.1. Four different ways to sell a share of stock that has a price S(0) at time 0. Question 5.2. Description Get Paid at Lose Ownership of Receive Payment
Chapter 5 Financial Forwards and Futures Question 5.1. Four different ways to sell a share of stock that has a price S(0) at time 0. Question 5.2. Description Get Paid at Lose Ownership of Receive Payment
4: SINGLE-PERIOD MARKET MODELS
 4: SINGLE-PERIOD MARKET MODELS Ben Goldys and Marek Rutkowski School of Mathematics and Statistics University of Sydney Semester 2, 2015 B. Goldys and M. Rutkowski (USydney) Slides 4: Single-Period Market
4: SINGLE-PERIOD MARKET MODELS Ben Goldys and Marek Rutkowski School of Mathematics and Statistics University of Sydney Semester 2, 2015 B. Goldys and M. Rutkowski (USydney) Slides 4: Single-Period Market
The Behavior of Bonds and Interest Rates. An Impossible Bond Pricing Model. 780 w Interest Rate Models
 780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
Integrals of Rational Functions
 Integrals of Rational Functions Scott R. Fulton Overview A rational function has the form where p and q are polynomials. For example, r(x) = p(x) q(x) f(x) = x2 3 x 4 + 3, g(t) = t6 + 4t 2 3, 7t 5 + 3t
Integrals of Rational Functions Scott R. Fulton Overview A rational function has the form where p and q are polynomials. For example, r(x) = p(x) q(x) f(x) = x2 3 x 4 + 3, g(t) = t6 + 4t 2 3, 7t 5 + 3t
Introduction to Arbitrage-Free Pricing: Fundamental Theorems
 Introduction to Arbitrage-Free Pricing: Fundamental Theorems Dmitry Kramkov Carnegie Mellon University Workshop on Interdisciplinary Mathematics, Penn State, May 8-10, 2015 1 / 24 Outline Financial market
Introduction to Arbitrage-Free Pricing: Fundamental Theorems Dmitry Kramkov Carnegie Mellon University Workshop on Interdisciplinary Mathematics, Penn State, May 8-10, 2015 1 / 24 Outline Financial market
On Market-Making and Delta-Hedging
 On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
Sensitivity analysis of utility based prices and risk-tolerance wealth processes
 Sensitivity analysis of utility based prices and risk-tolerance wealth processes Dmitry Kramkov, Carnegie Mellon University Based on a paper with Mihai Sirbu from Columbia University Math Finance Seminar,
Sensitivity analysis of utility based prices and risk-tolerance wealth processes Dmitry Kramkov, Carnegie Mellon University Based on a paper with Mihai Sirbu from Columbia University Math Finance Seminar,
