A Formula Sheet for Financial Economics
|
|
|
- Abigail Hampton
- 8 years ago
- Views:
Transcription
1 A Formula Sheet for Financial Economics William Benedict McCartney April 2012 Abstract This document is meant to be used solely as a formula sheet. It contains very little in the way of explanation and is not meant to be used as a substitute for a financial economics text. It is aimed specifically at those students preparing for exam MFE offered by the Society of Actuaries, but it should be of some use to everyone studying financial economics. It covers the important formulas and methods used in put-call parity, option pricing using binomial trees, Brownian motions, stochastic calculus, stock price dynamics, the Sharpe ratio, the Black-Scholes equation, the Black-Scholes formula, option greeks, risk management techniques, estimations of volatilities and rates of appreciation, exotic options (asian, barrier, compound, gap, and exchange), simulation, interest rate trees, the Black model, and several interest rate models (Rendleman-Bartter, Vasicek, and Cox-Ingersoll-Ross) 1
2 1 Forwards, Puts, and Calls 1.1 Forwards A forward contract is an agreement in which the buyer agrees at time t to pay the seller at time T and receive the asset at time T. F t,t (S) = S t e r(t t) = S t e r(t t) F V t,t (Dividends) = S t e (r δ)(t t) (1) A prepaid forward contract is an agreement in which the buyer agrees at time t to pay the seller at time t and receive the asset at time T. F P t,t (S) = S t or S t P V t,t (Dividends) or S t e δ(t t) (2) 1.2 Put-Call Parity Call options give the owner the right, but not the obligation, to buy an asset at some time in the future for a predetermined strike price. Put options give the owner the right to sell. The price of calls and puts is compared in the following put-call parity formula for European options. c(s t, K, t, T ) p(s t, K, t, T ) = F P t,t (S) Ke r(t t) (3) 1.3 Calls and Puts with Different Strikes For European calls and puts, with strike prices K 1 and K 2 where K 1 < K 2, we know the following 0 c(k 1 ) c(k 2 ) (K 2 K 1 )e rt (4) 0 p(k 2 ) p(k 1 ) (K 2 K 1 )e rt (5) For American options, we cannot be so strict. Delete the discount factor on the (K 2 K 1 ) term and then you re okay. Another important result arises for three different options with strike prices K 1 < K 2 < K 3 c(k 1 ) c(k 2 ) K 2 K 1 c(k 2) c(k 3 ) K 3 K 2 (6) p(k 2 ) p(k 1 ) K 2 K 1 p(k 3) p(k 2 ) K 3 K 2 (7) Exam MFE loves arbitrage questions. An important formula for determining arbitrage opportunities comes from the following equations. K 2 = λk 1 + (1 λ)k 3 (8) λ = K 3 K 2 K 3 K 1 (9) 2
3 The coefficients in front of each strike price in equation 8 represent the number of options of each strike price to buy for two equivalent portfolios in an arbitragefree market. 1.4 Call and Put Price Bounds The following equations give the bounds on the prices of European calls and puts. Note that the lower bounds are no less than zero. (F P t,t (S) Ke r(t t) ) + c(s t, K, t, T ) F P t,t (S) (10) (Ke r(t t) F P t,t (S)) + p(s t, K, t, T ) Ke r(t t) (11) We can also compare the prices of European and American options using the following inequalities. c(s t, K, t, T ) C(S t, K, t, T ) S t (12) p(s t, K, t, T ) P (S t, K, t, T ) K (13) 1.5 Varying Times to Expiration For American options only, when T 2 > T 1 C(S t, K, t, T 2 ) C(S t, K, t, T 1 ) S t (14) P (S t, K, t, T 2 ) P (S t, K, t, T 1 ) S t (15) 1.6 Early Exercise for American Options In the following inequality the two sides can be thought of the pros and cons of exercising the call early. The pros of exercising early are getting the stock s dividend payments. The cons are that we have to pay the strike earlier and therefore miss the interest on that money and we lose the put protection if the stock price should fall. So we exercise the call option if the pros are greater than the cons, specifically, we exercise if P V t,t (dividends) > p(s t, K) + K(1 e r(t t) ) (16) For puts, the situation is slightly different. The pros are the interest earned on the strike. The cons are the lost dividends on owning the stock and the call protection should the stock price rise. Explicitly, we exercise the put option early if K(1 e r(t t) ) > c(s t, K) + P V t,t (dividends) (17) 3
4 2 Binomial Trees 2.1 The One-Period Replicating Portfolio The main idea is to replicate the payoffs of the derivative with the stock and a risk-free bond. e δh S 0 u + Be rh = C u (18) e δh S 0 d + Be rh = C d (19) To replicate the derivative we buy shares and invest in B dollars. = e δh C u C d S 0 (u d) (20) B = e rh uc d dc u (21) u d Since we designed the portfolio to replicate the option they must, since there is no arbitrage, have the same time-0 price. 2.2 Risk-Neutral Probabilities C 0 = S 0 + B (22) We define the risk-neutral probability of the stock price going up as follows Then the price of the option is p = e(r δ)h d u d (23) C 0 = e rh [p C u (1 p )C d ] (24) A key result of the risk-neutral world is that the expected price of the stock at future time t is 2.3 Multi-Period Trees E [S t ] = p S 0 u + (1 p )S 0 d = S 0 e rt (25) The single period binomial trees formulas can be used to go back one step at a time on the tree. Also, note that for a European option we can use this shortcut formula. C 0 = e 2rh [(p ) 2 C uu + 2p (1 p )C ud + (1 p ) 2 C dd ] (26) For American options, however, it s important to check the price of the option at each node of the tree. If the price of the option is less than the payout, then the option would be exercised and the price at that node should be the payoff at that point. 4
5 2.4 Trees from Volatilities Assuming a forward tree u = e (r δ)h+σ h d = e (r δ)h σ h p 1 = 1 + e σ h Assuming a Cox-Ross-Rubinstein tree u = e σ h d = e σ h Assuming a Jarrow-Rudd (lognormal) forward tree u = e (r δ 1 2 σ2 )h+σ h d = e (r δ 1 2 σ2 )h σ h (27) (28) (29) (30) (31) (32) (33) 2.5 Options on Currencies The easiest way to deal with these options is to treat the exchange rate as a stock where x(t) is the underlying, r f is the dividend rate and r is the risk-free rate. It is helpful to understand these following equation = $x(t) (34) Remember that if you treat x(t) as the underlying asset than r is the risk-free rate for dollars, r f is the risk-free rate for pounds, and the option is considered dollar-denominated. 2.6 Options on Futures c(k, T ) p(k, T ) = x 0 e r f T Ke rt (35) The main difference between futures and the other previously discussed assets is that futures don t initially require any assets to change hands. The formulas are therefore adjusted as follows = C u C d F 0 (u d) B = C 0 = e rh ( 1 d u d C u + u 1 u d C d ) (36) (37) Note that the previous equation becomes clearer when we define p, which, because it is sometimes possible to think of a forward as a stock with δ = r, as 5
6 p = 1 d (38) u d The other formulas all work the same way. Notably, the put-call parity formula becomes 2.7 True Probability Pricing c(k, T ) p(k, T ) = F 0 e rt Ke rt (39) We ve been assuming a risk-free world in the previous formulas as it makes dealing with some problems nicer. But it s important to examine the following real-world or true probability formulas. E(S t ) = ps 0 u + (1 p)s 0 d = S 0 e (α δ)t (40) p = e(α δ)t d (41) u d It is possible to price options using real world probabilities. But r can no longer be used. Instead γ is the appropriate discount rate 2.8 State Prices e γt = S 0 B S 0 + B eαt + S 0 + B ert (42) C 0 = e γt [C u + (1 p)c d ] (43) State prices are so called because it s the cost of a security that pays one dollar upon reaching a particular state. Remember these following formulas for determining state prices Q H + Q L = e rt (44) S H Q H + S L Q L = F P 0,t(S) (45) C 0 = C H Q H + C L Q L (46) The above equations can easily be adapted for trinomial and higher order trees. The economic concept of utility also enters the stage in the following equations. Understand that, for example, U H is the utility value in today s dollars attached to one dollar received in the up state. This leads to the following result. p = Q H = pu H (47) Q L = (1 p)u L (48) pu H Q H = (49) pu H + (1 p)u L Q H + Q L 6
7 3 Continuous-Time Finance More specifically, this section is going to cover Brownian motions, stochastic calculus and the lognormality of stock prices and introduce the Black-Scholes equation. 3.1 Standard Brownian Motion The important properties of an SBM are as follows. One, Z(t) N(0, t). Two, {Z(t)} has independent increments. And three, {Z(t)} has stationary increments such that Z(t + s) Z(t) N(0, s). Also useful is the fact that given a Z(u) : 0 u t, Z(t + s) N(Z(t), s). 3.2 Arithmetic Brownian Motion We define X(t) to be an arithmetic Brownian motion with drift coefficient µ and volatility σ if X(t) = µt+σz(t). Note that an arithmetic Brownian motion with µ = 0 is called a driftless ABM. Finally, X(t) N(µt, σ 2 t). 3.3 Geometric Brownian Motion Arithmetic Brownian motions can be zero, though, and have a mean and variance that don t depend on the level of stock making them a poor model for stock prices. To solve these problems we consider a geometric Brownian motion. Y (t) = Y (0)e X(t) = Y (0)e [µt+σz(t)] (50) The following equations can be used to find the moments of a GBM. In equation 51, let U be any normal random variable. E(e ku ) = e ke(u)+ 1 2 k2 V ar(u) So specifically for geometric Brownian motions E[Y k (t)] = Y k (0)e (kµ+ 1 2 k2 σ 2 )t Also note, then, that Y (t) is lognormally distributed as follows (51) (52) 3.4 Ito s Lemma lny (t) N(lnY (0) + µt, σ 2 t) (53) First define X as a diffusion and present the following stochastic differential equation. Then for dx(t) = a(t, X(t))dt + b(t, X(t))dZ(t) (54) 7
8 We have Y (t) = f(t, X(t))dt (55) dy (t) = f t (t, X(t)) + f x (t, X(t))dX(t) f xx(t, X(t))[dX(t)] 2 (56) where 3.5 Stochastic Integrals Recall the fundamental theorem of calculus. d dt [dx(t)] 2 = b 2 (t, X(t))dt (57) t The rule for stochastic integrals looks very similar. d t 0 0 a(s, X(s))ds = a(t, X(t)) (58) b(s, X(s))dZ(s) = b(t, X(t))dZ(t) (59) 3.6 Solutions to Some Common SDEs For arithmetic Brownian motions, we can say the following dy (t) = αdt + σdz(t) (60) Y (t) = Y (0) + αt + σz(t) (61) For geometric Brownian motions, there are several equivalent statements. dy (t) = µy (t)dt + σy (t)dz(t) (62) ) d[lny (t)] = (µ σ2 dt + σdz(t) 2 (63) Y (t) = Y (0)e (µ σ2 2 ) t+σz(t) (64) And for Ornstein-Uhlenback processes, which will become very useful when we get to interest rate models, we know dy (t) = λ[α Y (t)]dt + σdz(t) (65) Y (t) = α + [Y (0) α]e λt + σ t 0 e λ(t s) dz(s) (66) 8
9 3.7 Brownian Motion Variation For any function Y(t), the kth-order variation is b a dy (t) k (67) For standard Brownian motions we can say that the total variation is infinity, the quadratic variation is (b a) and higher-order variations are zero. And for arithmetic Brownian motions we know that the total variation is infinity, the quadratic variation is (b a)σ 2 and higher-order variations are zero. 3.8 Stock Prices as a GBM A stock price that pays constant dividends at a rate δ, such that the rate of appreciation is α δ, follows the following stochastic differential equation and solution ds(t) S(t) = (α δ)dt + σdz(t) (68) σ2 (α δ S(t) = S(0)e 2 )t+σz(t) (69) d[lns(t)] = (α δ σ2 )dt + σdz(t) (70) 2 Therefore we can say that S(t) LN(lnS(0) + (α δ σ2 2 )t, σ2 t) (71) 3.9 Since Stock Prices are Lognormal Stock prices being lognormal gives many convenient formulas. First, we can determine the percentiles of different values of the future stock price and therefore confidence intervals as follows σ2 (α δ 100p th percentile of S(t) = S(0)e 2 )t+σ tn 1 (p) (72) σ2 (α δ 100(1 - β)% lognormal CI for S(t) is S(0)e 2 )t±z β/2σ t (73) Similarly we know the probability that the future stock price will be above or below some value. We find this probability using the following two equations. P (S(t) K) = N( ˆd 2 ) (74) ˆd 2 = ln S(0) K σ2 + (α δ 2 )t σ t (75) 9
10 And we can determine all the moments of the future stock price. E[S k (t)] = S k (0)e k(α δ)t+ 1 2 k(k 1)(σ2 t) Also very useful is the conditional expected price formulas. E[S(t) S(t) < K] = E[S(t)] N( ˆd 1 ) N( ˆd 2 ) E[S(t) S(t) > K] = E[S(t)] N( ˆd 1 ) N( ˆd 2 ) (76) (77) (78) where ˆd 2 is defined as before and ˆd 1 is ˆd 1 = ln S(0) K σ2 + (α δ + 2 )t σ t (79) 3.10 The Sharpe Ratio and Hedging For any asset that has the dynamics dx(t) X(t) = mdt + sdz(t) and a continuously compounded dividend rate δ, the Sharpe ratio is defined as φ = m + δ r (80) s Recalling that for a stock, m = α δ the Sharpe ratio of any asset written on a GBM is φ = α r (81) σ The key thing to remember is that for any two assets with the same dynamics the Sharpe ratio must be the same. Using this fact we can derive the following hedging formulas. We can hedge a position of long one unit of X by buying N units of Y and investing W dollars. N = s XX(t) s Y Y (t) 3.11 The Black-Scholes Equation (82) W = X(t) NY (t) (83) If we look at any derivative with value V (S(t), t), use Itô s lemma to find dv (S(t), t), and put this into the Sharpe ratio formula we can derive the Black- Scholes equation. rv = V t + (r δ)sv s σ2 S 2 V ss (84) 10
11 3.12 Risk-Neutral Valuation and Power Contracts Recall that to switch from the real world to the risk-neutral world we exchange α for r which leads to the following risk-neutral dynamics. ds(t) S(t) = (r δ)dt + σd[ Z(t)] (85) Using these risk-neutral equations we can show that Z(t) = Z(t) + φt (86) V (S(t), t) = e r(t t) E [V (S(T ), T ) S(T )] (87) This equation can be used to derive the following time-t price of a power contract. The payoff of a power contract is S a (T ) at time T and the price is F p t,t (Sa ) = S a (t)e ( r+a(r δ)+ 1 2 a(a 1)σ2 )(T t) (88) 11
12 4 The Black-Scholes Formula 4.1 Binary Options Binary in this case is a fancy way of saying all or nothing. Binary Option Price Cash-or-Nothing Call e r(t t) N(d 2 ) Cash-or-Nothing Put e r(t t) N( d 2 ) Asset-or-Nothing Call S(t)e r(t t) N(d 1 ) Asset-or-Nothing Put S(t)e r(t t) N( d 1 ) Where d 1 and d 2 are defined as before except that α is replaced with r. 4.2 The Black-Scholes Formula With the binary option formulas in hand it s just a hop, skip, and a jump to the Black Scholes Formulas. c(s(t), K, t) = S(t)e δ(t t) N(d 1 ) Ke r(t t) N(d 2 ) (89) p(s(t), K, t) = Ke r(t t) N( d 2 ) S(t)e δ(t t) N( d 1 ) (90) It s helpful to note that, just like with the binomial formulas, for options on currencies we can replace δ with r f and for options on futures we can replace δ with r. Another option is to use the more general prepaid forward version of the Black-Scholes option pricing formulas. 4.3 The Prepaid Forward Version c(s(t), K, t) = F P t,t (S)N(d 1 ) F P t,t (K)N(d 2 ) (91) where p(s(t), K, t) = F P t,t (K)N( d 2 ) F P t,t (S)N( d 1 ) (92) d 2 = d 1 = ln F P t,t (S) F P t,t (K) σ2 (T t) σ T t ln F P t,t (S) F P t,t (K) 1 2 σ2 (T t) σ T t (93) = d 1 σ T t (94) So far an option on any asset, just plug in the correct prepaid forward price. As a reminder, for currencies this is x(t)e r f (T t) and for futures this is F (t)e r(t t). In other words, we are simply replacing δ with the appropriate variable. 12
13 4.4 Greeks and Their Uses Greeks are what are used to measure the risk of a derivative. The following three are the most important. = V S, Γ = V SS = S, θ = V t (95) The formula for the delta of a call and the delta of a put are useful to have memorized. c = e δ(t t) N(d 1 ) (96) p = e δ(t t) N( d 1 ) (97) From the Black-Scholes equation we get the following relationship. θ + (r δ)s σ2 S 2 Γ = rv (98) The following are the most important properties of the greeks. is positive for calls and negative for puts. Γ is always positive. θ is negative for calls and puts generally, but can be positive for very in-the-money puts. Also, ν is always positive while ψ is negative for a call and positive for a put and ρ is positive for a call and negative for a put. Note that the signs of these greeks is assuming we are long. We can approximate the price of a new derivative if we know the price of a slightly different derivative using the Delta-Gamma approximation. V (S + ε, t) V (S, t) + (S, t)ε Γ(S, t)ε2 (99) This assumes an instantaneous change in the underlying price. A more useful formula is the Delta-Gamma-Theta Approximation. V (S(t + h), t + h) V (S(t), t) + (S(t), t)ε Γ(S(t), t)ε2 + θ(s(t), t)h (100) ε = S(t + h) S(t) (101) To find the Delta, Gamma, or Theta for a portfolio, as opposed to a single security, simply add up the greeks of the options in the portfolio. 4.5 Elasticity We can write the expected return and the volatility of a derivative. m V = Ωα + (1 Ω)r (102) s V = Ωσ = S σ V (103) 13
14 Ω is defined to be the elasticity. It s interpretation is the same as the economics interpretation. Also, we can conclude that if Ω > 1 than the option is riskier than the underlying. Unlike the greeks, we cannot just sum the elasticities of the options in the portfolio to find the portfolio s elasticity. Ω = Σ w iv i P Ω i (104) Where w i is how many units of the i th derivative are in the portfolio. 4.6 Greeks From Trees Γ(S h, h) = = e δh C u C d S u S d (105) (Su, h) (Sd, h) Su Sd (106) θ(s, 0) 1 2h [C(Sud, 2h) ε (S, 0) 1 2 ε2 Γ(S, 0) C(S, 0)] (107) 4.7 Delta-Hedging To Delta-Hedge a portfolio we want to make it such that the delta of the portfolio is zero. So if we are long one unit of an option written on X we purchase N units of the underlying and invest W dollars. N = s S σ XX(t) s Y Y (t) = V V = (108) σs W = X(t) NY (t) = V + S (109) It is also possible to hedge on any other greek, simply enter into other positions until the value of the greek for the portfolio is zero. It is easy to determine the hedge profit and variance of the expected hedge profit. Γ < 0 S(t)(1 σ h) < S(t + h) < S(t)(1 + σ h) (110) V ar(profit) = 1 2 {[Γ(S(t), t)σ2 S 2 (t)]h} 2 (111) 4.8 Estimation of Volatilities & Appreciation Rates Given a set of stock prices at different times, it is possible to estimate the volatility of the stock using the following methodology. First, define u i as ln Si S (i 1), then find the average of the u i s, then the find the sample variance, and with that estimate σ. ū = 1 n Σu i = 1 n lns n S 0 (112) 14
15 s 2 u = 1 n 1 Σ(u i ū) 2 (113) ˆσ = s u h (114) It s also possible to calculate the expected rate of appreciation. ˆα = ū h + δ + ˆσ2 lns(t ) lns(0) = + δ + ˆσ2 (115) 2 T 2 To test for normality, you can draw a normal probability plot. To do this arrange the data in ascending order, give the ith order statistic the quantile q = i 1 2 n, convert the q s to z s where z = N 1 (q), and plot the data. The straighter the plot, the more normal the data is. 15
16 5 Exotic Options & Simulations 5.1 Asian Options Asian options are options that are based on averages in place of either the price or the strike. The average can be either an arithmetic average or a geometric average. A(T ) = 1 ΣS(ih) (116) n G(T ) = [ΠS(ih)] 1 n (117) Then to price the option replace either the strike or the price with the appropriate path-dependent average, calculate the payoffs, and then discount them. 5.2 Barrier Options Barrier options are options that become activated (knocked-in) or deactivated (knocked-out) if the stock price passes above or below a pre-determined barrier. The price of these options can either be calculated with a binomial model or with a parity equation. Knock-in option + Knock-out option = Ordinary Option. 5.3 Compound Options Compound options are a pain in the butt to price and because of this the actuarial exam only asks that compound options be priced using the parity formula. Call on call + Put on call = Big Call - Ke rt. And similarly for puts. 5.4 Gap Options Gap options are options whose strike for determining if the option is exercised are different than the strike used to determine the payoff of the option. The formulas don t need to be written as long as the original Black-Scholes formulas are understood. The strike used to calculate the value of d 1 and d 2 is the strike that determines if the option is exercised and the strike used to calculate the price of the option is the strike that determines the payoff. 5.5 Exchange Options Exchange options can be priced using the following parity and duality equations. c[s(t), Q(t), K, t, T ] = Kp[S(t), Q(t), 1, t, T ] (118) K p[s(t), Q(t), K, t, T ] = Kc[S(t), Q(t), 1, t, T ] (119) K c[s(t), Q(t), K, t, T ] p[s(t), Q(t), K, t, T ] = F P t,t (S) KF P t,t (Q) (120) 16
17 In the above equations, S is the underlying asset and Q is the strike asset. Exchange options can also be priced using a formula very similar to the prepaid forward version of the Black-Scholes pricing equation. For example, the price of the option to get one unit of S in exchange for K units of Q can be written F P t,t (S)N(d 1 ) KF P t,t (Q)N(d 2 ) (121) d 1 = ln F P t,t (S) KF P t,t (Q) σ2 (T t) σ T t (122) d 2 = d 1 σ T t (123) σ 2 = σ 2 S + σ 2 Q 2ρσ S σ Q (124) Exchange options can be trivially applied to options that depend on a maximum or minimum of S or Q. The parity relationship is useful to know. max[s(t ), KQ(T )] + min[s(t ), KQ(T )] = F P t,t (S) + KF P t,t (Q) (125) 5.6 Monte-Carlo Simulations The way we re going to simulate stocks is by taking advantage of the lognormality of stock prices. We ll use the following method: start with iid uniform numbers u 1, u 2,..., u n, calculate z s where z i = N 1 (u 1 ), convert these to N(µ, σ 2 ) random variables by letting r 1 = µ + σz 1. To simulate a single stock price the following formula can be used. σ2 (α δ S(T ) = S(0)e 2 )T +σ T z (126) Or simulate the the stock price at some time T given the stock price at a closer future time t. σ2 (α δ S(T ) = S(t)e 2 )(T t)+σ[z(t ) Z(t)] (127) Monte-Carlo simulation goes like this: simulate stock prices, calculate the payoff of the option for each of those simulated prices, find the average payoff, and then discount the average payoff. The variance of the Monte-Carlo estimate, where g i is the ith simulated payoff, can be calculated. The variance is e 2rT s2 n where 5.7 Variance Reduction s 2 = 1 n 1 Σ[g(S i) ḡ] 2 (128) The first method of variance reduction is stratified sampling. To do this take the iid uniform numbers and instead of taking them as is make sure the correct number go into each group. As an example, given 20 variables re-define the first five to be distributed between 0 and.25 and then next five to be distributed between.25 and.5 and so on. Then proceed as before. 17
18 The other common technique involves finding an antithetic variate. To find this estimate use the normal distribution numbers as before to find V 1 and then flip the signs on all of them and do the process again to find V 2. Calculate the antithetic variate to be V 3 = V1+V
19 6 Interest Rate Trees 6.1 Bonds and Interest Rates Recall that the price of an s-year zero is P (0, S) = 1 [1 + r(0, s)] s or e r(0,s)s (129) After much algebra abuse we arrive at the following forward bond price formula. F t,t [P (T, T + s)] = P (t, T + s) P (t, T ) Also recall that we can calculate the non-continuous annualized rate. (130) 6.2 Caplets and Caps P (t, T )[1 + r t (T, T + s)] s = P (t, T + s) (131) Just as with stock prices, trees for interest rates can be made. Interest rate caplets and caps (which are the sums of the appropriate caplets) are most easily understood by examples. The only thing to memorize is that the payoff of the caplet is [r(t, T ) K] +. To calculate the price of the caplet make a table with the following columns. Path Probability Time t 1 Payoff Time t 2 Payoff Contribution of Path Then for each path find the total contribution and sum them to find the price of the caplet. Remembe rto either discount the contributions or the total to find the price. 6.3 Black-Derman-Toy Model The BDT model is a commonly used interest rate model with the following features: r d = R h and r u = R h e 2σ1 h. And the next time step uses σ 2. The interest rates are all annual effective. Also, the way the rates are defined means that the following equality holds ruu r ud The yield volatility for period-3 is = r ud r dd. 1 2 h lny u y d (132) P (r u, h, 3h) = 1 ( ) r u r uu rud (133) y u = [P (r u, h, 3h)] 1 2h 1 (134) 19
20 6.4 The Black Formula The Black formula is similar to the prepaid forward version of the Black-Scholes formula except that it uses forward prices. c(s(0), K, T ) = P (0, t)[f 0,T (S)N(d 1 ) KN(d 2 )] (135) p(s(0), K, T ) = P (0, t)[kn( d 2 ) F 0,T (S)N( d 1 )] (136) d 1 = ln F 0,T (S) K σ T + σ2 T The Black Model for Bond Options There is a put-call parity equation for zeros. (137) d 2 = d 1 σ T (138) c(k, T ) p(k, T ) = P (0, T + s) KP (0, T ) (139) We can further specify the Black Formula for Bond Options. c(s(0), K, T ) = P (0, t)[f )N(d 1 ) KN(d 2 )] (140) p(s(0), K, T ) = P (0, t)[kn( d 2 ) F N( d 1 )] (141) F = F 0,T [P (T, T + s)] (142) d 1 = ln F K + σ 2 T 2 σ T (143) d 2 = d 1 σ T (144) σ 2 = 1 V ar[lnp (T, T + s)] (145) T And we can further specify the Black Formula for Caplets as well. ( ) N( d2 ) caplet(k, T, T + s) = P (0, T ) 1 + K F N( d 1) d 1 = F = P (0, T + s) P (0, T ) σ ln[f (1 + K)] + 2 T 2 σ T (146) (147) (148) d 2 = d 1 σ T (149) 20
21 6.6 Interest Rate Models We ve been assuming that the interest rates are known. Now we will model them instead and see how this affects our other models. dr(t) = a(r(t))dt + σ(r(t))dz(t) (150) P (t, T ) = e T t r(s)ds And the dynamics of a derivative on a non-dividend paying stock are now dv (r(t), t) V (r(t), t) And so the Sharpe Ratio is (151) = α(r(t), t, T )dt q(r(t), t, T )dz(t) (152) α(r(t), t, T ) r(t) φ(r(t), t) q(r(t), t, T ) (153) And the following hedging rules apply. If you own one interest rate derivative V 1 buy N of V 2 N = q 1(r(t), t, T 1 )V 1 (r(t), t) q 2 (r(t), t, T 2 )V 2 (r(t), t) (154) 6.7 The Term Structure Equation Using a process very similar for how we dealt with stocks and the Black-Scholes equation we derive the following equations. V t + [a(r) + σ(r)φ(r, t)]v r + [σ(r)]2 V rr = rv 2 (155) q(r(t), t, T ) = σ(r(t))v r(r(t), t) = Ωσ V (r(t), t) (156) 6.8 Risk Neutral Valuation Again, the thought process is similar. The formulas are adjusted appropriately, but look the same. dr(t) = [a(r) + σ(r)φ(r(t), t)]dt + σ(r)d Zt (157) Z(t) = Z(t) t 0 φ(r(s), s)ds (158) d Z(t) = dz(t) φ(r(t), t)dt (159) 21
22 6.9 Greeks for Interest Rate Derivatives The greeks all work the same and lead to the same approximation formulas. Some bonds do follow what s called an affine structure. A bond has an affine structure if it has a certain pricing formula. P (r, t, T ) = A(t, T )e rb(t,t ) (160) The greeks for interest rate derivatives under an affine structure are easy to calculate Rendleman-Bartter This is just a straightforward GBM. = B(t, T )P (r, t, T ) (161) Γ = [B(t, T )] 2 P (r, t, T ) (162) q(r, t, T ) = σ(r)b(t, T ) (163) dr(t) = ar(t)dt + σr(t)dz(t) (164) r(t) = r(0)e ( a σ2 2 )t+σz(t) (165) 6.11 Vasicek This is an Ornstein-Uhlenback process. dr(t) = a[b r(t)]dt + σdz(t) (166) r(t) = b + [r(0) b]e at + σ t This model has some nice formulas about its bond prices. 0 e a(t s) dz(s) (167) P (r, t, T ) = A(T t)e rb(t t) (168) r = b + σφ a σ2 2a 2 (169) t) 1 e a(t B(T t) = a (170) q(r, t, T ) = σb(t t) (171) dr = [a(b r)] + σφ]dt + σd Z = a(b r)dt + σd Z (172) b = b + σφ a (173) y(r, t, T ) = 1 ln[p (r, t, T )] (174) T t 22
23 6.12 Cox-Ingersoll-Ross The short rate process and other useful formulas and equations are below. dr(t) = a[b r(t)]dt + σ r(t)dz(t) (175) P (r, t, T ) = A(T t)e rb(t t) (176) γ = (α φ) 2 + 2σ 2 (177) q(r, t, T ) = σ rb(t t) (178) yield to maturity = 2ab a φ + γ (179) 23
TABLE OF CONTENTS. A. Put-Call Parity 1 B. Comparing Options with Respect to Style, Maturity, and Strike 13
 TABLE OF CONTENTS 1. McDonald 9: "Parity and Other Option Relationships" A. Put-Call Parity 1 B. Comparing Options with Respect to Style, Maturity, and Strike 13 2. McDonald 10: "Binomial Option Pricing:
TABLE OF CONTENTS 1. McDonald 9: "Parity and Other Option Relationships" A. Put-Call Parity 1 B. Comparing Options with Respect to Style, Maturity, and Strike 13 2. McDonald 10: "Binomial Option Pricing:
(1) Consider a European call option and a European put option on a nondividend-paying stock. You are given:
 (1) Consider a European call option and a European put option on a nondividend-paying stock. You are given: (i) The current price of the stock is $60. (ii) The call option currently sells for $0.15 more
(1) Consider a European call option and a European put option on a nondividend-paying stock. You are given: (i) The current price of the stock is $60. (ii) The call option currently sells for $0.15 more
ACTS 4302 SOLUTION TO MIDTERM EXAM Derivatives Markets, Chapters 9, 10, 11, 12, 18. October 21, 2010 (Thurs)
 Problem ACTS 4302 SOLUTION TO MIDTERM EXAM Derivatives Markets, Chapters 9, 0,, 2, 8. October 2, 200 (Thurs) (i) The current exchange rate is 0.0$/. (ii) A four-year dollar-denominated European put option
Problem ACTS 4302 SOLUTION TO MIDTERM EXAM Derivatives Markets, Chapters 9, 0,, 2, 8. October 2, 200 (Thurs) (i) The current exchange rate is 0.0$/. (ii) A four-year dollar-denominated European put option
The Black-Scholes Formula
 FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
FIN-40008 FINANCIAL INSTRUMENTS SPRING 2008 The Black-Scholes Formula These notes examine the Black-Scholes formula for European options. The Black-Scholes formula are complex as they are based on the
Exam MFE Spring 2007 FINAL ANSWER KEY 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D
 Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
Exam MFE Spring 2007 FINAL ANSWER KEY Question # Answer 1 B 2 A 3 C 4 E 5 D 6 C 7 E 8 C 9 A 10 B 11 D 12 A 13 E 14 E 15 C 16 D 17 B 18 A 19 D **BEGINNING OF EXAMINATION** ACTUARIAL MODELS FINANCIAL ECONOMICS
Lectures. Sergei Fedotov. 20912 - Introduction to Financial Mathematics. No tutorials in the first week
 Lectures Sergei Fedotov 20912 - Introduction to Financial Mathematics No tutorials in the first week Sergei Fedotov (University of Manchester) 20912 2010 1 / 1 Lecture 1 1 Introduction Elementary economics
Lectures Sergei Fedotov 20912 - Introduction to Financial Mathematics No tutorials in the first week Sergei Fedotov (University of Manchester) 20912 2010 1 / 1 Lecture 1 1 Introduction Elementary economics
Exam MFE/3F Sample Questions and Solutions #1 to #76
 Exam MFE/3F Sample Questions and Solutions #1 to #76 In this version, standard normal distribution values are obtained by using the Cumulative Normal Distribution Calculator and Inverse CDF Calculator
Exam MFE/3F Sample Questions and Solutions #1 to #76 In this version, standard normal distribution values are obtained by using the Cumulative Normal Distribution Calculator and Inverse CDF Calculator
Exam MFE/Exam 3F Review
 Exam MFE/Exam 3F Review Johnew Zhang April 22, 2012 Contents 1 Put-Call Parity 4 1.1 Review....................................... 4 1.1.1 Forwards................................. 4 1.1.2 Call and put
Exam MFE/Exam 3F Review Johnew Zhang April 22, 2012 Contents 1 Put-Call Parity 4 1.1 Review....................................... 4 1.1.1 Forwards................................. 4 1.1.2 Call and put
Institutional Finance 08: Dynamic Arbitrage to Replicate Non-linear Payoffs. Binomial Option Pricing: Basics (Chapter 10 of McDonald)
 Copyright 2003 Pearson Education, Inc. Slide 08-1 Institutional Finance 08: Dynamic Arbitrage to Replicate Non-linear Payoffs Binomial Option Pricing: Basics (Chapter 10 of McDonald) Originally prepared
Copyright 2003 Pearson Education, Inc. Slide 08-1 Institutional Finance 08: Dynamic Arbitrage to Replicate Non-linear Payoffs Binomial Option Pricing: Basics (Chapter 10 of McDonald) Originally prepared
Financial Options: Pricing and Hedging
 Financial Options: Pricing and Hedging Diagrams Debt Equity Value of Firm s Assets T Value of Firm s Assets T Valuation of distressed debt and equity-linked securities requires an understanding of financial
Financial Options: Pricing and Hedging Diagrams Debt Equity Value of Firm s Assets T Value of Firm s Assets T Valuation of distressed debt and equity-linked securities requires an understanding of financial
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price
 On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
On Black-Scholes Equation, Black- Scholes Formula and Binary Option Price Abstract: Chi Gao 12/15/2013 I. Black-Scholes Equation is derived using two methods: (1) risk-neutral measure; (2) - hedge. II.
Option Valuation. Chapter 21
 Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Option Valuation Chapter 21 Intrinsic and Time Value intrinsic value of in-the-money options = the payoff that could be obtained from the immediate exercise of the option for a call option: stock price
Black-Scholes and the Volatility Surface
 IEOR E4707: Financial Engineering: Continuous-Time Models Fall 2009 c 2009 by Martin Haugh Black-Scholes and the Volatility Surface When we studied discrete-time models we used martingale pricing to derive
IEOR E4707: Financial Engineering: Continuous-Time Models Fall 2009 c 2009 by Martin Haugh Black-Scholes and the Volatility Surface When we studied discrete-time models we used martingale pricing to derive
Jorge Cruz Lopez - Bus 316: Derivative Securities. Week 11. The Black-Scholes Model: Hull, Ch. 13.
 Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
Week 11 The Black-Scholes Model: Hull, Ch. 13. 1 The Black-Scholes Model Objective: To show how the Black-Scholes formula is derived and how it can be used to value options. 2 The Black-Scholes Model 1.
S 1 S 2. Options and Other Derivatives
 Options and Other Derivatives The One-Period Model The previous chapter introduced the following two methods: Replicate the option payoffs with known securities, and calculate the price of the replicating
Options and Other Derivatives The One-Period Model The previous chapter introduced the following two methods: Replicate the option payoffs with known securities, and calculate the price of the replicating
Option Values. Determinants of Call Option Values. CHAPTER 16 Option Valuation. Figure 16.1 Call Option Value Before Expiration
 CHAPTER 16 Option Valuation 16.1 OPTION VALUATION: INTRODUCTION Option Values Intrinsic value - profit that could be made if the option was immediately exercised Call: stock price - exercise price Put:
CHAPTER 16 Option Valuation 16.1 OPTION VALUATION: INTRODUCTION Option Values Intrinsic value - profit that could be made if the option was immediately exercised Call: stock price - exercise price Put:
Lecture 21 Options Pricing
 Lecture 21 Options Pricing Readings BM, chapter 20 Reader, Lecture 21 M. Spiegel and R. Stanton, 2000 1 Outline Last lecture: Examples of options Derivatives and risk (mis)management Replication and Put-call
Lecture 21 Options Pricing Readings BM, chapter 20 Reader, Lecture 21 M. Spiegel and R. Stanton, 2000 1 Outline Last lecture: Examples of options Derivatives and risk (mis)management Replication and Put-call
第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model
 1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
1 第 9 讲 : 股 票 期 权 定 价 : B-S 模 型 Valuing Stock Options: The Black-Scholes Model Outline 有 关 股 价 的 假 设 The B-S Model 隐 性 波 动 性 Implied Volatility 红 利 与 期 权 定 价 Dividends and Option Pricing 美 式 期 权 定 价 American
Chapter 11 Options. Main Issues. Introduction to Options. Use of Options. Properties of Option Prices. Valuation Models of Options.
 Chapter 11 Options Road Map Part A Introduction to finance. Part B Valuation of assets, given discount rates. Part C Determination of risk-adjusted discount rate. Part D Introduction to derivatives. Forwards
Chapter 11 Options Road Map Part A Introduction to finance. Part B Valuation of assets, given discount rates. Part C Determination of risk-adjusted discount rate. Part D Introduction to derivatives. Forwards
The Behavior of Bonds and Interest Rates. An Impossible Bond Pricing Model. 780 w Interest Rate Models
 780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
780 w Interest Rate Models The Behavior of Bonds and Interest Rates Before discussing how a bond market-maker would delta-hedge, we first need to specify how bonds behave. Suppose we try to model a zero-coupon
Martingale Pricing Applied to Options, Forwards and Futures
 IEOR E4706: Financial Engineering: Discrete-Time Asset Pricing Fall 2005 c 2005 by Martin Haugh Martingale Pricing Applied to Options, Forwards and Futures We now apply martingale pricing theory to the
IEOR E4706: Financial Engineering: Discrete-Time Asset Pricing Fall 2005 c 2005 by Martin Haugh Martingale Pricing Applied to Options, Forwards and Futures We now apply martingale pricing theory to the
Notes on Black-Scholes Option Pricing Formula
 . Notes on Black-Scholes Option Pricing Formula by De-Xing Guan March 2006 These notes are a brief introduction to the Black-Scholes formula, which prices the European call options. The essential reading
. Notes on Black-Scholes Option Pricing Formula by De-Xing Guan March 2006 These notes are a brief introduction to the Black-Scholes formula, which prices the European call options. The essential reading
Buy a number of shares,, and invest B in bonds. Outlay for portfolio today is S + B. Tree shows possible values one period later.
 Replicating portfolios Buy a number of shares,, and invest B in bonds. Outlay for portfolio today is S + B. Tree shows possible values one period later. S + B p 1 p us + e r B ds + e r B Choose, B so that
Replicating portfolios Buy a number of shares,, and invest B in bonds. Outlay for portfolio today is S + B. Tree shows possible values one period later. S + B p 1 p us + e r B ds + e r B Choose, B so that
Consider a European call option maturing at time T
 Lecture 10: Multi-period Model Options Black-Scholes-Merton model Prof. Markus K. Brunnermeier 1 Binomial Option Pricing Consider a European call option maturing at time T with ihstrike K: C T =max(s T
Lecture 10: Multi-period Model Options Black-Scholes-Merton model Prof. Markus K. Brunnermeier 1 Binomial Option Pricing Consider a European call option maturing at time T with ihstrike K: C T =max(s T
Options/1. Prof. Ian Giddy
 Options/1 New York University Stern School of Business Options Prof. Ian Giddy New York University Options Puts and Calls Put-Call Parity Combinations and Trading Strategies Valuation Hedging Options2
Options/1 New York University Stern School of Business Options Prof. Ian Giddy New York University Options Puts and Calls Put-Call Parity Combinations and Trading Strategies Valuation Hedging Options2
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com
 Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Four Derivations of the Black Scholes PDE by Fabrice Douglas Rouah www.frouah.com www.volopta.com In this Note we derive the Black Scholes PDE for an option V, given by @t + 1 + rs @S2 @S We derive the
Lecture 9. Sergei Fedotov. 20912 - Introduction to Financial Mathematics. Sergei Fedotov (University of Manchester) 20912 2010 1 / 8
 Lecture 9 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 8 Lecture 9 1 Risk-Neutral Valuation 2 Risk-Neutral World 3 Two-Steps Binomial
Lecture 9 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 8 Lecture 9 1 Risk-Neutral Valuation 2 Risk-Neutral World 3 Two-Steps Binomial
Binomial lattice model for stock prices
 Copyright c 2007 by Karl Sigman Binomial lattice model for stock prices Here we model the price of a stock in discrete time by a Markov chain of the recursive form S n+ S n Y n+, n 0, where the {Y i }
Copyright c 2007 by Karl Sigman Binomial lattice model for stock prices Here we model the price of a stock in discrete time by a Markov chain of the recursive form S n+ S n Y n+, n 0, where the {Y i }
Lecture 12: The Black-Scholes Model Steven Skiena. http://www.cs.sunysb.edu/ skiena
 Lecture 12: The Black-Scholes Model Steven Skiena Department of Computer Science State University of New York Stony Brook, NY 11794 4400 http://www.cs.sunysb.edu/ skiena The Black-Scholes-Merton Model
Lecture 12: The Black-Scholes Model Steven Skiena Department of Computer Science State University of New York Stony Brook, NY 11794 4400 http://www.cs.sunysb.edu/ skiena The Black-Scholes-Merton Model
Pricing Barrier Options under Local Volatility
 Abstract Pricing Barrier Options under Local Volatility Artur Sepp Mail: artursepp@hotmail.com, Web: www.hot.ee/seppar 16 November 2002 We study pricing under the local volatility. Our research is mainly
Abstract Pricing Barrier Options under Local Volatility Artur Sepp Mail: artursepp@hotmail.com, Web: www.hot.ee/seppar 16 November 2002 We study pricing under the local volatility. Our research is mainly
Black-Scholes Equation for Option Pricing
 Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
Black-Scholes Equation for Option Pricing By Ivan Karmazin, Jiacong Li 1. Introduction In early 1970s, Black, Scholes and Merton achieved a major breakthrough in pricing of European stock options and there
An Introduction to Exotic Options
 An Introduction to Exotic Options Jeff Casey Jeff Casey is entering his final semester of undergraduate studies at Ball State University. He is majoring in Financial Mathematics and has been a math tutor
An Introduction to Exotic Options Jeff Casey Jeff Casey is entering his final semester of undergraduate studies at Ball State University. He is majoring in Financial Mathematics and has been a math tutor
UCLA Anderson School of Management Daniel Andrei, Derivative Markets 237D, Winter 2014. MFE Midterm. February 2014. Date:
 UCLA Anderson School of Management Daniel Andrei, Derivative Markets 237D, Winter 2014 MFE Midterm February 2014 Date: Your Name: Your Equiz.me email address: Your Signature: 1 This exam is open book,
UCLA Anderson School of Management Daniel Andrei, Derivative Markets 237D, Winter 2014 MFE Midterm February 2014 Date: Your Name: Your Equiz.me email address: Your Signature: 1 This exam is open book,
CS 522 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options
 CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
CS 5 Computational Tools and Methods in Finance Robert Jarrow Lecture 1: Equity Options 1. Definitions Equity. The common stock of a corporation. Traded on organized exchanges (NYSE, AMEX, NASDAQ). A common
Introduction to Options. Derivatives
 Introduction to Options Econ 422: Investment, Capital & Finance University of Washington Summer 2010 August 18, 2010 Derivatives A derivative is a security whose payoff or value depends on (is derived
Introduction to Options Econ 422: Investment, Capital & Finance University of Washington Summer 2010 August 18, 2010 Derivatives A derivative is a security whose payoff or value depends on (is derived
Lecture 11: The Greeks and Risk Management
 Lecture 11: The Greeks and Risk Management This lecture studies market risk management from the perspective of an options trader. First, we show how to describe the risk characteristics of derivatives.
Lecture 11: The Greeks and Risk Management This lecture studies market risk management from the perspective of an options trader. First, we show how to describe the risk characteristics of derivatives.
Lecture 6: Option Pricing Using a One-step Binomial Tree. Friday, September 14, 12
 Lecture 6: Option Pricing Using a One-step Binomial Tree An over-simplified model with surprisingly general extensions a single time step from 0 to T two types of traded securities: stock S and a bond
Lecture 6: Option Pricing Using a One-step Binomial Tree An over-simplified model with surprisingly general extensions a single time step from 0 to T two types of traded securities: stock S and a bond
Review of Basic Options Concepts and Terminology
 Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
Review of Basic Options Concepts and Terminology March 24, 2005 1 Introduction The purchase of an options contract gives the buyer the right to buy call options contract or sell put options contract some
The Binomial Option Pricing Model André Farber
 1 Solvay Business School Université Libre de Bruxelles The Binomial Option Pricing Model André Farber January 2002 Consider a non-dividend paying stock whose price is initially S 0. Divide time into small
1 Solvay Business School Université Libre de Bruxelles The Binomial Option Pricing Model André Farber January 2002 Consider a non-dividend paying stock whose price is initially S 0. Divide time into small
On Market-Making and Delta-Hedging
 On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing On Market-Making and Delta-Hedging 1 Market Makers 2 Market-Making and Bond-Pricing What to market makers do? Provide
Valuing Stock Options: The Black-Scholes-Merton Model. Chapter 13
 Valuing Stock Options: The Black-Scholes-Merton Model Chapter 13 Fundamentals of Futures and Options Markets, 8th Ed, Ch 13, Copyright John C. Hull 2013 1 The Black-Scholes-Merton Random Walk Assumption
Valuing Stock Options: The Black-Scholes-Merton Model Chapter 13 Fundamentals of Futures and Options Markets, 8th Ed, Ch 13, Copyright John C. Hull 2013 1 The Black-Scholes-Merton Random Walk Assumption
Option pricing. Vinod Kothari
 Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
Option pricing Vinod Kothari Notation we use this Chapter will be as follows: S o : Price of the share at time 0 S T : Price of the share at time T T : time to maturity of the option r : risk free rate
The Black-Scholes-Merton Approach to Pricing Options
 he Black-Scholes-Merton Approach to Pricing Options Paul J Atzberger Comments should be sent to: atzberg@mathucsbedu Introduction In this article we shall discuss the Black-Scholes-Merton approach to determining
he Black-Scholes-Merton Approach to Pricing Options Paul J Atzberger Comments should be sent to: atzberg@mathucsbedu Introduction In this article we shall discuss the Black-Scholes-Merton approach to determining
Chapter 21 Valuing Options
 Chapter 21 Valuing Options Multiple Choice Questions 1. Relative to the underlying stock, a call option always has: A) A higher beta and a higher standard deviation of return B) A lower beta and a higher
Chapter 21 Valuing Options Multiple Choice Questions 1. Relative to the underlying stock, a call option always has: A) A higher beta and a higher standard deviation of return B) A lower beta and a higher
Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008
 : A Stern School of Business New York University Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008 Outline 1 2 3 4 5 6 se notes review the principles underlying option pricing and some of
: A Stern School of Business New York University Invesco Great Wall Fund Management Co. Shenzhen: June 14, 2008 Outline 1 2 3 4 5 6 se notes review the principles underlying option pricing and some of
Digital Options. and d 1 = d 2 + σ τ, P int = e rτ[ KN( d 2) FN( d 1) ], with d 2 = ln(f/k) σ2 τ/2
![Digital Options. and d 1 = d 2 + σ τ, P int = e rτ[ KN( d 2) FN( d 1) ], with d 2 = ln(f/k) σ2 τ/2 Digital Options. and d 1 = d 2 + σ τ, P int = e rτ[ KN( d 2) FN( d 1) ], with d 2 = ln(f/k) σ2 τ/2](/thumbs/37/17740647.jpg) Digital Options The manager of a proprietary hedge fund studied the German yield curve and noticed that it used to be quite steep. At the time of the study, the overnight rate was approximately 3%. The
Digital Options The manager of a proprietary hedge fund studied the German yield curve and noticed that it used to be quite steep. At the time of the study, the overnight rate was approximately 3%. The
Options 1 OPTIONS. Introduction
 Options 1 OPTIONS Introduction A derivative is a financial instrument whose value is derived from the value of some underlying asset. A call option gives one the right to buy an asset at the exercise or
Options 1 OPTIONS Introduction A derivative is a financial instrument whose value is derived from the value of some underlying asset. A call option gives one the right to buy an asset at the exercise or
Jorge Cruz Lopez - Bus 316: Derivative Securities. Week 9. Binomial Trees : Hull, Ch. 12.
 Week 9 Binomial Trees : Hull, Ch. 12. 1 Binomial Trees Objective: To explain how the binomial model can be used to price options. 2 Binomial Trees 1. Introduction. 2. One Step Binomial Model. 3. Risk Neutral
Week 9 Binomial Trees : Hull, Ch. 12. 1 Binomial Trees Objective: To explain how the binomial model can be used to price options. 2 Binomial Trees 1. Introduction. 2. One Step Binomial Model. 3. Risk Neutral
Option Properties. Liuren Wu. Zicklin School of Business, Baruch College. Options Markets. (Hull chapter: 9)
 Option Properties Liuren Wu Zicklin School of Business, Baruch College Options Markets (Hull chapter: 9) Liuren Wu (Baruch) Option Properties Options Markets 1 / 17 Notation c: European call option price.
Option Properties Liuren Wu Zicklin School of Business, Baruch College Options Markets (Hull chapter: 9) Liuren Wu (Baruch) Option Properties Options Markets 1 / 17 Notation c: European call option price.
1 The Black-Scholes model: extensions and hedging
 1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
1 The Black-Scholes model: extensions and hedging 1.1 Dividends Since we are now in a continuous time framework the dividend paid out at time t (or t ) is given by dd t = D t D t, where as before D denotes
Black-Scholes. Ser-Huang Poon. September 29, 2008
 Black-Scholes Ser-Huang Poon September 29, 2008 A European style call (put) option is a right, but not an obligation, to purchase (sell) an asset at a strike price on option maturity date, T. An American
Black-Scholes Ser-Huang Poon September 29, 2008 A European style call (put) option is a right, but not an obligation, to purchase (sell) an asset at a strike price on option maturity date, T. An American
Option Pricing with S+FinMetrics. PETER FULEKY Department of Economics University of Washington
 Option Pricing with S+FinMetrics PETER FULEKY Department of Economics University of Washington August 27, 2007 Contents 1 Introduction 3 1.1 Terminology.............................. 3 1.2 Option Positions...........................
Option Pricing with S+FinMetrics PETER FULEKY Department of Economics University of Washington August 27, 2007 Contents 1 Introduction 3 1.1 Terminology.............................. 3 1.2 Option Positions...........................
More Exotic Options. 1 Barrier Options. 2 Compound Options. 3 Gap Options
 More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options Definition; Some types The payoff of a Barrier option is path
More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options More Exotic Options 1 Barrier Options 2 Compound Options 3 Gap Options Definition; Some types The payoff of a Barrier option is path
Options: Valuation and (No) Arbitrage
 Prof. Alex Shapiro Lecture Notes 15 Options: Valuation and (No) Arbitrage I. Readings and Suggested Practice Problems II. Introduction: Objectives and Notation III. No Arbitrage Pricing Bound IV. The Binomial
Prof. Alex Shapiro Lecture Notes 15 Options: Valuation and (No) Arbitrage I. Readings and Suggested Practice Problems II. Introduction: Objectives and Notation III. No Arbitrage Pricing Bound IV. The Binomial
Additional questions for chapter 4
 Additional questions for chapter 4 1. A stock price is currently $ 1. Over the next two six-month periods it is expected to go up by 1% or go down by 1%. The risk-free interest rate is 8% per annum with
Additional questions for chapter 4 1. A stock price is currently $ 1. Over the next two six-month periods it is expected to go up by 1% or go down by 1%. The risk-free interest rate is 8% per annum with
Finance 436 Futures and Options Review Notes for Final Exam. Chapter 9
 Finance 436 Futures and Options Review Notes for Final Exam Chapter 9 1. Options: call options vs. put options, American options vs. European options 2. Characteristics: option premium, option type, underlying
Finance 436 Futures and Options Review Notes for Final Exam Chapter 9 1. Options: call options vs. put options, American options vs. European options 2. Characteristics: option premium, option type, underlying
Black-Scholes Option Pricing Model
 Black-Scholes Option Pricing Model Nathan Coelen June 6, 22 1 Introduction Finance is one of the most rapidly changing and fastest growing areas in the corporate business world. Because of this rapid change,
Black-Scholes Option Pricing Model Nathan Coelen June 6, 22 1 Introduction Finance is one of the most rapidly changing and fastest growing areas in the corporate business world. Because of this rapid change,
Mathematical Finance
 Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
Mathematical Finance Option Pricing under the Risk-Neutral Measure Cory Barnes Department of Mathematics University of Washington June 11, 2013 Outline 1 Probability Background 2 Black Scholes for European
where N is the standard normal distribution function,
 The Black-Scholes-Merton formula (Hull 13.5 13.8) Assume S t is a geometric Brownian motion w/drift. Want market value at t = 0 of call option. European call option with expiration at time T. Payout at
The Black-Scholes-Merton formula (Hull 13.5 13.8) Assume S t is a geometric Brownian motion w/drift. Want market value at t = 0 of call option. European call option with expiration at time T. Payout at
Part V: Option Pricing Basics
 erivatives & Risk Management First Week: Part A: Option Fundamentals payoffs market microstructure Next 2 Weeks: Part B: Option Pricing fundamentals: intrinsic vs. time value, put-call parity introduction
erivatives & Risk Management First Week: Part A: Option Fundamentals payoffs market microstructure Next 2 Weeks: Part B: Option Pricing fundamentals: intrinsic vs. time value, put-call parity introduction
Bond Options, Caps and the Black Model
 Bond Options, Caps and the Black Model Black formula Recall the Black formula for pricing options on futures: C(F, K, σ, r, T, r) = Fe rt N(d 1 ) Ke rt N(d 2 ) where d 1 = 1 [ σ ln( F T K ) + 1 ] 2 σ2
Bond Options, Caps and the Black Model Black formula Recall the Black formula for pricing options on futures: C(F, K, σ, r, T, r) = Fe rt N(d 1 ) Ke rt N(d 2 ) where d 1 = 1 [ σ ln( F T K ) + 1 ] 2 σ2
IN THE DEFERRED ANNUITIES MARKET, THE PORTION OF FIXED- RATE ANNUITIES in annual
 W ORKSHOP B Y H A N G S U C K L E E Pricing Equity-Indexed Annuities Embedded with Exotic Options IN THE DEFERRED ANNUITIES MARKET, THE PORTION OF FIXED- RATE ANNUITIES in annual sales has declined from
W ORKSHOP B Y H A N G S U C K L E E Pricing Equity-Indexed Annuities Embedded with Exotic Options IN THE DEFERRED ANNUITIES MARKET, THE PORTION OF FIXED- RATE ANNUITIES in annual sales has declined from
THE BLACK-SCHOLES MODEL AND EXTENSIONS
 THE BLAC-SCHOLES MODEL AND EXTENSIONS EVAN TURNER Abstract. This paper will derive the Black-Scholes pricing model of a European option by calculating the expected value of the option. We will assume that
THE BLAC-SCHOLES MODEL AND EXTENSIONS EVAN TURNER Abstract. This paper will derive the Black-Scholes pricing model of a European option by calculating the expected value of the option. We will assume that
The Intuition Behind Option Valuation: A Teaching Note
 The Intuition Behind Option Valuation: A Teaching Note Thomas Grossman Haskayne School of Business University of Calgary Steve Powell Tuck School of Business Dartmouth College Kent L Womack Tuck School
The Intuition Behind Option Valuation: A Teaching Note Thomas Grossman Haskayne School of Business University of Calgary Steve Powell Tuck School of Business Dartmouth College Kent L Womack Tuck School
Black-Scholes. 3.1 Digital Options
 3 Black-Scholes In this chapter, we will study the value of European digital and share digital options and standard European puts and calls under the Black-Scholes assumptions. We will also explain how
3 Black-Scholes In this chapter, we will study the value of European digital and share digital options and standard European puts and calls under the Black-Scholes assumptions. We will also explain how
How To Value Options In Black-Scholes Model
 Option Pricing Basics Aswath Damodaran Aswath Damodaran 1 What is an option? An option provides the holder with the right to buy or sell a specified quantity of an underlying asset at a fixed price (called
Option Pricing Basics Aswath Damodaran Aswath Damodaran 1 What is an option? An option provides the holder with the right to buy or sell a specified quantity of an underlying asset at a fixed price (called
Call and Put. Options. American and European Options. Option Terminology. Payoffs of European Options. Different Types of Options
 Call and Put Options A call option gives its holder the right to purchase an asset for a specified price, called the strike price, on or before some specified expiration date. A put option gives its holder
Call and Put Options A call option gives its holder the right to purchase an asset for a specified price, called the strike price, on or before some specified expiration date. A put option gives its holder
Introduction to Binomial Trees
 11 C H A P T E R Introduction to Binomial Trees A useful and very popular technique for pricing an option involves constructing a binomial tree. This is a diagram that represents di erent possible paths
11 C H A P T E R Introduction to Binomial Trees A useful and very popular technique for pricing an option involves constructing a binomial tree. This is a diagram that represents di erent possible paths
American Options. An Undergraduate Introduction to Financial Mathematics. J. Robert Buchanan. J. Robert Buchanan American Options
 American Options An Undergraduate Introduction to Financial Mathematics J. Robert Buchanan 2010 Early Exercise Since American style options give the holder the same rights as European style options plus
American Options An Undergraduate Introduction to Financial Mathematics J. Robert Buchanan 2010 Early Exercise Since American style options give the holder the same rights as European style options plus
Overview. Option Basics. Options and Derivatives. Professor Lasse H. Pedersen. Option basics and option strategies
 Options and Derivatives Professor Lasse H. Pedersen Prof. Lasse H. Pedersen 1 Overview Option basics and option strategies No-arbitrage bounds on option prices Binomial option pricing Black-Scholes-Merton
Options and Derivatives Professor Lasse H. Pedersen Prof. Lasse H. Pedersen 1 Overview Option basics and option strategies No-arbitrage bounds on option prices Binomial option pricing Black-Scholes-Merton
Stock. Call. Put. Bond. Option Fundamentals
 Option Fundamentals Payoff Diagrams hese are the basic building blocks of financial engineering. hey represent the payoffs or terminal values of various investment choices. We shall assume that the maturity
Option Fundamentals Payoff Diagrams hese are the basic building blocks of financial engineering. hey represent the payoffs or terminal values of various investment choices. We shall assume that the maturity
1 Simulating Brownian motion (BM) and geometric Brownian motion (GBM)
 Copyright c 2013 by Karl Sigman 1 Simulating Brownian motion (BM) and geometric Brownian motion (GBM) For an introduction to how one can construct BM, see the Appendix at the end of these notes A stochastic
Copyright c 2013 by Karl Sigman 1 Simulating Brownian motion (BM) and geometric Brownian motion (GBM) For an introduction to how one can construct BM, see the Appendix at the end of these notes A stochastic
VALUATION IN DERIVATIVES MARKETS
 VALUATION IN DERIVATIVES MARKETS September 2005 Rawle Parris ABN AMRO Property Derivatives What is a Derivative? A contract that specifies the rights and obligations between two parties to receive or deliver
VALUATION IN DERIVATIVES MARKETS September 2005 Rawle Parris ABN AMRO Property Derivatives What is a Derivative? A contract that specifies the rights and obligations between two parties to receive or deliver
Lecture 15. Sergei Fedotov. 20912 - Introduction to Financial Mathematics. Sergei Fedotov (University of Manchester) 20912 2010 1 / 6
 Lecture 15 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 6 Lecture 15 1 Black-Scholes Equation and Replicating Portfolio 2 Static
Lecture 15 Sergei Fedotov 20912 - Introduction to Financial Mathematics Sergei Fedotov (University of Manchester) 20912 2010 1 / 6 Lecture 15 1 Black-Scholes Equation and Replicating Portfolio 2 Static
The Black-Scholes Model
 Chapter 4 The Black-Scholes Model 4. Introduction Easily the best known model of option pricing, the Black-Scholes model is also one of the most widely used models in practice. It forms the benchmark model
Chapter 4 The Black-Scholes Model 4. Introduction Easily the best known model of option pricing, the Black-Scholes model is also one of the most widely used models in practice. It forms the benchmark model
OPTIONS and FUTURES Lecture 2: Binomial Option Pricing and Call Options
 OPTIONS and FUTURES Lecture 2: Binomial Option Pricing and Call Options Philip H. Dybvig Washington University in Saint Louis binomial model replicating portfolio single period artificial (risk-neutral)
OPTIONS and FUTURES Lecture 2: Binomial Option Pricing and Call Options Philip H. Dybvig Washington University in Saint Louis binomial model replicating portfolio single period artificial (risk-neutral)
The Black-Scholes pricing formulas
 The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
The Black-Scholes pricing formulas Moty Katzman September 19, 2014 The Black-Scholes differential equation Aim: Find a formula for the price of European options on stock. Lemma 6.1: Assume that a stock
Derivatives: Principles and Practice
 Derivatives: Principles and Practice Rangarajan K. Sundaram Stern School of Business New York University New York, NY 10012 Sanjiv R. Das Leavey School of Business Santa Clara University Santa Clara, CA
Derivatives: Principles and Practice Rangarajan K. Sundaram Stern School of Business New York University New York, NY 10012 Sanjiv R. Das Leavey School of Business Santa Clara University Santa Clara, CA
Exam P - Total 23/23 - 1 -
 Exam P Learning Objectives Schools will meet 80% of the learning objectives on this examination if they can show they meet 18.4 of 23 learning objectives outlined in this table. Schools may NOT count a
Exam P Learning Objectives Schools will meet 80% of the learning objectives on this examination if they can show they meet 18.4 of 23 learning objectives outlined in this table. Schools may NOT count a
CHAPTER 3 Pricing Models for One-Asset European Options
 CHAPTER 3 Pricing Models for One-Asset European Options The revolution on trading and pricing derivative securities in financial markets and academic communities began in early 1970 s. In 1973, the Chicago
CHAPTER 3 Pricing Models for One-Asset European Options The revolution on trading and pricing derivative securities in financial markets and academic communities began in early 1970 s. In 1973, the Chicago
Path-dependent options
 Chapter 5 Path-dependent options The contracts we have seen so far are the most basic and important derivative products. In this chapter, we shall discuss some complex contracts, including barrier options,
Chapter 5 Path-dependent options The contracts we have seen so far are the most basic and important derivative products. In this chapter, we shall discuss some complex contracts, including barrier options,
A Genetic Algorithm to Price an European Put Option Using the Geometric Mean Reverting Model
 Applied Mathematical Sciences, vol 8, 14, no 143, 715-7135 HIKARI Ltd, wwwm-hikaricom http://dxdoiorg/11988/ams144644 A Genetic Algorithm to Price an European Put Option Using the Geometric Mean Reverting
Applied Mathematical Sciences, vol 8, 14, no 143, 715-7135 HIKARI Ltd, wwwm-hikaricom http://dxdoiorg/11988/ams144644 A Genetic Algorithm to Price an European Put Option Using the Geometric Mean Reverting
The interest volatility surface
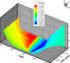 The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
The interest volatility surface David Kohlberg Kandidatuppsats i matematisk statistik Bachelor Thesis in Mathematical Statistics Kandidatuppsats 2011:7 Matematisk statistik Juni 2011 www.math.su.se Matematisk
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies
 Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Hedging Illiquid FX Options: An Empirical Analysis of Alternative Hedging Strategies Drazen Pesjak Supervised by A.A. Tsvetkov 1, D. Posthuma 2 and S.A. Borovkova 3 MSc. Thesis Finance HONOURS TRACK Quantitative
Monte Carlo Methods in Finance
 Author: Yiyang Yang Advisor: Pr. Xiaolin Li, Pr. Zari Rachev Department of Applied Mathematics and Statistics State University of New York at Stony Brook October 2, 2012 Outline Introduction 1 Introduction
Author: Yiyang Yang Advisor: Pr. Xiaolin Li, Pr. Zari Rachev Department of Applied Mathematics and Statistics State University of New York at Stony Brook October 2, 2012 Outline Introduction 1 Introduction
Options Pricing. This is sometimes referred to as the intrinsic value of the option.
 Options Pricing We will use the example of a call option in discussing the pricing issue. Later, we will turn our attention to the Put-Call Parity Relationship. I. Preliminary Material Recall the payoff
Options Pricing We will use the example of a call option in discussing the pricing issue. Later, we will turn our attention to the Put-Call Parity Relationship. I. Preliminary Material Recall the payoff
Chapter 14 Review Note Sample Excerpt
 Chapter 14 Review Note Sample Excerpt Exotic Options: I Derivatives Markets (2 nd Edition) Online Excerpt of Section 14.5 with hree Questions and Solutions Introduction his document provides a sample excerpt
Chapter 14 Review Note Sample Excerpt Exotic Options: I Derivatives Markets (2 nd Edition) Online Excerpt of Section 14.5 with hree Questions and Solutions Introduction his document provides a sample excerpt
Option Portfolio Modeling
 Value of Option (Total=Intrinsic+Time Euro) Option Portfolio Modeling Harry van Breen www.besttheindex.com E-mail: h.j.vanbreen@besttheindex.com Introduction The goal of this white paper is to provide
Value of Option (Total=Intrinsic+Time Euro) Option Portfolio Modeling Harry van Breen www.besttheindex.com E-mail: h.j.vanbreen@besttheindex.com Introduction The goal of this white paper is to provide
arxiv:1108.4393v2 [q-fin.pr] 25 Aug 2011
![arxiv:1108.4393v2 [q-fin.pr] 25 Aug 2011 arxiv:1108.4393v2 [q-fin.pr] 25 Aug 2011](/thumbs/25/5675369.jpg) arxiv:1108.4393v2 [q-fin.pr] 25 Aug 2011 Pricing Variable Annuity Contracts with High-Water Mark Feature V.M. Belyaev Allianz Investment Management, Allianz Life Minneapolis, MN, USA August 26, 2011 Abstract
arxiv:1108.4393v2 [q-fin.pr] 25 Aug 2011 Pricing Variable Annuity Contracts with High-Water Mark Feature V.M. Belyaev Allianz Investment Management, Allianz Life Minneapolis, MN, USA August 26, 2011 Abstract
7: The CRR Market Model
 Ben Goldys and Marek Rutkowski School of Mathematics and Statistics University of Sydney MATH3075/3975 Financial Mathematics Semester 2, 2015 Outline We will examine the following issues: 1 The Cox-Ross-Rubinstein
Ben Goldys and Marek Rutkowski School of Mathematics and Statistics University of Sydney MATH3075/3975 Financial Mathematics Semester 2, 2015 Outline We will examine the following issues: 1 The Cox-Ross-Rubinstein
CURRENCY OPTION PRICING II
 Jones Grauate School Rice University Masa Watanabe INTERNATIONAL FINANCE MGMT 657 Calibrating the Binomial Tree to Volatility Black-Scholes Moel for Currency Options Properties of the BS Moel Option Sensitivity
Jones Grauate School Rice University Masa Watanabe INTERNATIONAL FINANCE MGMT 657 Calibrating the Binomial Tree to Volatility Black-Scholes Moel for Currency Options Properties of the BS Moel Option Sensitivity
How To Value Real Options
 FIN 673 Pricing Real Options Professor Robert B.H. Hauswald Kogod School of Business, AU From Financial to Real Options Option pricing: a reminder messy and intuitive: lattices (trees) elegant and mysterious:
FIN 673 Pricing Real Options Professor Robert B.H. Hauswald Kogod School of Business, AU From Financial to Real Options Option pricing: a reminder messy and intuitive: lattices (trees) elegant and mysterious:
Currency Options (2): Hedging and Valuation
 Overview Chapter 9 (2): Hedging and Overview Overview The Replication Approach The Hedging Approach The Risk-adjusted Probabilities Notation Discussion Binomial Option Pricing Backward Pricing, Dynamic
Overview Chapter 9 (2): Hedging and Overview Overview The Replication Approach The Hedging Approach The Risk-adjusted Probabilities Notation Discussion Binomial Option Pricing Backward Pricing, Dynamic
Chapter 13 The Black-Scholes-Merton Model
 Chapter 13 The Black-Scholes-Merton Model March 3, 009 13.1. The Black-Scholes option pricing model assumes that the probability distribution of the stock price in one year(or at any other future time)
Chapter 13 The Black-Scholes-Merton Model March 3, 009 13.1. The Black-Scholes option pricing model assumes that the probability distribution of the stock price in one year(or at any other future time)
LECTURE 15: AMERICAN OPTIONS
 LECTURE 15: AMERICAN OPTIONS 1. Introduction All of the options that we have considered thus far have been of the European variety: exercise is permitted only at the termination of the contract. These
LECTURE 15: AMERICAN OPTIONS 1. Introduction All of the options that we have considered thus far have been of the European variety: exercise is permitted only at the termination of the contract. These
QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS
 QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS L. M. Dieng ( Department of Physics, CUNY/BCC, New York, New York) Abstract: In this work, we expand the idea of Samuelson[3] and Shepp[,5,6] for
QUANTIZED INTEREST RATE AT THE MONEY FOR AMERICAN OPTIONS L. M. Dieng ( Department of Physics, CUNY/BCC, New York, New York) Abstract: In this work, we expand the idea of Samuelson[3] and Shepp[,5,6] for
Lecture 4: The Black-Scholes model
 OPTIONS and FUTURES Lecture 4: The Black-Scholes model Philip H. Dybvig Washington University in Saint Louis Black-Scholes option pricing model Lognormal price process Call price Put price Using Black-Scholes
OPTIONS and FUTURES Lecture 4: The Black-Scholes model Philip H. Dybvig Washington University in Saint Louis Black-Scholes option pricing model Lognormal price process Call price Put price Using Black-Scholes
STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 STAT2400&3400 STAT2400&3400 STAT2400&3400 STAT2400&3400 STAT3400 STAT3400
 Exam P Learning Objectives All 23 learning objectives are covered. General Probability STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 1. Set functions including set notation and basic elements
Exam P Learning Objectives All 23 learning objectives are covered. General Probability STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 STAT2400 1. Set functions including set notation and basic elements
EXP 481 -- Capital Markets Option Pricing. Options: Definitions. Arbitrage Restrictions on Call Prices. Arbitrage Restrictions on Call Prices 1) C > 0
 EXP 481 -- Capital Markets Option Pricing imple arbitrage relations Payoffs to call options Black-choles model Put-Call Parity Implied Volatility Options: Definitions A call option gives the buyer the
EXP 481 -- Capital Markets Option Pricing imple arbitrage relations Payoffs to call options Black-choles model Put-Call Parity Implied Volatility Options: Definitions A call option gives the buyer the
