ECO 317 Economics of Uncertainty Fall Term 2009 Notes for lectures 2. The Expected Utility Theory of Choice Under Risk
|
|
|
- Miranda Hill
- 7 years ago
- Views:
Transcription
1 ECO 7 Economics of Uncertainty Fall Term 2009 Notes for lectures 2. The Expected Utility Theory of Choice Under Risk Basic Concepts The individual (usually consumer, investor, or firm) chooses action denoted by a, from a set of feasible actions A. The set of states of the world is denoted by S, and probabilities are defined for events in S. et π(s) denote the probability of the elementary event s. Here we take these probabilities to be exogenous. Actions can lead to different consequences in different states of the world. et C denote the set of consequences, and F : A S C denote the consequence function, so c = F (a, s) is the consequence of action a in state s. For example, an investor s action might be a vector of proportions of the initial wealth that he puts into different kinds of assets. Then, depending upon the state of the world, e.g. interest rates rise and the dollar goes down, so bonds do badly but foreign stocks do well in dollar terms, there will be a consequence, namely his final wealth. First suppose C is finite, say C = {c, c 2,... c n }, and write p i (a) for the probability that action a yields consequence c i, that is, p i (a) = r { s F (a, s) = c i }. Of course p i (a) may equal zero for some i. In many economic applications, the set of consequences C is an infinite continuum of consumption quantities, or amounts of income or wealth. But the same idea has the obvious generalization. Each action induces a cumulative distribution function or a density function on C, e.g. we can define p(w; a) such that p(w; a) dw is the probability that the action a yields wealth between w and w + dw. We will usually develop the theory for consequence sets that are finite, but use it in the more general contexts without going into the rigorous (pedantic?) math of this. Thus each action induces a probability distribution over C. Call such a probability distribution a lottery. The set of feasible actions will map into a set of feasible lotteries. Different consequence functions may yield equivalent lotteries. Example: The state space is the set of possible outcomes of the roll of a die: {, 2,, 4, 5, 6}. The consequence space is a set of prizes, {$,$2,$}. The actions are bets. Bet, labeled a, yields $ if the die shows, 2, or, $2 if it shows 4 or 5, and $ if it shows 6. Bet 2, labeled a 2, yields $ if the die shows an even number, $2 if it shows or 5, and $ if it shows. Then the two actions induce the same lottery, namely: the probability of getting $ is /2, that of getting $2 is /, and that of getting $ is /6. In orthodox economic theory (we will consider some newer ideas such as framing and reference points later), a decision maker cares only about consequences and their probabilities. Therefore we can think of the choice problem as one of choosing among a set of feasible lotteries.
2 We will denote a lottery by a letter symbol, usually with some label. More fully spelled out, it is a vector of probabilities of all the consequences {c, c 2,... c n } in the correct order: (p, p 2,... p n ). Some of the p i may be zero, and they sum to : p i 0 for all i; n i= p i =. Therefore we have a geometric representation: a lottery = (p, p 2,... p n ) can be represented by a point on the unit simplex in n-dimensional space. If many of the p i in a lottery are zero, it may be simpler to write the lottery by listing the consequences that have non-zero probabilities, along with those probabilities, for example as = (c, c, c 7 ; p, p, p 7 ), where now p, p, and p 7 are positive and sum to. Much of the theory can be illustrated using the case n =. Here an even simpler geometric representation, which we will call the probability triangle diagram, is available. Order the consequences so that C is the worst and C is the best for our decision-maker. In a two-dimensional diagram (Figure ), show the probability p of the worst consequence on the horizontal axis and the probability p of the best consequence on the vertical axis. The point representing the lottery then lies in (or on the boundary of) the triangle given by p 0, p 0 and p + p. The horizontal and vertical coordinates of the point show p and p as usual, whereas p 2 can be found implicitly as the horizontal (or vertical) distance of the point from the line p + p =. One of the probabilities is zero along each side of the triangle; for example along the long side (line p + p = ), we have p 2 = 0. The extreme cases of no uncertainty correspond to the vertices of the triangle; for example, the origin is the degenerate lottery with p = p = 0 so p 2 =. 2 Figure : The probability triangle diagram 2
3 2 Composite or compound lotteries These are lotteries whose prizes are themselves lotteries. Write a lottery that yields a prize consisting of lottery with probability p, a prize consisting of lottery 2 with probability p 2, etc. using a self-evident notation: Suppose each of these lotteries is simple: = {, 2... k ; p, p 2,... p k }. () j = (q j, q j 2,... q j n). (2) The probability that you will get consequence c i if you own the lottery is then given by r(c i ) = k j= r(c i j ) r( j ) = k j= q j i p j. () et us check that these probabilities sum to when i ranges from to n: i= n k q j i p j j= k = = { n } p j q j i j= i= k k j= p j = j= p j =. Making such checks is a good idea to improve one s mastery of the techniques and to avoid errors. In the future I will leave them as exercises. A general presumption of standard microeconomic theory is that people care only about what they finally get to consume, and not by the path or process by which they arrived at this consumption vector. The natural analog in the case of uncertainty is that people should care only about the essential properties of any lottery or combination of lotteries they might hold, namely the probabilities of all the consequences. Specifically, they should be indifferent between a compound lottery () consisting of the constituent simple lotteries (2) on the one hand, and a single simple lottery whose probabilities are given by () on the other hand. This is sometimes called the compound lottery axiom or the reduction of compound lotteries. This axiom will be violated if the decision-maker likes or dislikes the process by which uncertainty is resolved; for example he may enjoy the suspense that builds up as a lottery yields a prize that is another lottery, or he may find it worrying. Some recent research considers departures of this kind from the standard theory, but we will not go into that. Given the compound lottery axiom, we can show compound lotteries in the probability triangle diagram by showing their equivalent simple lotteries. Given any two lotteries and The textbook (p. 28) considers a different kind of compounding, where additional uncertainty is added, and a risk-averse consumer dislikes this. We are leaving all the probabilities of all the consequences the same in the simple lottery as they were in the original compound lottery.
4 2, and any probability p, consider the compound lottery = {, 2 ; p, ( p)}. Write the probabilities of consequences and under j, using the above notation as (q j, q j ) for j =, 2. Then the probabilities of these consequences under are (p q + ( p) q 2, p q + ( p) q 2 ). Therefore the point in the probability triangle diagram, the point that represents is on the straight line joining (q, q ) and (q 2, q 2 ), dividing the distance in proportions p : ( p). If p is close to, then is close to ; if p is close to zero, is close to 2. -p p 2 Figure 2: Compound lottery in probability triangle diagram Indifference maps and expected utility Again proceeding under the assumption of the compound lottery axiom, the consumer s preferences can only depend on the consequences (c, c 2,... c n ) and their respective probabilities (p, p 2,... p n ). In fact, when the list of consequences is comprehensive, and we are comparing different lotteries with different probabilities, we can just focus on these probabilities. In general one might think that the preferences can be represented by a set of indifference curves, or an indifference map, in our probability triangle diagram of Figure. As usual, we can attach numbers to these indifference curves, where a curve corresponding to a higher level of preference gets a bigger number. This is a utility function, which can be written with the consequences and probabilities as its arguments: F (c, c 2,... c n ; p, p 2,... p n ). The indifference map then consists of the contours F = constant. However, such a general function is too unwieldy, and some special cases acquire special interest. The case most commonly used is one where F has the form p u(c ) + p 2 u(c 2 ) p n u(c n ). (4) 4
5 In other words, there is a utility function u defined over consequences, and a lottery is evaluated by the mathematical expectation or expected value of this utility. The underlying u function is sometimes called a Bernoulli utility function or a von Neumann-Morgenstern utility function after the pioneers of this idea, and the overall expression above (4) is called expected utility of the lottery; write it as EU(). Recall that a degenerate lottery yields only one consequence with probability ; the probabilities of all other consequences are zero for this lottery. For a degenerate lottery (6) yielding the consequence 6 with certainty, for example, expected utility is just EU( (6) ) = u(c 6 ) = u(c 6 ). So we can think of the Bernoulli utilities as the utilities of consequences, or as expected utilities of degenerate lotteries, whichever is better in any specific instance. Because the functional form of EU() in (4) is a very special case of the general function F, we cannot hope to represent any and all types of preferences over lotteries in this way. Just what restriction on preferences is implied by the expected utility form? We can see this in the probability triangle. Note that with three consequences, a contour of expected utility is given by p u(c ) + p 2 u(c 2 ) + p u(c ) = constant = k, say,, or or p u(c ) + ( p p ) u(c 2 ) + p u(c ) = k, p [u(c ) u(c 2 )] p [u(c 2 ) u(c )] = k u(c 2 ). (5) Since in the probability triangle diagram the consequences are a fixed and exhaustive list while the probabilities vary, the numbers u(c i ) are constants independent of the p i. And since we are labeling the consequences so that c is the worst and c is the best, we must have u(c ) < u(c 2 ) < u(c ). Therefore [u(c ) u(c 2 )] and [u(c 2 ) u(c )] are both positive constants. Therefore (5) is the equation of a straight line, with positive slope [u(c 2 ) u(c )]/[u(c ) u(c 2 )]. This slope is the same for all indifference curves (independent of the constant k); therefore the indifference map must be a family of parallel straight lines. And since expected utility must increase when the probability of the best consequence increases and/or the probability of the worst consequence falls, expected utility must increase as we move to the North-West from one indifference curve to another. All this is shown in Figure.) 4 Foundations of expected utility We can also interpret the parallel indifference curve property in terms of the basic preference relationship among lotteries. This is a binary relation. For two lotteries and 2, the statement 2 means that the decision-maker regards at least as good as 2. The opposite relation is. Recall the Axioms of Rational Choice of consumer theory in the absence of uncertainty that enabled you to use a utility function in ECO 0, e.g. Nicholson s Microeconomic Theory ninth edition Chapter, pp The first is completeness, which says that the consumer can always compare any two situations. Here it means that for any two lotteries and 2, 5
6 Direction of increasing expected utility Figure : Indifference curves of expected utility at least one of 2 and 2 must be true. If both are true, then the decision-maker regards as indifferent to 2, written 2. If 2 is true but 2 is false, then is strictly preferred to 2, written 2. And the other way round. Then we have transitivity; if 2 and 2 are both true, then must be true. Finally we have continuity; if 2 and is sufficiently close to 2 (here it means the probability vectors of 2 and are sufficiently close), then it will be true that. The same three axioms will enable us to establish the existence of a general utility function like the F above. But we need another axiom to have the expected utility form (4) Figure 4: Independence axiom To find and interpret this extra condition, consider Figure 4. I have shown two lotteries 6
7 and 2 on one indifference curve and therefore indifferent to each other, and any third lottery. (In this figure is preferred to either of and 2, but that plays no role in the argument; as a useful exercise to reinforce your understanding, you should construct the figure and the argument when 2.) Now take any probability p, and consider the compound lotteries 4 = (, ; p, p), 5 = ( 2, ; p, p). (6) Recalling the procedure explained in Figure 2, 4 is represented by its equivalent simple lottery, namely the point on the straight line joining to and dividing it in proportions p : ( p), and similarly for 5 in relation to 2 and. The proportions p : ( p) being the same in the two cases, the line joining 4 to 5 must be parallel to the line joining to 2. But we took and 2 to be indifferent to each other, so the line joining them is simply an indifference curve of expected utility. But if the indifference map represents expected utility contours, then indifference curves are a family of parallel straight lines. Therefore the line joining 4 to 5 must constitute an indifference curve as well. That is, 4 and 5 must be indifferent to each other. So we have the result: If preferences can be represented by expected utility, then for any two mutually indifferent lotteries and 2, any third lottery, and any probability p, the lotteries 4 and 5 defined by (6) must be indifferent as well. A slightly different but equivalent way to put it is: If, in a compound lottery (, ; p, p) we replace by another lottery 2 that is indifferent to, the resulting compound lottery ( 2, ; p, p) is indifferent to the original compound lottery. If we move to a slightly higher indifference curve than 2, then 4 will move to a slightly higher indifference curve than 5. This gives us the following property: If preferences can be represented by expected utility, then for any two lotteries and 2 such that 2, any third lottery, and any probability p, the lotteries 4 and 5 defined by (6) must satisfy 4 5. The converse is also true. Give the name the independence axiom to the property that for any two lotteries and 2 satisfying 2, any third lottery, and any probability p, the lotteries 4 and 5 defined by (6) must satisfy 4 5. Then we have the Expected Utility Theorem: If a decision-maker s preferences satisfy the independence axiom, together with the usual properties of completeness, transitivity, and continuity, then the preferences can be represented by expected utility. The proof is complicated and I omit most of it, merely giving the construction of such a utility function in a slightly special context. This supposes that among all feasible lotteries, there is a best, say, and a worst,. If the set of all possible consequences is finite, then one need merely pick the best and the worst consequences. If the set is infinite, and open or unbounded at the extremes, then the procedure below needs to be modified. For those interested, the Herstein-Milnor article in the optional reading, or a fuller reading of those pages from Arrow s chapter which are to be skimmed in the required reading, give the complete proof. Consider any lottery, and compare it to the compound lottery p = (, ; p, p) as p ranges from 0 to. This p must surely rank higher and higher in preferences as p increases. 7
8 For p close to zero, p so our original lottery must be better than p. For p close to, p so our original lottery must be worse than p. Therefore there must be one and only one p such that p. (The difficulties of the formal proof consist entirely of making all these statements precise and rigorous as is done in real analysis or point set topology.) Define the utility of to be this number p itself. So the utility function u we are trying to construct has u() = p. We check that it has the expected utility property. First, consider all the pure consequences c i. Think of each as a degenerate lottery, and let q i be its utility according to this definition, that is q i is the number such that c i is indifferent to (, ; q i, q i ). So u(c i ) = q i. Now suppose our lottery, or its equivalent simple lottery, is expressed in terms of the consequences as (c, c 2,... c n ; p, p 2,... p n ). The degenerate lottery c is indifferent to (, ; q, q ). Using the independence axiom in its interpretation of replacing one part of a compound lottery by something indifferent to it, we see that is indifferent to the compound lottery = (,, c 2,... c n ; p q, p ( q ), p 2,... p n ). Next, c 2 is indifferent to (, ; q 2, q 2 ). By the independence axiom again, the lottery is indifferent to (,,,, c,... c n ; p q, p ( q ), p 2 q 2, p 2 ( q 2 ), p... p n ). roceeding in this way, our initial lottery is finally indifferent to (,,,,..., ; p q, p ( q ), p 2 q 2, p 2 ( q 2 ),... p n q n, p n ( q n )). By the compound lottery axiom only the eventual consequences and their probabilities matter, so we can collect this into or (, ; p q + p 2 q p n q n, p ( q ) + p 2 ( q 2 ) p n ( q n )), (, ; p q + p 2 q p n q n, (p q + p 2 q p n q n )). Therefore, by our definition of u, u() = p q + p 2 q p n q n = p u(c ) + p 2 u(c 2 ) p n u(c n ), (7) which is exactly the expected utility form. So henceforth instead of writing it as u() we will write it as EU(). 5 Cardinal utility If in (7) we replace the Bernoulli (or von Neumann-Morgenstern) utility function u of consequences by another function ũ defined by ũ(c) = a + b u(c) 8
9 for all consequences c, where a and b are constants, with b > 0, the expected utility of any lottery becomes EŨ() = p ũ(c ) + p 2 ũ(c 2 ) p n ũ(c n ) = p [a + b u(c )] + p 2 [a + b u(c 2 )] [a + b p n u(c n )] = a (p + p p n ) + b [ p u(c ) + p 2 u(c 2 ) p n u(c n ) ] = a (p + p p n ) + b [ p u(c ) + p 2 u(c 2 ) p n u(c n ) ] = a + b EU() Therefore for any two lotteries and 2, EŨ( ) > EŨ(2 ) if and only if EU( ) > EU( 2 ). Therefore EU and EŨ represent the same preferences. Thus the expected utility representation is not unique; the choice of the underlying Bernoulli utility function of consequences is arbitrary up to an increasing linear transformation. Roughly speaking, the origin and the scale of Bernoulli utility can be chosen arbitrarily. In consumer choice theory under certainty, the choice of a utility function to represent a given set of preferences was even more arbitrary, namely up to an increasing transformation, linear or non-linear. For example, Cobb-Douglas preferences could be represented equally well by either X α Y β, or α ln(x) + β ln(y ). A similar freedom to use nonlinear transformation of the Bernoulli utility function is not available under uncertainty, because the expected values of a non-linear transform is not any non-linear transform of the expected value. For example p ln(c ) + p 2 ln(c 2 ) ln( p c + p 2 c 2 ). The two correspond to different degrees of risk aversion. An intuitive explanation follows; we will develop the concept of risk aversion in more detail later. In consumer theory under certainty, only the ordering of utility numbers matters: an indifference curve higher up in the preferences should be assigned a larger number, but how much larger is immaterial and has no significance. Therefore that utility is said to be ordinal. Under uncertainty, and specifically under the expected utility theory, the size of differences in utility numbers matters, at least up to a choice of scale. For example, if the consequences are magnitudes of wealth with c < c 2 < c, then whether [u(c 2 ) u(c )] is bigger or less than [u(c ) u(c 2 )] matters for whether u(c 2 ) > or < 2 u(c ) + 2 u(c ), and therefore for whether the decision-maker would prefer to have c 2 for sure, or a 50:50 gamble between c and c. Therefore a non-linear transformation of the u(c) numbers, which could change [u(c 2 ) u(c )] and [u(c ) u(c 2 )] in quite different ways, can affect this comparison and therefore would not represent the same underlying attitude toward risk. 9
10 Since the magnitudes of utility numbers matter (up to the choice of origin and scale), the utility function that goes into expected utility calculations is said to be cardinal. Some people think that this gives utility a tangible quality, and speak of measuring utility much as one might measure the quantity of corn or steel. I think this is a mistake. Expected utility theory is an economists construct, to enable us to represent preferences numerically and therefore to facilitate calculations about choice and later about equilibrium. The utility numbers constructed in this theory don t exist out there ; there is no point in trying to attach them any physical or tangible significance. 6 imits to expected utility theory Indifference curves being parallel straight lines in the probability triangle diagram (or a higher dimensional simplex), and the corresponding property of preferences, namely independence axiom, is a restriction. Not all preferences, even fully rational ones (satisfying completeness and transitivity), need satisfy this restriction. But is it a reasonable restriction in practice? It may seem innocuous to replace one lottery by another which is indifferent to it, but in many experiments and observations actual behavior does run counter to the independence axiom. In the questionnaire posted in advance of this topic, Question 2 asked how you would choose between the lotteries A and B, where A: $2,500 with probability 0., $2,400 with probability 0.66, $0 with probability 0.0 B: $2,400 for sure Question posed the hypothetical choice between C and D, where $2,500 with probability 0., $0 with probability 0.67 $2,400 with probability 0.4, $0 with probability 0.66 as If your behavior conformed to expected utility theory, you would choose A or B according 0. u(2500) u(2400) + 0.0u(0) > or < u(2400), that is, 0. u(2500) + 0.0u(0) > or < 0.4 u(2400). In Question 6 you were asked to choose between two different lotteries: C yielding $2,500 with probability 0. and $0 with probability 0.67, and D yielding $2,400 with probability 0.4 and $0 with probability If your behavior conformed to expected utility theory, you would choose C or D according as 0. u(2500) u(0) > or < 0.4 u(2400) u(0), that is, 0. u(2500) + 0.0u(0) > or < 0.4 u(2400). The two criteria are the same! Therefore you would be choosing A in Question 5 if and only if you choose C in Question 6. Which of the choice combinations A, C or B, D you make would depend on your risk aversion, which is reflected in the function u. But regardless of 0
11 that, you would choose one of these two combinations. If you choose either A, D or B, C, then your behavior is inconsistent with expected utility theory. When I ran this experiment in the Fall 2007 class, the actual returns were: AC = 0, BC = 8, BD = 5, AD =. This is slightly better for expected utility theory than is usual in such experiments, but the sample size is too small to draw any strong conclusions. The argument that many rational decision-makers would choose BC in such situations was made by Maurice Allais, and this became known as the Allais aradox. Actually there is nothing paradoxical in the sense of irrationality. However, there are some other associated aspects that constitute stronger violations of the standard economic theory of rational choice. In a couple of weeks we will discuss the Allais aradox and some related departures from expected utility theory more fully. We will relate such behavior to the shape of the indifference map in the probability triangle diagram, and discuss somewhat more general classes of preferences and some of their consequences. That said, expected utility theory remains the basic approach to the analysis of economic and financial choices and equilibria, and we will follow it also.
Lecture 11 Uncertainty
 Lecture 11 Uncertainty 1. Contingent Claims and the State-Preference Model 1) Contingent Commodities and Contingent Claims Using the simple two-good model we have developed throughout this course, think
Lecture 11 Uncertainty 1. Contingent Claims and the State-Preference Model 1) Contingent Commodities and Contingent Claims Using the simple two-good model we have developed throughout this course, think
Choice under Uncertainty
 Choice under Uncertainty Part 1: Expected Utility Function, Attitudes towards Risk, Demand for Insurance Slide 1 Choice under Uncertainty We ll analyze the underlying assumptions of expected utility theory
Choice under Uncertainty Part 1: Expected Utility Function, Attitudes towards Risk, Demand for Insurance Slide 1 Choice under Uncertainty We ll analyze the underlying assumptions of expected utility theory
1 Uncertainty and Preferences
 In this chapter, we present the theory of consumer preferences on risky outcomes. The theory is then applied to study the demand for insurance. Consider the following story. John wants to mail a package
In this chapter, we present the theory of consumer preferences on risky outcomes. The theory is then applied to study the demand for insurance. Consider the following story. John wants to mail a package
The Graphical Method: An Example
 The Graphical Method: An Example Consider the following linear program: Maximize 4x 1 +3x 2 Subject to: 2x 1 +3x 2 6 (1) 3x 1 +2x 2 3 (2) 2x 2 5 (3) 2x 1 +x 2 4 (4) x 1, x 2 0, where, for ease of reference,
The Graphical Method: An Example Consider the following linear program: Maximize 4x 1 +3x 2 Subject to: 2x 1 +3x 2 6 (1) 3x 1 +2x 2 3 (2) 2x 2 5 (3) 2x 1 +x 2 4 (4) x 1, x 2 0, where, for ease of reference,
1. Briefly explain what an indifference curve is and how it can be graphically derived.
 Chapter 2: Consumer Choice Short Answer Questions 1. Briefly explain what an indifference curve is and how it can be graphically derived. Answer: An indifference curve shows the set of consumption bundles
Chapter 2: Consumer Choice Short Answer Questions 1. Briefly explain what an indifference curve is and how it can be graphically derived. Answer: An indifference curve shows the set of consumption bundles
Managerial Economics Prof. Trupti Mishra S.J.M. School of Management Indian Institute of Technology, Bombay. Lecture - 13 Consumer Behaviour (Contd )
 (Refer Slide Time: 00:28) Managerial Economics Prof. Trupti Mishra S.J.M. School of Management Indian Institute of Technology, Bombay Lecture - 13 Consumer Behaviour (Contd ) We will continue our discussion
(Refer Slide Time: 00:28) Managerial Economics Prof. Trupti Mishra S.J.M. School of Management Indian Institute of Technology, Bombay Lecture - 13 Consumer Behaviour (Contd ) We will continue our discussion
3 Introduction to Assessing Risk
 3 Introduction to Assessing Risk Important Question. How do we assess the risk an investor faces when choosing among assets? In this discussion we examine how an investor would assess the risk associated
3 Introduction to Assessing Risk Important Question. How do we assess the risk an investor faces when choosing among assets? In this discussion we examine how an investor would assess the risk associated
Figure 1.1 Vector A and Vector F
 CHAPTER I VECTOR QUANTITIES Quantities are anything which can be measured, and stated with number. Quantities in physics are divided into two types; scalar and vector quantities. Scalar quantities have
CHAPTER I VECTOR QUANTITIES Quantities are anything which can be measured, and stated with number. Quantities in physics are divided into two types; scalar and vector quantities. Scalar quantities have
Lecture notes for Choice Under Uncertainty
 Lecture notes for Choice Under Uncertainty 1. Introduction In this lecture we examine the theory of decision-making under uncertainty and its application to the demand for insurance. The undergraduate
Lecture notes for Choice Under Uncertainty 1. Introduction In this lecture we examine the theory of decision-making under uncertainty and its application to the demand for insurance. The undergraduate
Decision Making under Uncertainty
 6.825 Techniques in Artificial Intelligence Decision Making under Uncertainty How to make one decision in the face of uncertainty Lecture 19 1 In the next two lectures, we ll look at the question of how
6.825 Techniques in Artificial Intelligence Decision Making under Uncertainty How to make one decision in the face of uncertainty Lecture 19 1 In the next two lectures, we ll look at the question of how
Expected Utility Analysis
 Expected Utility Analysis L A T E X file: expectedutility Daniel A. Graham, September 8, 2 Preferences for Probabilities We now turn to characterizing preferences for lotteries with the goal of identifying
Expected Utility Analysis L A T E X file: expectedutility Daniel A. Graham, September 8, 2 Preferences for Probabilities We now turn to characterizing preferences for lotteries with the goal of identifying
Economics 1011a: Intermediate Microeconomics
 Lecture 12: More Uncertainty Economics 1011a: Intermediate Microeconomics Lecture 12: More on Uncertainty Thursday, October 23, 2008 Last class we introduced choice under uncertainty. Today we will explore
Lecture 12: More Uncertainty Economics 1011a: Intermediate Microeconomics Lecture 12: More on Uncertainty Thursday, October 23, 2008 Last class we introduced choice under uncertainty. Today we will explore
MATH10212 Linear Algebra. Systems of Linear Equations. Definition. An n-dimensional vector is a row or a column of n numbers (or letters): a 1.
 MATH10212 Linear Algebra Textbook: D. Poole, Linear Algebra: A Modern Introduction. Thompson, 2006. ISBN 0-534-40596-7. Systems of Linear Equations Definition. An n-dimensional vector is a row or a column
MATH10212 Linear Algebra Textbook: D. Poole, Linear Algebra: A Modern Introduction. Thompson, 2006. ISBN 0-534-40596-7. Systems of Linear Equations Definition. An n-dimensional vector is a row or a column
6.254 : Game Theory with Engineering Applications Lecture 2: Strategic Form Games
 6.254 : Game Theory with Engineering Applications Lecture 2: Strategic Form Games Asu Ozdaglar MIT February 4, 2009 1 Introduction Outline Decisions, utility maximization Strategic form games Best responses
6.254 : Game Theory with Engineering Applications Lecture 2: Strategic Form Games Asu Ozdaglar MIT February 4, 2009 1 Introduction Outline Decisions, utility maximization Strategic form games Best responses
Chapter 3. The Concept of Elasticity and Consumer and Producer Surplus. Chapter Objectives. Chapter Outline
 Chapter 3 The Concept of Elasticity and Consumer and roducer Surplus Chapter Objectives After reading this chapter you should be able to Understand that elasticity, the responsiveness of quantity to changes
Chapter 3 The Concept of Elasticity and Consumer and roducer Surplus Chapter Objectives After reading this chapter you should be able to Understand that elasticity, the responsiveness of quantity to changes
6.4 Normal Distribution
 Contents 6.4 Normal Distribution....................... 381 6.4.1 Characteristics of the Normal Distribution....... 381 6.4.2 The Standardized Normal Distribution......... 385 6.4.3 Meaning of Areas under
Contents 6.4 Normal Distribution....................... 381 6.4.1 Characteristics of the Normal Distribution....... 381 6.4.2 The Standardized Normal Distribution......... 385 6.4.3 Meaning of Areas under
a 11 x 1 + a 12 x 2 + + a 1n x n = b 1 a 21 x 1 + a 22 x 2 + + a 2n x n = b 2.
 Chapter 1 LINEAR EQUATIONS 1.1 Introduction to linear equations A linear equation in n unknowns x 1, x,, x n is an equation of the form a 1 x 1 + a x + + a n x n = b, where a 1, a,..., a n, b are given
Chapter 1 LINEAR EQUATIONS 1.1 Introduction to linear equations A linear equation in n unknowns x 1, x,, x n is an equation of the form a 1 x 1 + a x + + a n x n = b, where a 1, a,..., a n, b are given
Chapter 4 Online Appendix: The Mathematics of Utility Functions
 Chapter 4 Online Appendix: The Mathematics of Utility Functions We saw in the text that utility functions and indifference curves are different ways to represent a consumer s preferences. Calculus can
Chapter 4 Online Appendix: The Mathematics of Utility Functions We saw in the text that utility functions and indifference curves are different ways to represent a consumer s preferences. Calculus can
Microeconomics Sept. 16, 2010 NOTES ON CALCULUS AND UTILITY FUNCTIONS
 DUSP 11.203 Frank Levy Microeconomics Sept. 16, 2010 NOTES ON CALCULUS AND UTILITY FUNCTIONS These notes have three purposes: 1) To explain why some simple calculus formulae are useful in understanding
DUSP 11.203 Frank Levy Microeconomics Sept. 16, 2010 NOTES ON CALCULUS AND UTILITY FUNCTIONS These notes have three purposes: 1) To explain why some simple calculus formulae are useful in understanding
Unified Lecture # 4 Vectors
 Fall 2005 Unified Lecture # 4 Vectors These notes were written by J. Peraire as a review of vectors for Dynamics 16.07. They have been adapted for Unified Engineering by R. Radovitzky. References [1] Feynmann,
Fall 2005 Unified Lecture # 4 Vectors These notes were written by J. Peraire as a review of vectors for Dynamics 16.07. They have been adapted for Unified Engineering by R. Radovitzky. References [1] Feynmann,
The Keynesian Cross. A Fixed Price Level. The Simplest Keynesian-Cross Model: Autonomous Consumption Only
 The Keynesian Cross Some instructors like to develop a more detailed macroeconomic model than is presented in the textbook. This supplemental material provides a concise description of the Keynesian-cross
The Keynesian Cross Some instructors like to develop a more detailed macroeconomic model than is presented in the textbook. This supplemental material provides a concise description of the Keynesian-cross
Unraveling versus Unraveling: A Memo on Competitive Equilibriums and Trade in Insurance Markets
 Unraveling versus Unraveling: A Memo on Competitive Equilibriums and Trade in Insurance Markets Nathaniel Hendren January, 2014 Abstract Both Akerlof (1970) and Rothschild and Stiglitz (1976) show that
Unraveling versus Unraveling: A Memo on Competitive Equilibriums and Trade in Insurance Markets Nathaniel Hendren January, 2014 Abstract Both Akerlof (1970) and Rothschild and Stiglitz (1976) show that
Insurance. Michael Peters. December 27, 2013
 Insurance Michael Peters December 27, 2013 1 Introduction In this chapter, we study a very simple model of insurance using the ideas and concepts developed in the chapter on risk aversion. You may recall
Insurance Michael Peters December 27, 2013 1 Introduction In this chapter, we study a very simple model of insurance using the ideas and concepts developed in the chapter on risk aversion. You may recall
On Lexicographic (Dictionary) Preference
 MICROECONOMICS LECTURE SUPPLEMENTS Hajime Miyazaki File Name: lexico95.usc/lexico99.dok DEPARTMENT OF ECONOMICS OHIO STATE UNIVERSITY Fall 993/994/995 Miyazaki.@osu.edu On Lexicographic (Dictionary) Preference
MICROECONOMICS LECTURE SUPPLEMENTS Hajime Miyazaki File Name: lexico95.usc/lexico99.dok DEPARTMENT OF ECONOMICS OHIO STATE UNIVERSITY Fall 993/994/995 Miyazaki.@osu.edu On Lexicographic (Dictionary) Preference
Economics 1011a: Intermediate Microeconomics
 Lecture 11: Choice Under Uncertainty Economics 1011a: Intermediate Microeconomics Lecture 11: Choice Under Uncertainty Tuesday, October 21, 2008 Last class we wrapped up consumption over time. Today we
Lecture 11: Choice Under Uncertainty Economics 1011a: Intermediate Microeconomics Lecture 11: Choice Under Uncertainty Tuesday, October 21, 2008 Last class we wrapped up consumption over time. Today we
REVIEW OF MICROECONOMICS
 ECO 352 Spring 2010 Precepts Weeks 1, 2 Feb. 1, 8 REVIEW OF MICROECONOMICS Concepts to be reviewed Budget constraint: graphical and algebraic representation Preferences, indifference curves. Utility function
ECO 352 Spring 2010 Precepts Weeks 1, 2 Feb. 1, 8 REVIEW OF MICROECONOMICS Concepts to be reviewed Budget constraint: graphical and algebraic representation Preferences, indifference curves. Utility function
Continued Fractions and the Euclidean Algorithm
 Continued Fractions and the Euclidean Algorithm Lecture notes prepared for MATH 326, Spring 997 Department of Mathematics and Statistics University at Albany William F Hammond Table of Contents Introduction
Continued Fractions and the Euclidean Algorithm Lecture notes prepared for MATH 326, Spring 997 Department of Mathematics and Statistics University at Albany William F Hammond Table of Contents Introduction
Elements of a graph. Click on the links below to jump directly to the relevant section
 Click on the links below to jump directly to the relevant section Elements of a graph Linear equations and their graphs What is slope? Slope and y-intercept in the equation of a line Comparing lines on
Click on the links below to jump directly to the relevant section Elements of a graph Linear equations and their graphs What is slope? Slope and y-intercept in the equation of a line Comparing lines on
.4 120 +.1 80 +.5 100 = 48 + 8 + 50 = 106.
 Chapter 16. Risk and Uncertainty Part A 2009, Kwan Choi Expected Value X i = outcome i, p i = probability of X i EV = pix For instance, suppose a person has an idle fund, $100, for one month, and is considering
Chapter 16. Risk and Uncertainty Part A 2009, Kwan Choi Expected Value X i = outcome i, p i = probability of X i EV = pix For instance, suppose a person has an idle fund, $100, for one month, and is considering
ECON 459 Game Theory. Lecture Notes Auctions. Luca Anderlini Spring 2015
 ECON 459 Game Theory Lecture Notes Auctions Luca Anderlini Spring 2015 These notes have been used before. If you can still spot any errors or have any suggestions for improvement, please let me know. 1
ECON 459 Game Theory Lecture Notes Auctions Luca Anderlini Spring 2015 These notes have been used before. If you can still spot any errors or have any suggestions for improvement, please let me know. 1
Copyright 2011 Casa Software Ltd. www.casaxps.com. Centre of Mass
 Centre of Mass A central theme in mathematical modelling is that of reducing complex problems to simpler, and hopefully, equivalent problems for which mathematical analysis is possible. The concept of
Centre of Mass A central theme in mathematical modelling is that of reducing complex problems to simpler, and hopefully, equivalent problems for which mathematical analysis is possible. The concept of
Review of Fundamental Mathematics
 Review of Fundamental Mathematics As explained in the Preface and in Chapter 1 of your textbook, managerial economics applies microeconomic theory to business decision making. The decision-making tools
Review of Fundamental Mathematics As explained in the Preface and in Chapter 1 of your textbook, managerial economics applies microeconomic theory to business decision making. The decision-making tools
c 2008 Je rey A. Miron We have described the constraints that a consumer faces, i.e., discussed the budget constraint.
 Lecture 2b: Utility c 2008 Je rey A. Miron Outline: 1. Introduction 2. Utility: A De nition 3. Monotonic Transformations 4. Cardinal Utility 5. Constructing a Utility Function 6. Examples of Utility Functions
Lecture 2b: Utility c 2008 Je rey A. Miron Outline: 1. Introduction 2. Utility: A De nition 3. Monotonic Transformations 4. Cardinal Utility 5. Constructing a Utility Function 6. Examples of Utility Functions
Mathematical Induction
 Mathematical Induction In logic, we often want to prove that every member of an infinite set has some feature. E.g., we would like to show: N 1 : is a number 1 : has the feature Φ ( x)(n 1 x! 1 x) How
Mathematical Induction In logic, we often want to prove that every member of an infinite set has some feature. E.g., we would like to show: N 1 : is a number 1 : has the feature Φ ( x)(n 1 x! 1 x) How
S-Parameters and Related Quantities Sam Wetterlin 10/20/09
 S-Parameters and Related Quantities Sam Wetterlin 10/20/09 Basic Concept of S-Parameters S-Parameters are a type of network parameter, based on the concept of scattering. The more familiar network parameters
S-Parameters and Related Quantities Sam Wetterlin 10/20/09 Basic Concept of S-Parameters S-Parameters are a type of network parameter, based on the concept of scattering. The more familiar network parameters
Tastes and Indifference Curves
 C H A P T E R 4 Tastes and Indifference Curves This chapter begins a -chapter treatment of tastes or what we also call preferences. In the first of these chapters, we simply investigate the basic logic
C H A P T E R 4 Tastes and Indifference Curves This chapter begins a -chapter treatment of tastes or what we also call preferences. In the first of these chapters, we simply investigate the basic logic
Chapter 3 Consumer Behavior
 Chapter 3 Consumer Behavior Read Pindyck and Rubinfeld (2013), Chapter 3 Microeconomics, 8 h Edition by R.S. Pindyck and D.L. Rubinfeld Adapted by Chairat Aemkulwat for Econ I: 2900111 1/29/2015 CHAPTER
Chapter 3 Consumer Behavior Read Pindyck and Rubinfeld (2013), Chapter 3 Microeconomics, 8 h Edition by R.S. Pindyck and D.L. Rubinfeld Adapted by Chairat Aemkulwat for Econ I: 2900111 1/29/2015 CHAPTER
Chapter 27: Taxation. 27.1: Introduction. 27.2: The Two Prices with a Tax. 27.2: The Pre-Tax Position
 Chapter 27: Taxation 27.1: Introduction We consider the effect of taxation on some good on the market for that good. We ask the questions: who pays the tax? what effect does it have on the equilibrium
Chapter 27: Taxation 27.1: Introduction We consider the effect of taxation on some good on the market for that good. We ask the questions: who pays the tax? what effect does it have on the equilibrium
Integer Operations. Overview. Grade 7 Mathematics, Quarter 1, Unit 1.1. Number of Instructional Days: 15 (1 day = 45 minutes) Essential Questions
 Grade 7 Mathematics, Quarter 1, Unit 1.1 Integer Operations Overview Number of Instructional Days: 15 (1 day = 45 minutes) Content to Be Learned Describe situations in which opposites combine to make zero.
Grade 7 Mathematics, Quarter 1, Unit 1.1 Integer Operations Overview Number of Instructional Days: 15 (1 day = 45 minutes) Content to Be Learned Describe situations in which opposites combine to make zero.
Why is Insurance Good? An Example Jon Bakija, Williams College (Revised October 2013)
 Why is Insurance Good? An Example Jon Bakija, Williams College (Revised October 2013) Introduction The United States government is, to a rough approximation, an insurance company with an army. 1 That is
Why is Insurance Good? An Example Jon Bakija, Williams College (Revised October 2013) Introduction The United States government is, to a rough approximation, an insurance company with an army. 1 That is
Chapter 25: Exchange in Insurance Markets
 Chapter 25: Exchange in Insurance Markets 25.1: Introduction In this chapter we use the techniques that we have been developing in the previous 2 chapters to discuss the trade of risk. Insurance markets
Chapter 25: Exchange in Insurance Markets 25.1: Introduction In this chapter we use the techniques that we have been developing in the previous 2 chapters to discuss the trade of risk. Insurance markets
Decision & Risk Analysis Lecture 6. Risk and Utility
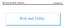 Risk and Utility Risk - Introduction Payoff Game 1 $14.50 0.5 0.5 $30 - $1 EMV 30*0.5+(-1)*0.5= 14.5 Game 2 Which game will you play? Which game is risky? $50.00 Figure 13.1 0.5 0.5 $2,000 - $1,900 EMV
Risk and Utility Risk - Introduction Payoff Game 1 $14.50 0.5 0.5 $30 - $1 EMV 30*0.5+(-1)*0.5= 14.5 Game 2 Which game will you play? Which game is risky? $50.00 Figure 13.1 0.5 0.5 $2,000 - $1,900 EMV
6.207/14.15: Networks Lecture 15: Repeated Games and Cooperation
 6.207/14.15: Networks Lecture 15: Repeated Games and Cooperation Daron Acemoglu and Asu Ozdaglar MIT November 2, 2009 1 Introduction Outline The problem of cooperation Finitely-repeated prisoner s dilemma
6.207/14.15: Networks Lecture 15: Repeated Games and Cooperation Daron Acemoglu and Asu Ozdaglar MIT November 2, 2009 1 Introduction Outline The problem of cooperation Finitely-repeated prisoner s dilemma
C H A P T E R Regular Expressions regular expression
 7 CHAPTER Regular Expressions Most programmers and other power-users of computer systems have used tools that match text patterns. You may have used a Web search engine with a pattern like travel cancun
7 CHAPTER Regular Expressions Most programmers and other power-users of computer systems have used tools that match text patterns. You may have used a Web search engine with a pattern like travel cancun
Math 4310 Handout - Quotient Vector Spaces
 Math 4310 Handout - Quotient Vector Spaces Dan Collins The textbook defines a subspace of a vector space in Chapter 4, but it avoids ever discussing the notion of a quotient space. This is understandable
Math 4310 Handout - Quotient Vector Spaces Dan Collins The textbook defines a subspace of a vector space in Chapter 4, but it avoids ever discussing the notion of a quotient space. This is understandable
CHAPTER 3 CONSUMER BEHAVIOR
 CHAPTER 3 CONSUMER BEHAVIOR EXERCISES 2. Draw the indifference curves for the following individuals preferences for two goods: hamburgers and beer. a. Al likes beer but hates hamburgers. He always prefers
CHAPTER 3 CONSUMER BEHAVIOR EXERCISES 2. Draw the indifference curves for the following individuals preferences for two goods: hamburgers and beer. a. Al likes beer but hates hamburgers. He always prefers
Linear Programming. Solving LP Models Using MS Excel, 18
 SUPPLEMENT TO CHAPTER SIX Linear Programming SUPPLEMENT OUTLINE Introduction, 2 Linear Programming Models, 2 Model Formulation, 4 Graphical Linear Programming, 5 Outline of Graphical Procedure, 5 Plotting
SUPPLEMENT TO CHAPTER SIX Linear Programming SUPPLEMENT OUTLINE Introduction, 2 Linear Programming Models, 2 Model Formulation, 4 Graphical Linear Programming, 5 Outline of Graphical Procedure, 5 Plotting
The Binomial Distribution
 The Binomial Distribution James H. Steiger November 10, 00 1 Topics for this Module 1. The Binomial Process. The Binomial Random Variable. The Binomial Distribution (a) Computing the Binomial pdf (b) Computing
The Binomial Distribution James H. Steiger November 10, 00 1 Topics for this Module 1. The Binomial Process. The Binomial Random Variable. The Binomial Distribution (a) Computing the Binomial pdf (b) Computing
Lecture L3 - Vectors, Matrices and Coordinate Transformations
 S. Widnall 16.07 Dynamics Fall 2009 Lecture notes based on J. Peraire Version 2.0 Lecture L3 - Vectors, Matrices and Coordinate Transformations By using vectors and defining appropriate operations between
S. Widnall 16.07 Dynamics Fall 2009 Lecture notes based on J. Peraire Version 2.0 Lecture L3 - Vectors, Matrices and Coordinate Transformations By using vectors and defining appropriate operations between
Demand, Supply and Elasticity
 Demand, Supply and Elasticity CHAPTER 2 OUTLINE 2.1 Demand and Supply Definitions, Determinants and Disturbances 2.2 The Market Mechanism 2.3 Changes in Market Equilibrium 2.4 Elasticities of Supply and
Demand, Supply and Elasticity CHAPTER 2 OUTLINE 2.1 Demand and Supply Definitions, Determinants and Disturbances 2.2 The Market Mechanism 2.3 Changes in Market Equilibrium 2.4 Elasticities of Supply and
Economics of Insurance
 Economics of Insurance In this last lecture, we cover most topics of Economics of Information within a single application. Through this, you will see how the differential informational assumptions allow
Economics of Insurance In this last lecture, we cover most topics of Economics of Information within a single application. Through this, you will see how the differential informational assumptions allow
Arrangements And Duality
 Arrangements And Duality 3.1 Introduction 3 Point configurations are tbe most basic structure we study in computational geometry. But what about configurations of more complicated shapes? For example,
Arrangements And Duality 3.1 Introduction 3 Point configurations are tbe most basic structure we study in computational geometry. But what about configurations of more complicated shapes? For example,
Follow links for Class Use and other Permissions. For more information send email to: permissions@pupress.princeton.edu
 COPYRIGHT NOTICE: Ariel Rubinstein: Lecture Notes in Microeconomic Theory is published by Princeton University Press and copyrighted, c 2006, by Princeton University Press. All rights reserved. No part
COPYRIGHT NOTICE: Ariel Rubinstein: Lecture Notes in Microeconomic Theory is published by Princeton University Press and copyrighted, c 2006, by Princeton University Press. All rights reserved. No part
WRITING PROOFS. Christopher Heil Georgia Institute of Technology
 WRITING PROOFS Christopher Heil Georgia Institute of Technology A theorem is just a statement of fact A proof of the theorem is a logical explanation of why the theorem is true Many theorems have this
WRITING PROOFS Christopher Heil Georgia Institute of Technology A theorem is just a statement of fact A proof of the theorem is a logical explanation of why the theorem is true Many theorems have this
2x + y = 3. Since the second equation is precisely the same as the first equation, it is enough to find x and y satisfying the system
 1. Systems of linear equations We are interested in the solutions to systems of linear equations. A linear equation is of the form 3x 5y + 2z + w = 3. The key thing is that we don t multiply the variables
1. Systems of linear equations We are interested in the solutions to systems of linear equations. A linear equation is of the form 3x 5y + 2z + w = 3. The key thing is that we don t multiply the variables
Lecture 2. Marginal Functions, Average Functions, Elasticity, the Marginal Principle, and Constrained Optimization
 Lecture 2. Marginal Functions, Average Functions, Elasticity, the Marginal Principle, and Constrained Optimization 2.1. Introduction Suppose that an economic relationship can be described by a real-valued
Lecture 2. Marginal Functions, Average Functions, Elasticity, the Marginal Principle, and Constrained Optimization 2.1. Introduction Suppose that an economic relationship can be described by a real-valued
6.3 Conditional Probability and Independence
 222 CHAPTER 6. PROBABILITY 6.3 Conditional Probability and Independence Conditional Probability Two cubical dice each have a triangle painted on one side, a circle painted on two sides and a square painted
222 CHAPTER 6. PROBABILITY 6.3 Conditional Probability and Independence Conditional Probability Two cubical dice each have a triangle painted on one side, a circle painted on two sides and a square painted
Properties of Real Numbers
 16 Chapter P Prerequisites P.2 Properties of Real Numbers What you should learn: Identify and use the basic properties of real numbers Develop and use additional properties of real numbers Why you should
16 Chapter P Prerequisites P.2 Properties of Real Numbers What you should learn: Identify and use the basic properties of real numbers Develop and use additional properties of real numbers Why you should
Mathematical Induction. Mary Barnes Sue Gordon
 Mathematics Learning Centre Mathematical Induction Mary Barnes Sue Gordon c 1987 University of Sydney Contents 1 Mathematical Induction 1 1.1 Why do we need proof by induction?.... 1 1. What is proof by
Mathematics Learning Centre Mathematical Induction Mary Barnes Sue Gordon c 1987 University of Sydney Contents 1 Mathematical Induction 1 1.1 Why do we need proof by induction?.... 1 1. What is proof by
Lies My Calculator and Computer Told Me
 Lies My Calculator and Computer Told Me 2 LIES MY CALCULATOR AND COMPUTER TOLD ME Lies My Calculator and Computer Told Me See Section.4 for a discussion of graphing calculators and computers with graphing
Lies My Calculator and Computer Told Me 2 LIES MY CALCULATOR AND COMPUTER TOLD ME Lies My Calculator and Computer Told Me See Section.4 for a discussion of graphing calculators and computers with graphing
1 if 1 x 0 1 if 0 x 1
 Chapter 3 Continuity In this chapter we begin by defining the fundamental notion of continuity for real valued functions of a single real variable. When trying to decide whether a given function is or
Chapter 3 Continuity In this chapter we begin by defining the fundamental notion of continuity for real valued functions of a single real variable. When trying to decide whether a given function is or
You Are What You Bet: Eliciting Risk Attitudes from Horse Races
 You Are What You Bet: Eliciting Risk Attitudes from Horse Races Pierre-André Chiappori, Amit Gandhi, Bernard Salanié and Francois Salanié March 14, 2008 What Do We Know About Risk Preferences? Not that
You Are What You Bet: Eliciting Risk Attitudes from Horse Races Pierre-André Chiappori, Amit Gandhi, Bernard Salanié and Francois Salanié March 14, 2008 What Do We Know About Risk Preferences? Not that
Reflection and Refraction
 Equipment Reflection and Refraction Acrylic block set, plane-concave-convex universal mirror, cork board, cork board stand, pins, flashlight, protractor, ruler, mirror worksheet, rectangular block worksheet,
Equipment Reflection and Refraction Acrylic block set, plane-concave-convex universal mirror, cork board, cork board stand, pins, flashlight, protractor, ruler, mirror worksheet, rectangular block worksheet,
Chapter 5 Uncertainty and Consumer Behavior
 Chapter 5 Uncertainty and Consumer Behavior Questions for Review 1. What does it mean to say that a person is risk averse? Why are some people likely to be risk averse while others are risk lovers? A risk-averse
Chapter 5 Uncertainty and Consumer Behavior Questions for Review 1. What does it mean to say that a person is risk averse? Why are some people likely to be risk averse while others are risk lovers? A risk-averse
WHERE DOES THE 10% CONDITION COME FROM?
 1 WHERE DOES THE 10% CONDITION COME FROM? The text has mentioned The 10% Condition (at least) twice so far: p. 407 Bernoulli trials must be independent. If that assumption is violated, it is still okay
1 WHERE DOES THE 10% CONDITION COME FROM? The text has mentioned The 10% Condition (at least) twice so far: p. 407 Bernoulli trials must be independent. If that assumption is violated, it is still okay
So let us begin our quest to find the holy grail of real analysis.
 1 Section 5.2 The Complete Ordered Field: Purpose of Section We present an axiomatic description of the real numbers as a complete ordered field. The axioms which describe the arithmetic of the real numbers
1 Section 5.2 The Complete Ordered Field: Purpose of Section We present an axiomatic description of the real numbers as a complete ordered field. The axioms which describe the arithmetic of the real numbers
Choice Under Uncertainty
 Decision Making Under Uncertainty Choice Under Uncertainty Econ 422: Investment, Capital & Finance University of ashington Summer 2006 August 15, 2006 Course Chronology: 1. Intertemporal Choice: Exchange
Decision Making Under Uncertainty Choice Under Uncertainty Econ 422: Investment, Capital & Finance University of ashington Summer 2006 August 15, 2006 Course Chronology: 1. Intertemporal Choice: Exchange
Math 3000 Section 003 Intro to Abstract Math Homework 2
 Math 3000 Section 003 Intro to Abstract Math Homework 2 Department of Mathematical and Statistical Sciences University of Colorado Denver, Spring 2012 Solutions (February 13, 2012) Please note that these
Math 3000 Section 003 Intro to Abstract Math Homework 2 Department of Mathematical and Statistical Sciences University of Colorado Denver, Spring 2012 Solutions (February 13, 2012) Please note that these
U = x 1 2. 1 x 1 4. 2 x 1 4. What are the equilibrium relative prices of the three goods? traders has members who are best off?
 Chapter 7 General Equilibrium Exercise 7. Suppose there are 00 traders in a market all of whom behave as price takers. Suppose there are three goods and the traders own initially the following quantities:
Chapter 7 General Equilibrium Exercise 7. Suppose there are 00 traders in a market all of whom behave as price takers. Suppose there are three goods and the traders own initially the following quantities:
8 Square matrices continued: Determinants
 8 Square matrices continued: Determinants 8. Introduction Determinants give us important information about square matrices, and, as we ll soon see, are essential for the computation of eigenvalues. You
8 Square matrices continued: Determinants 8. Introduction Determinants give us important information about square matrices, and, as we ll soon see, are essential for the computation of eigenvalues. You
CHAPTER 5 Round-off errors
 CHAPTER 5 Round-off errors In the two previous chapters we have seen how numbers can be represented in the binary numeral system and how this is the basis for representing numbers in computers. Since any
CHAPTER 5 Round-off errors In the two previous chapters we have seen how numbers can be represented in the binary numeral system and how this is the basis for representing numbers in computers. Since any
Chapter 4 One Dimensional Kinematics
 Chapter 4 One Dimensional Kinematics 41 Introduction 1 4 Position, Time Interval, Displacement 41 Position 4 Time Interval 43 Displacement 43 Velocity 3 431 Average Velocity 3 433 Instantaneous Velocity
Chapter 4 One Dimensional Kinematics 41 Introduction 1 4 Position, Time Interval, Displacement 41 Position 4 Time Interval 43 Displacement 43 Velocity 3 431 Average Velocity 3 433 Instantaneous Velocity
3. Mathematical Induction
 3. MATHEMATICAL INDUCTION 83 3. Mathematical Induction 3.1. First Principle of Mathematical Induction. Let P (n) be a predicate with domain of discourse (over) the natural numbers N = {0, 1,,...}. If (1)
3. MATHEMATICAL INDUCTION 83 3. Mathematical Induction 3.1. First Principle of Mathematical Induction. Let P (n) be a predicate with domain of discourse (over) the natural numbers N = {0, 1,,...}. If (1)
A New Interpretation of Information Rate
 A New Interpretation of Information Rate reproduced with permission of AT&T By J. L. Kelly, jr. (Manuscript received March 2, 956) If the input symbols to a communication channel represent the outcomes
A New Interpretation of Information Rate reproduced with permission of AT&T By J. L. Kelly, jr. (Manuscript received March 2, 956) If the input symbols to a communication channel represent the outcomes
Graph Theory Problems and Solutions
 raph Theory Problems and Solutions Tom Davis tomrdavis@earthlink.net http://www.geometer.org/mathcircles November, 005 Problems. Prove that the sum of the degrees of the vertices of any finite graph is
raph Theory Problems and Solutions Tom Davis tomrdavis@earthlink.net http://www.geometer.org/mathcircles November, 005 Problems. Prove that the sum of the degrees of the vertices of any finite graph is
In order to describe motion you need to describe the following properties.
 Chapter 2 One Dimensional Kinematics How would you describe the following motion? Ex: random 1-D path speeding up and slowing down In order to describe motion you need to describe the following properties.
Chapter 2 One Dimensional Kinematics How would you describe the following motion? Ex: random 1-D path speeding up and slowing down In order to describe motion you need to describe the following properties.
INCIDENCE-BETWEENNESS GEOMETRY
 INCIDENCE-BETWEENNESS GEOMETRY MATH 410, CSUSM. SPRING 2008. PROFESSOR AITKEN This document covers the geometry that can be developed with just the axioms related to incidence and betweenness. The full
INCIDENCE-BETWEENNESS GEOMETRY MATH 410, CSUSM. SPRING 2008. PROFESSOR AITKEN This document covers the geometry that can be developed with just the axioms related to incidence and betweenness. The full
Mathematical Induction
 Mathematical Induction (Handout March 8, 01) The Principle of Mathematical Induction provides a means to prove infinitely many statements all at once The principle is logical rather than strictly mathematical,
Mathematical Induction (Handout March 8, 01) The Principle of Mathematical Induction provides a means to prove infinitely many statements all at once The principle is logical rather than strictly mathematical,
Solving Simultaneous Equations and Matrices
 Solving Simultaneous Equations and Matrices The following represents a systematic investigation for the steps used to solve two simultaneous linear equations in two unknowns. The motivation for considering
Solving Simultaneous Equations and Matrices The following represents a systematic investigation for the steps used to solve two simultaneous linear equations in two unknowns. The motivation for considering
. In this case the leakage effect of tax increases is mitigated because some of the reduction in disposable income would have otherwise been saved.
 Chapter 4 Review Questions. Explain how an increase in government spending and an equal increase in lump sum taxes can generate an increase in equilibrium output. Under what conditions will a balanced
Chapter 4 Review Questions. Explain how an increase in government spending and an equal increase in lump sum taxes can generate an increase in equilibrium output. Under what conditions will a balanced
Decision Theory. 36.1 Rational prospecting
 36 Decision Theory Decision theory is trivial, apart from computational details (just like playing chess!). You have a choice of various actions, a. The world may be in one of many states x; which one
36 Decision Theory Decision theory is trivial, apart from computational details (just like playing chess!). You have a choice of various actions, a. The world may be in one of many states x; which one
1 Error in Euler s Method
 1 Error in Euler s Method Experience with Euler s 1 method raises some interesting questions about numerical approximations for the solutions of differential equations. 1. What determines the amount of
1 Error in Euler s Method Experience with Euler s 1 method raises some interesting questions about numerical approximations for the solutions of differential equations. 1. What determines the amount of
Cumulative Diagrams: An Example
 Cumulative Diagrams: An Example Consider Figure 1 in which the functions (t) and (t) denote, respectively, the demand rate and the service rate (or capacity ) over time at the runway system of an airport
Cumulative Diagrams: An Example Consider Figure 1 in which the functions (t) and (t) denote, respectively, the demand rate and the service rate (or capacity ) over time at the runway system of an airport
Demand. Lecture 3. August 2015. Reading: Perlo Chapter 4 1 / 58
 Demand Lecture 3 Reading: Perlo Chapter 4 August 2015 1 / 58 Introduction We saw the demand curve in chapter 2. We learned about consumer decision making in chapter 3. Now we bridge the gap between the
Demand Lecture 3 Reading: Perlo Chapter 4 August 2015 1 / 58 Introduction We saw the demand curve in chapter 2. We learned about consumer decision making in chapter 3. Now we bridge the gap between the
AK 4 SLUTSKY COMPENSATION
 AK 4 SLUTSKY COMPENSATION ECON 210 A. JOSEPH GUSE (1) (a) First calculate the demand at the original price p b = 2 b(p b,m) = 1000 20 5p b b 0 = b(2) = 40 In general m c = m+(p 1 b p0 b )b 0. If the price
AK 4 SLUTSKY COMPENSATION ECON 210 A. JOSEPH GUSE (1) (a) First calculate the demand at the original price p b = 2 b(p b,m) = 1000 20 5p b b 0 = b(2) = 40 In general m c = m+(p 1 b p0 b )b 0. If the price
CHAPTER 2 Estimating Probabilities
 CHAPTER 2 Estimating Probabilities Machine Learning Copyright c 2016. Tom M. Mitchell. All rights reserved. *DRAFT OF January 24, 2016* *PLEASE DO NOT DISTRIBUTE WITHOUT AUTHOR S PERMISSION* This is a
CHAPTER 2 Estimating Probabilities Machine Learning Copyright c 2016. Tom M. Mitchell. All rights reserved. *DRAFT OF January 24, 2016* *PLEASE DO NOT DISTRIBUTE WITHOUT AUTHOR S PERMISSION* This is a
2. Length and distance in hyperbolic geometry
 2. Length and distance in hyperbolic geometry 2.1 The upper half-plane There are several different ways of constructing hyperbolic geometry. These different constructions are called models. In this lecture
2. Length and distance in hyperbolic geometry 2.1 The upper half-plane There are several different ways of constructing hyperbolic geometry. These different constructions are called models. In this lecture
Bedford, Fowler: Statics. Chapter 4: System of Forces and Moments, Examples via TK Solver
 System of Forces and Moments Introduction The moment vector of a force vector,, with respect to a point has a magnitude equal to the product of the force magnitude, F, and the perpendicular distance from
System of Forces and Moments Introduction The moment vector of a force vector,, with respect to a point has a magnitude equal to the product of the force magnitude, F, and the perpendicular distance from
Web Appendix for Reference-Dependent Consumption Plans by Botond Kőszegi and Matthew Rabin
 Web Appendix for Reference-Dependent Consumption Plans by Botond Kőszegi and Matthew Rabin Appendix A: Modeling Rational Reference-Dependent Behavior Throughout the paper, we have used the PPE solution
Web Appendix for Reference-Dependent Consumption Plans by Botond Kőszegi and Matthew Rabin Appendix A: Modeling Rational Reference-Dependent Behavior Throughout the paper, we have used the PPE solution
Descriptive Statistics and Measurement Scales
 Descriptive Statistics 1 Descriptive Statistics and Measurement Scales Descriptive statistics are used to describe the basic features of the data in a study. They provide simple summaries about the sample
Descriptive Statistics 1 Descriptive Statistics and Measurement Scales Descriptive statistics are used to describe the basic features of the data in a study. They provide simple summaries about the sample
Math 120 Final Exam Practice Problems, Form: A
 Math 120 Final Exam Practice Problems, Form: A Name: While every attempt was made to be complete in the types of problems given below, we make no guarantees about the completeness of the problems. Specifically,
Math 120 Final Exam Practice Problems, Form: A Name: While every attempt was made to be complete in the types of problems given below, we make no guarantees about the completeness of the problems. Specifically,
Chapter 21: The Discounted Utility Model
 Chapter 21: The Discounted Utility Model 21.1: Introduction This is an important chapter in that it introduces, and explores the implications of, an empirically relevant utility function representing intertemporal
Chapter 21: The Discounted Utility Model 21.1: Introduction This is an important chapter in that it introduces, and explores the implications of, an empirically relevant utility function representing intertemporal
Elasticity Theory Basics
 G22.3033-002: Topics in Computer Graphics: Lecture #7 Geometric Modeling New York University Elasticity Theory Basics Lecture #7: 20 October 2003 Lecturer: Denis Zorin Scribe: Adrian Secord, Yotam Gingold
G22.3033-002: Topics in Computer Graphics: Lecture #7 Geometric Modeling New York University Elasticity Theory Basics Lecture #7: 20 October 2003 Lecturer: Denis Zorin Scribe: Adrian Secord, Yotam Gingold
Decision making in the presence of uncertainty II
 CS 274 Knowledge representation Lecture 23 Decision making in the presence of uncertainty II Milos Hauskrecht milos@cs.pitt.edu 5329 Sennott Square Information-gathering actions Many actions and their
CS 274 Knowledge representation Lecture 23 Decision making in the presence of uncertainty II Milos Hauskrecht milos@cs.pitt.edu 5329 Sennott Square Information-gathering actions Many actions and their
Elementary Number Theory and Methods of Proof. CSE 215, Foundations of Computer Science Stony Brook University http://www.cs.stonybrook.
 Elementary Number Theory and Methods of Proof CSE 215, Foundations of Computer Science Stony Brook University http://www.cs.stonybrook.edu/~cse215 1 Number theory Properties: 2 Properties of integers (whole
Elementary Number Theory and Methods of Proof CSE 215, Foundations of Computer Science Stony Brook University http://www.cs.stonybrook.edu/~cse215 1 Number theory Properties: 2 Properties of integers (whole
Let s explore the content and skills assessed by Heart of Algebra questions.
 Chapter 9 Heart of Algebra Heart of Algebra focuses on the mastery of linear equations, systems of linear equations, and linear functions. The ability to analyze and create linear equations, inequalities,
Chapter 9 Heart of Algebra Heart of Algebra focuses on the mastery of linear equations, systems of linear equations, and linear functions. The ability to analyze and create linear equations, inequalities,
For example, estimate the population of the United States as 3 times 10⁸ and the
 CCSS: Mathematics The Number System CCSS: Grade 8 8.NS.A. Know that there are numbers that are not rational, and approximate them by rational numbers. 8.NS.A.1. Understand informally that every number
CCSS: Mathematics The Number System CCSS: Grade 8 8.NS.A. Know that there are numbers that are not rational, and approximate them by rational numbers. 8.NS.A.1. Understand informally that every number
Lecture 13: Risk Aversion and Expected Utility
 Lecture 13: Risk Aversion and Expected Utility Uncertainty over monetary outcomes Let x denote a monetary outcome. C is a subset of the real line, i.e. [a, b]. A lottery L is a cumulative distribution
Lecture 13: Risk Aversion and Expected Utility Uncertainty over monetary outcomes Let x denote a monetary outcome. C is a subset of the real line, i.e. [a, b]. A lottery L is a cumulative distribution
The fundamental question in economics is 2. Consumer Preferences
 A Theory of Consumer Behavior Preliminaries 1. Introduction The fundamental question in economics is 2. Consumer Preferences Given limited resources, how are goods and service allocated? 1 3. Indifference
A Theory of Consumer Behavior Preliminaries 1. Introduction The fundamental question in economics is 2. Consumer Preferences Given limited resources, how are goods and service allocated? 1 3. Indifference
MATH10040 Chapter 2: Prime and relatively prime numbers
 MATH10040 Chapter 2: Prime and relatively prime numbers Recall the basic definition: 1. Prime numbers Definition 1.1. Recall that a positive integer is said to be prime if it has precisely two positive
MATH10040 Chapter 2: Prime and relatively prime numbers Recall the basic definition: 1. Prime numbers Definition 1.1. Recall that a positive integer is said to be prime if it has precisely two positive
